Appendix: Perturbative Terms of the OPE
In this appendix, we present the full expressions for the perturbative term on the OPE side of the correlation function. The non-perturbative terms require several pages to be presented, so we will not present here; they can be found in Ref. [6].
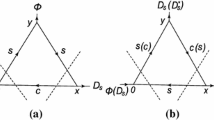
1.1 A Off-shell J/ψ
The spectral density of the perturbative contribution is related to the double discontinuity as \(\rho ^{pert}_{\mu \nu }=\frac {1}{\pi }\bar {D}\bar {D}\left [ {\Pi }^{0} \right ] \). The double discontinuity for the perturbative term in (18) is given by:
$$ \begin{array}{@{}rcl@{}} \bar{D}\bar{D}\left [ {\Pi}^{0} \right ] &=& \frac{-3}{\sqrt{\lambda}} \left [ (4\bar{E} - 4\bar{H})p^{\prime}_{\nu} p^{\prime}_{\mu} p^{\prime}_{\lambda} + (2\bar{C} - 4\bar{L})p^{\prime}_{\nu} p^{\prime}_{\mu} p_{\lambda} + (2\bar{C} + 2\bar{E} - 2\bar{B} - 4\bar{L})p^{\prime}_{\nu} p_{\mu} p^{\prime}_{\lambda} \right.\\ &&+(4\bar{C} + 2\bar{E} - 2\bar{B} - 4\bar{L}) p_{\nu} p^{\prime}_{\mu} p^{\prime}_{\lambda} + (4\bar{C} + 2\bar{D} - 2\bar{A} - 4\bar{K})p_{\nu} p_{\mu} p^{\prime}_{\lambda} + (2\bar{C} - 4\bar{K}) p^{\prime}_{\nu} p_{\mu} p_{\lambda} \\ &&+(p^{\prime}\cdot p\bar{A} - 2p^{\prime} \cdot \bar{k}\bar{A} - {m_{c}^{2}}\bar{A} + \bar{k}^{2}\bar{A} + 2\bar{F} - 4\bar{I} + p^{\prime}\cdot \bar{k} + m_{s}m_{c} - \bar{k}^{2})g_{\mu\lambda}p_{\nu} \\ &&+(p^{\prime}\cdot p\bar{B} - 2p^{\prime}\cdot \bar{k}\bar{B} - {m_{c}^{2}}\bar{B} + \bar{k}^{2}\bar{B} - 4\bar{J} - m_{s}m_{c} -p\cdot \bar{k} + \bar{k}^{2})g_{\mu\lambda}p^{\prime}_{\nu} \\ &&+(-p^{\prime}\cdot p\bar{A} + {m_{c}^{2}}\bar{A} - 2m_{s}m_{c}\bar{A} + \bar{k}^{2}\bar{A} + 2\bar{F} - 4\bar{I} + p^{\prime}\cdot \bar{k} + m_{s}m_{c} - \bar{k}^{2})g_{\nu\lambda}p_{\mu} \\ &&+(-p^{\prime}\cdot p\bar{B} + {m_{c}^{2}}\bar{B} - 2m_{s}m_{c}\bar{B} + \bar{k}^{2}\bar{B} + 2\bar{F} - 4\bar{J} + m_{s}m_{c} + p\cdot \bar{k} - \bar{k}^{2})g_{\nu\lambda}p^{\prime}_{\mu} \\ &&+(p^{\prime}\cdot p\bar{B} - {m_{c}^{2}}\bar{B} - 2p\cdot \bar{k}\bar{B} + \bar{k}^{2}\bar{B} + 2\bar{F} - 4\bar{J} + m_{s}m_{c} + p\cdot \bar{k} - \bar{k}^{2})g_{\nu\mu}p^{\prime}_{\lambda} \\ & &+(p^{\prime}\cdot p\bar{A} - {m_{c}^{2}}\bar{A} - 2p\cdot \bar{k}\bar{A} + \bar{k}^{2}\bar{A} - 4\bar{I} - p^{\prime} \cdot \bar{k} - m_{s}m_{c} + \bar{k}^{2}) g_{\nu\mu}p_{\lambda} \\ &&\left . +(2\bar{C} + 2\bar{D} - 2\bar{A} - 4\bar{K})p_{\nu} p^{\prime}_{\mu} p_{\lambda} + (4\bar{D} - 4\bar{G})p_{\nu} p_{\mu} p_{\lambda} \right ] {\Theta} \left (1 - \overline{\cos^{2}\theta} \right ), \end{array} $$
(A.1)
where we have the following definitions:
$$ \begin{array}{@{}rcl@{}} A &=& \left [ \frac{\bar{k_{0}}}{\sqrt{s}} - \frac{p^{\prime}_{0}\overline{|\vec{k}|}\overline{\cos\theta}}{|\vec{p^{\prime}}|\sqrt{s}} \right ]; \end{array} $$
(A.2)
$$ \begin{array}{@{}rcl@{}} B &=& \frac{\overline{|\vec{k}|}\overline{\cos \theta}}{|\vec{p^{\prime}}|}. \end{array} $$
(A.3)
$$ \begin{array}{@{}rcl@{}} C &=& \frac{\overline{|\vec{k}|}}{p_{0}\overline{|\vec{p^{\prime}}|}} \left [ \bar{k_{0}}\overline{\cos\theta} - \frac{\overline{|\vec{k}|}p^{\prime}_{0}}{\overline{|\vec{p^{\prime}}|}} \left (\frac{3\overline{\cos\theta}^{2} - 1}{2} \right ) \right]; \end{array} $$
(A.4)
$$ \begin{array}{@{}rcl@{}} D &=& \frac{1}{{p_{0}^{2}}} \left [ \bar{k_{0}}^{2} + \frac{\overline{|\vec{k}|}^{2}p^{\prime2}_{0}}{|\vec{p^{\prime}}|^{2}} \left (\frac{3\overline{\cos\theta}^{2} - 1}{2} \right ) -\frac{2\overline{|\vec{k}|}p^{\prime}_{0}\bar{k_{0}}\overline{\cos\theta}}{|\vec{p^{\prime}}|}\right.\\ &&- \left. \frac{\overline{|\vec{k}|}^{2}}{2}(\overline{\cos\theta}^{2} - 1) \right ]; \end{array} $$
(A.5)
$$ \begin{array}{@{}rcl@{}} E &=& \frac{\overline{|\vec{k}|}^{2}}{\overline{|\vec{p^{\prime}}|}^{2}}\left (\frac{3\overline{\cos\theta}^{2} - 1}{2} \right ); \end{array} $$
(A.6)
$$ \begin{array}{@{}rcl@{}} F &=& \frac{\overline{|\vec{k}|}^{2}(\overline{\cos\theta}^{2}- 1)}{2}. \end{array} $$
(A.7)
$$ \begin{array}{@{}rcl@{}} G &=& \frac{1}{{p^{3}_{0}}}\left (\bar{k_{0}}^{3} {\kern1.7pt}-{\kern1.7pt} H p^{\prime3}_{0} - 3Ip_{0} {\kern1.7pt}-{\kern1.7pt} 3Jp^{\prime}_{0} {\kern1.7pt}-{\kern1.7pt} 3K{p_{0}^{2}}p^{\prime}_{0} - 3Lp^{\prime2}_{0}p_{0} \right ); \end{array} $$
(A.8)
$$ \begin{array}{@{}rcl@{}} H &=& \frac{\overline{|\vec{k}|}^{3}}{|\vec{p^{\prime}}|^{3}}\left (\frac{5\overline{\cos\theta}^{3} - 3 \overline{\cos\theta}}{2}\right ); \end{array} $$
(A.9)
$$ \begin{array}{@{}rcl@{}} I &=& \frac{\overline{|\vec{k}|}^{2}(1-\overline{\cos\theta}^{2})}{2p_{0}}\left( \frac{p^{\prime}_{0}\overline{|\vec{k}|}\overline{\cos\theta}}{|\vec{p^{\prime}}|} - \bar{k_{0}} \right); \end{array} $$
(A.10)
$$ \begin{array}{@{}rcl@{}} J &=& -\frac{\overline{|\vec{k}|}^{3}\overline{\cos\theta}(1-\overline{\cos\theta}^{2})}{2|\vec{p^{\prime}}|}; \end{array} $$
(A.11)
$$ \begin{array}{@{}rcl@{}} K &=& \frac{1}{{p^{2}_{0}}}\left (\frac{\bar{k_{0}}^{2}\overline{|\vec{k}|}\overline{\cos\theta}}{|\vec{p^{\prime}}|} - p^{\prime2}_{0}H - J - 2Lp^{\prime}_{0}p_{0} \right ); \end{array} $$
(A.12)
$$ \begin{array}{@{}rcl@{}} L &=& \frac{\overline{|\vec{k}|}^{2}}{2p_{0}|\vec{p^{\prime}}|^{2}} \left [ 3\bar{k_{0}}\overline{\cos\theta}^{2} - \bar{k_{0}} - \frac{\overline{|\vec{k}|}p^{\prime}_{0}}{|\vec{p^{\prime}}|} \left (5\overline{\cos\theta}^{3} - 3 \overline{\cos\theta} \right ) \right ]. \end{array} $$
(A.13)
$$ \overline{|\vec{k}|^{2}} = {k_{0}^{2}} - {m_{s}^{2}}. $$
(A.14)
$$ \cos \theta = \frac{2p^{\prime}_{0}k_{0} + {m^{2}_{c}} - {m^{2}_{s}} - u}{2|\vec{p^{\prime}}||\vec{k}|}. $$
(A.15)
$$ k_{0} = \frac{s + {m_{s}^{2}} - {m_{c}^{2}}}{2\sqrt{s}}. $$
(A.16)
1.2 B Off-Shell \(D_{s}^{*}\)
$$ \begin{array}{@{}rcl@{}} DD[{\Pi}^{0}] &=& \frac{-3}{\sqrt{\lambda}} \left [ (4\bar{E} - 4\bar{H})p^{\prime}_{\mu} p^{\prime}_{\nu} p^{\prime}_{\lambda} + (2\bar{C} - 4\bar{L})p^{\prime}_{\mu} p^{\prime}_{\nu} p_{\lambda} + (2\bar{C} + 2\bar{E} - 2\bar{B} - 4\bar{L}) \right.\\ &&\times p^{\prime}_{\mu} p_{\nu} p^{\prime}_{\lambda} + (4\bar{C} + 2\bar{E} - 2\bar{B} - 4\bar{L}) p_{\mu} p^{\prime}_{\nu} p^{\prime}_{\lambda} + (2\bar{C} - 4\bar{K}) p^{\prime}_{\mu} p_{\nu} p_{\lambda} + (4\bar{D} - 4\bar{G})p_{\mu} p_{\nu} p_{\lambda}\\ &&+(4\bar{C} + 2\bar{D} - 2\bar{A} - 4\bar{K})p_{\mu} p_{\nu} p^{\prime}_{\lambda} + (2\bar{C} + 2\bar{D} - 2\bar{A} - 4\bar{K})p_{\mu} p^{\prime}_{\nu} p_{\lambda} + (-p^{\prime}\cdot p\bar{B} + 2\bar{F}\\ &&- 4\bar{J} + p\cdot \bar{k} )g_{\mu\lambda}p^{\prime}_{\nu} + (p^{\prime}\cdot p\bar{A} - 2p^{\prime}\cdot \bar{k}\bar{A} - 2m_{c}m_{s}\bar{A} + 2 {m_{c}^{2}}\bar{A} + 2\bar{F} - 4\bar{I} + p^{\prime}\cdot \bar{k} \\ &&+m_{c}m_{s} - {m_{c}^{2}})g_{\nu\lambda}p_{\mu} + (p^{\prime}\cdot p\bar{B} - 2p^{\prime}\cdot \bar{k}\bar{B} - 2m_{c}m_{s}\bar{B} + 2{m_{c}^{2}}\bar{B} - 4\bar{J} -p\cdot \bar{k} )g_{\nu\lambda}p^{\prime}_{\mu} \\ &&+(-p^{\prime}\cdot p\bar{A} + 2\bar{F} - 4\bar{I} + p^{\prime}\cdot \bar{k} + m_{c}m_{s} - {m_{c}^{2}})g_{\mu\lambda}p_{\nu} \\ &&+(p^{\prime} \cdotp\bar{A} - 2p\cdot \bar{k}\bar{A} - 4\bar{I} - p^{\prime} \cdot\bar{k} - m_{c}m_{s} + {m_{c}^{2}}) g_{\mu\nu}p_{\lambda} \\ &&\left. +(p^{\prime}\cdot p\bar{B} - 2p \cdot \bar{k}\bar{B} + 2\bar{F} - 4\bar{J} + p \cdot \bar{k})g_{\mu\nu}y_{\lambda} \right ] {\Theta} \left (1 - \overline{\cos^{2}\theta} \right ), \end{array} $$
(A.17)
where:
$$ \begin{array}{@{}rcl@{}} p^{\prime}\cdot p &=& \frac{s+u-t}{2};\\ p\cdot \bar{k} &=& \frac{s}{2};\\ p^{\prime} \cdot \bar{k} &=& \frac{u + {m_{c}^{2}} - {m_{s}^{2}}}{2}. \end{array} $$
The quantities \(\bar {A}-\bar {L}\), \(\bar {k}^{2}\), \(\bar {k}_{0}\), \(\overline {|\vec {k}|}^{2}\) and \(\overline {\cos \limits \theta }\) are the same ones defined for the off-shell J/ψ case.