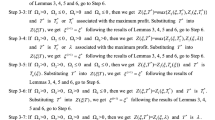An inventory model under price and stock dependent demand for controllable deterioration rate with shortages and preservation technology investment: revisited

Abstract

Mishra et al. (Ann Oper Res 254(1–2):165–190, 2017) proposed an EOQ model for a deteriorating seasonal product where demand was considered as a function of stock and selling price. Their model practiced preservation technology in order to control the deterioration rate. Further, shortages were allowed and two different scenarios, complete backordering and partial backordering were dealt with in individual cases. The model jointly optimized selling price, ordering frequency and investment in preservation technology under a profit maximization scenario. The problem they investigated depicts a practical real-life scenario, but the mathematical modelling is incorrect. Thus, the numerical results, special cases and managerial insights are debatable. Realizing the significant contribution of the model in question to the existing literature, we revisit the model to provide a corrected comprehensive version. The present model’s optimality has been proved mathematically. Further, the model has been validated using numerical analysis. Sensitivity analysis has been performed to test the robustness of the model.

Appendices

Appendix 1

$$\begin{aligned} TP(p,\alpha ,n) & = \frac{{p\beta D(p)\gamma^{2} T^{2} }}{2n} + pD(p)T - cD(p)\gamma T - \frac{{cD(p)(\lambda (\alpha ) + \beta )\gamma^{2} T^{2} }}{2n} \\ & \quad - cD(p)T(1 - \gamma ) - \frac{{hD(p)\gamma^{2} T^{2} }}{2n} + \frac{sD(p)}{n}\left( {2\gamma T^{2} - \gamma^{2} T^{2} - T^{2} } \right) - nA - \alpha T \\ \end{aligned}$$

(46)

All the second order derivatives are

$$\frac{{\partial^{2} TP}}{\partial \alpha \partial p} = \frac{{ucD'(p)\gamma^{2} T^{2} \lambda (\alpha )}}{2n} = - \frac{{ucb\gamma^{2} T^{2} \lambda (\alpha )}}{2n}$$

(47)

$$\frac{{\partial^{2} TP}}{\partial p\partial \alpha } = - \frac{{ucb\gamma^{2} T^{2} \lambda (\alpha )}}{2n}$$

(48)

$$\frac{{\partial^{2} TP}}{{\partial p^{2} }} = - \frac{{b(\beta \gamma^{2} T^{2} + 2nT)}}{n}$$

(49)

Appendix 2

Lemma 1

For a fixed number of ordering frequency n, there exist a unique $\alpha^{*}$ and $p^{*}$ if $(2D(p)\beta - cb\lambda (\alpha )) > 0$.

The second condition for sufficiency is,

$$\left( {\left( {\frac{{\partial^{2} TP}}{{\partial p^{2} }}.\frac{{\partial^{2} TP}}{{\partial \alpha^{2} }}} \right) - \left( {\frac{{\partial^{2} TP}}{\partial p\partial \alpha }.\frac{{\partial^{2} TP}}{\partial \alpha \partial p}} \right)} \right) > 0$$

(50)

All the second order derivatives are given in “Appendix 1”.

$$\begin{aligned} \left( {\left( {\frac{{\partial^{2} TP}}{{\partial p^{2} }}.\frac{{\partial^{2} TP}}{{\partial \alpha^{2} }}} \right) - \left( {\frac{{\partial^{2} TP}}{\partial p\partial \alpha }.\frac{{\partial^{2} TP}}{\partial \alpha \partial p}} \right)} \right) & = \left( {\frac{{u^{2} cD(p)\gamma^{2} T^{2} \lambda (\alpha )}}{2n}} \right)\left( {\frac{{b(\beta \gamma^{2} T^{2} + 2nT)}}{n}} \right) \\ & \quad - \left( {\frac{{ucb\gamma^{2} T^{2} \lambda (\alpha )}}{2n}} \right)^{2} \\ \end{aligned}$$

(51)

$$\left( {\left( {\frac{{\partial^{2} TP}}{{\partial p^{2} }}.\frac{{\partial^{2} TP}}{{\partial \alpha^{2} }}} \right) - \left( {\frac{{\partial^{2} TP}}{\partial p\partial \alpha }.\frac{{\partial^{2} TP}}{\partial \alpha \partial p}} \right)} \right) = \frac{{u^{2} c\gamma^{2} T^{2} \lambda (\alpha )}}{{4n^{2} }}\left( \begin{aligned} 4D(p)bnT + b\gamma^{2} T^{2} (2D(p)\beta \hfill \\ - cb\lambda (\alpha )) \hfill \\ \end{aligned} \right)$$

(52)

$${\text{Since}}\;\lambda (\alpha ) < < 1\;{\text{and}}\;b < 1 \Rightarrow (2D(p)\beta - cb\lambda (\alpha )) > 0$$

(53)

Hence

$$\left( {4D(p)bnT + b\gamma^{2} T^{2} (2D(p)\beta - cb\lambda (\alpha ))} \right) > 0$$

(54)

This implies that

$$\frac{{u^{2} c\gamma^{2} T^{2} \lambda (\alpha )}}{{4n^{2} }}\left( {4D(p)bnT + b\gamma^{2} T^{2} (2D(p)\beta - cb\lambda (\alpha ))} \right) > 0\quad \forall \, (2D(p)\beta - cb\lambda (\alpha )){ > 0}$$

(55)

Appendix 3

$$\begin{aligned} TP(p,\alpha ,n) & = \frac{{p\beta D(p)\gamma^{2} T^{2} }}{2n} + \frac{pD(p)T}{n}\left[ {\left( {1 - \gamma } \right) - \frac{\eta T}{2n}\left( {1 - \gamma } \right)^{2} } \right] - cD(p)\gamma T \\ & \quad - \frac{{cD(p)(\lambda (\alpha ) + \beta )\gamma^{2} T^{2} }}{2n} - cD(p)T(1 - \gamma ) - \frac{{cD(p)\eta T^{2} }}{2n}\left( {1 - \gamma } \right)^{2} \\ & \quad - \frac{{hD(p)\gamma^{2} T^{2} }}{2n} - \frac{sD(p)}{2n}\left( {2\gamma T^{2} - \gamma^{2} T^{2} - T^{2} } \right) - nsD(p) + \\ & \quad \frac{sD(p)\eta T}{2}\left( {1 - \gamma } \right) - \frac{{c_{1} D(p)\eta T^{2} }}{2n}\left( {1 - \gamma } \right)^{2} - nA - \alpha T \\ \end{aligned}$$

(56)

All the second order derivatives are

$$\frac{{\partial^{2} TP}}{{\partial \alpha^{2} }} = - \frac{{u^{2} cD(p)\gamma^{2} T^{2} \lambda (\alpha )}}{2n}$$

(57)

$$\frac{{\partial^{2} TP}}{\partial \alpha \partial p} = \frac{{ucD'(p)\gamma^{2} T^{2} \lambda (\alpha )}}{2n} = - \frac{{ucb\gamma^{2} T^{2} \lambda (\alpha )}}{2n}$$

(58)

$$\frac{{\partial^{2} TP}}{\partial p\partial \alpha } = - \frac{{ucb\gamma^{2} T^{2} \lambda (\alpha )}}{2n}$$

(59)

$$\frac{{\partial^{2} TP}}{{\partial p^{2} }} = - \frac{b}{2n}\left[ {\beta \gamma^{2} T^{2} + (1 - \gamma )\left[ {1 - T(1 - \gamma ))} \right]} \right]$$

(60)

Appendix 4

Lemma 2

For a fixed number of ordering frequency n, there exist a unique $\alpha^{*}$ and $p^{*}$ if $(D(p)\beta - cb\lambda (\alpha )) > 0$.

The second condition for sufficiency is

$$\left( {\left( {\frac{{\partial^{2} TP}}{{\partial p^{2} }}.\frac{{\partial^{2} TP}}{{\partial \alpha^{2} }}} \right) - \left( {\frac{{\partial^{2} TP}}{\partial p\partial \alpha }.\frac{{\partial^{2} TP}}{\partial \alpha \partial p}} \right)} \right) > 0.$$

(61)

All the second order derivatives are given in “Appendix 3”.

$$\begin{aligned} & \left( {\left( {\frac{{\partial^{2} TP}}{{\partial p^{2} }}.\frac{{\partial^{2} TP}}{{\partial \alpha^{2} }}} \right) - \left( {\frac{{\partial^{2} TP}}{\partial p\partial \alpha }.\frac{{\partial^{2} TP}}{\partial \alpha \partial p}} \right)} \right) \\ & \quad = \left( { - \frac{b}{2n}\left[ {\beta \gamma^{2} T^{2} + (1 - \gamma )\left[ {1 - T(1 - \gamma ))} \right]} \right]} \right)\left( {\frac{{b(\beta \gamma^{2} T^{2} + 2nT)}}{n}} \right) - \left( {\frac{{ucb\gamma^{2} T^{2} \lambda (\alpha )}}{2n}} \right)^{2} \\ \end{aligned}$$

(62)

$$\left( {\left( {\frac{{\partial^{2} TP}}{{\partial p^{2} }}.\frac{{\partial^{2} TP}}{{\partial \alpha^{2} }}} \right) - \left( {\frac{{\partial^{2} TP}}{\partial p\partial \alpha }.\frac{{\partial^{2} TP}}{\partial \alpha \partial p}} \right)} \right) = \frac{{u^{2} cb\gamma^{2} T^{2} \lambda (\alpha )}}{{4n^{2} }}\left( \begin{aligned} D(p)(1 - \gamma )(1 - T + T\gamma ) \hfill \\ - cb\gamma^{2} T^{2} \lambda (\alpha ) + D(p)\beta \gamma^{2} T^{2} \hfill \\ \end{aligned} \right)$$

(63)

$${\text{Since}}\;\lambda (\alpha ) < < 1\;{\text{and}}\;b < 1 \Rightarrow (D(p)\beta - cb\lambda (\alpha )) > 0$$

(64)

Hence

$$\left( {D(p)\beta \gamma^{2} T^{2} + D(p)(1 - \gamma )(1 - T + T\gamma ) - cb\gamma^{2} T^{2} \lambda (\alpha )} \right) > 0$$

(65)

This implies that

$$\frac{{u^{2} c\gamma^{2} T^{2} \lambda (\alpha )}}{{4n^{2} }}\left( \begin{aligned} D(p)\beta \gamma^{2} T^{2} + D(p)(1 - \gamma )(1 - T + T\gamma ) \hfill \\ - cb\gamma^{2} T^{2} \lambda (\alpha ) \hfill \\ \end{aligned} \right) > 0\quad \forall (D(p)\beta - cb\lambda (\alpha )) > 0$$

(66)