Skip to main content
SpringerLink
Log in

Caputo–Riesz-Feller fractional wave equation: analytic and approximate solutions and their continuation

  • Original Research
  • Published:
Journal of Applied Mathematics and Computing Aims and scope Submit manuscript

Abstract

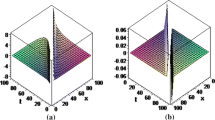
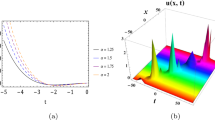
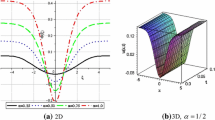
In this article a time-space fractional wave equation is studied. In the new proposed fractional model, the second-order time derivative is replaced with a fractional derivative in Caputo sense, and the second-order space derivative is replaced with a Riesz-Feller derivative defined on infinite space domain. The fundamental solution of fractional wave equation is obtained in terms of Mittag-Leffler function in two parameters, that is by using the joint Laplace–Fourier transform method. We prove the continuation of the solution of the generalized Riesz wave equation as the skewness parameter tends to zero to the one of the corresponding fractional wave equation with classical Riesz derivative, this is accomplished by using the Lebesgue’s dominated convergence theorem. The optimal homotopy analysis method (OHAM) is employed to obtain semi-analytic solution of a newly proposed initial-value fractional wave problem, considering three numerical simulations. The continuation of the optimal solution and its dependence on the fractional derivative parameters are investigated. The study reveals that the OHAM is reliable and effective in case of fractional Riesz-Feller operator represents the fractional Laplacian operator.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9

Similar content being viewed by others

References

  1. Agrawal, O.P.: Solution for a fractional diffusion-wave equation defined in a bounded domain. Nonlinear Dyn. 29(1–4), 145–155 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  2. Çelik, C., Duman, M.: Crank-Nicolson method for the fractional diffusion equation with the Riesz fractional derivative. J. Comput. Phys. 231(4), 1743–1750 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  3. Chen, W., Holm, S.: Fractional Laplacian time-space models for linear and nonlinear lossy media exhibiting arbitrary frequency power-law dependency. J. Acoust. Soc. Am. 115(4), 1424–1430 (2004)

    Article  Google Scholar 

  4. Dehghan, M., Manafian, J., Saadatmandi, A.: Solving nonlinear fractional partial differential equations using the homotopy analysis method. Numer. Methods Partial Differ. Equ. 26(2), 448–479 (2010)

    MathSciNet  MATH  Google Scholar 

  5. Elsaid, A.: The variational iteration method for solving Riesz fractional partial differential equations. Comput. Math. Appl. 60(7), 1940–1947 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  6. Elsaid, A.: Homotopy analysis method for solving a class of fractional partial differential equations. Commun. Nonlinear Sci. Numer. Simul. 16(9), 3655–3664 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  7. Elsaid, A.: Adomian polynomials: A powerful tool for iterative methods of series solution of nonlinear equations. J. Appl. Anal. Comput. 2(4), 381–394 (2012)

    MathSciNet  MATH  Google Scholar 

  8. Elsaid, A., Latif, A., Maneea, M.: Similarity solutions for multiterm time-fractional diffusion equation. Adv. Math. Phys. 2016 (2016). https://doi.org/10.1155/2016/7304659

  9. Elsaid, A., Madkour, S., Elkalla, I.: A study of a spatial fractional burger equation via the optimal homotopy analysis method. Commun. Adv. Comput. Sci. Appl. 2016(2), 73–81 (2016)

    Google Scholar 

  10. Elsaid, A., Shamseldeen, S., Madkour, S.: Iterative solution of fractional diffusion equation modelling anomalous diffusion. Appl. Appl. Math. Int. J. 11(2), 815–827 (2016)

    MathSciNet  MATH  Google Scholar 

  11. Elsaid, A., Shamseldeen, S., Madkour, S.: Semianalytic solution of space-time fractional diffusion equation. Int. J. Differ. Equ. 2016 (2016)

  12. Gorenflo, R., Luchko, Y., Mainardi, F.: Wright functions as scale-invariant solutions of the diffusion-wave equation. J. Comput. Appl. Math. 118(1), 175–191 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  13. Gorenflo, R., Mainardi, F.: Fractional diffusion processes: probability distributions and continuous time random walk. In: Processes with long-range correlations, pp. 148–166. Springer (2003)

  14. Gorenflo, R., Mainardi, F., Moretti, D., Pagnini, G., Paradisi, P.: Discrete random walk models for space-time fractional diffusion. Chem. Phys. 284(1), 521–541 (2002)

    Article  MATH  Google Scholar 

  15. Gorenflo, R., Vivoli, A.: Fully discrete random walks for space-time fractional diffusion equations. Signal Process. 83(11), 2411–2420 (2003)

    Article  MATH  Google Scholar 

  16. Herzallah, M.A., El-Sayed, A.M., Baleanu, D.: On the fractional-order diffusion-wave process. Rom. J. Phys. 55(3–4), 274–284 (2010)

    MathSciNet  MATH  Google Scholar 

  17. Huang, F., Liu, F.: The fundamental solution of the space-time fractional advection-dispersion equation. J. Appl. Math. Comput. 18(1–2), 339–350 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  18. Jafari, H., Seifi, S.: Homotopy analysis method for solving linear and nonlinear fractional diffusion-wave equation. Commun. Nonlinear Sci. Numer. Simul. 14(5), 2006–2012 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  19. Liao, S.: Beyond Perturbation: Introduction to the Homotopy Analysis Method. CRC Press, Boca Raton (2003)

    Book  Google Scholar 

  20. Liao, S.: An optimal homotopy-analysis approach for strongly nonlinear differential equations. Commun. Nonlinear Sci. Numer. Simul. 15(8), 2003–2016 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  21. Luchko, Y.: Fractional wave equation and damped waves. J. Math. Phys. 54(3), 031505 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  22. Mainardi, F., Pagnini, G., Luchko, Y.: The fundamental solution of the space-time fractional diffusion equation. Fract. Calc. Appl. Anal. 4(cond-mat/0702419), 153–192 (2007)

  23. Mainardi, F., Spada, G.: Creep, relaxation and viscosity properties for basic fractional models in rheology. Eur. Phys. J. Spec. Top. 193(1), 133–160 (2011)

    Article  Google Scholar 

  24. Metzler, R., Klafter, J.: The random walk’s guide to anomalous diffusion: a fractional dynamics approach. Phys. Rep. 339(1), 1–77 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  25. Molliq, Y., Noorani, M.S.M., Hashim, I.: Variational iteration method for fractional heat-and wave-like equations. Nonlinear Anal. Real World Appl. 10(3), 1854–1869 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  26. Momani, S.: Analytical approximate solution for fractional heat-like and wave-like equations with variable coefficients using the decomposition method. Appl. Math. Comput. 165(2), 459–472 (2005)

    MathSciNet  MATH  Google Scholar 

  27. Podlubny, I.: Fractional Differential Equations: An Introduction to Fractional Derivatives, Fractional Differential Equations, to Methods of Their Solution and Some of Their Applications, vol. 198. Academic press, Cambrifge (1998)

    MATH  Google Scholar 

  28. Ray, S.S.: A new analytical modelling for nonlocal generalized riesz fractional sine-gordon equation. J. King Saud Univ. Sci. 28(1), 48–54 (2016)

    Article  MathSciNet  Google Scholar 

  29. Saxena, R.K., Tomovski, Ž., Sandev, T.: Fractional helmholtz and fractional wave equations with riesz-feller and generalized riemann-liouville fractional derivatives. Eur. J. Pure Appl. Math. 7(3), 312–334 (2014)

    MathSciNet  MATH  Google Scholar 

  30. Schneider, W., Wyss, W.: Fractional diffusion and wave equations. J. Math. Phys. 30(1), 134–144 (1989)

    Article  MathSciNet  MATH  Google Scholar 

  31. Secer, A.: Approximate analytic solution of fractional heat-like and wave-like equations with variable coefficients using the differential transforms method. Adv. Differ. Equ. 2012(1), 1–10 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  32. Shamseldeen, S.: Approximate solution of space and time fractional higher order phase field equation. Phys. A Stat. Mech. Appl. 494, 308–316 (2018)

    Article  MathSciNet  Google Scholar 

  33. Sun, H., Chen, W., Chen, Y.: Variable-order fractional differential operators in anomalous diffusion modeling. Phys. A Stat. Mech. Appl. 388(21), 4586–4592 (2009)

    Article  Google Scholar 

  34. Yang, Q., Liu, F., Turner, I.: Numerical methods for fractional partial differential equations with Riesz space fractional derivatives. Appl. Math. Model. 34(1), 200–218 (2010)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Mathematics and Engineering Physics Department, Faculty of Engineering, Mansoura University, Mansoura, 35516, Egypt

    S. Shamseldeen, A. Elsaid & S. Madkour

Authors
  1. S. Shamseldeen
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. A. Elsaid
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. S. Madkour
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to S. Shamseldeen.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Shamseldeen, S., Elsaid, A. & Madkour, S. Caputo–Riesz-Feller fractional wave equation: analytic and approximate solutions and their continuation. J. Appl. Math. Comput. 59, 423–444 (2019). https://doi.org/10.1007/s12190-018-1186-8

Download citation

  • Received:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s12190-018-1186-8

Keywords

Mathematics Subject Classification

Search

Navigation