Are time delays always destabilizing? Revisiting the role of time delays and the Allee effect

Abstract

One of the main challenges in ecology is to determine the cause of population fluctuations. Both theoretical and empirical studies suggest that delayed density dependence instigates cyclic behavior in many populations; however, underlying mechanisms through which this occurs are often difficult to determine and may vary within species. In this paper, we consider single species population dynamics affected by the Allee effect coupled with discrete time delay. We use two different mathematical formulations of the Allee effect and analyze (both analytically and numerically) the role of time delay in different feedback mechanisms such as competition and cooperation. The bifurcation value of the delay (that results in the Hopf bifurcation) as a function of the strength of the Allee effect is obtained analytically. Interestingly, depending on the chosen delayed mechanism, even a large time delay may not necessarily lead to instability. We also show that, in case the time delay affects positive feedback (such as cooperation), the population dynamics can lead to self-organized formation of intermediate quasi-stationary states. Finally, we discuss ecological implications of our findings.

Appendices

Appendix 1: Linear stability analysis

The general approach adopted in this and the following appendix is based on the standard linearization technique coupled with basic knowledge of ODE theory used to obtain a bifurcation value of the time delay parameter, τ. Since the method in question can be easily extended and applied to all models introduced in this paper, stability analysis will be shown for one model (Eq. 5) as an illustrative example, and results for other models listed. Stability of the upper positive equilibrium is considered as it is known that the intermediate equilibrium U _∗=β is always unstable.

Suppose x is a small perturbation from the steady state U _∗=K=1:

$$ x=U-U_{*} $$

(14)

To determine equilibrium stability, we investigate the behavior of this small perturbation, whether it grows or decays, and for that purpose we linearize around the steady state, ignore higher order terms of Eq. 5, which gives:

$$ \frac{dx}{dt}=(\beta \gamma- \gamma)x_{\tau}. $$

(15)

Note that we treat the delayed variables, U(t−τ)=U _τ and x(t−τ)=x _τ , as separate variables throughout linearising. We look for solutions of form x=c e ^λt, where c is a constant and λ are the eigenvalues of the system which determine its stability. By substituting this solution form in Eq. 15, we obtain the transcendental characteristic equation:

$$ \lambda=(\beta \gamma - \gamma)e^{-\lambda \tau}, $$

(16)

for which analytical solutions are difficult to find. Nevertheless, from a stability viewpoint it is important to find whether there are any solutions with R e λ>0 which implies instability (since the perturbation grows exponentially with time). By setting λ=i ω, we assume R e λ=0 and obtain an expression for the critical value at which the multiplicative system passes through the Hopf bifurcation, below which stability prevails and above which instability occurs. Substituting into Eq. 16 and separating real and imaginary parts of the transcendental equation, we obtain the system:

$$\begin{array}{@{}rcl@{}} \ -(\beta \gamma - \gamma)\cos(\omega \tau)=0\end{array} $$

(17)

$$\begin{array}{@{}rcl@{}} \omega =-(\beta \gamma -\gamma)\sin(\omega \tau) \end{array} $$

(118)

Following some elementary calculations, we are able to find the critical value of τ:

$$ \tau_{c}=-\frac{\pi}{2(\beta \gamma- \gamma)} $$

(19)

In all subsequent analysis, the characteristic of growth rate was taken as γ=1. In the below table, we summarize the stability conditions for all other models used.

Table 2 Note that U(t)=U, U(t−τ)=U _τ , and parameter γ=1

For the delayed cooperation (Eq. 6), stability analysis confirmed that the equilibrium remains stable as:

$$ \lambda=\beta-1 $$

(20)

thus the eigenvalue is always negative for all biologically viable values of β. Loss of stability does not occur for the model incorporating time delay in the maturation term (Eq. 10), as the stability condition in this case reads:

$$ \lambda=\frac{\arccos\left(\frac{2}{1+\beta}\right)}{\sqrt{\frac{1+\beta}{2}}} $$

(21)

Since −1≤β≤0.5, $\arccos \left (\frac {2}{1+\beta }\right )$ is not defined, there are no bifurcation values, τ _c . As for the additive model incorporating time delay in direct competition (11), we were able to map all critical values, τ _c :

$$ \tau_{c}=\frac{\arccos \beta}{\sin(\arccos \beta)}, $$

(22)

with the exception of β=−1 as it is a singularity. Addition of another delayed term into direct competition results in Eq. (12) for which the stability condition reads:

$$ \tau_{c}=\frac{\arccos\left(\frac{\beta+1}{2}\right)}{\sqrt{4-(\beta+1)^{2}}} $$

(23)

The analytical bifurcation value, τ _c , for the model with two delayed terms (13) the same as in model with delayed competition (5).

Appendix 2: Loss of monotonicity analysis

Following the linearization method previously described, we obtain expressions (and subsequently values) for the time delay parameter, τ, when the system loses monotonicity, i.e., when the solution still approaches the stable equilibrium U _∗=K, but in a nonmonotonous manner (referred to as damped oscillations). We introduce the conditions of monotonicity, solving for one model, and list results for all others, as above.

We consider Eq. 5 and its corresponding characteristic equation:

$$ F(\lambda;\tau)=\lambda+e^{-\lambda \tau}(\gamma-\beta \gamma)=0 $$

(24)

In general, whether the solution is monotone or not depends on the roots of this eigenvalue equation and loss of monotonicity is associated with the total loss of all relevant real eigenvalues. Obviously, when τ=0, the eigenvalue is negative for all biologically relevant values of β, with the exception for β=−1, in which case stability of system cannot be inferred. By increasing the time delay, the eigenvalue goes complex, at a value we will denote by τ _∗. At this critical value, the two curves intersect and the following condition holds:

$$ \frac{\partial F}{\partial \lambda}(\lambda; \tau)=0 $$

(25)

On elimination of λ, using elementary calculus, one finds an explicit condition for τ:

$$ \tau_{*}=\frac{1}{e(G-\beta G)} $$

(26)

and so loss of monotonicity is predicted for τ≥τ _∗. Note, this is the only case in which we may obtain an explicit expression, as with all other models implicit expressions are presented. In the below table, we summarize all critical values, τ _∗, for which solutions lose monotonicity for all subsequent models.

Table 3 Note that U(t)=U, U(t−τ)=U _τ , and parameter γ=1

In Eq. 6, the solution remains monotonous as the eigenvalues are always negative for all applicable values of the Allee threshold (λ=β−1).

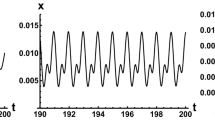
Equation 10, yielding the most interesting step-like results, clearly preserves monotonicity throughout all possible values of the time delay parameter, τ, as the monotonicity condition does not have any roots and reads:

$$ \frac{1}{\tau(\beta+1)}+e^{1+2\tau}=0 $$

(27)

For Eq. 11, the implicit expression for the critical time delay is:

$$ \frac{1}{\tau}-e^{1-\beta \tau}=0 $$

(28)

A differing monotonicity condition is obtained for a fully delayed density dependent mortality (Eq. 12):

$$ \frac{1}{2 \tau}-e^{1-(1+\beta)\tau} $$

(29)

Our two delay model yielded the same condition as in Eq. 5, as was expected and confirmed in numerical simulations.