Public verifiable measurement-only blind quantum computation based on entanglement witnesses

Abstract

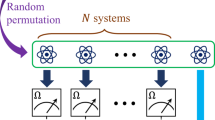
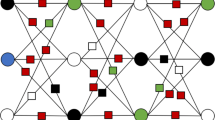
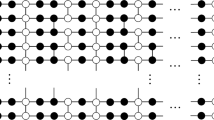
Recently, Sato et al. proposed an public verifiable blind quantum computation (BQC) protocol by inserting a third-party arbiter. However, it is not true public verifiable in a sense, because the arbiter is determined in advance and participates in the whole process. In this paper, a public verifiable protocol for measurement-only BQC is proposed. The fidelity between arbitrary states and the graph states of 2-colorable graphs is estimated by measuring the entanglement witnesses of the graph states, so as to verify the correctness of the prepared graph states. Compared with the previous protocol, our protocol is public verifiable in the true sense by allowing other random clients to execute the public verification. It also has greater advantages in the efficiency, where the number of local measurements is $O(n^3\log {n})$ and graph states’ copies is $O(n^2\log {n})$.

Appendix 1 Proof of Theorem 1

In the theorem, (1) has been proved [15] with a condition $n\ge 6$. Now, we prove (2). At first we introduce the following two probability bounds which will be used in the analysis, where $\Pr \left( \cdot \right)$ represents the event probability and $\textrm{E}\left( \cdot \right)$ represents the mathematical expectation:

• (1) Serfling’s bound[26] Given a set $Y = (Y_1,Y_2,...,Y_T )$ of T binary random variables with $Y_k\in \{0, 1\}$ and two arbitrary positive integers N and K that satisfy $T = N + K$, select K samples that are distinguished from each other independently, evenly and randomly from Y, and let $\Pi$ be the set of these samples, ${\overline{\Pi }}=Y-\Pi$, then $\forall 0<v<1$, we have

  $$\begin{aligned} \begin{aligned}&\Pr \left( {\sum \limits _{k \in {{\overline{\Pi }}} } {{Y_k}} \le {N \over K}\sum \limits _{k \in \Pi } {{Y_k}} + Nv} \right) \\&\ge 1 - \exp \left( { - {{2{v^2}N{K^2}} \over {\left( {N + K} \right) \left( {K + 1} \right) }}} \right) . \end{aligned}\end{aligned}$$

  (A1)

• (2) Azuma–Hoeffding bound[23] Given independent random variables ${\xi _1},{\xi _2}, \cdots ,{\xi _n}$ where ${\xi _i} \in \left[ {{a_i},{b_i}} \right] , i=1,2,\cdots ,n$, then $\forall t > 0$, we have

  $$\begin{aligned} \begin{aligned}&\Pr \left( {{{{\xi _1} + {\xi _2} + \cdots + {\xi _n}} \over n} - \mathrm{{E} }\left( {{{{\xi _1} + {\xi _2} + \cdots + {\xi _n}} \over n}} \right) \le t} \right) \\&\ge 1 - \exp \left( { - {{2{n^2}{t^2}} \over {\sum \limits _{i = 1}^n {\left( {{b_i} - {a_i}} \right) } }}} \right) . \end{aligned}\end{aligned}$$

  (A2)

For the first K registers selected, we denote them as $\Pi ^{(1)}$ and the rest 4K as ${\overline{\Pi }}^{(1)}$. Let $T = 5K, N=4K, Y_k = \left\{ \begin{matrix}0,M_1^{\varrho '_k} = 1 \\ 1,M_1^{\varrho '_k} = 0 \end{matrix}\right.$, where $\varrho '_k$ is the state in the k-th register in $\Pi ^{(1)}$ or ${\overline{\Pi }}^{(1)}$, then we have

$$\begin{aligned} \Pr \left( {\sum \limits _{k \in {{{{\overline{\Pi }}} }^{\left( 1 \right) }}} {{Y_k}} \le {{4K} \over K}\sum \limits _{k \in {\Pi ^{\left( 1 \right) }}} {{Y_k}} + 4Kv} \right) \ge 1 - \exp \left( { - {{2{v^2}4K{K^2}} \over {\left( {4K + K} \right) \left( {K + 1} \right) }}} \right) \nonumber \\ \end{aligned}$$

(A3)

by Eq. A1, which means if we perform the j-th measurement on the rest 4K registers, then the upper bound of the number of the registers satisfying $M_1^\varrho = 0$ (i.e., ${Y_k} = 1$) in ${{{\overline{\Pi }}} ^{\left( 1 \right) }}$ is $4\sum \limits _{k \in {\Pi ^{\left( 1 \right) }}} {{Y_k}} + 4Kv$, with the probability on the right side of Eq. A3. Similarly, for the second K registers selected, we denote them as $\Pi ^{(2)}$ and the rest 3K as ${\overline{\Pi }}^{(2)}$. Let $T = 4K, N=3K, Y_k = \left\{ \begin{matrix} {0,M_2^{\varrho '_k} = 1}\\ {1,M_2^{\varrho '_k} = 0} \end{matrix} \right.$, where $\varrho '_k$ is the state in the k-th register in $\Pi ^{(2)}$ or ${\overline{\Pi }}^{(2)}$, then we have

$$\begin{aligned} \Pr \left( {\sum \limits _{k \in {{{{\overline{\Pi }}} }^{2}}} {{Y_k}} \le {{3K} \over K}\sum \limits _{k \in {\Pi ^{2}}} {{Y_k}} + 3Kv} \right) \ge 1 - \exp \left( { - {{2{v^2}3K{K^2}} \over {\left( {3K + K} \right) \left( {K + 1} \right) }}} \right) ,\nonumber \\ \end{aligned}$$

(A4)

which means if we perform the j-th measurement on the rest 3K registers, then the upper bound of the number of the registers satisfying $M_2^\varrho = 0$ in ${{{\overline{\Pi }}} ^{\left( 2 \right) }}$ is $4\sum \limits _{k \in {\Pi ^{\left( 2 \right) }}} {{Y_k}} + 4Kv$, with the probability on the right side of Eq. A4. In the protocol, any two clients do not trust each other, and thus, it can be considered that the rest 3K registers have not been measured. If we perform the first measurement on the rest 3K registers, there will be $3K - \left( {4\sum \limits _{k \in {\Pi ^{\left( 1 \right) }}} {{Y_k}} + 4Kv} \right)$ registers satisfying $M_1^\varrho = 1$ at least, i.e.,

$$\begin{aligned} \sum \limits _{k = 1}^{3K} {M_1^{{\varrho _k}}} \ge 3K - \left( {4\sum \limits _{k \in {\Pi ^{\left( 1 \right) }}} {{Y_k}} + 4Kv} \right) . \end{aligned}$$

(A5)

Similarly, we have

$$\begin{aligned} \sum \limits _{k = 1}^{3K} {M_2^{{\varrho _k}}} \ge 3K - \left( {3\sum \limits _{k \in {\Pi ^{\left( 2 \right) }}} {{Y_k}} + 3Kv} \right) . \end{aligned}$$

(A6)

Let $n = 3K, \xi _k = M_1^\varrho$ or $M_2^\varrho$, then by Eq. A2 we have

$$\begin{aligned} \Pr \left( {{1 \over {3K}}\sum \limits _{k = 1}^{3K} {M_1^{{\varrho _k}}} - {\overline{M}} _1^\varrho \le t} \right) \ge 1 - \exp \left( { - 2 \cdot 3K{t^2}} \right) . \end{aligned}$$

(A7)

By $Tr\left( {\prod \limits _{i \in {S_1}} {{{{g_i} + I} \over 2}} \varrho } \right) = {\overline{M}} _1^\varrho$ and Eq. A5, we have

$$\begin{aligned} \begin{aligned}&\Pr \left( {Tr\left( {\prod \limits _{i \in {S_1}} {{{{g_i} + I} \over 2}} \varrho } \right) > 1 - {1 \over {3K}}\left( {4\sum \limits _{k \in {\Pi ^{\left( 1 \right) }}} {{Y_k}} + 4Kv} \right) - t} \right) \\&\ge 1 - \exp \left( { - 6K{t^2}} \right) \end{aligned}, \end{aligned}$$

(A8)

and similarly, we have

$$\begin{aligned} \begin{aligned}&\Pr \left( {Tr\left( {\prod \limits _{i \in {S_2}} {{{{g_i} + I} \over 2}} \varrho } \right) > 1 - {1 \over {3K}}\left( {3\sum \limits _{k \in {\Pi ^{\left( 2 \right) }}} {{Y_k}} + 3Kv} \right) - t} \right) \\&\ge 1 - \exp \left( { - 6K{t^2}} \right) \end{aligned}. \end{aligned}$$

(A9)

Therefore, we have

$$\begin{aligned} \begin{aligned}&F \ge {1 \over 2} - {1 \over 2}Tr\left( {{W^{\left( 2 \right) }}\varrho } \right) \\&= {1 \over 2} - {1 \over 2}Tr\left( {3I\varrho } \right) + {1 \over 2}Tr\left( {2\prod \limits _{i \in {S_1}} {{{{g_i} + I} \over 2}\varrho } } \right) + Tr\left( {2\prod \limits _{i \in {S_2}} {{{{g_i} + I} \over 2}\varrho } } \right) \\&= - 1 + 1 - {1 \over {AK}}\left( {4\sum \limits _{k \in {\Pi ^{\left( 1 \right) }}} {{Y_k}} + 4Kv} \right) - t + 1 - {1 \over {3K}}\left( {3\sum \limits _{k \in {\Pi ^{\left( 2 \right) }}} {{Y_k}} + 3Kv} \right) - t \\&= 1 - \left( {2 + {1 \over 3}} \right) v - 2t - {1 \over {3K}}\left( {4\sum \limits _{k \in {\Pi ^{\left( 1 \right) }}} {{Y_k}} + 3\sum \limits _{k \in {\Pi ^{\left( 2 \right) }}} {{Y_k}} } \right) \\&\ge 1 - \left( {2 + {1 \over 3}} \right) v - 2t - {4 \over {3K}}\left( {\sum \limits _{k \in {\Pi ^{\left( 1 \right) }}} {{Y_k}} + \sum \limits _{k \in {\Pi ^{\left( 2 \right) }}} {{Y_k}} } \right) \\&= 1 - \left( {2 + {1 \over 3}} \right) v - 2t - {4 \over {3K}}\left( {{K_1} + {K_2}} \right) . \end{aligned} \end{aligned}$$

(A10)

with a probability

$$\begin{aligned} \begin{aligned}&P \ge \left[ {1 - \exp \left( { - {{8{v^2}K} \over {5\left( {1 + {1 \over K}} \right) }}} \right) } \right] \left[ {1 - \exp \left( { - {{3{v^2}K} \over {2\left( {1 + {1 \over K}} \right) }}} \right) } \right] {\left[ {1 - \exp \left( { - 6K{t^2}} \right) } \right] ^2} \\&\ge {\left[ {1 - \exp \left( { - K{v^2}} \right) } \right] ^2}{\left[ {1 - \exp \left( { - 6K{t^2}} \right) } \right] ^2} \end{aligned}, \end{aligned}$$

(A11)

where the second inequality in Eq. A11 holds as long as $K \ge 2$. Obviously, $\sum \limits _{k \in {\Pi ^{\left( 1 \right) }}} {{Y_k}} = {K_1}$ and $\sum \limits _{k \in {\Pi ^{\left( 2 \right) }}} {{Y_k}} = {K_2}$ in Eq. A10. To make $F = \langle G |\rho |G \rangle$ is $1 - O\left( {{1 \over n}} \right)$, which is high enough, we need that $v = O\left( {{1 \over n}} \right) , t = O\left( {{1 \over n}} \right) , {4 \over {3K}}\left( {{K_1} + {K_2}} \right) \le {1 \over n}$ which leads to $F = 1 - O\left( {{1 \over n}} \right)$. Therefore, we set $v = {{\sqrt{{\lambda _2}} } \over n}, t = {{\sqrt{{\lambda _2}} } \over {\sqrt{6} n}}$, and then consider the acceptance condition ${K_1} + {K_2} \le {{3K} \over {4n}}$ in Algorithm 1; we have

$$\begin{aligned} F \ge 1 - {7 \over 3}{{\sqrt{{\lambda _2}} } \over n} - 2{{\sqrt{{\lambda _2}} } \over {\sqrt{6} n}} - {1 \over n} = 1 - {{\left( {{7 \over 3} + {2 \over {\sqrt{6} }}} \right) \sqrt{{\lambda _2}} + 1} \over n} \ge 1 - {{3.15\sqrt{{\lambda _2}} + 1} \over n}\nonumber \\ \end{aligned}$$

(A12)

with a probability

$$\begin{aligned} P \ge {\left[ {1 - \exp \left( { - {{{\lambda _2}} \over {{n^2}}}K} \right) } \right] ^4} \ge 1 - 4\exp \left( { - {{{\lambda _2}} \over {{n^2}}}K} \right) \ge 1 - 4\exp \left( { - {{{\lambda _2}} \over {{n^2}}}{n^2}\log n} \right) .\nonumber \\ \end{aligned}$$

(A13)

To make the probability $P=1 - O \left( {n ^ {-\lambda }}\right)$ for a constant $\lambda$, we set $K = \left\lceil {{n^2}\log n} \right\rceil$, then

$$\begin{aligned} P \ge 1 - 4\exp \left( { - {{{\lambda _2}} \over {{n^2}}}{n^2}\log n} \right) = 1 - 4{n^{ - {\lambda _2}}}, \end{aligned}$$

(A14)

which is high enough. The condition for above F, P both to be positive is ${\log _n}4 \le {\lambda _2} \le {{{{\left( {n - 1} \right) }^2}} \over {10}}$, where $n \ge 5$. When $n \ge 5$, we have ${n^2} > {{{{\left( {n - 1} \right) }^2}} \over {10}}$, thus ${\lambda _2} < {n^2}$, then $v = {{\sqrt{{\lambda _2}} } \over n} < 1$. Consider the condition of (1), we have $n\ge 6$.