Evolution properties of Laguerre higher order cosh-Gaussian beam propagating through fractional Fourier transform optical system

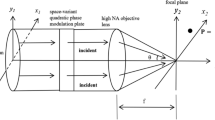
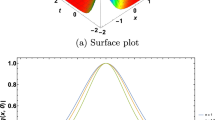
Abstract

We theoretically investigate the propagation properties of a Laguerre higher order cosh Gaussian beam (LHOchGB) in a fractional Fourier transform (FRFT) optical system. Based on the Collins formula and the expansion of the hard aperture function into a finite sum of Gaussian functions, we derive analytical expressions for a LHOchGB propagating through apertured and unapertured FRFT systems. The analysis of the evolution of the intensity distribution at the output plane has shown from the obtained expressions, using illustrative numerical examples. The results show that the intensity distribution of the considered beam propagating in FRFT is significantly influenced by the source beam parameters and the parameters of the FRFT system. It is possible to demonstrate the potential benefits of the results obtained for applications in laser beam shaping, optical trapping, and micro-particle manipulation.

Appendix: Derivation of Eqs. (8) and (13)

A) Derivation of Eq. (8)

We start by substituting Eq. (4) into Eq. (5) as:

$$ \begin{gathered} E\left( {r_{2} ,\theta_{2} ,z} \right) = \frac{{A_{0} i\left( {{{\sqrt 2 } \mathord{\left/ {\vphantom {{\sqrt 2 } {\omega_{0} }}} \right. \kern-0pt} {\omega_{0} }}} \right)^{l} }}{{2^{n} \lambda \,f\sin \varphi }}\exp \left[ { - i\frac{{\,\pi r_{2}^{2} }}{\lambda f\tan \varphi }} \right]\left[ {\int\limits_{0}^{\infty } {\left\{ {\int\limits_{0}^{2\pi } {\exp \left[ {\frac{{i2\pi \,r_{1} r_{2} }}{\lambda f\sin \varphi }\,\cos \left( {\theta_{1} - \theta_{2} } \right) + il\theta_{1} } \right]} d\theta_{1} } \right\}} } \right.\, \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\left. { \times \,r_{1}^{l + 1} L_{m}^{l} \left( {\frac{{2r_{1}^{2} }}{{\omega_{0}^{2} }}} \right)\,\exp \left[ { - \gamma r_{1}^{2} } \right]dr_{1} } \right], \hfill \\ \end{gathered} $$

(A1)

with $\gamma = \alpha + \frac{i\pi }{{\lambda f\tan \varphi }},$ and $\alpha = \frac{1}{{\omega_{0}^{2} }} - \Omega \,a_{sn}$.

Then, we recall the following azimutal integral formula (Gradshteyn and Ryzhik, 1994)

$$ \int\limits_{0}^{2\pi } {\exp \left[ {iv\theta + iz\cos \left( {\theta - \varphi } \right)} \right]d\theta } = 2\pi i^{v} \exp \left( {iv\varphi } \right)\;J_{v} \left( z \right), $$

(A2)

one obtain

$$ \begin{gathered} E\left( {r_{2} ,\theta_{2} ,z} \right) = \frac{{2\pi i^{l + 1} A_{0} \left( {{{\sqrt 2 } \mathord{\left/ {\vphantom {{\sqrt 2 } {\omega_{0} }}} \right. \kern-0pt} {\omega_{0} }}} \right)^{l} }}{{2^{n} \lambda \,f\sin \varphi }}\exp \left[ { - i\frac{{\,\pi r_{2}^{2} }}{\lambda f\tan \varphi }} \right]\, \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \times \,\int\limits_{0}^{\infty } {r_{1}^{l + 1} L_{m}^{l} \left( {\frac{{2r_{1}^{2} }}{{\omega_{0}^{2} }}} \right)\,\exp \left[ { - \gamma r_{1}^{2} } \right]J_{l} \left( {\frac{{2\pi r_{1} }}{\lambda f\sin \varphi }} \right)dr_{1} } . \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \hfill \\ \end{gathered} $$

(A3)

By recalling the following radial integral (Gradshteyn and Ryzhik, 1994)

$$ \int\limits_{0}^{\infty } {x^{v + 1} \exp \left( { - \beta x^{2} } \right)L_{n}^{v} \left( {\alpha x^{2} } \right)J_{v} \left( {xy} \right)dx} = 2^{ - v - 1} \beta^{ - v - n - 1} \left( {\beta - \alpha } \right)^{n} y^{v} \exp \left( { - \frac{{y^{2} }}{4\beta }} \right)L_{n}^{v} \left( {\frac{{\alpha y^{2} }}{{4\beta \left( {\alpha - \beta } \right)}}} \right), $$

(A4)

Eq. (A3) becomes

$$ \begin{gathered} E\left( {r_{2} ,\theta_{2} ,z} \right) = \frac{{\pi A_{0} i^{l + 1} \left( {{{\sqrt 2 } \mathord{\left/ {\vphantom {{\sqrt 2 } {\omega_{0} }}} \right. \kern-0pt} {\omega_{0} }}} \right)^{l} }}{{2^{n + l} \lambda \,f\sin \varphi }}\exp \left( { - i\frac{\,\pi }{{\lambda f\tan \varphi }}r_{2}^{2} } \right)\,\sum\limits_{s = 0}^{n} {\frac{n!}{{\left( {n - s} \right)!s!}}} e^{{il\theta_{2} }} \gamma^{ - l - m - 1} \left( {\gamma - \frac{2}{{\omega_{0}^{2} }}} \right)^{m} \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \times \left( {\frac{{2\pi r_{2} }}{\lambda f\sin \varphi }} \right)^{l} \exp \left( {\frac{{ - \pi^{2} r_{2}^{2} }}{{\lambda^{2} f^{2} \gamma \sin^{2} \varphi }}} \right)L_{m}^{l} \left( {\frac{{2\pi^{2} r_{2}^{2} }}{{\lambda^{2} f^{2} \gamma \left( {{2 \mathord{\left/ {\vphantom {2 {\omega_{0}^{2} - \gamma }}} \right. \kern-0pt} {\omega_{0}^{2} - \gamma }}} \right)\sin^{2} \varphi }}} \right). \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \hfill \\ \end{gathered} $$

(A5)

B) Derivation of Eq. (13)

In the following, for the convenience of simplification, let's assume a circular aperture is located at the output plane of the optical system. In this condition, we can express the beam at the output plane by introducing the hard-edged-aperture function in Eq. (9). Eq. (9) can written as

$$ \begin{gathered} E\left( {r_{2} ,\theta_{2} ,z} \right) = \frac{i}{\lambda \,f\sin \varphi }\int\limits_{0}^{\infty } {A_{p} \left( {r_{1} } \right)E\left( {r_{1} ,\theta_{1} } \right)} \,\exp \left[ { - i\frac{\,\pi }{{\lambda f\tan \varphi }}\left( {r_{1}^{2} + r_{2}^{2} } \right)} \right]r_{1} dr_{1} \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \times \,\int\limits_{0}^{2\pi } {\exp \left[ {\frac{{i2\pi \,r_{1} r_{2} }}{\lambda f\sin \varphi }\,\cos \left( {\theta_{1} - \theta_{2} } \right)} \right]} d\theta_{1} , \hfill \\ \end{gathered} $$

(B1)

and then, substituting Eq. (4) into Eq. (B1) as

$$ \begin{gathered} E\left( {r_{2} ,\theta_{2} ,z} \right) = \frac{{A_{0} \left( {{{\sqrt 2 } \mathord{\left/ {\vphantom {{\sqrt 2 } {\omega_{0} }}} \right. \kern-0pt} {\omega_{0} }}} \right)^{l} }}{{2^{n} \lambda \,f\sin \varphi }}\exp \left[ { - i\frac{{\,\pi r_{2}^{2} }}{\lambda f\tan \varphi }} \right]\left[ {\int\limits_{0}^{\infty } {\left\{ {\int\limits_{0}^{2\pi } {\exp \left[ {\frac{{i2\pi \,r_{1} r_{2} }}{\lambda f\sin \varphi }\,\cos \left( {\theta_{1} - \theta_{2} } \right) + il\theta_{1} } \right]} d\theta_{1} } \right\}} } \right]\, \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\left. { \times \,r_{1}^{l + 1} L_{m}^{l} \left( {\frac{{2r_{1}^{2} }}{{\omega_{0}^{2} }}} \right)\,\exp \left[ { - \gamma_{h} r_{1}^{2} } \right]dr_{1} } \right], \hfill \\ \end{gathered} $$

(B2)

where.

$\gamma_{h} = \alpha + \frac{i\pi }{{\lambda f\tan \varphi }} + \frac{{B_{h} }}{{\beta^{2} \omega_{0}^{2} }},$ and $\beta = {a \mathord{\left/ {\vphantom {a {\omega_{0} }}} \right. \kern-0pt} {\omega_{0} }},$

then, we recall the above azimutal and radial integrals of Eqs. (A2) and (A4) respectively (Gradshteyn and Ryzhik, 1994), one obtains

$$ \begin{gathered} E\left( {r_{2} ,\theta ,z} \right) = \frac{{\pi A_{0} i^{l + 1} \left( {{{\sqrt 2 } \mathord{\left/ {\vphantom {{\sqrt 2 } {\omega_{0} }}} \right. \kern-0pt} {\omega_{0} }}} \right)^{l} }}{{2^{n + l} \lambda \,f\sin \varphi }}\exp \left( { - i\frac{\,\pi }{{\lambda f\tan \varphi }}r_{2}^{2} } \right)\,\sum\limits_{s = 1}^{n} {\frac{n!}{{\left( {n - s} \right)!s!}}} \sum\limits_{h = 1}^{M} {A_{h} } \gamma_{h}^{ - l - m - 1} \left( {\gamma_{h} - \frac{2}{{\omega_{0}^{2} }}} \right)^{m} \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \times \,\,\left( {\frac{2\pi r}{{\lambda f\sin \varphi }}} \right)^{m} \exp \left( {\frac{{ - \pi^{2} r^{2} }}{{\lambda^{2} f^{2} \gamma_{h} \sin^{2} \varphi }}} \right)L_{m}^{l} \left( {\frac{{4\pi^{2} r^{2} }}{{\gamma_{h} \lambda^{2} f^{2} \sin^{2} \varphi \left( {\frac{2}{{\omega_{0}^{2} }} - \gamma_{h} } \right)}}} \right). \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \hfill \\ \end{gathered} $$

(B3)