Sensitivity analysis of the rotor-bearing system with fractional power nonlinearity using multicomplex variable derivation

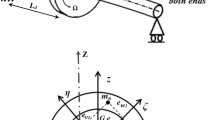
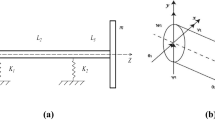
Abstract

In the computation of dynamic response sensitivity for rotor-bearing systems using the multicomplex variable derivation method, the presence of nonlinearity, particularly “fractional power” nonlinearity, in the forces generated at the supports may introduce rounding errors, potentially destabilizing the sensitivity calculation results. To address this issue, this paper proposes an enhanced method for sensitivity calculation using multicomplex variable derivation method. The approach leverages De Moivre’s theorem to conduct recursive operations on multicomplex numbers, thereby circumventing the rounding errors associated with the “fractional power” nonlinearity. This method facilitates the simultaneous calculation of sensitivity for each order and hybrid sensitivity. Subsequently, the viability of the proposed method is substantiated through a nonlinear single disk rotor system and a simulated gas generator rotor system. The results demonstrate that the proposed method maintains high accuracy and stability in dynamic response sensitivity computations even in the presence of “fractional power” nonlinearity.

Appendices

Appendix A: Finite difference methods and multicomplex variable derivation method

According to the theory of finite difference methods, the function f(x) is Taylor-expanded at x = x₀ and made such that x = x₀ + h, where h is a sufficiently small regenerative step:

$$ f(x_{0} + h) = f(x_{0} ) + hf^{\prime}(x_{0} ) + h^{2} \frac{{f^{\prime\prime}(x_{0} )}}{2!} + \ldots + h^{n} \frac{{f^{(n)} (x_{0} )}}{n!} + O(h^{n + 1} ). $$

(21)

$$ f^{\prime}(x_{0} ) \approx \frac{{f(x_{0} + h) - f(x_{0} )}}{h} $$

(22)

Further generalization to multicomplex domains still Taylor unfolds at x = x₀ and adds the imaginary part up to the nth order individual after x₀:

$$ f\left( {x_{0} + i_{1} h + i_{2} h + ... + i_{n} h} \right) = f\left( {x_{0} } \right) + \left( {i_{1} h + i_{2} h + ... + i_{n} h} \right)f^{\prime } \left( {x_{0} } \right){\text{ }} + \left( {i_{1} h + i_{2} h + ... + i_{n} h} \right)^{2} \frac{{f^{{\prime \prime }} (x_{0} )}}{{2!}} + \ldots + \left( {i_{1} h + i_{2} h + ... + i_{n} h} \right)^{n} \frac{{f^{{\left( n \right)}} \left( {x_{0} } \right)}}{{n!}} + O\left( {h^{{n + 1}} } \right) $$

(23)

Continuing the derivation of the above equation can be obtained according to the rules of arithmetic of multicomplex numbers:

$$ f^{(n)} (x_{0} ) = \frac{{Im_{1...n} [f(x_{0} + i_{1} h + i_{2} h + ... + i_{n} h)]}}{{h^{n} }} + O(h^{2} ). $$

(24)

Further:

$$ f^{(n)} (x) \approx \frac{{Im_{1...n} [f(x + i_{1} h + i_{2} h + ... + i_{n} h)]}}{{h^{n} }} $$

(25)

where Im_1…n denotes taking the multicomplex parts i₁i₂…i_n.

For multiple mixed derivatives with different parameters, they can also be computed by extracting the corresponding imaginary part of the corresponding derivative order, e.g.:

$$ \frac{{\partial ^{n} f\left( {x,y,z} \right)}}{{\partial x^{j} \partial y^{k} \partial z^{{\left( {n - j - k} \right)}} }} \approx \frac{{Im_{{1...n}} \left[ {f\left( {x + \left( {i_{1} + ... + i_{j} } \right)h_{a} ,y + \left( {i_{{j + 1}} + ... + i_{k} } \right)h_{b} ,z + \left( {i_{{k + 1}} + ... + i_{n} } \right)h_{c} } \right)} \right]}}{{h_{a}^{j} h_{b}^{k} h_{c}^{{\left( {n - j - k} \right)}} }} $$

(26)

Appendix B: Multicomplex domain complex numbers and cauchy riemann matrices

A complex number is shaped like x₁ + x₂i and consists of a real part x₁ and an imaginary part x₂. x₁, x₂ are both real numbers.

$$ C^{1} : \, = \left\{ {\left. {X = x_{1} + x_{2} i} \right|x_{1} , \, x_{2} \in R} \right\} $$

(27)

where C¹ denotes the set of complex numbers, R denotes the set of real numbers, i is the imaginary unit, and i² =  − 1.

Expanding the concept of complex numbers by adding more than one imaginary unit i_n(n = 1,2,3…), to the real numbers, where i_ji_k = i_ki_j,(j,k = 1,2,3…), to expand the space of complex numbers to a higher order. For example, the double complex numbers are defined as:

$$ \begin{gathered} C^{2} = \left\{ {X = a + bi_{2} \left| {a,b \in C^{1} } \right.} \right\} \hfill \\ = \left\{ {X = (x_{1} + x_{2} i_{1} ) + (x_{3} + x_{4} i_{1} )i_{2} = x_{1} + x_{2} i_{1} + x_{3} i_{2} + x_{4} i_{1} i_{2} \left| {x_{1} ,x_{2} ,x_{3} ,x_{4} \in R} \right.} \right\} \hfill \\ \end{gathered} $$

(28)

where i₂ is another imaginary unit different from i₁, a and b are single complex numbers.

Similarly, continuing to repeat the process introduced in Eq. (28), one can define a poly complex number with three or more imaginary units, e.g.:

$$ \begin{aligned} C^{3} & = \left\{ {X = \alpha + \beta i_{3} \left| {\alpha ,\beta \in C^{2} } \right.} \right\} \\ & = \left\{ {X = a + bi_{2} + ci_{2} + di_{2} i_{3} \left| {a,b,c,d \in C^{1} } \right.} \right\} \\ & = \left\{ {\begin{array}{*{20}c} {X = x_{1} + x_{2} i_{1} + x_{3} i_{2} + x_{4} i_{1} i_{2} + \quad \quad \quad \quad \quad \quad \quad \quad \quad \quad \quad } \\ {x_{5} i_{3} + x_{6} i_{1} i_{3} + x_{7} i_{2} i_{3} + x_{8} i_{1} i_{2} i_{3} \left| {x_{1} ,x_{2} ,x_{3} ,x_{4} ,x_{5} ,x_{6} ,x_{7} ,x_{8} \in R} \right.} \\ \end{array} } \right\} \\ \end{aligned} $$

(29)

The composition of the set of multicomplex numbers Cⁿ and the recurrence relation between the set of multicomplex numbers and the set of real numbers can be visualized in Fig. 

Fig. 12

Multicomplex expression form

12.

In addition, each poly complex number has a real matrix corresponding to it, called the Cauchy-Riemann matrix, and the arithmetic operations on poly complex numbers are also equivalent to matrix operations.

For example, for a single complex number a = x₁ + x₂i, to separate its real part from its imaginary part, the equivalent form of the complex number can be obtained in the form of a real matrix, which is represented as a 2 × 2 matrix:

$$ \{ \left. {a = x_{1} + x_{2} i} \right|x_{1} ,x_{2} \in R\} \leftrightarrow \{ \left[ {\begin{array}{*{20}c} {x_{1} } & { - x_{2} } \\ {x_{2} } & {x_{1} } \\ \end{array} } \right]\left| {x_{1} ,x_{2} \in R} \right.\} $$

(30)

According to the relationship between the various complex number domains of a poly complex number presented in Fig. 12, a number α in a complex domain can be expanded into a singly complex number domain, and then, by repeating the process of Eq. (30) twice, it can be expressed as a 4 × 4 matrix, viz:

$$ \begin{aligned} \alpha & = \left\{ {a + bi\left| {a,b \in C^{1} } \right.} \right\} \\ & \leftrightarrow \left\{ {x_{1} + x_{2} i_{1} + x_{3} i_{2} + x_{4} i_{1} i_{2} \left| {x_{1} ,x_{2} ,x_{3} ,x_{4} \in R} \right.} \right\} \\ & \leftrightarrow \left\{ {\left[ {\begin{array}{*{20}c} a & { - b} \\ b & a \\ \end{array} } \right]\left| {a,b} \right. \in C^{1} } \right\} \\ & \leftrightarrow \left\{ {\left[ {\begin{array}{*{20}c} {x_{1} } & { - x_{2} } & { - x_{3} } & {x_{4} } \\ {x_{2} } & {x_{1} } & { - x_{4} } & { - x_{3} } \\ {x_{3} } & { - x_{4} } & {x_{1} } & { - x_{2} } \\ {x_{4} } & {x_{3} } & {x_{2} } & {x_{1} } \\ \end{array} } \right]\left| {x_{1} ,x_{2} ,x_{3} ,x_{4} \in R} \right.} \right\} \\ \end{aligned} $$

(31)

And for the poly complex numbers in the nth order complex domain, the process of converting into real matrices is the same, both repeating the same above process until the poly complex numbers in the nth order complex domain within Cⁿ are expanded into a 2ⁿ × 2ⁿ matrix of real numbers.

Appendix C: direct derivation method for calculating sensitivity of nonlinear rotor support system

The direct derivation method is a method for calculating the sensitivity by directly deriving the differential equation of motion concerning parameters. This method can directly derive the differential equation of nonlinear dynamic response sensitivity calculation. Then the Newmark-beta and Newton–Raphson methods are used to solve the numerical solution. Then the accurate value of the system’s nonlinear dynamic response parameter sensitivity can be effectively solved.

In the nonlinear rotor-bearing system, the motion equation of the system is shown in Eq. (1). The partial derivative of the parameter θ in F_nl is obtained on both sides of the system.

$$ {\varvec{M}}\frac{{\partial \ddot{\varvec{x}}}}{\partial \theta } + ({\varvec{C}} + \omega {\varvec{G}})\frac{{\partial \dot{\varvec{x}}}}{\partial \theta } + {\varvec{K}}\frac{{\partial {\varvec{x}}}}{\partial \theta } = \frac{{\partial {\varvec{F}}_{{{\varvec{nl}}}} }}{{\partial {\varvec{x}}}}\frac{{\partial {\varvec{x}}}}{\partial \theta } + \frac{{\partial {\varvec{F}}_{{{\varvec{nl}}}} }}{{\partial \dot{\varvec{x}}}}\frac{{\partial \dot{\varvec{x}}}}{\partial \theta } + \frac{{\partial {\varvec{F}}_{{{\varvec{nl}}}} }}{\partial \theta } $$

(32)

Both sides of Eq. (32) are obtained by simultaneously taking partial derivatives concerning the parameter θ in F_nl:

$$ \begin{gathered} {\varvec{M}}\frac{{\partial^{2} \ddot{\varvec{x}}}}{{\partial \theta^{2} }} + ({\varvec{C}} + \omega {\varvec{G}})\frac{{\partial^{2} \dot{\varvec{x}}}}{{\partial \theta^{2} }} + {\varvec{K}}\frac{{\partial^{2} {\varvec{x}}}}{{\partial \theta^{2} }} = \frac{{\partial^{2} {\varvec{F}}_{{{\varvec{nl}}}} }}{{\partial {\varvec{x}}^{2} }}(\frac{{\partial {\varvec{x}}}}{\partial \theta })^{2} + \frac{{\partial^{2} {\varvec{F}}_{{{\varvec{nl}}}} }}{{\partial {\varvec{x}}\partial \theta }}\frac{{\partial {\varvec{x}}}}{\partial \theta } + \frac{{\partial {\varvec{F}}_{{{\varvec{nl}}}} }}{\partial \theta }\frac{{\partial^{2} {\varvec{x}}}}{{\partial \theta^{2} }} \hfill \\ + \frac{{\partial^{2} {\varvec{F}}_{{{\varvec{nl}}}} }}{{\partial \dot{\varvec{x}}^{2} }}(\frac{{\partial \dot{\varvec{x}}}}{\partial \theta })^{2} + \frac{{\partial^{2} {\varvec{F}}_{{{\varvec{nl}}}} }}{{\partial \dot{\varvec{x}}\partial \theta }}\frac{{\partial \dot{\varvec{x}}}}{\partial \theta } + \frac{{\partial {\varvec{F}}_{{{\varvec{nl}}}} }}{\partial \theta }\frac{{\partial^{2} \dot{\varvec{x}}}}{{\partial \theta^{2} }} + \frac{{\partial^{2} {\varvec{F}}_{{{\varvec{nl}}}} }}{{\partial {\varvec{x}}\partial \theta }}\frac{\partial x}{{\partial \theta }} + \frac{{\partial^{2} {\varvec{F}}_{{{\varvec{nl}}}} }}{{\partial \dot{\varvec{x}}\partial \theta }}\frac{{\partial \dot{\varvec{x}}}}{\partial \theta } + \frac{{\partial^{2} {\varvec{F}}_{{{\varvec{nl}}}} }}{{\partial \theta^{2} }} \hfill \\ \end{gathered} $$

(33)

Equation (32) is the first-order sensitivity equation, and Eq. (33) is the second-order sensitivity equation. The Newmark-beta method combined with the Newton–Raphson method can solve the two equations numerically.