Influence of simultaneous time-varying bearing and tooth mesh stiffness fluctuations on spur gear pair vibration

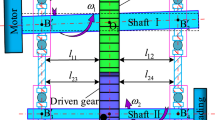
Abstract

This work investigates the influence of time-varying roller bearing stiffness on gear-bearing system vibration. An isolated bearing model established by a finite element/contact mechanics approach is introduced to analyze the variation of bearing stiffness due to the continuous change of roller circumferential locations. Then, dynamic gear-bearing models with constant and time-varying bearing stiffness are built by analytical and finite element methods. Comparisons are conducted among the dynamic response of the different gear-bearing models to verify their accuracy and to indicate the special features introduced by the time-varying bearing stiffness. Additional resonances (or parametric instabilities) are caused by the time-varying bearing stiffness, and modulation between the mesh frequency and the bearing ball pass frequency is observed in the dynamic response. Theoretical explanations of the parametric instabilities of the system with simultaneous mesh and bearing stiffness fluctuations are derived using perturbation analysis and verified with Floquet theory.

Appendices

A Instability region boundaries: individual excitation

• 1. $s_1{\varOmega _\mathrm{m}} \approx 2\omega _2$

  $$\begin{aligned} {\varOmega _\mathrm{m}}= & {} \frac{2}{s_1} \left( \omega _2 \pm \varepsilon {\frac{{\sqrt{{\alpha ^2} + {\beta ^2}} }}{{2{\omega _2}}}}\right) . \end{aligned}$$

  (57)
  $$\begin{aligned} \alpha= & {} {K_{22}^{m} {b_{{s_1}}^{m}} }, \quad \beta = -{ K_{22}^{m} { a_{{s_1}}^{m}} }. \end{aligned}$$

  (58)

• 2. $s_2{\varOmega _\mathrm{b}} \approx 2\omega _1$

  $$\begin{aligned} {\varOmega _\mathrm{m}}= & {} \frac{2}{s_2 R} \left( \omega _1 \pm \varepsilon {\frac{{\sqrt{{\alpha ^2} + {\beta ^2}} }}{{2{\omega _1}}}}\right) . \end{aligned}$$

  (59)
  $$\begin{aligned} \alpha= & {} {K_{11}^{b} {b_{{s_2}}^{b}} }, \quad \beta = -{ K_{11}^{b} { a_{{s_2}}^{b}} }. \end{aligned}$$

  (60)

• 3. $s_2{\varOmega _\mathrm{b}} \approx 2\omega _2$

  $$\begin{aligned} {\varOmega _\mathrm{m}}= & {} \frac{2}{s_2 R} \left( \omega _2 \pm \varepsilon {\frac{{\sqrt{{\alpha ^2} + {\beta ^2}} }}{{2{\omega _2}}}}\right) . \end{aligned}$$

  (61)
  $$\begin{aligned} \alpha= & {} {K_{22}^{b} {b_{{s_2}}^{b}} }, \quad \beta = -{ K_{22}^{b} { a_{{s_2}}^{b}} }. \end{aligned}$$

  (62)

• 4. $s_2{\varOmega _\mathrm{b}} \approx \omega _1 + \omega _2$

  $$\begin{aligned} {\varOmega _\mathrm{m}}= & {} \frac{1}{s_2 R} \left( \omega _1 + \omega _2 \pm \varepsilon \sqrt{\frac{{{\alpha _1}{\alpha _2} + {\beta _1}{\beta _2}}}{{{\omega _1}{\omega _2}}}}\right) . \nonumber \\ \end{aligned}$$

  (63)
  $$\begin{aligned} \alpha _1= & {} {K_{21}^{b} {b_{{s_2}}^{b}} }, \quad \beta _1 = - { K_{21}^{b} { a_{{s_2}}^{b}} }; \end{aligned}$$

  (64)
  $$\begin{aligned} \alpha _2= & {} {K_{12}^{b} {b_{{s_2}}^{b}} } , \quad \beta _2 = - { K_{12}^{b} { a_{{s_2}}^{b}} }. \end{aligned}$$

  (65)

B Instability region boundaries: coupled excitation

• 1. $s_1{\varOmega _\mathrm{m}} - s_2{\varOmega _\mathrm{b}} \approx 2\omega _2$

  $$\begin{aligned} {\varOmega _\mathrm{m}}= & {} \frac{2R}{s_1 R - s_2} \left[ \omega _2 + \frac{{2\psi \pm \sqrt{({\alpha ^2} + {\beta ^2})} }}{2 \omega _2} \varepsilon ^2 \right] , \nonumber \\\end{aligned}$$

  (66)
  $$\begin{aligned} \psi= & {} \psi _\mathrm{m} + \psi _\mathrm{b}, \end{aligned}$$

  (67)
  $$\begin{aligned} \psi _\mathrm{m}= & {} \sum \limits _{t = 1}^2 \frac{{K_{2t}^mK_{t2}^m}}{2}\left[ \frac{{{{(a_{{s_1}}^m)}^2} + {{(b_{{s_1}}^m)}^2}}}{{{{({\omega _2} + {s_1}R{\hat{\omega }} )}^2} - \omega _t^2}} \nonumber \right. \\&\left. + \frac{{{{(a_{{s_1}}^m)}^2} + {{(b_{{s_1}}^m)}^2}}}{{{{({\omega _2} - {s_1}R{\hat{\omega }} )}^2} - \omega _t^2}} \right] , \end{aligned}$$

  (68)
  $$\begin{aligned} \psi _\mathrm{b}= & {} \sum \limits _{t = 1}^2 \frac{{K_{2t}^bK_{t2}^b}}{2}\left[ \frac{{{{(a_{{s_2}}^b)}^2} + {{(b_{{s_2}}^b)}^2}}}{{{{({\omega _2} + {s_2}{\hat{\omega }} )}^2} - \omega _t^2}} \nonumber \right. \\&\left. + \frac{{{(a_{{s_2}}^b)}^2} + {{(b_{{s_2}}^b)}^2}}{{{{({\omega _2} - {s_2}{\hat{\omega }} )}^2} - \omega _t^2}} \right] . \end{aligned}$$

  (69)
  $$\begin{aligned} \alpha= & {} \sum \limits _{t = 1}^2 \left[ \frac{{K_{2t}^mK_{t2}^b(a_{{s_2}}^ba_{{s_1}}^m + b_{{s_2}}^bb_{{s_1}}^m)}}{{{{({\omega _2} + {s_2}{\hat{\omega }} )}^2} - \omega _t^2}} \nonumber \right. \\&\left. + \frac{{K_{2t}^bK_{t2}^m(a_{{s_1}}^ma_{{s_2}}^b + b_{{s_1}}^mb_{{s_2}}^b)}}{{{{({\omega _2} - {s_1}R{\hat{\omega }} )}^2} - \omega _t^2}} \right] , \end{aligned}$$

  (70)
  $$\begin{aligned} \beta= & {} \sum \limits _{t = 1}^2 \left[ \frac{{K_{2t}^mK_{t2}^b(a_{{s_2}}^bb_{{s_1}}^m - a_{{s_1}}^mb_{{s_2}}^b)}}{{{{({\omega _2} + {s_2}{\hat{\omega }} )}^2} - \omega _t^2}} \nonumber \right. \\&\left. + \frac{{K_{2t}^bK_{t2}^m(a_{{s_2}}^bb_{{s_1}}^m - a_{{s_1}}^mb_{{s_2}}^b)}}{{{{({\omega _2} - {s_1}R{\hat{\omega }} )}^2} - \omega _t^2}} \right] , \end{aligned}$$

  (71)
  $$\begin{aligned} {\hat{\omega }}= & {} \frac{2\omega _2}{s_1 R -s_2} . \end{aligned}$$

  (72)

• 2. $s_1{\varOmega _\mathrm{m}} + s_2{\varOmega _\mathrm{b}} \approx 2\omega _1$

  $$\begin{aligned} {\varOmega _\mathrm{m}}= & {} \frac{2R}{s_1 R + s_2} \left[ \omega _1 + \frac{{2\psi \pm \sqrt{({\alpha ^2} + {\beta ^2})} }}{2 \omega _1} \varepsilon ^2 \right] , \nonumber \\ \end{aligned}$$

  (73)
  $$\begin{aligned} \psi= & {} \psi _\mathrm{m} + \psi _\mathrm{b}, \end{aligned}$$

  (74)
  $$\begin{aligned} \psi _\mathrm{m}= & {} \sum \limits _{t = 1}^2 \frac{{K_{1t}^mK_{t1}^m}}{2}\left[ \frac{{{{(a_{{s_1}}^m)}^2} + {{(b_{{s_1}}^m)}^2}}}{{{{({\omega _1} + {s_1}R{\hat{\omega }} )}^2} - \omega _t^2}} \nonumber \right. \\&\left. + \frac{{{{(a_{{s_1}}^m)}^2} + {{(b_{{s_1}}^m)}^2}}}{{{{({\omega _1} - {s_1}R{\hat{\omega }} )}^2} - \omega _t^2}} \right] , \end{aligned}$$

  (75)
  $$\begin{aligned} \psi _\mathrm{b}= & {} \sum \limits _{t = 1}^2 \frac{{K_{1t}^bK_{t1}^b}}{2}\left[ \frac{{{{(a_{{s_2}}^b)}^2} + {{(b_{{s_2}}^b)}^2}}}{{{{({\omega _1} - {s_2}{\hat{\omega }} )}^2} - \omega _t^2}} \nonumber \right. \\&\left. + \frac{{{(a_{{s_2}}^b)}^2} + {{(b_{{s_2}}^b)}^2}}{{{{({\omega _1} + {s_2}{\hat{\omega }} )}^2} - \omega _t^2}} \right] . \end{aligned}$$

  (76)
  $$\begin{aligned} \alpha= & {} \sum \limits _{t = 1}^2 \left[ \frac{{K_{1t}^mK_{t1}^b( -a_{{s_2}}^b a_{{s_1}}^m + b_{{s_2}}^bb_{{s_1}}^m)}}{{{{({\omega _1} - {s_2}{\hat{\omega }} )}^2} - \omega _t^2}} \nonumber \right. \\&\left. + \frac{{K_{1t}^bK_{t1}^m(-a_{{s_1}}^m a_{{s_2}}^b + b_{{s_1}}^mb_{{s_2}}^b)}}{{{{({\omega _1} - {s_1}R{\hat{\omega }} )}^2} - \omega _t^2}} \right] , \end{aligned}$$

  (77)
  $$\begin{aligned} \beta= & {} \sum \limits _{t = 1}^2 \left[ \frac{{K_{1t}^mK_{t1}^b(-a_{{s_2}}^bb_{{s_1}}^m - a_{{s_1}}^mb_{{s_2}}^b)}}{{{{({\omega _1} - {s_2}{\hat{\omega }} )}^2} - \omega _t^2}} \nonumber \right. \\&\left. + \frac{{K_{1t}^bK_{t1}^m(-a_{{s_2}}^bb_{{s_1}}^m - a_{{s_1}}^mb_{{s_2}}^b)}}{{{{({\omega _1} - {s_1}R{\hat{\omega }} )}^2} - \omega _t^2}} \right] , \end{aligned}$$

  (78)
  $$\begin{aligned} {\hat{\omega }}= & {} \frac{2\omega _1}{s_1 R +s_2} . \end{aligned}$$

  (79)

• 3. $s_1{\varOmega _\mathrm{m}} + s_2{\varOmega _\mathrm{b}} \approx 2\omega _2$

  $$\begin{aligned} {\varOmega _\mathrm{m}}= & {} \frac{2R}{s_1 R + s_2} \left[ \omega _2 + \frac{{2\psi \pm \sqrt{({\alpha ^2} + {\beta ^2})} }}{2 \omega _2} \varepsilon ^2 \right] , \nonumber \\\end{aligned}$$

  (80)
  $$\begin{aligned} \psi= & {} \psi _\mathrm{m} + \psi _\mathrm{b}, \end{aligned}$$

  (81)
  $$\begin{aligned} \psi _\mathrm{m}= & {} \sum \limits _{t = 1}^2 \frac{{K_{2t}^mK_{t2}^m}}{2}\left[ \frac{{{{(a_{{s_1}}^m)}^2} + {{(b_{{s_1}}^m)}^2}}}{{{{({\omega _2} + {s_1}R{\hat{\omega }} )}^2} - \omega _t^2}} \nonumber \right. \\&\left. + \frac{{{{(a_{{s_1}}^m)}^2} + {{(b_{{s_1}}^m)}^2}}}{{{{({\omega _2} - {s_1}R{\hat{\omega }} )}^2} - \omega _t^2}} \right] , \end{aligned}$$

  (82)
  $$\begin{aligned} \psi _\mathrm{b}= & {} \sum \limits _{t = 1}^2 \frac{{K_{2t}^bK_{t2}^b}}{2}\left[ \frac{{{{(a_{{s_2}}^b)}^2} + {{(b_{{s_2}}^b)}^2}}}{{{{({\omega _2} - {s_2}{\hat{\omega }} )}^2} - \omega _t^2}} \nonumber \right. \\&\left. + \frac{{{(a_{{s_2}}^b)}^2} + {{(b_{{s_2}}^b)}^2}}{{{{({\omega _2} + {s_2}{\hat{\omega }} )}^2} - \omega _t^2}} \right] . \end{aligned}$$

  (83)
  $$\begin{aligned} \alpha= & {} \sum \limits _{t = 1}^2 \left[ \frac{{K_{2t}^mK_{t2}^b(b_{{s_2}}^bb_{{s_1}}^m-a_{{s_2}}^ba_{{s_1}}^m) }}{{{{({\omega _2} - {s_2}{\hat{\omega }} )}^2} - \omega _t^2}} \nonumber \right. \\&\left. + \frac{{K_{2t}^bK_{t2}^m(b_{{s_1}}^mb_{{s_2}}^b-a_{{s_1}}^ma_{{s_2}}^b )}}{{{{({\omega _2} - {s_1}R{\hat{\omega }} )}^2} - \omega _t^2}} \right] , \end{aligned}$$

  (84)
  $$\begin{aligned} \beta= & {} \sum \limits _{t = 1}^2 \left[ \frac{{K_{2t}^mK_{t2}^b(-a_{{s_2}}^bb_{{s_1}}^m - a_{{s_1}}^mb_{{s_2}}^b)}}{{{{({\omega _2} - {s_2}{\hat{\omega }} )}^2} - \omega _t^2}} \nonumber \right. \\&\left. + \frac{{K_{2t}^bK_{t2}^m(-a_{{s_2}}^bb_{{s_1}}^m - a_{{s_1}}^mb_{{s_2}}^b)}}{{{{({\omega _2} - {s_1}R{\hat{\omega }} )}^2} - \omega _t^2}} \right] , \end{aligned}$$

  (85)
  $$\begin{aligned} {\hat{\omega }}= & {} \frac{2\omega _2}{s_1 R +s_2} . \end{aligned}$$

  (86)

• 4. $s_1{\varOmega _\mathrm{m}} + s_2{\varOmega _\mathrm{b}} \approx \omega _1 + \omega _2$

  $$\begin{aligned} {\varOmega _\mathrm{m}}= & {} \frac{R}{s_1 R + s_2} \left[ \omega _1 + \omega _2 \pm \varepsilon ^2 \sqrt{\frac{{{\alpha _1}{\alpha _2} + {\beta _1}{\beta _2}}}{{{\omega _1}{\omega _2}}}} \right] , \nonumber \\\end{aligned}$$

  (87)
  $$\begin{aligned} {\alpha _1}= & {} \sum \limits _{t = 1}^2 \left[ \frac{{K_{2t}^mK_{t1}^b(-a_{{s_2}}^ba_{{s_1}}^m + b_{{s_2}}^bb_{{s_1}}^m)}}{{{{({\omega _1} - {s_2}{\hat{\omega }} )}^2} - \omega _t^2}} \nonumber \right. \\&\left. + \frac{{K_{2t}^bK_{t1}^m(-a_{{s_1}}^ma_{{s_2}}^b + b_{{s_1}}^mb_{{s_2}}^b)}}{{{{({\omega _1} - {s_1}R{\hat{\omega }} )}^2} - \omega _t^2}} \right] , \end{aligned}$$

  (88)
  $$\begin{aligned} {\beta _1}= & {} \sum \limits _{t = 1}^2 \left[ \frac{{K_{2t}^mK_{t1}^b(-a_{{s_2}}^bb_{{s_1}}^m - b_{{s_2}}^ba_{{s_1}}^m)}}{{{{({\omega _1} - {s_2}{\hat{\omega }} )}^2} - \omega _t^2}} \nonumber \right. \\&\left. + \frac{{K_{2t}^bK_{t1}^m( - a_{{s_1}}^mb_{{s_2}}^b - b_{{s_1}}^ma_{{s_2}}^b)}}{{{{({\omega _1} - {s_1}R{\hat{\omega }} )}^2} - \omega _t^2}} \right] , \end{aligned}$$

  (89)
  $$\begin{aligned} {\alpha _2}= & {} \sum \limits _{t = 1}^2 \left[ \frac{{K_{1t}^mK_{t2}^b(-a_{{s_2}}^ba_{{s_1}}^m + b_{{s_2}}^bb_{{s_1}}^m)}}{{{{({\omega _2} - {s_2}{\hat{\omega }} )}^2} - \omega _t^2}} \nonumber \right. \\&\left. + \frac{{ K_{1t}^b K_{t2}^m(-a_{{s_1}}^ma_{{s_2}}^b + b_{{s_1}}^mb_{{s_2}}^b)}}{{{{({\omega _2} - {s_1}R{\hat{\omega }} )}^2} - \omega _t^2}} \right] , \end{aligned}$$

  (90)
  $$\begin{aligned} {\beta _2}= & {} \sum \limits _{t = 1}^2 \left[ \frac{{K_{1t}^mK_{t2}^b(-a_{{s_2}}^bb_{{s_1}}^m - b_{{s_2}}^ba_{{s_1}}^m)}}{{{{({\omega _2} - {s_2}{\hat{\omega }} )}^2} - \omega _t^2}} \nonumber \right. \\&\left. + \frac{{K_{1t}^bK_{t2}^m( - a_{{s_1}}^mb_{{s_2}}^b - b_{{s_1}}^ma_{{s_2}}^b)}}{{{{({\omega _2} - {s_1}R{\hat{\omega }} )}^2} - \omega _t^2}} \right] , \end{aligned}$$

  (91)
  $$\begin{aligned} {\hat{\omega }}= & {} \frac{\omega _1 + \omega _2}{s_1 R+s_2} . \end{aligned}$$

  (92)