Skip to main content
SpringerLink
Log in

Partially unstable attractors in networks of forced integrate-and-fire oscillators

  • Original Paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

Abstract

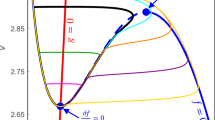
The asymptotic attractors of a nonlinear dynamical system play a key role in the long-term physically observable behaviors of the system. The study of attractors and the search for distinct types of attractor have been a central task in nonlinear dynamics. In smooth dynamical systems, an attractor is often enclosed completely in its basin of attraction with a finite distance from the basin boundary. Recent works have uncovered that, in neuronal networks, unstable attractors with a remote basin can arise, where almost every point on the attractor is locally transversely repelling. Herewith we report our discovery of a class of attractors: partially unstable attractors, in pulse-coupled integrate-and-fire networks subject to a periodic forcing. The defining feature of such an attractor is that it can simultaneously possess locally stable and unstable sets, both of positive measure. Exploiting the structure of the key dynamical events in the network, we develop a symbolic analysis that can fully explain the emergence of the partially unstable attractors. To our knowledge, such exotic attractors have not been reported previously, and we expect them to arise commonly in biological networks whose dynamics are governed by pulse (or spike) generation.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5

Similar content being viewed by others

References

  1. Ott, E.: Chaos in Dynamical Systems, 2nd edn. Cambridge University Press, Cambridge (2002)

    Book  MATH  Google Scholar 

  2. Hopfield, J.J.: Neural networks and physical systems with emergent collective computational abilities. Proc. Natl. Acad. Sci. (USA) 79(8), 2554–2558 (1982)

    Article  MathSciNet  Google Scholar 

  3. Zhang, Y., Zhang, H., Gao, W.: Multiple wada basins with common boundaries in nonlinear driven oscillators. Nonlinear Dyn. 79(4), 2667–2674 (2015)

    Article  MathSciNet  Google Scholar 

  4. Liu, X., Hong, L., Jiang, J., Tang, D., Yang, L.: Global dynamics of fractional-order systems with an extended generalized cell mapping method. Nonlinear Dyn. 83(3), 1419–1428 (2016)

    Article  MathSciNet  Google Scholar 

  5. Milnor, J.: On the concept of attractor. In: Hunt, B.R., Li, T.-Y., Kennedy, J.A., Nusse, H.E. (eds.) The Theory of Chaotic Attractors, pp. 243–264. Springer, New York (2004)

  6. Kaneko, K.: Dominance of milnor attractors and noise-induced selection in a multiattractor system. Phys. Rev. Lett. 78(14), 2736–2739 (1997)

    Article  Google Scholar 

  7. Alexander, J.C., Yorke, J.A., You, Z., Kan, I.: Riddled basins. Int. J. Bifur. Chaos Appl. Sci. Eng. 2, 795–813 (1992)

    Article  MathSciNet  MATH  Google Scholar 

  8. Ott, E., Sommerer, J.C., Alexander, J.C., Kan, I., Yorke, J.A.: Scaling behavior of chaotic systems with riddled basins. Phys. Rev. Lett. 71, 4134–4137 (1993)

    Article  MathSciNet  MATH  Google Scholar 

  9. Ashwin, P., Buescu, J., Stewart, I.: Bubbling of attractors and synchronisation of oscillators. Phys. Lett. A 193, 126–139 (1994)

    Article  MathSciNet  MATH  Google Scholar 

  10. Heagy, J.F., Carroll, T.L., Pecora, L.M.: Experimental and numerical evidence for riddled basins in coupled chaotic systems. Phys. Rev. Lett. 73, 3528–3531 (1994)

    Article  Google Scholar 

  11. Lai, Y.-C., Grebogi, C., Yorke, J.A., Venkataramani, S.: Riddling bifurcation in chaotic dynamical systems. Phys. Rev. Lett. 77, 55–58 (1996)

    Article  Google Scholar 

  12. Lai, Y.-C., Grebogi, C.: Noise-induced riddling in chaotic dynamical systems. Phys. Rev. Lett. 77, 5047–5050 (1996)

    Article  Google Scholar 

  13. Nakajima, H., Ueda, Y.: Riddled basins of the optimal states in learning dynamical systems. Phys. D 99, 35–44 (1996)

    Article  MathSciNet  MATH  Google Scholar 

  14. Ashwin, P., Buescu, J., Stewart, I.: From attractor to chaotic saddle: a tale of transverse instability. Nonlinearity 9, 703–737 (1996)

    Article  MathSciNet  MATH  Google Scholar 

  15. Lai, Y.-C.: Scaling laws for noise-induced temporal riddling in chaotic systems. Phys. Rev. E 56, 3897–3908 (1997)

    Article  MathSciNet  Google Scholar 

  16. Billings, L., Curry, J.H., Phipps, E.: Lyapunov exponents, singularities, and a riddling bifurcation. Phys. Rev. Lett. 79, 1018–1021 (1997)

    Article  Google Scholar 

  17. Maistrenko, Yu., Kapitaniak, T., Szuminski, P.: Locally and globally riddled basins in two coupled piecewise-linear maps. Phys. Rev. E 56, 6393–6399 (1997)

    Article  MathSciNet  Google Scholar 

  18. Maistrenko, Y.L., Maistrenko, V.L., Popovich, A., Mosekilde, E.: Transverse instability and riddled basins in a system of two coupled logistic maps. Phys. Rev. E 57, 2713–2724 (1998)

    Article  MathSciNet  Google Scholar 

  19. Kapitaniak, T., Maistrenko, Y., Stefanski, A., Brindley, J.: Bifurcation from locally to globally riddled basins. Phys. Rev. E 58, 8052–8052 (1998)

    Article  MathSciNet  Google Scholar 

  20. Lai, Y.-C., Grebogi, C.: Riddling of chaotic sets in periodic windows. Phys. Rev. Lett. 83, 2926–2929 (1999)

    Article  Google Scholar 

  21. Woltering, M., Markus, M.: Riddled-like basins of transient chaos. Phys. Rev. Lett. 84, 630–633 (2000)

    Article  Google Scholar 

  22. Lai, Y.-C., Tél, T.: Transient Chaos: Complex Dynamics on Finite-Time Scales. Springer, New York (2011)

    Book  MATH  Google Scholar 

  23. Munteanu, L., Brişan, C., Chiroiu, V., Dumitriu, D., Ioan, R.: Chaos—hyperchaos transition in a class of models governed by sommerfeld effect. Nonlinear Dyn. 78(3), 1877–1889 (2014)

    Article  MathSciNet  Google Scholar 

  24. Timme, M., Wolf, F., Geisel, T.: Prevalence of unstable attractors in networks of pulse-coupled oscillators. Phys. Rev. Lett. 89(15), 154105 (2002)

    Article  Google Scholar 

  25. Timme, M., Wolf, F., Geisel, T.: Unstable attractors induce perpetual synchronization and desynchronization. Chaos 13(1), 377–387 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  26. Mirollo, R.E., Strogatz, S.H.: Synchronization of pulse-coupled biological oscillators. SIAM J. Appl. Math. 50(6), 1645–1662 (1990)

    Article  MathSciNet  MATH  Google Scholar 

  27. van Vreeswijk, C.: Partial synchronization in populations of pulse-coupled oscillators. Phys. Rev. E 54, 5522–5537 (1996)

    Article  Google Scholar 

  28. Gong, P., van Leeuwen, C.: Dynamically maintained spike timing sequences in networks of pulse-coupled oscillators with delays. Phys. Rev. Lett. 98(4), 048104 (2007)

    Article  Google Scholar 

  29. Kanamaru, T., Aihara, K.: Roles of inhibitory neurons in rewiring-induced synchronization in pulse-coupled neural networks. Neural Comput. 22(5), 1383–1398 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  30. Luccioli, S., Politi, A.: Irregular collective behavior of heterogeneous neural networks. Phys. Rev. Lett. 105(15), 158104 (2010)

    Article  Google Scholar 

  31. Luccioli, S., Olmi, S., Politi, A., Torcini, A.: Collective dynamics in sparse networks. Phys. Rev. Lett. 109(13), 138103 (2012)

    Article  Google Scholar 

  32. Zumdieck, A., Timme, M., Geisel, T., Wolf, F.: Long chaotic transients in complex networks. Phys. Rev. Lett. 93(24), 244103 (2004)

    Article  Google Scholar 

  33. Zillmer, R., Livi, R., Politi, A., Torcini, A.: Desynchronization in diluted neural networks. Phys. Rev. E 74(3), 036203 (2006)

    Article  MathSciNet  Google Scholar 

  34. Jahnke, S., Memmesheimer, R.-M., Timme, M.: Stable irregular dynamics in complex neural networks. Phys. Rev. Lett. 100(4), 048102 (2008)

    Article  Google Scholar 

  35. Kirst, C., Timme, M.: How precise is the timing of action potentials? Front. Neurosci. 3(1), 2–3 (2009)

    Article  Google Scholar 

  36. Zillmer, R., Brunel, N., Hansel, D.: Very long transients, irregular firing, and chaotic dynamics in networks of randomly connected inhibitory integrate-and-fire neurons. Phys. Rev. E 79(3), 031909 (2009)

    Article  MathSciNet  Google Scholar 

  37. Zou, H., Guan, S., Lai, C.-H.: Dynamical formation of stable irregular transients in discontinuous map systems. Phys. Rev. E 80(4), 046214 (2009)

    Article  Google Scholar 

  38. Rothkegel, A., Lehnertz, K.: Irregular macroscopic dynamics due to chimera states in small-world networks of pulse-coupled oscillators. New J. Phys. 16(5), 055006 (2014)

    Article  Google Scholar 

  39. Timme, M., Wolf, F., Geisel, T.: Coexistence of regular and irregular dynamics in complex networks of pulse-coupled oscillators. Phys. Rev. Lett. 89(25), 258701 (2002)

    Article  Google Scholar 

  40. Politi, A., Luccioli, S.: Dynamics of networks of leaky-integrate-and-fire neurons. In: Estrada, E., Fox, M., Higham, D.J., Oppo, G.-L. (eds.) Network Science, pp. 217–242. Springer, London (2010)

  41. Olmi, S., Torcini, A., Politi, A.: Linear stability in networks of pulse-coupled neurons. Front. Comp. Neurosci. 8, 00008 (2014)

    MATH  Google Scholar 

  42. Politi, A., Rosenblum, M.: Equivalence of phase-oscillator and integrate-and-fire models. Phys. Rev. E 91(4), 042916 (2015)

    Article  Google Scholar 

  43. Zou, H., Gong, X., Lai, C.-H.: Unstable attractors with active simultaneous firing in pulse-coupled oscillators. Phys. Rev. E 82(4), 046209 (2010)

    Article  MathSciNet  Google Scholar 

  44. Ashwin, P., Timme, M.: Unstable attractors: existence and robustness in networks of oscillators with delayed pulse coupling. Nonlinearity 18(5), 2035–2060 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  45. Broer, H., Efstathiou, K., Subramanian, E.: Robustness of unstable attractors in arbitrarily sized pulse-coupled networks with delay. Nonlinearity 21(1), 13–49 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  46. Broer, H., Efstathiou, K., Subramanian, E.: Heteroclinic cycles between unstable attractors. Nonlinearity 21(6), 1385–1410 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  47. Kirst, C., Timme, M.: From networks of unstable attractors to heteroclinic switching. Phys. Rev. E 78(6), 065201 (2008)

    Article  MathSciNet  Google Scholar 

  48. Kirst, C., Geisel, T., Timme, M.: Sequential desynchronization in networks of spiking neurons with partial reset. Phys. Rev. Lett. 102(6), 068101 (2009)

    Article  Google Scholar 

  49. Neves, F.S., Timme, M.: Computation by switching in complex networks of states. Phys. Rev. Lett. 109(1), 018701 (2012)

    Article  Google Scholar 

  50. Neves, F.S., Timme, M.: Controlled perturbation-induced switching in pulse-coupled oscillator networks. J. Phys. A Math. Theor. 42(34), 345103 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  51. Manjunath, G., Tino, P., Jaeger, H.: Theory of input driven dynamical systems. Dice. Ucl. Ac. Be., pp. 25–27 (2012)

  52. Keener, J.P., Hoppensteadt, F.C., Rinzel, J.: Integrate-and-fire models of nerve membrane response to oscillatory input. SIAM J. Appl. Math. 41(3), 503–517 (1981)

    Article  MathSciNet  MATH  Google Scholar 

  53. Zou, H.-L., Li, M., Lai, C.-H., Lai, Y.-C.: Origin of chaotic transients in excitatory pulse-coupled networks. Phys. Rev. E 86(6), 066214 (2012)

    Article  Google Scholar 

  54. Memmesheimer, R.-M., Timme, M.: Designing the dynamics of spiking neural networks. Phys. Rev. Lett. 97(18), 188101 (2006)

    Article  Google Scholar 

  55. Newman, M.E.J.: Networks: An Introduction, 1st edn. Oxford University Press, Oxford (2010)

    Book  MATH  Google Scholar 

  56. Buonomano, D.V., Maass, W.: State-dependent computations: spatiotemporal processing in cortical networks. Nat. Rev. Neurosci. 10(2), 113–125 (2009)

    Article  Google Scholar 

  57. Rabinovich, M., Volkovskii, A., Lecanda, P., Huerta, R., Abarbanel, H.D.I., Laurent, G.: Dynamical encoding by networks of competing neuron groups: winnerless competition. Phys. Rev. Lett. 87(6), 068102 (2001)

    Article  Google Scholar 

  58. Ashwin, P., Borresen, J.: Discrete computation using a perturbed heteroclinic network. Phys. Lett. A 347(4), 208–214 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  59. Zou, H.-L., Katori, Y., Deng, Z.-C., Aihara, K., Lai, Y.-C.: Controlled generation of switching dynamics among metastable states in pulse-coupled oscillator networks. Chaos 25(10), 103109 (2015)

    Article  MathSciNet  Google Scholar 

Download references

Acknowledgements

This research is supported by the National Natural Science Foundation of China (11502200, 91648101), “The Fundamental Research Funds for the Central Universities” (No. 3102014JCQ01036), and SRF for ROCS, SEM. This research is also supported by the Aihara Project, the FIRST program from JSPS, initiated by CSTP, and CREST, JST. YCL is supported by ARO under Grant No. W911NF-14-1-0504.

Author information

Authors and Affiliations

  1. School of Mechanics, Civil Engineering and Architecture, Northwestern Polytechnical University, Xian, 710072, China

    Hai-Lin Zou, Zi-Chen Deng & Wei-Peng Hu

  2. Institute of Industrial Science, University of Tokyo, 4-6-1 Komaba, Meguro-ku, Tokyo, 153-8505, Japan

    Kazuyuki Aihara

  3. School of Electrical, Computer and Energy Engineering, Arizona State University, Tempe, AZ, 85287, USA

    Ying-Cheng Lai

Authors
  1. Hai-Lin Zou
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Zi-Chen Deng
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Wei-Peng Hu
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Kazuyuki Aihara
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Ying-Cheng Lai
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Hai-Lin Zou.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Zou, HL., Deng, ZC., Hu, WP. et al. Partially unstable attractors in networks of forced integrate-and-fire oscillators. Nonlinear Dyn 89, 887–900 (2017). https://doi.org/10.1007/s11071-017-3490-5

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-017-3490-5

Keywords

Search

Navigation