Complex Frequency-Shifted Perfectly Matched Layers for 2.5D Frequency-Domain Marine Controlled-Source EM Field Simulations

Abstract

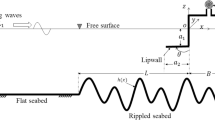
For geophysical electromagnetic (EM) forward modeling problems, the accuracy of solutions mainly depends on the numerical modeling method used and the corresponding boundary conditions. Most multi-dimensional EM studies deal with numerical methods for discretisation (e.g., finite-difference, finite-element, integral equation, etc.) and pay less attention to the boundaries. This review presents the recent research on optimizing boundary conditions for the frequency-domain marine controlled-source EM (CSEM) forward modeling algorithm. Current geophysical EM field simulation techniques usually utilize the truncated Dirichlet boundary condition, which requires the modeling domain boundaries to be far away from the area of interest and field values to be zero at the boundaries to mitigate artificial reflections/refractions resulting from truncated boundaries. The perfectly matched layer (PML) approach with few additional absorbing layers can serve as an alternative boundary to supress these truncated boundary effects. In this review, the application of the PML boundary condition to marine CSEM using a staggered finite-difference scheme for the 2.5D problem in vertical transverse isotropic (VTI) conductivity structures is introduced. This new algorithm utilizes the complex frequency-shifted PML (CFS-PML) boundary condition. The selection of optimal PML parameters are also further investigated for numerical stability. Numerical tests for several Earth conductivity models show that the CFS-PML approach is of similar high accuracy compared to using traditional Dirichlet boundary condition and exhibits additional advantages in terms of computational time and memory usage. Furthermore, the numerical tests indicate that the proposed forward modeling algorithm using CFS-PML boundary condition works well for both shallow and deep water cases, including the application to real field example from the Troll Field in Norway. The detectability of subsurface-related EM fields in airwave dominated shallow waters can be enhanced by using the weighted difference fields for mitigating the effect of airwaves on the models.

Appendix: 2.5D SFD Discretization

For the 2D SFD discretization (Fig. A1), the y- and z-components of $\mathbf{{{{\widetilde{E}}}}}$ evaluated at (j, k), $(j+\frac{1}{2},k)$, and $(j,k+\frac{1}{2})$ in Equation 10 are expressed as

Fig. A1

The 2D staggered-grid (j, k) modified after Li and Han (2017), where $\Delta y(j)$ and $\Delta z(k)$ is the horizontal size and vertical size, respectively. The electric field $\mathbf{{{{\widetilde{E}}}}}$ and the magnetic field $\mathbf{{{{\widetilde{H}}}}}$ are shown in (x, y, z) domain.

$$\begin{aligned}&\frac{\mathrm{i}k_x}{\gamma ^{yh}_j \Delta y_j} \left[ {\widetilde{E}}^{yS}_{j+\frac{1}{2},k} - {{{\widetilde{E}}}}^{yS}_{j-\frac{1}{2},k} \right] \nonumber \\&\quad - \frac{1}{\gamma ^{yh}_j \Delta y_j} \left[ \frac{{{{\widetilde{E}}}}^{xS}_{j+1,k} - {\widetilde{E}}^{xS}_{j,k}}{\gamma ^{ye}_{j+\frac{1}{2}} \Delta y_{j+\frac{1}{2}}} - \frac{{{{\widetilde{E}}}}^{xS}_{j,k} - {\widetilde{E}}^{xS}_{j-1,k}}{\gamma ^{ye}_{j-\frac{1}{2}} \Delta y_{j-\frac{1}{2}}} \right] \nonumber \\&\quad + \frac{\mathrm{i}k_x}{\gamma ^{zh}_k \Delta z_k} \left[ {{{\widetilde{E}}}}^{zS}_{j,k+\frac{1}{2}} - {{{\widetilde{E}}}}^{zS}_{j,k-\frac{1}{2}} \right] \nonumber \\&\quad - \frac{1}{\gamma ^{zh}_k \Delta z_k} \left[ \frac{{\widetilde{E}}^{xS}_{j,k+1} - {\widetilde{E}}^{xS}_{j,k}}{\gamma ^{ze}_{k+\frac{1}{2}} \Delta z_{k+\frac{1}{2}}} - \frac{{{{\widetilde{E}}}}^{xS}_{j,k} - {\widetilde{E}}^{xS}_{j,k-1}}{\gamma ^{ze}_{k-\frac{1}{2}} \Delta z_{k-\frac{1}{2}}} \right] \nonumber \\&\quad - \mathrm{i}\omega \mu _0 \underline{\underline{\sigma }}^{x*}_{j,k} {{{\widetilde{E}}}}^{xS}_{j,k} = \mathrm{i}\omega \mu _0 \left( \underline{\underline{\sigma }}^{x*}_{j,k} - \underline{\underline{\sigma }}^{xP*}_{j,k} \right) {\widetilde{E}}^{xP}_{j,k}, \end{aligned}$$

(A1)

$$\begin{aligned}&\frac{1}{\gamma ^{zh}_k \Delta z_k} \left[ \frac{{\widetilde{E}}^{zS}_{j+1,k+\frac{1}{2}} - {{{\widetilde{E}}}}^{zS}_{j,k+\frac{1}{2}} }{\gamma ^{ye}_{j+\frac{1}{2}} \Delta y_{j+\frac{1}{2}}} - \frac{{{{\widetilde{E}}}}^{zS}_{j+1,k-\frac{1}{2}} - {\widetilde{E}}^{zS}_{j,k-\frac{1}{2}} }{\gamma ^{ye}_{j+\frac{1}{2}} \Delta y_{j+\frac{1}{2}}} \right] \nonumber \\&\quad - \frac{1}{\gamma ^{zh}_k \Delta z_k} \left[ \frac{{{{\widetilde{E}}}}^{yS}_{j+\frac{1}{2},k+1} - {{{\widetilde{E}}}}^{yS}_{j+\frac{1}{2},k} }{\gamma ^{ze}_{k+\frac{1}{2}} \Delta z_{k+\frac{1}{2}}} - \frac{{{{\widetilde{E}}}}^{yS}_{j+\frac{1}{2},k} - {{{\widetilde{E}}}}^{yS}_{j+\frac{1}{2},k-1} }{\gamma ^{ze}_{k-\frac{1}{2}} \Delta z_{k-\frac{1}{2}}} \right] \nonumber \\&\quad + \frac{\mathrm{i}k_x}{\gamma ^{ye}_{j+\frac{1}{2}} \Delta y_{j+\frac{1}{2}}} \left[ {{{\widetilde{E}}}}^{xS}_{j+1,k} - {\widetilde{E}}^{xS}_{j,k} \right] \nonumber \\&\quad + \left( k^2_x - \mathrm{i}\omega \mu _0 \underline{\underline{\sigma }}^{y*}_{j+\frac{1}{2},k} \right) {{{\widetilde{E}}}}^{yS}_{j+\frac{1}{2},k} = \mathrm{i}\omega \mu _0\nonumber \\&\quad \left( \underline{\underline{\sigma }}^{y*}_{j+\frac{1}{2},k} - \underline{\underline{\sigma }}^{yP*}_{j+\frac{1}{2},k} \right) {{{\widetilde{E}}}}^{yP}_{j+\frac{1}{2},k}, \end{aligned}$$

(A2)

$$\begin{aligned}&\frac{\mathrm{i}k_x}{\gamma ^{ze}_{k+\frac{1}{2}} \Delta z_{k+\frac{1}{2}}} \left[ {{{\widetilde{E}}}}^{xS}_{j,k+1} - {\widetilde{E}}^{xS}_{j,k} \right] \nonumber \\&\quad + \frac{1}{\gamma ^{yh}_j \Delta y_j} \left[ \frac{{{{\widetilde{E}}}}^{yS}_{j+\frac{1}{2},k+1} - {{{\widetilde{E}}}}^{yS}_{j+\frac{1}{2},k} }{\gamma ^{ze}_{k+\frac{1}{2}} \Delta z_{k+\frac{1}{2}}} - \frac{{{{\widetilde{E}}}}^{yS}_{j-\frac{1}{2},k+1} - {{{\widetilde{E}}}}^{yS}_{j-\frac{1}{2},k} }{\gamma ^{ze}_{k+\frac{1}{2}} \Delta z_{k+\frac{1}{2}}} \right] \nonumber \\&\quad - \frac{1}{\gamma ^{yh}_j \Delta y_j} \left[ \frac{{\widetilde{E}}^{zS}_{j+1,k+\frac{1}{2}} - {{{\widetilde{E}}}}^{zS}_{j,k+\frac{1}{2}} }{\gamma ^{ye}_{j+\frac{1}{2}} \Delta y_{j+\frac{1}{2}}} - \frac{{{{\widetilde{E}}}}^{zS}_{j,k+\frac{1}{2}} - {\widetilde{E}}^{zS}_{j-1,k+\frac{1}{2}} }{\gamma ^{ye}_{j-\frac{1}{2}} \Delta y_{j-\frac{1}{2}}} \right] \nonumber \\&\quad + \left( k^2_x - \mathrm{i}\omega \mu _0 \underline{\underline{\sigma }}^{z*}_{j,k+\frac{1}{2}} \right) {{{\widetilde{E}}}}^{zS}_{j,k+\frac{1}{2}} \nonumber \\&\quad = \mathrm{i}\omega \mu _0 \left( \underline{\underline{\sigma }}^{z*}_{j,k+\frac{1}{2}} - \underline{\underline{\sigma }}^{zP*}_{j,k+\frac{1}{2}} \right) {{{\widetilde{E}}}}^{zP}_{j,k+\frac{1}{2}}, \end{aligned}$$

(A3)

where $\Delta y_{j+\frac{1}{2}} = \left( \Delta y_j + \Delta y_{j+1} \right) /2$ and $\Delta z_{k+\frac{1}{2}} = \left( \Delta z_k + \Delta z_{k+1} \right) /2$. The system matrix is transformed to be symmetric by multiplying the related cell area, i.e., Equation A1 by $\left( \gamma ^{yh}_j \Delta y_j \right) \left( \gamma ^{zh}_k \Delta z_k \right)$, Equation A2 by $\left( \gamma ^{ye}_{j+\frac{1}{2}} \Delta y_{j+\frac{1}{2}} \right) \left( \gamma ^{zh}_k \Delta z_k \right)$, and Equation A3 by $\left( \gamma ^{yh}_j \Delta y_j \right) \left( \gamma ^{ze}_{k+\frac{1}{2}} \Delta z_{k+\frac{1}{2}} \right)$ (e.g., Streich 2009; Li et al. 2018).

Smith (1996) and Haber et al. (2000) present the harmonic averaged conductivity to make the electric current density crossing cell boundary continuous. In this study, we present the harmonic averaged conductivity along y- or z-directions as

$$\begin{aligned} \underline{\underline{\sigma }}^{y*}_{j+\frac{1}{2},k}= & {} \frac{2\Delta y_{j+\frac{1}{2}}}{\Delta y_j/\underline{\underline{\sigma }}^{y*}_{j,k} + \Delta y_{j+1}/\underline{\underline{\sigma }}^{y*}_{j+1,k}}, \end{aligned}$$

(A4)

$$\begin{aligned} \underline{\underline{\sigma }}^{z*}_{j,k+\frac{1}{2}}= & {} \frac{2\Delta z_{k+\frac{1}{2}}}{\Delta z_k/\underline{\underline{\sigma }}^{z*}_{j,k} + \Delta z_{k+1}/\underline{\underline{\sigma }}^{z*}_{z,k+1}}. \end{aligned}$$

(A5)

Equation 17 can then be discretized as

$$\begin{aligned} {{{\widetilde{H}}}}^{xS}_{j+\frac{1}{2},k+\frac{1}{2}}= &; {} \frac{1}{\mathrm{i}\omega \mu _0} \left( \frac{{{{\widetilde{E}}}}^{zS}_{j+1,k+\frac{1}{2}} - {{{\widetilde{E}}}}^{zS}_{j,k+\frac{1}{2}}}{\Delta y_{j+\frac{1}{2}}} - \frac{{{{\widetilde{E}}}}^{yS}_{j+\frac{1}{2},k+1} - {\widetilde{E}}^{yS}_{j+\frac{1}{2},k}}{\Delta z_{k+\frac{1}{2}}} \right), \end{aligned}$$

(A6)

$$\begin{aligned} {{{\widetilde{H}}}}^{yS}_{j,k+\frac{1}{2}}= &; {} -\frac{1}{\mathrm{i}\omega \mu _0} \left(\mathrm{i}k_x {{{\widetilde{E}}}}^{zS}_{j,k+\frac{1}{2}} - \frac{{{{\widetilde{E}}}}^{xS}_{j,k+1} - {\widetilde{E}}^{xS}_{j,k}}{\Delta z_{k+\frac{1}{2}}}\right), \end{aligned}$$

(A7)

$$\begin{aligned} {{{\widetilde{H}}}}^{zS}_{j+\frac{1}{2},k}= &; {} \frac{1}{\mathrm{i}\omega \mu _0} \left(\mathrm{i}k_x {{{\widetilde{E}}}}^{yS}_{j+\frac{1}{2},k} - \frac{{{{\widetilde{E}}}}^{xS}_{j+1,k} - {\widetilde{E}}^{xS}_{j,k}}{\Delta y_{j+\frac{1}{2}}}\right). \end{aligned}$$

(A8)