An analysis of deformation and failure in rectangular tensile bars accounting for void shape changes

Abstract

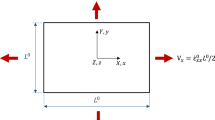
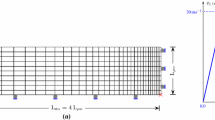
A three-dimensional finite deformation study of necking and failure in rectangular tensile bars is carried out using a constitutive relation for porous material plasticity. The fully dynamic formulation accounts for void nucleation and growth along with thermal and rate effects, but here focus is on quasi-static response with a specified initial void volume fraction. The constitutive relation takes into account void shape changes and associated void rotations for three-dimensional voids. The constitutive update is carried out using a generalized rate tangent scheme for an elastic-viscoplastic solid. The sensitivity of necking and failure patterns to the aspect ratio of the rectangular bar is investigated with focus on the plane strain limit and a square tensile bar. The calculations predict the well-known slant fracture in plane strain tension and the emergence of a cup-cone like failure region for a square cross-section. Details are provided for the development of porosity in the bar with a square cross-section, including void shape changes and void rotations. The numerical examples show the capability of a constitutive relation for porous plasticity that can model details of void evolution, thus paving the way for advanced analyses of ductile failure under arbitrary loadings.

Appendices

Appendix A: Specification of terms in the Madou–Leblond potential

The components of the quadratic form ${{\mathcal {Q}}}({\varvec{\sigma }})$ and linear form ${{\mathcal {H}}}({\varvec{\sigma }})$ in Eqs. (15) and (16), respectively, are expressed on the orthonormal basis ($\mathbf {n}_1, \mathbf {n}_2, \mathbf {n}_3$) associated with the voids and components of tensors are given in Cartesian tensor notation. The general form of the expressions was obtained by homogenization but additional heuristics was included, based on a large set of numerical (limit-analysis) simulations in (Madou and Leblond 2012b). This involved some interpolation from the previously studied cases of prolate and oblate voids using geometric parameters, such as void and cell eccentricities as parameters. Hence, some familiarity with the geometry of the underlying unit cell is needed.

The semi-axes of the void are denoted $a \ge b \ge c$. Those of the cell, taken to be confocal with the void, are denoted $A \ge B \ge C$. Thus,

$$\begin{aligned} A=\sqrt{a^{2}+\varLambda }, \quad B=\sqrt{b^{2}+\varLambda }, \quad C=\sqrt{c^{2}+\varLambda }\nonumber \\ \end{aligned}$$

(A-1)

where $\varLambda$ is the unique positive root of:

$$\begin{aligned} \left( a^{2}+\varLambda \right) \left( b^{2}+\varLambda \right) \left( c^{2}+\varLambda \right) -\frac{a^{2}b{^2}c^{2}}{f^{2}}=0\nonumber \\ \end{aligned}$$

(A-2)

A special case of interest is the completely flat confocal ellipsoid having semi-axes ${{\bar{a}}} \ge {{\bar{b}}} \ge {{\bar{c}}} =0$ and aspect ratio k:

$$\begin{aligned} {\bar{a}}=\sqrt{a^{2}-c^{2}}, \quad {\bar{b}}=\sqrt{b^{2}-c^{2}}, \quad k=\frac{{\bar{b}}}{{\bar{a}}} \end{aligned}$$

(A-3)

It is used to define the ‘second porosity’ g and related quantities through:

$$\begin{aligned} g=\frac{{\bar{a}}{\bar{b}}^{2}}{ABC}, \quad g_{1}=\frac{g}{1+g}, \quad g_{f}=\frac{g}{f+g} \end{aligned}$$

(A-4)

Physically, g has the meaning of void volume fraction of some fictitious prolate void obtained by rotating the completely flat ellipsoid (of semi-axes $\bar{a}, \bar{b}, \bar{c} = 0$) about its major axis (Madou and Leblond 2012b).

Finally, the eccentricities of the inner and outer ellipsoids are given by:

$$\begin{aligned} e_{xz}=\frac{{\bar{a}}}{a}, \quad E_{xz}=\frac{{\bar{a}}}{A}, \quad \quad E_{yz}=\frac{{\bar{b}}}{B} \end{aligned}$$

(A-5)

The tensor ${\varvec{H}}$ in Eq. (16) has a unit trace ($H_{kk} = 1$) and is diagonal in the ‘void’ basis:

$$\begin{aligned} {\varvec{H}} = \sum _{i=1,3} H_i \, \mathbf {n}_i\otimes \mathbf {n}_i\end{aligned}$$

(A-6)

with

$$\begin{aligned} \begin{aligned} \left\{ \begin{array}{l l} \displaystyle {H_1= \left( 1-k^{2}\right) H_1^\mathrm{prol}+k^{2}H_1^\mathrm{obl}, } \\ \displaystyle {H_2= \left( 1-k\right) H_2^\mathrm{prol}+kH_2^\mathrm{obl}+} \\ \displaystyle { \qquad \frac{1}{2}\left( 1-k\right) \frac{\alpha ^{2}+\beta ^{2}}{\alpha }\frac{E_{xz}^{3/2} \left( 1-\alpha -E_{xz} \right) }{\left( 1-\alpha -E_{xz} \right) ^{2}+\beta ^{2}},} \\ \displaystyle {\alpha = \frac{4k^{2}}{1+9k^{2}}, \quad \beta =\frac{3k^{2}}{1+30k^{2}} } \end{array} \right. \end{aligned} \end{aligned}$$

(A-7)

where

$$\begin{aligned} \begin{aligned} H_1^\mathrm{prol}=1-2H_2^\mathrm{prol}, \quad H_2^\mathrm{prol}=\frac{1}{3}\left( 1+E_{xz}^{2}-\frac{E_{xz}^{4}}{2} \right) , \\ H_1^\mathrm{obl} = H_2^\mathrm{obl} = \frac{1}{3}\frac{\left( 2-7E_{xz}^{2}+5E_{xz}^{4}\right) }{\left( 2-7E_{xz}^{2}+10E_{xz}^{4}\right) } \end{aligned} \end{aligned}$$

(A-8)

A key parameter entering Eq. (13) is

$$\begin{aligned} \kappa = \frac{3}{2 {{\bar{F}}}} \end{aligned}$$

(A-9)

with, in general,

$$\begin{aligned} \begin{aligned}&{\bar{F}}=1+\frac{1}{\ln \left( g_{f}/g_{1} \right) } \Biggl [ -\left( 1-k\right) \left( 1-\frac{\sqrt{3}}{2} \right) \\&\quad \ln \frac{11k^{2}+5g_{f}}{11k^{2}+5g_{1}}+ \\&\quad \frac{3}{5}\left( 1-k\right) ^{2}\ln \frac{8-5g_{1}}{8-5g_{f}} +\frac{13}{10}k\left( g_{f}-g_{1} \right) -\frac{3}{10}k\left( g_{f}^{5}-g_{1}^{5} \right) \Biggr ] \end{aligned} \end{aligned}$$

(A-10)

For a prolate spheroidal void, the following expression is used:

$$\begin{aligned} {\bar{F}}=1+\frac{1-\sqrt{3}/2}{\ln f} \ln \frac{11+5e_{xz}^{3}/\left( 1-e_{xz}^{2} \right) }{11+5fe_{xz}^{3}/\left( 1-e_{xz}^{2} \right) } \end{aligned}$$

(A-11)

In the case of spherical voids, $H_1 = H_2 = H_3= 1/3$ and ${{\bar{F}}} = 1$ so that ${{\mathcal {H}}}({\varvec{\sigma }})$ reduces to the mean normal stress $\sigma _{kk}/3$ and $\kappa = 3/2$ as in the GT constitutive relation.

The quadratic form in Eq. (15) is based on the Willis bound. It may be written as:

$$\begin{aligned}&{\mathcal {Q}}\left( {\varvec{\sigma }}\right) = {\mathcal {Q}}^{W}\left( {\varvec{\sigma }}\right) - (1+g)(f+g)\kappa ^{2} {{{\mathcal {H}}}}^2 \end{aligned}$$

(A-12)

where it is convenient to formally isolate diagonal stress components as follows:

$$\begin{aligned}&{\mathcal {Q}}^{W}\left( {\varvec{\sigma }}\right) = {\varvec{\sigma }}^{\texttt {dg}} \cdot {\varvec{Q}}^{\texttt {dg}} \cdot {\varvec{\sigma }}^{\texttt {dg}}\nonumber \\&+ {\varvec{\sigma }}^{\texttt {offdg}} \cdot {\varvec{Q}}^{\texttt {offdg}} \cdot {\varvec{\sigma }}^{\texttt {offdg}} \end{aligned}$$

(A-13)

with

$$\begin{aligned} {\varvec{\sigma }}^{\texttt {dg}} \equiv \left( \begin{array}{l l} \displaystyle {\sigma _{xx}} \\ \displaystyle {\sigma _{yy}} \\ \displaystyle {\sigma _{zz}} \\ \end{array} \right) , \quad {\varvec{\sigma }}^{\texttt {offdg}} \equiv \left( \begin{array}{l l} \displaystyle {\sigma _{xy}} \\ \displaystyle {\sigma _{yz}} \\ \displaystyle {\sigma _{xz}} \\ \end{array} \right) \end{aligned}$$

(A-14)

Here and in what follows, we identify the x-, y- and z-axes with the void axes, for convenience. Then ${\varvec{Q}}^{\texttt {dg}}$ and ${\varvec{Q}}^{\texttt {offdg}}$ are symmetric second-rank tensors defined by

$$\begin{aligned}&{\varvec{Q}}^{\texttt {dg}} \equiv \left( 1-f \right) \begin{bmatrix} 1 &{} -\frac{1}{2} &{} -\frac{1}{2} \\ -\frac{1}{2} &{} 1 &{} -\frac{1}{2} \\ -\frac{1}{2} &{} -\frac{1}{2} &{} 1 \end{bmatrix}\nonumber \\&+ \frac{3f}{2} \begin{bmatrix} T_{\texttt {xxxx}} &{} T_{\texttt {xxyy}} &{} T_{\texttt {xxzz}} \\ T_{\texttt {yyxx}} &{} T_{\texttt {yyyy}} &{} T_{\texttt {yyzz}} \\ T_{\texttt {zzxx}} &{} T_{\texttt {zzyy}} &{} T_{\texttt {zzzz}} \end{bmatrix}^{-1} \end{aligned}$$

(A-15)

$$\begin{aligned}&{\varvec{Q}}^{\texttt {offdg}} \equiv 3\left( 1-f \right) \begin{bmatrix} 1 &{} 0 &{} 0 \\ 0 &{} 1 &{} 0 \\ 0 &{} 0 &{} 1 \end{bmatrix}\nonumber \\&+ \frac{3f}{2} \begin{bmatrix} T_{\texttt {xyxy}} &{} 0 &{} 0 \\ 0 &{} T_{\texttt {yzyz}} &{} 0\\ 0 &{} 0 &{} T_{\texttt {zxzx}} \end{bmatrix}^{-1} \end{aligned}$$

(A-16)

where $\mathbb {T}$ is a fourth-order tensor connected to the Eshelby tensor $\mathbb {S}(\nu )$ of an ellipsoidal void of semi-axes a,b,c embedded in a fictitious infinite elastic medium with shear modulus $\mu$ and Poisson ratio $\nu$, through the relation

$$\begin{aligned} \mathbb {T} = \lim _{\nu \rightarrow 1/2} \frac{1}{2\mu } \mathbb {L}\left( \mu ,\nu \right) :\left[ \mathbb {I}-\mathbb {S}\left( \nu \right) \right] \end{aligned}$$

(A-17)

where $\mathbb {L}\left( \mu ,\nu \right)$ denotes the stiffness tensor of the medium and $\mathbb {I}$ the identity tensor. The components of tensor $\mathbb {T}$ involve implicit integrals and obey the symmetry relations $T_{ijkl}$= $T_{jikl}$=$T_{ijlk}$=$T_{klij}$. They are listed in Appendix C of Madou and Leblond (2012b).

The parameters involved in the linear form ${{{\mathcal {H}}}}({\varvec{\sigma }})$ were assessed against a large dataset of numerical limit analyses (Madou and Leblond 2012b). Also, such calculations are typically carried out using a “frozen’ microstructure” and the quadratic form ${{{\mathcal {Q}}}}({\varvec{\sigma }})$ is based on a tight bound, which remains nevertheless a bound. To account for the effects of deviation from the assumptions underlying the limit analyses (including, for example, the effects of the evolution of the normal to the flow potential surface, strain hardening and strain-rate hardening) Tvergaard’s parameters $q_1$ and $q_2$ (multiplying $\kappa$) have been kept. In the results shown in the paper, constant values of $q_1$ and $q_2$ have been specified. Morin et al. (2016) have proposed a more complex formula for $q_1$, which is not used here.

Appendix B: Specification of the concentration tensors

As in Appendix A component expressions, when given, are expressed in Cartesian tensor notation. For an elastic porous matrix, strain concentration is determined in some average sense by

$$\begin{aligned} {\varvec{D}}^{v} = \mathbb {C}^\mathrm{e}: \mathbf{d}, \quad \mathbb {C}^\mathrm{e} = \left[ \mathbb {I} -\left( 1-f\right) \mathbb {S} \right] ^{-1} \end{aligned}$$

(B-1)

where $\mathbb {S}$ is Eshelby (1957)’s first tensor as above; see (Ponte Castañeda and Zaidman 1994).

For a plastic porous matrix, using the above linear estimate leads to poor predictions, as expected (Madou et al. 2013). Using the following definition of a plastic strain concentration tensor:

$$\begin{aligned} {\varvec{D}}^{v} = \mathbb {C}: \mathbf{d}^p \end{aligned}$$

(B-2)

Madou and Leblond introduced a well-calibrated tensor $\mathbb {C}$ based on a correction of $\mathbb {C}^\mathrm{e}$ as follows. For convenience, a change of variable is made for strain-rate tensors, e.g. $\mathbf{d}$:

$$\begin{aligned} \left\{ \begin{array}{l llll} \displaystyle {{\bar{d}}_{1}}= d_{m} \\ \displaystyle {{\bar{d}}_{2}}= d_{xx}-d_{m} \\ \displaystyle {{\bar{d}}_{3}}= d_{yy}-d_{zz} \\ \displaystyle {{\bar{d}}_{4}}= d_{xy}\\ \displaystyle {{\bar{d}}_{5}}= d_{xz}\\ \displaystyle {{\bar{d}}_{6}}= d_{yz} \end{array} \right. \end{aligned}$$

(B-3)

Similar transformations are made for ${\varvec{D}}^v$ and $\mathbf{d}^p$. This is somewhat like a Voigt transformation. Also, note that we have kept the x-, y- and z indices for components in the void basis ($\mathbf {n}_1, \mathbf {n}_2, \mathbf {n}_3$) as in presenting the quadratic form in Appendix A. In matrix form, Eqs. (B-1) and (B-2) now write using barred notation:

$$\begin{aligned} {\bar{D}}^v = {\bar{C}}^\mathrm{e} \cdot {\bar{d}}, \qquad {\bar{D}}^v = {\bar{C}} \cdot {\bar{d}}^p \end{aligned}$$

(B-4)

We thus have:

$$\begin{aligned} {\bar{C}} = \begin{pmatrix} {\bar{C}}_{11} &{} {\bar{C}}_{12} &{} {\bar{C}}_{13} &{} 0 &{} 0 &{} 0 \\ {\bar{C}}_{21} &{} {\bar{C}}_{22} &{} {\bar{C}}_{23} &{} 0 &{} 0 &{} 0 \\ {\bar{C}}_{31} &{} {\bar{C}}_{32} &{} {\bar{C}}_{33} &{} 0 &{} 0 &{} 0 \\ 0 &{} 0 &{} 0 &{} {\bar{C}}_{44} &{} 0 &{} 0 \\ 0 &{} 0 &{} 0 &{} 0 &{} {\bar{C}}_{55} &{} 0 \\ 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} {\bar{C}}_{66} \\ \end{pmatrix} \end{aligned}$$

(B-5)

where the following relations hold in view of the notation condensation inherent to Eq. (B-3):

$$\begin{aligned} \left\{ \begin{array}{l lllll} \displaystyle {{\bar{C}}_{11}}= 1/f; \quad {\bar{C}}_{12}={\bar{C}}_{13}=0 \\ \displaystyle {{\bar{C}}_{21}= C_{xxxx}+C_{xxyy}+C_{xxzz}-1/f} \\ \displaystyle {{\bar{C}}_{22}}= \left( 2C_{xxxx}-C_{xxyy}-C_{xxzz}\right) /2 \\ \displaystyle {{\bar{C}}_{23}}= \left( C_{xxyy}-C_{xxzz}\right) /2 \\ \displaystyle {{\bar{C}}_{31}}= C_{yyxx}+C_{yyyy}+C_{yyzz}\\ -C_{zzxx}-C_{zzyy}+C_{zzzz} \\ \displaystyle {{\bar{C}}_{32}}= \Big ( 2C_{yyxx}-C_{yyyy}-C_{yyzz}\\ -2C_{zzxx}+C_{zzyy}+C_{zzzz}\Big )/2 \\ \displaystyle {{\bar{C}}_{33}}= \left( C_{yyyy}-C_{yyzz}-C_{zzyy}+C_{zzzz}\right) /2 \\ \displaystyle {{\bar{C}}_{44}}= 2C_{xyxy}; \quad {\bar{C}}_{55}=2C_{xzxz}; \quad {\bar{C}}_{66}=2C_{yzyz} \\ \end{array} \right. \end{aligned}$$

(B-6)

The problem reduces to specifying the components of ${\bar{C}}$ in terms of the known components of ${\bar{C}}^\mathrm{e}$:

$$\begin{aligned} {\bar{C}}_{\alpha \beta } = h_{\alpha \beta }{\bar{C}}_{\alpha \beta }^\mathrm{e} \qquad \left( \text {no} \text{ sum } \text{ on } \alpha \text{ or } \beta \right) \end{aligned}$$

(B-7)

where $h_{\alpha \beta }$ are correction factors taken to depend on void volume fraction f, void shape, as well as the stress state (via dimensionless ratios of stress invariants; see below). In fact, only three coefficients are needed since (Madou et al. 2013):

$$\begin{aligned} {\mathbf {h}} = \begin{pmatrix} 1 &{} 1 &{} 1 &{} 0 &{} 0 &{} 0 \\ 1 &{} h_{22} &{} h_{22} &{} 0 &{} 0 &{} 0 \\ 1 &{} h_{22} &{} h_{33} &{} 0 &{} 0 &{} 0 \\ 0 &{} 0 &{} 0 &{} h_{44} &{} 0 &{} 0 \\ 0 &{} 0 &{} 0 &{} 0 &{} h_{44} &{} 0 \\ 0 &{} 0 &{} 0 &{} 0 &{} 0 &{} h_{33} \\ \end{pmatrix} \end{aligned}$$

(B-8)

The stress state dependence, which reflects strongly nonlinear effects, is taken into account via the stress triaxiality ratio, T, the Lode parameter L, the lateral triaxiality $T_h$ and a deviatoric transverse invariant X defined through:

$$\begin{aligned} T \equiv \frac{\sigma _{\mathrm{m}}}{\sigma _{\mathrm{eq}}}; \qquad L \equiv \cos \left( 3\phi \right) \equiv \frac{27}{2} \texttt {det} \frac{{\varvec{\sigma }}'}{\sigma _{\mathrm{eq}}} \end{aligned}$$

(B-9)

where $0 \le \phi \le \pi /3$, and

$$\begin{aligned} T_{h} \equiv \frac{\frac{1}{2}\left( \sigma _{yy}+\sigma _{zz} \right) }{\sigma _{\mathrm{eq}}}; \qquad X \equiv \frac{\sigma _{xx}-\frac{1}{2}\left( \sigma _{yy}+\sigma _{zz} \right) }{\sigma _{\mathrm{eq}}}\nonumber \\ \end{aligned}$$

(B-10)

The void shape dependence of the $h_{\alpha \beta }$’s enters via factor k and eccentricity $e_{xz}$, defined in Eqs. (A-3) and (A-5), respectively.

With the above definitions, the corrections proceed by interpolating the general case between the prolate and oblate special cases, which themselves are interpolated between a sphere and a cylinder. Thus,

$$\begin{aligned} \left\{ \begin{array}{l llll} \displaystyle {{h}_{22}\left( f,k,e_{xz},{\varvec{\sigma }}\right) }= \left( 1-k \right) h_{22}^\mathrm{prol} \left( f,e_{xz},{\varvec{\sigma }}\right) \\ +k h^\mathrm{obl} \left( f,e_{xz},{\varvec{\sigma }}\right) \\ \displaystyle {{h}_{33}\left( f,k,e_{xz},{\varvec{\sigma }}\right) }= \left( 1-k \right) h_{33}^\mathrm{prol} \left( f,e_{xz},{\varvec{\sigma }}\right) \\ +k h^\mathrm{obl} \left( f,e_{xz},{\varvec{\sigma }}\right) \\ \displaystyle {{h}_{44}\left( f,k,e_{xz},{\varvec{\sigma }}\right) }= \left( 1-k \right) h_{44}^\mathrm{prol} \left( f,e_{xz},{\varvec{\sigma }}\right) \\ +k h^\mathrm{obl} \left( f,e_{xz},{\varvec{\sigma }}\right) \end{array} \right. \end{aligned}$$

(B-11)

where ${\varvec{\sigma }}$ indicates stress-state dependence and $h_{22}^\mathrm{prol}$, $h_{33}^\mathrm{prol}$, $h_{44}^\mathrm{prol}$ and $h^\mathrm{obl}$ are given by:

$$\begin{aligned}&\left\{ \begin{array}{l llll} \displaystyle {{h}_{22}^\mathrm{prol}\left( f,e_{xz},{\varvec{\sigma }}\right) }= \left( 1-e_{xz}^{30} \right) h^\mathrm{sph} \left( f,{\varvec{\sigma }}\right) + e_{xz}^{30}\\ \displaystyle {{h}_{33}^\mathrm{prol}\left( f,e_{xz},{\varvec{\sigma }}\right) }= \left( 1-e_{xz}^{30} \right) h^\mathrm{sph} \left( f,{\varvec{\sigma }}\right) + e_{xz}^{30}h_{33}^\mathrm{cyl}\left( f,{\varvec{\sigma }}\right) \\ \displaystyle {{h}_{44}^\mathrm{prol}\left( f,e_{xz},{\varvec{\sigma }}\right) }= \left( 1-e_{xz}^{30} \right) h^\mathrm{sph} \left( f,{\varvec{\sigma }}\right) + e_{xz}^{30}h_{44}^\mathrm{cyl}\left( f,{\varvec{\sigma }}\right) \end{array} \right. \nonumber \\\end{aligned}$$

(B-12)

$$\begin{aligned}&{h}^\mathrm{obl}\left( f,e_{xz},{\varvec{\sigma }}\right) = \left( 1-e_{xz}^{50} \right) h^\mathrm{sph} \left( f,{\varvec{\sigma }}\right) + e_{xz}^{50}\nonumber \\ \end{aligned}$$

(B-13)

in which

$$\begin{aligned} h^\mathrm{sph}= & {} \frac{1}{7}\left( 9-10\sqrt{f}+8f \right) - \nonumber \\&\left( 1-\sqrt{f} \right) ^{8}\frac{11T^{4}}{5\left( 11+|T|^{3} \right) } \left[ 1+\frac{L}{3}{} \texttt {sgn}\left( T\right) \right] \end{aligned}$$

(B-14)

$$\begin{aligned}&h_{33}^\mathrm{cyl}= \frac{1}{2}\left( 3-5\sqrt{f}+4f \right) -\left( 1-\sqrt{f} \right) ^{5}\frac{9T_{h}^{4}}{20+T_{h}^{4}} \nonumber \\\end{aligned}$$

(B-15)

$$\begin{aligned}&h_{44}^\mathrm{cyl}= 1+\frac{11}{2}\left( 1-\sqrt{f} \right) ^{11} -\left( 1-\sqrt{f} \right) ^{11}\frac{84X^{2}}{1+20X^{2}}\nonumber \\ \end{aligned}$$

(B-16)

All of the above expressions were validated against direct numerical simulations by limit analysis (Madou et al. 2013). Large values of exponents indicate the strong nonlinearity of some effects.

The procedure for calculating the void rotation rate is quite similar to that use for the void strain rate and the same numerical results were used by Madou and Leblond (Madou et al. 2013) to obtain corrections for mean void rotations.

Define void rotation-rate concentration tensors $\mathbb {R}^\mathrm{e}$ and $\mathbb {R}$ through:

$$\begin{aligned} {\varvec{\varOmega }}^{v}= & {} {\varvec{\varOmega }}+ \mathbb {R}^\mathrm{e}: \mathbf{d}, \quad \mathbb {R}^\mathrm{e} \equiv \left( 1-f\right) \mathbb {P}: \mathbb {C}^\mathrm{e} \end{aligned}$$

(B-17)

$$\begin{aligned} {\varvec{\varOmega }}^{v}= & {} {\varvec{\varOmega }}+ \mathbb {R} : \mathbf{d}^p , \end{aligned}$$

(B-18)

for the elastic and plastic cases, respectively. Here, $\mathbb {P}$ is Eshelby’s second tensor and $\mathbb {C}^\mathrm{e}$ the elastic strain-rate concentration tensor, as above. Eq. (B-17B-18) was derived using homogenization by Kailasam and Ponte Castaneda (1998).

Simpler corrections are introduced for the non-zero components of $\mathbb {R}$ on the basis of those, known, of $\mathbb {R}^\mathrm{e}$:

$$\begin{aligned}&R_{xyxy}= \rho R_{xyxy}^\mathrm{e}, \quad R_{xzxz}= \rho R_{xzxz}^\mathrm{e},\nonumber \\&\quad R_{yzyz}= R_{yzyz}^\mathrm{e} \end{aligned}$$

(B-19)

which involve only one coefficient, $\rho$, given by:

$$\begin{aligned} \rho \left( f,k,e_{xz},{\varvec{\sigma }}\right) = \left( 1-k\right) \rho ^\mathrm{prol}\left( f,e_{xz},{\varvec{\sigma }}\right) +k\nonumber \\ \end{aligned}$$

(B-20)

with

$$\begin{aligned} \rho ^\mathrm{prol}\left( f,e_{xz},{\varvec{\sigma }}\right) = 1-e_{xz}^{30}+e_{xz}^{30}\rho ^\mathrm{cyl} \left( f,{\varvec{\sigma }}\right) \end{aligned}$$

(B-21)

and

$$\begin{aligned} \rho ^\mathrm{cyl}= 1+11\left( 1-\sqrt{f} \right) ^{11} -\left( 1-\sqrt{f} \right) ^{11}\frac{170X^{2}}{1+20X^{2}}\nonumber \\ \end{aligned}$$

(B-22)