Analysis of self-similar rate-dependent interfacial crack propagation in mode II

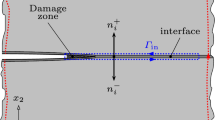
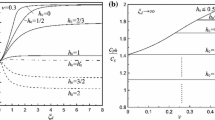
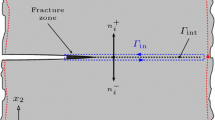
Abstract

The present study analyzes the fundamental properties of a rate-dependent cohesive model applied to the description of dynamic mode-II crack propagation. To make a semi-analytical treatment possible, the idealised problem of a crack along the interface between a semi-infinite elastic layer and a rigid substrate is considered. Solutions corresponding to the propagation of the crack tip at a constant speed are constructed. Using asymptotic properties of the solution far from the crack tip allows obtaining the complete solution of the boundary value problem by direct integration without iterations, using a specific form of the shooting method. By conversion of the problem to dimensionless variables, the behavior of the system for all possible crack velocities and arbitrary combinations of material and geometric parameters can be characterized. The dependence of fracture energy and other important characteristics on model parameters and the crack speed can then be analyzed. Even if the approach is applied to a specific form of damage rate dependence and motivated by the analysis of delamination propagation, the same technique could be used for other classes of interfacial cohesive rate-dependent models.

Appendix: Peeling test

Mode-II delamination can be regarded as a special case of the peeling test, in which an elastic strip is detached from a rigid substrate by a force $F_c$ that pulls in an inclined direction, under a prescribed angle $\theta$; see Fig. 16a. If the peeling process is considered as rate-independent, the energy balance equation written for a steady process contains terms that represent the external work, energy dissipated by debonding, increase of potential energy of elastic deformation and increase of kinetic energy. For an increment in which the length of the debonded zone increases by $\mathrm{d}a$, the energy balance equation can be written as

$$\begin{aligned} F_c\,\mathrm{d}u = G_{c0}b\,\mathrm{d}a + \frac{1}{2}E\varepsilon _c^2bh\,\mathrm{d}a + \frac{1}{2}\rho v^2bh\,\mathrm{d}a \end{aligned}$$

(142)

where $\mathrm{d}u$ is the distance by which the loaded end of the sheet subjected to force $F_c$ moves in the direction aligned with the force, $\varepsilon _c$ is the axial strain in the debonded sheet, and v is the constant speed at which this part moves. The left-hand side of (142) is the incremental supplied work, and the terms on the right-hand side correspond to the energy dissipated by creating a newly debonded area $b\,\mathrm{d}a$, elastic energy stored by stretching the newly debonded segment of volume $bh\,\mathrm{d}a$ to strain $\varepsilon _c$, and kinetic energy of the newly debonded segment that was initially at rest and after debonding is moving at speed v. It is worth noting that the first term on the right-hand side of (142), which represents the dissipated energy (or work of separation), is considered here as independent of angle $\theta$. This means that mode mixity is assumed to have no influence on the energy needed to separate the surfaces. In the case of adhesion, this assumption is acceptable, as proven, e.g., by the experiments of Kendall (1975) performed on rubber crosslinked against a glass plate.

As shown in Fig. 16b, the distance $\mathrm{d}u$ can be expressed as the difference between the length of the newly debonded stretched segment, $\mathrm{d}a(1+\varepsilon _c)$, and the projection of the initial length of this segment, $\mathrm{d}a\cos \theta$. Substituting

$$\begin{aligned} \mathrm{d}u = (1+\varepsilon _c)\mathrm{d}a - \cos \theta \mathrm{d}a \end{aligned}$$

(143)

into (142) and dividing both sides by $b\,\mathrm{d}a$, we obtain

$$\begin{aligned} \frac{F_c}{b}(1+\varepsilon _c-\cos \theta ) = G_{c0} + \frac{1}{2}Eh\varepsilon _c^2 + \frac{1}{2}\rho h v^2\qquad \end{aligned}$$

(144)

Since the sheet is assumed to be linear elastic, the strain is easily expressed as

$$\begin{aligned} \varepsilon _c = \frac{F_c}{Ebh} \end{aligned}$$

(145)

From Fig. 16b it is also possible to deduce the speed at which the sheet end (and thus the entire debonded segment) moves. The horizontal component of the displacement of the sheet end is $\mathrm{d}a-\mathrm{d}a(1+\varepsilon _c)\cos \theta$ and the vertical component is $\mathrm{d}a(1+\varepsilon _c)\sin \theta$. Consequently, the speed of the delaminated part, v, can be linked to the crack speed, ${\dot{a}}$, by the relation

$$\begin{aligned} v= & {} {\dot{a}}\sqrt{\left( 1-(1+\varepsilon _c)\cos \theta \right) ^2+\left( (1+\varepsilon _c)\sin \theta \right) ^2}\nonumber \\= & {} {\dot{a}}\sqrt{(1+\varepsilon _c)^2-2(1+\varepsilon _c)\cos \theta +1} \end{aligned}$$

(146)

Substituting (145) and (146) into (144), we construct a quadratic equation for the force $F_c$. The derivation is somewhat easier to manage if we consider $\varepsilon _c$ instead of $F_c$ as the primary unknown. Equation (144) with $F_c$ replaced by $Ebh\varepsilon _c$ and with v expressed according to (146) reads

$$\begin{aligned}&Eh\varepsilon _c(1+\varepsilon _c-\cos \theta ) = G_{c0} + \frac{1}{2}Eh\varepsilon _c^2 \nonumber \\&\quad + \frac{1}{2}\rho h {\dot{a}}^2\left( (1+\varepsilon _c)^2-2(1+\varepsilon _c)\cos \theta +1\right) \nonumber \\ \end{aligned}$$

(147)

When (147) is divided by Eh and the fraction $\rho /E$ is replaced by $1/c^2$ where c is the elastic wave speed, we obtain

$$\begin{aligned}&\varepsilon _c(1+\varepsilon _c-\cos \theta ) = \frac{G_{c0}}{Eh} + \frac{1}{2}\varepsilon _c^2 \nonumber \\&\quad + \frac{1}{2}\frac{{\dot{a}}^2}{c^2}\left( (1+\varepsilon _c)^2-2(1+\varepsilon _c)\cos \theta +1\right) \end{aligned}$$

(148)

This is the energy balance equation in a dimensionless form, which can further be rewritten as

$$\begin{aligned}&2\varepsilon _c(1+\varepsilon _c-\cos \theta ) = 2\gamma + \varepsilon _c^2 \nonumber \\&\quad +\,\alpha ^2\left( (1+\varepsilon _c)^2-2(1+\varepsilon _c)\cos \theta +1\right) \end{aligned}$$

(149)

where $\alpha ={\dot{a}}/c$ is the relative crack speed and

$$\begin{aligned} \gamma =\frac{G_{c0}}{Eh} \end{aligned}$$

(150)

is a dimensionless parameter characterizing the ratio between the fracture energy and the elastic sectional stiffness per unit width. For stiff and weakly bonded sheets, this parameter is small compared to 1.

Equation (149) can be recast into a quadratic equation

$$\begin{aligned}&(1-\alpha ^2)\varepsilon _c^2 + 2(1-\alpha ^2)(1-\cos \theta ) \varepsilon _c \nonumber \\&\quad -\,2 (\gamma +\alpha ^2(1-\cos \theta )) = 0 \end{aligned}$$

(151)

with $\varepsilon _c$ as the unknown variable. Since the coefficients at $\varepsilon _c^2$ and at $\varepsilon _c$ are positive while the absolute term is negative, the equation has two real roots, one positive and one negative. Since the peeling test leads to tension, only the positive solution is physically meaningful, and so we get

$$\begin{aligned} \varepsilon _c= & {} \frac{-\,2(1-\alpha ^2)(1-\cos \theta )+\sqrt{4(1-\alpha ^2)^2(1-\cos \theta )^2+4(1-\alpha ^2)2 (\gamma +\alpha ^2(1-\cos \theta ))}}{2(1-\alpha ^2)} \nonumber \\= & {} \sqrt{(1-\cos \theta )^2+\frac{ 2\gamma +2\alpha ^2(1-\cos \theta )}{1-\alpha ^2}}-\,(1-\cos \theta ) \end{aligned}$$

(152)

This is the fully general solution, from which a number of special cases can be derived by various simplifications.

• 1. Static peeling test:

  Setting $\alpha =0$, we obtain

  $$\begin{aligned} \varepsilon _c= & {} \sqrt{(1-\cos \theta )^2+ 2\gamma }-(1-\cos \theta ) \nonumber \\= & {} \sqrt{(1-\cos \theta )^2+ \frac{2G_{c0}}{Eh}}-(1-\cos \theta )\nonumber \\ \end{aligned}$$

  (153)
  $$\begin{aligned} F_c= & {} Ebh\varepsilon _c = b \sqrt{E^2h^2(1-\cos \theta )^2+ 2EhG_{c0}}\nonumber \\&-\,Ebh(1-\cos \theta ) \end{aligned}$$

  (154)

  This is the static solution, valid for slow tests in which the kinetic energy remains very small, and so the last term on the right-hand side of (144) can be neglected. A quadratic equation for $F_c$ that corresponds to such a reduced version of (144) was constructed by Kendall (1975), who then validated formula (154) experimentally by performing peeling experiments at various angles on sheets of ethylene propylene rubber crosslinked against a glass plate. In these tests, the rubber sheet thickness was $h=0.75$ mm and the elastic modulus measured in tensile tests was $E=1.21$ MPa. Kendall was aware of the fact that the debonding energy is actually rate-dependent and he adjusted the loading such that the crack speed was the same for all peeling angles (about 80 $\upmu$m/s). At this speed, the debonding energy taken from Gent and Kinloch (1971) was $G_{c0}=5$ J/m$^2$. The corresponding value of parameter $\gamma =G_{c0}/(Eh)=5.5\cdot 10^{-3}$ is much smaller than 1.

• 2. Static peeling test, stiff sheet:

  Setting $\alpha =0$ and $\gamma \ll 1$, we obtain

  $$\begin{aligned} \varepsilon _c= & {} (1-\cos \theta )\left( \sqrt{1+ \frac{2\gamma }{(1-\cos \theta )^2}}-1\right) \nonumber \\\approx & {} (1-\cos \theta )\frac{\gamma }{(1-\cos \theta )^2} = \frac{\gamma }{1-\cos \theta }\nonumber \\= & {} \frac{G_{c0}}{Eh(1-\cos \theta )} \end{aligned}$$

  (155)
  $$\begin{aligned} F_c= & {} Ebh\varepsilon _c \approx \frac{bG_{c0}}{1-\cos \theta } \end{aligned}$$

  (156)

  This case corresponds to a slow peeling test performed with a sheet which has a high axial stiffness (note that the assumption $\gamma \ll 1$ means that $Eh\gg G_{c0}$). In this case, the elastically stored energy is negligible compared to the dissipated energy, and the effect of axial strain on the external work (reflected in (144) by the term $F_c\varepsilon _c/b$) can be ignored, too. With these simplifications and under static conditions, the energy balance equation reduces to

  $$\begin{aligned} \frac{F_c}{b}(1-\cos \theta ) = G_{c0} \end{aligned}$$

  (157)

  and formula (156) can be deduced directly, with no need to solve a quadratic equation. In fact, the same result could also be obtained by neglecting the quadratic term in equation (151). For $\alpha =0$, the reduced linear equation for $\varepsilon _c$ reads

  $$\begin{aligned} 2(1-\cos \theta ) \varepsilon _c - 2 \gamma = 0 \end{aligned}$$

  (158)

  and its solution

  $$\begin{aligned} \varepsilon _c = \frac{\gamma }{1-\cos \theta } \end{aligned}$$

  (159)

  corresponds to (155).

• 3. Static mode-II delamination:

  Setting $\alpha =0$ and $\cos \theta =1$, we obtain

  $$\begin{aligned} \varepsilon _c= & {} \sqrt{2\gamma } =\sqrt{\frac{2G_{c0}}{Eh}} \end{aligned}$$

  (160)
  $$\begin{aligned} F_c= & {} Ebh\varepsilon _c = b\sqrt{2EhG_{c0}} \end{aligned}$$

  (161)

  In this case, the sheet is pulled in the direction parallel to the interface, which corresponds to the Mode-II delamination problem analyzed in the present paper. Naturally, the derived formula (161) corresponds to formula (53) from Sect. 4.1 (in which $F_c$ is denoted as $F_{c0}$). Note that in this case it would not be correct to simplify equation (144) by setting $\varepsilon _c=0$, even if the strain is actually small. The reason is that if angle $\theta$ vanishes, we have $\cos \theta =1$ and $\varepsilon _c$ is no longer negligible with respect to $1-\cos \theta$. Also, the term with $\varepsilon _c^2$ on the right-hand side of (144), which is related to the elastic energy, must not be deleted because it is of the same order as the left-hand side, which is related to external work. Since the left-hand side of (144) is proportional to the product of the force and strain, no external work would be supplied if the sheet were considered as infinitely stiff. This is a particular feature that distinguishes the special case of mode-II delamination ($\theta =0$) from the peeling test in an inclined direction ($\theta >0$). The elastic energy might be negligible for sufficiently large angles and stiff materials, but as the peeling angle tends to zero, this energy becomes important and in the limit case it is absolutely essential, as noticed already by Kendall (1975). The present formula (161) corresponds (in a somewhat different notation) to Kendall’s formula (6), and the present formula (156) corresponds to Kendall’s formula (5). In fact, the approximation used in (155) if $\gamma$ is small with respect to $(1-\cos \theta )^2$, so for small peeling angles it is not sufficient to check that $\gamma \ll 1$. Indeed, the peeling forces reported in Kendall (1975) are very well approximated by (156) for peeling angles for which $1-\cos \theta$ is 0.2 or greater (i.e., $(1-\cos \theta )^2$ is at least 0.04 and exceeds $\gamma =5.5\cdot 10^{-3}$ by an order of magnitude, but a clearly marked deviation is observed if $1-\cos \theta$ is at or below 0.1.

• 4. Dynamic mode-II delamination:

  Setting $\cos \theta =1$, we obtain

  $$\begin{aligned} \varepsilon _c= & {} \sqrt{\frac{2\gamma }{1-\alpha ^2}} =\sqrt{\frac{2G_{c0}}{Eh(1-\alpha ^2)}} \end{aligned}$$

  (162)
  $$\begin{aligned} F_c= & {} Ebh\varepsilon _c = b\sqrt{\frac{2EhG_{c0}}{1-\alpha ^2}} \end{aligned}$$

  (163)

  The derived expression for $F_c$ corresponds to equation (51) from Sect. 4.1, in which the applied force is denoted as $F_c^{(i)}$, to emphasize that the result is valid for a rate-independent delamination model.

• 5. Dynamic peeling test at moderate speed, stiff sheet:

  This simplification is based on assumptions that $\alpha ^2\ll 1$ and $\gamma \ll 1$, but the derivation requires detailed justification. In analogy to case 2, but this time with a nonzero value of the relative crack speed $\alpha$, it is assumed that the quadratic term in (151) is negligible with respect to the other terms. The reduced linear equation

  $$\begin{aligned} 2(1-\alpha ^2)(1-\cos \theta ) \varepsilon _c - 2 (\gamma +\alpha ^2(1-\cos \theta )) = 0 \end{aligned}$$

  (164)

  leads to

  $$\begin{aligned} \varepsilon _c= & {} \frac{\gamma +\alpha ^2(1-\cos \theta )}{(1-\alpha ^2)(1-\cos \theta )} = \frac{\gamma }{(1-\alpha ^2)(1-\cos \theta )} \nonumber \\&+ \frac{\alpha ^2}{1-\alpha ^2} \end{aligned}$$

  (165)

  The assumption that the quadratic term in (151) is negligible is justified if $\varepsilon _c\ll 1$. Looking at the resulting formula (165), we realize that the assumption is valid only if $\gamma \ll 1$ and $\alpha ^2\ll 1$. Consequently, as long as $\varepsilon _c$ remains small, the factors $1-\alpha ^2$ are very close to 1, and so (165) can be reduced to

  $$\begin{aligned} \varepsilon _c = \frac{\gamma }{1-\cos \theta } + \alpha ^2 \end{aligned}$$

  (166)

  The corresponding force

  $$\begin{aligned} F_c=Ebh\varepsilon _c = \frac{bG_{c0}}{1-\cos \theta } + \rho bh{\dot{a}}^2 \end{aligned}$$

  (167)

  agrees with the result that is obtained directly from (144) if $\varepsilon _c$ is set to zero and v is expressed from (146) with $\varepsilon _c$ set to zero. For ${\dot{a}}=0$, formula (167) reduces to (156), as expected. It is important to note that even though the static formula (156) is accurate whenever $G_{c0}\ll Eh$, its extension to the dynamic formula (167) is applicable only if the crack speed ${\dot{a}}$ is sufficiently small, such that ${\dot{a}}^2\ll c^2$. If this is not the case, the strain cannot remain small and it is better to evaluate it from the full formula (152). Of course, for large strains it becomes questionable whether the linear elastic law can still be used. To reflect the true material behavior, one would need to replace the quadratic expression for the elastic energy (i.e., the second term on the right-hand side of (144)) by a more appropriate hyperelastic potential.