Dual-channel recycling e-waste pricing decision under the impact of recyclers’ loss aversion and consumers’ bargaining power

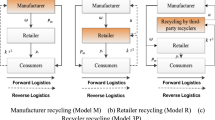
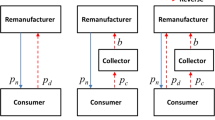
Abstract

The issue of e-waste recycling is imminent. With the continuous enhancement of Internet technology and people's awareness of sustainable development, the dual-channel reverse supply chain management combining online and offline becomes particularly important. In order to promote the sustainable development of dual-channel reverse supply chain recycling, this paper uses Stackelberg game and Rubinstein alternating offers bargaining game, and builds a dual-channel reverse supply chain model under the influence of loss aversion of recyclers and bargaining power of consumers. The purpose of this paper is to explore the recycling pricing decision of dual-channel reverse supply chain under the dual impact of loss aversion of recyclers and bargaining power of consumers. The results show that the increase in loss aversion of recyclers makes the recycling price of dual-channel recyclers decrease, but it increases their profits; the enhanced bargaining power of consumers raises the recycling price of products and increases the profits of members; the competition of recycling channels helps to improve the recycling price and profit of each member of the reverse supply chain. The results of this study provide theoretical basis for the members of dual-channel reverse supply chain to make optimal decisions in the case of bounded rationality, thereby promoting the sustainable development of reverse supply chain.

Appendices

Appendix A

The bargaining game process between the loss-averse online recycler and the loss-neutral remanufacturer is described as follows. At some point in $t \in T_{odd} = \left\{ {1,3 \ldots } \right\}$, the remanufacturer makes offer $\pi^{o} = (\pi_{M}^{o} ,\pi_{a}^{o} )$ and the online recycler decides whether to accept the offer or not. At some point in $t \in T_{even} = \left\{ {2,4 \ldots } \right\}$, the online recycler makes offer $\pi^{e} = (\pi_{M}^{e} ,\pi_{a}^{e} )$, and the remanufacturer decides whether to accept the online recycler's price offer. If the proposal $\pi^{*} = (\pi_{M}^{*} ,\pi_{a}^{*} )$ ($\pi_{M}^{*} + \pi_{a}^{*} = \pi_{SC}$) is accepted, the subgame process ends. If the offer is rejected, the game continues at the discount rate of $\delta (0 < \delta < 1)$ and ends with the probability of $1 - \delta$.

At the time of $t \in T_{odd}$, in order to make the online recycler accept the remanufacturer's proposal, the utility of the online recycler at the time of $t$ is required to be greater than or equal to the utility at the time of $t + 1$, namely:

$$(1 + \lambda_{a} )\pi_{a}^{o} - \lambda_{a} \max \left\{ {v_{a} ,\pi_{a}^{o} } \right\} \ge \delta \left[ {(1 + \lambda_{a} )\pi_{a}^{e} - \lambda_{a} \max \left\{ {\pi_{a}^{e} ,\max \left\{ {v_{a} ,\pi_{a}^{o} } \right\}} \right\}} \right] - (1 - \delta )\lambda_{a} \max \left\{ {v_{a} ,\pi_{a}^{o} } \right\}$$

(10)

At the time $t \in T_{even}$, in order for the remanufacturer to accept the recycler's proposal, the utility of the remanufacturer at the time t is required to be greater than or equal to the utility at the time $t + 1$, namely:

$$\pi_{M}^{e} \ge \delta \pi_{M}^{o}$$

(11)

For the loss aversion reference point $\lambda_{a}$, we assume that the initial reference point is 0. At any time t, the reference point is the highest historical transaction price, namely $v_{a}^{t} = \max \left\{ {0,\pi_{a}^{n} |n = 1,3 \ldots \le t} \right\}$.

In order to construct the perfect equilibrium point of the subgame, we assume that (10) and (11) are equations, and construct the three cases of (11):

(A) $v_{a} > \pi_{a}^{e} > \pi_{a}^{o}$, $\delta \pi_{a}^{e} = \pi_{a}^{o}$.

(B) $\pi_{a}^{e} \ge v_{a} > \pi_{a}^{o}$, $\delta \pi_{a}^{e} = (1 + \lambda_{a} )\pi_{a}^{o} - \delta \lambda_{a} v_{a}$.

(C) $\pi_{a}^{e} > \pi_{a}^{o} > v_{a}$,$\delta \pi_{a}^{e} = \left[ {1 + (1 - \delta )\lambda_{a} } \right]\pi_{a}^{o}$.

In the 11-A, $\pi^{o} = \left( {\frac{{\delta \pi_{sc} }}{\delta + 1},\frac{{\pi_{sc} }}{\delta + 1}} \right)$,$\pi^{e} = \left( {\frac{{\pi_{sc} }}{\delta + 1},\frac{{\delta \pi_{sc} }}{\delta + 1}} \right)$;In the 11-B,

$$\pi^{o} = \left( {\frac{{\delta (1 - \delta )\pi_{sc} + \delta \lambda_{a} v_{a} }}{{1 + \lambda_{a} - \delta^{2} }},\frac{{(1 + \lambda_{a} - \delta )\pi_{sc} - \delta \lambda_{a} v_{a} }}{{1 + \lambda_{a} - \delta^{2} }}} \right)$$

$$\pi^{e} = \left( {\frac{{(1 - \delta )(1 + \lambda_{a} )\pi_{sc} + \delta^{2} \lambda_{a} v_{a} }}{{1 + \lambda_{a} - \delta^{2} }},\frac{{\delta (1 + \lambda_{a} - \delta )\pi_{sc} - \delta^{2} \lambda_{a} v_{a} }}{{1 + \lambda_{a} - \delta^{2} }}} \right)$$

In the 11-C,$\pi^{o} = \left( {\frac{{\delta \pi_{sc} }}{{1 + \lambda_{a} + \delta }},\frac{{(1 + \lambda_{a} )\pi_{sc} }}{{1 + \lambda_{a} + \delta }}} \right)$$\pi^{e} = \left( {\frac{{\left[ {1 + (1 - \delta )\lambda_{a} } \right]\pi_{sc} }}{{1 + \lambda_{a} + \delta }},\frac{{\delta (1 + \lambda_{a} )\pi_{sc} }}{{1 + \lambda_{a} + \delta }}} \right)$.

The equilibrium result in 11-A is the same as the result in classic Rubinstein's that both sides of the game are risk neutral. As for the equilibrium result in 11-B, the reference point of loss aversion is taken as the influence factor, indicating that this equilibrium point is not unique. Therefore, neither 11-A nor 11-B can be regarded as loss aversion reference points for online recyclers. The equilibrium point of 11-B is unique, so the perfect equilibrium point of the subgame is $\pi^{e} = \left( {\frac{{\left[ {1 + (1 - \delta )\lambda_{a} } \right]\pi_{sc} }}{{1 + \lambda_{a} + \delta }},\frac{{\delta (1 + \lambda_{a} )\pi_{sc} }}{{1 + \lambda_{a} + \delta }}} \right)$. The reference point for the online recycler is $v_{a}^{*} = \frac{{\delta (1 + \lambda_{a} )\pi_{SC}^{a} }}{{1 + \lambda_{a} + \delta }}$.

Similarly, we can get the perfect equilibrium point of the subgame of offline recyclers is $\pi^{e} = \left( {\frac{{\left[ {1 + (1 - \delta )\lambda_{b} } \right]\pi_{sc} }}{{1 + \lambda_{b} + \delta }},\frac{{\delta (1 + \lambda_{b} )\pi_{sc} }}{{1 + \lambda_{b} + \delta }}} \right)$.The reference point of offline recyclers is $v_{b}^{*} = \frac{{\delta (1 + \lambda_{b} )\pi_{SC}^{b} }}{{1 + \lambda_{b} + \delta }}$.

Appendix B

2.1 Proof of Proposition 1

Since $0 < l < 1,\beta > 0$, we get $\frac{{\partial^{2} \pi_{sc}^{a} }}{{\partial^{2} p_{a} }} = - 2\left( {l + \beta } \right) < 0$, indicating that $\pi_{SC}^{a}$ is a concave function about the variable $p_{a}$, and there is a unique solution. And because $0 < \rho < 1$, then $\frac{{\partial^{2} \pi_{sc}^{b} }}{{\partial^{2} p_{b} }} = - 2\left( {l + \beta } \right)\left( {1 + \rho } \right) < 0$, indicating that $\pi_{SC}^{b}$ is a concave function about the variable $p_{b}$, and there is a unique solution.

2.2 Proof of Proposition 2

From $0 < \delta < 1$ and $0 < \lambda_{i} < 2(i = a,b)$, it can be seen $\frac{{\partial v_{a}^{*} }}{{\partial \lambda_{a} }} = \frac{{\delta^{2} \pi_{SC}^{a} }}{{\left( {1 + \delta + \lambda_{a} } \right)^{2} }} > 0$ $\frac{{\partial v_{a}^{*} }}{\partial \delta } = \frac{{\pi_{SC}^{a} \left( {1 + \lambda_{a} } \right)^{2} }}{{\left( {1 + \delta + \lambda_{a} } \right)^{2} }} > 0$ $\frac{{\partial v_{b}^{*} }}{{\partial \lambda_{b} }} = \frac{{\delta^{2} \pi_{SC}^{b} }}{{\left( {1 + \delta + \lambda_{b} } \right)^{2} }} > 0$ $\frac{{\partial v_{b}^{*} }}{\partial \delta } = \frac{{\pi_{SC}^{b} \left( {1 + \lambda_{b} } \right)^{2} }}{{\left( {1 + \delta + \lambda_{b} } \right)^{2} }} > 0$.

2.3 Proof of Proposition 3

According to Eqs. (4) and (5), the first-order derivative of the recovery price is obtained.$\frac{{\partial \pi_{a} }}{{\partial p_{a} }} = - \alpha - \left( {l + \beta } \right)c_{a} - 2\left( {l + \beta } \right)p_{a} + lp_{b} + lp_{t} + \beta p_{t}$ $\frac{{\partial \pi_{b} }}{{\partial p_{b} }} = - \alpha + l\left( {1 + \rho } \right)\left( {p_{a} - p_{b} } \right) - \beta \left( {1 + \rho } \right)p_{b} - \left( {l + \beta } \right)\left( {1 + \rho } \right)\left( {c_{b} + p_{b} - p_{t} } \right)$.

In combination with $\frac{{\partial \pi_{a} }}{{\partial p_{a} }}{ = }0$,$\frac{{\partial \pi_{b} }}{{\partial p_{b} }}{ = }0$ we get

$$\left\{ {\begin{array}{*{20}l} {p_{a} = - \frac{\begin{gathered} - \left( { - l\left( {1 + \rho } \right) - \beta \left( {1 + \rho } \right) - \left( {l + \beta } \right)\left( {1 + \rho } \right)} \right)\left( { - \alpha - \left( {l + \beta } \right)c_{a} + lp_{t} + \beta p_{t} } \right) \hfill \\ + l\left( { - \alpha - \left( {l + \beta } \right)\left( {1 + \rho } \right)c_{b} + \left( {l + \beta } \right)\left( {1 + \rho } \right)p_{t} } \right) \hfill \\ \end{gathered} }{{ - 3l^{2} - 8l\beta - 4\beta^{2} - 3l^{2} \rho - 8l\beta \rho - 4\beta^{2} \rho }}} \hfill \\ {p_{b} = - \frac{\begin{gathered} 3l\alpha + 2\alpha \beta + l\alpha \rho + l^{2} c_{a} + l\beta c_{a} + l^{2} \rho c_{a} + l\beta \rho c_{a} + 2l^{2} c_{b} + 4l\beta c_{b} + 2\beta^{2} c_{b} \hfill \\ + 2l^{2} \rho c_{b} + 4l\beta \rho c_{b} + 2\beta^{2} \rho c_{b} - 3l^{2} p_{t} - 5l\beta p_{t} - 2\beta^{2} p_{t} - 3l^{2} \rho p_{t} - 5l\beta \rho p_{t} - 2\beta^{2} \rho p_{t} \hfill \\ \end{gathered} }{{\left( {3l^{2} + 8l\beta + 4\beta^{2} } \right)\left( {1 + \rho } \right)}}} \hfill \\ \end{array} } \right.$$

(12)

Substitute (12) into Eq. (1), and

$$\pi_{M} = - \frac{\begin{gathered} \left( {H - p_{t} } \right)( - 2\alpha \left( {l + 2\beta } \right)\left( {3l + 2\beta } \right)\left( {1 + \rho } \right) + \left( {\beta - l\rho } \right)(2\left( {l + \beta } \right)\left( {1 + \rho } \right) \hfill \\ \left( {\alpha + \left( {l + \beta } \right)\left( {c_{a} - p_{t} } \right)} \right) + l\left( {\alpha + \left( {l + \beta } \right)\left( {1 + \rho } \right)\left( {c_{b} - p_{t} } \right)} \right)) \hfill \\ + \left( {\beta + l\rho + \beta \rho } \right)\left( {\alpha \left( {2\beta + l\left( {3 + \rho } \right)} \right) + \left( {l + \beta } \right)\left( {1 + \rho } \right)\left( {lc_{a} + 2\left( {l + \beta } \right)c_{b} - \left( {3l + 2\beta } \right)p_{t} } \right)} \right)) \hfill \\ \end{gathered} }{{\left( {l + 2\beta } \right)\left( {3l + 2\beta } \right)\left( {1 + \rho } \right)}}$$

(13)

Set $\frac{{\partial \pi_{M} }}{{\partial p_{t} }}{ = }0$ to obtain the optimal recovery transfer price of the remanufacturer

$$p_{t}^{N} = \frac{\begin{gathered} 2\beta \left( {1 + \rho } \right)\left( { - 2\alpha + H\beta \left( {2 + \rho } \right)} \right) + l\left( {3H\beta \left( {1 + \rho } \right)\left( {2 + \rho } \right) - \alpha \left( {6 + \rho \left( {6 + \rho } \right)} \right)} \right) \hfill \\ - \left( {1 + \rho } \right)\left( { - \beta \left( {3l + 2\beta } \right) + l\left( {l + \beta } \right)\rho } \right)c_{a} + \left( {1 + \rho } \right)\left( {l^{2} \rho + 2\beta^{2} \left( {1 + \rho } \right) + l\beta \left( {3 + 4\rho } \right)} \right)c_{b} \hfill \\ \end{gathered} }{{2\beta \left( {3l + 2\beta } \right)\left( {1 + \rho } \right)\left( {2 + \rho } \right)}}$$

(14)

Substitute $p_{t}^{N}$ into Eq. (12) to obtain the optimal online and offline recovery prices, respectively, as follows:

$$p_{a}^{N} = \frac{\begin{gathered} - 2\beta^{2} \left( {1 + \rho } \right)\left( { - H\beta \left( {2 + \rho } \right) + 2\alpha \left( {3 + \rho } \right)} \right) + l^{2} \left( \begin{gathered} 3H\beta \left( {1 + \rho } \right)\left( {2 + \rho } \right) \hfill \\ - \alpha \left( {6 + \rho \left( {6 + \rho } \right)} \right) \hfill \\ \end{gathered} \right) \hfill \\ + l\beta \left( {5H\beta \left( {1 + \rho } \right)\left( {2 + \rho } \right) - \alpha \left( {22 + \rho \left( {24 + 5\rho } \right)} \right)} \right) - \left( {l + \beta } \right)\left( {1 + \rho } \right) \hfill \\ \left( {\left( {\beta \left( {5l + 6\beta } \right) + \left( {l + \beta } \right)\left( {l + 4\beta } \right)\rho } \right)c_{a} - \left( {l^{2} \rho + 2\beta^{2} \left( {1 + \rho } \right) + l\beta \left( { - 1 + 2\rho } \right)} \right)c_{b} } \right) \hfill \\ \end{gathered} }{{2\beta \left( {l + 2\beta } \right)\left( {3l + 2\beta } \right)\left( {1 + \rho } \right)\left( {2 + \rho } \right)}}$$

(15)

$$p_{b}^{N} = - \frac{\begin{gathered} 2\alpha \beta \left( {2 + \rho } \right)\left( {2\beta + l\left( {3 + \rho } \right)} \right) + \left( {l + \beta } \right)( - 2\beta \left( {1 + \rho } \right)\left( { - 2\alpha + H\beta \left( {2 + \rho } \right)} \right) \hfill \\ + l\left( { - 3H\beta \left( {1 + \rho } \right)\left( {2 + \rho } \right) + \alpha \left( {6 + \rho \left( {6 + \rho } \right)} \right)} \right) \hfill \\ + \left( {1 + \rho } \right)\left( {\left( {\left( {l - 2\beta } \right)\beta + l\left( {l + 3\beta } \right)\rho } \right)c_{a} + \left( {5l\beta - l^{2} \rho + 2\beta^{2} \left( {3 + \rho } \right)} \right)c_{b} } \right)) \hfill \\ \end{gathered} }{{2\beta \left( {l + 2\beta } \right)\left( {3l + 2\beta } \right)\left( {1 + \rho } \right)\left( {2 + \rho } \right)}}$$

(16)

At this point, the optimal recovery profits of online and offline recyclers are, respectively

$$\pi_{a}^{N} = \frac{\begin{gathered} \left( {l + \beta } \right)(2\beta \left( {1 + \rho } \right)\left( {2\alpha \left( {1 + \rho } \right) + H\beta \left( {2 + \rho } \right)} \right) + l\left( \begin{gathered} 3H\beta \left( {1 + \rho } \right)\left( {2 + \rho } \right) \hfill \\ + \alpha \left( {6 + \rho \left( {8 + 3\rho } \right)} \right) \hfill \\ \end{gathered} \right) \hfill \\ - \left( {1 + \rho } \right)\left( {2\beta^{2} \left( {3 + 2\rho } \right) + l^{2} \left( {4 + 3\rho } \right) + l\beta \left( {13 + 9\rho } \right)} \right)c_{a} \hfill \\ + \left( {1 + \rho } \right)\left( {2\beta^{2} \left( {1 + \rho } \right) + l^{2} \left( {4 + 3\rho } \right) + l\beta \left( {7 + 6\rho } \right)} \right)c_{b} )^{2} \hfill \\ \end{gathered} }{{4\left( {l + 2\beta } \right)^{2} \left( {3l + 2\beta } \right)^{2} \left( {1 + \rho } \right)^{2} \left( {2 + \rho } \right)^{2} }}$$

(17)

$$\pi_{b}^{N} = \frac{{\left( {l + \beta } \right)\left( \begin{gathered} 4\alpha \beta + 3Hl\beta \left( {1 + \rho } \right)\left( {2 + \rho } \right) + 2H\beta^{2} \left( {1 + \rho } \right)\left( {2 + \rho } \right) \hfill \\ + l\alpha \left( {6 + \rho \left( {4 + \rho } \right)} \right) + \left( {1 + \rho } \right)\left( \begin{gathered} \left( {2\beta^{2} + l^{2} \left( {4 + \rho } \right) + l\beta \left( {7 + \rho } \right)} \right)c_{a} \hfill \\ - \left( {2\beta^{2} \left( {3 + \rho } \right) + l^{2} \left( {4 + \rho } \right) + l\beta \left( {13 + 4\rho } \right)} \right)c_{b} \hfill \\ \end{gathered} \right) \hfill \\ \end{gathered} \right)^{2} }}{{4\left( {l + 2\beta } \right)^{2} \left( {3l + 2\beta } \right)^{2} \left( {1 + \rho } \right)\left( {2 + \rho } \right)^{2} }}$$

(18)

2.4 Proof of Proposition 4

From $0 < \delta < 1$, we get $- 1 - \delta < 0, - 1 + \delta < 0$.

It can be seen that $\frac{{\partial^{2} U_{a} }}{{\partial^{2} p_{a} }}{ = }\frac{{2\left( {l + \beta } \right)\left( {1 + \lambda_{a} } \right)\left( { - 1 - \delta + \left( { - 1 + \delta } \right)\lambda_{a} } \right)}}{{1 + \delta + \lambda_{a} }} < 0$, indicating that $U_{a}$ is a concave function about the variable $p_{a}$, and there is a unique solution.

2.5 Proof of Proposition 5

By substituting $p_{t}^{N}$ and $p_{b}^{N}$ into Eq. (8) from Proposition 3 and 4, let $\frac{{\partial U_{a} }}{{\partial p_{a} }}{ = }0$, and the solution is obtained

$$p_{a}^{*} = \frac{A}{{4\beta \left( {l + 2\beta } \right)\left( {3l + 2\beta } \right)\left( {1 + \rho } \right)\left( {2 + \rho } \right)\left( { - 1 - \delta + \left( { - 1 + \delta } \right)\lambda_{a} } \right)}},$$

$$\pi_{a}^{*} = - \frac{{\left( {l + \beta } \right)\left( {1 + \lambda_{a} } \right)B^{2} }}{{16\beta^{2} \left( {l + 2\beta } \right)^{2} \left( {3l + 2\beta } \right)^{2} \left( {1 + \rho } \right)^{2} \left( {2 + \rho } \right)^{2} \left( {1 + \delta + \lambda_{a} } \right)\left( { - 1 - \delta + \left( { - 1 + \delta } \right)\lambda_{a} } \right)}}$$

Here the simplification for A and B are as follows:

$$\begin{aligned} A = & 2\left( {1 + \delta } \right)\left( \begin{gathered} - 2\beta^{2} \left( {1 + \rho } \right)\left( {H\beta \left( {2 + \rho } \right) - 2\alpha \left( {3 + \rho } \right)} \right) + l^{2} \left( { - 3H\beta \left( {1 + \rho } \right)\left( {2 + \rho } \right) + \alpha \left( {6 + \rho \left( {6 + \rho } \right)} \right)} \right) \hfill \\ + l\beta \left( { - 5H\beta \left( {1 + \rho } \right)\left( {2 + \rho } \right) + \alpha \left( {22 + \rho \left( {24 + 5\rho } \right)} \right)} \right) \hfill \\ \end{gathered} \right) \\ & + (4\beta^{2} \left( {1 + \rho } \right)\left( {H\beta \left( { - 1 + 2\delta } \right)\left( {2 + \rho } \right) + 2\alpha \left( {3 + \rho - \delta \left( {2 + \rho } \right)} \right)} \right) + l^{2} \left( \begin{gathered} 3H\beta \left( { - 2 + 3\delta } \right)\left( {1 + \rho } \right) \hfill \\ \left( {2 + \rho } \right) - \alpha \left( { - 2 + \delta } \right)\left( {6 + \rho \left( {6 + \rho } \right)} \right) \hfill \\ \end{gathered} \right) \\ & + 2l\beta \left( {H\beta \left( { - 5 + 9\delta } \right)\left( {1 + \rho } \right)\left( {2 + \rho } \right) + \alpha \left( {22 + \rho \left( {24 + 5\rho } \right) - 2\delta \left( {7 + 2\rho \left( {4 + \rho } \right)} \right)} \right)} \right))\lambda_{a} \\ & + \left( {1 + \rho } \right)c_{b} \left( \begin{gathered} - 2\left( {l + \beta } \right)\left( {1 + \delta } \right)\left( {l^{2} \rho + 2\beta^{2} \left( {1 + \rho } \right) + l\beta \left( { - 1 + 2\rho } \right)} \right) \hfill \\ + \left( {l^{2} \beta \left( {2 - 5\delta - 6\rho } \right) + l^{3} \left( { - 2 + \delta } \right)\rho - 4\beta^{3} \left( {1 + \rho } \right) - 2l\beta^{2} \left( {1 + 3\delta + \left( {4 + \delta } \right)\rho } \right)} \right)\lambda_{a} \hfill \\ \end{gathered} \right) + \left( {1 + \rho } \right)c_{a} \\ & \left( {2\left( {l + \beta } \right)\left( {1 + \delta } \right)\left( {\beta \left( {5l + 6\beta } \right) + \left( {l + \beta } \right)\left( {l + 4\beta } \right)\rho } \right) + \left( \begin{gathered} - l^{3} \left( { - 2 + \delta } \right)\rho + l^{2} \beta \left( {10 - 13\delta + 12\rho - 9\delta \rho } \right) + 2l\beta^{2} \hfill \\ \left( {11 - 15\delta + 9\rho - 8\delta \rho } \right) + 4\beta^{3} \left( {3 + 2\rho - 2\delta \left( {2 + \rho } \right)} \right) \hfill \\ \end{gathered} \right)\lambda_{a} } \right) \\ \end{aligned}$$

$\begin{aligned} B = & 12l\alpha \beta + 12Hl\beta ^{2} + 8\alpha \beta ^{2} + 8H\beta ^{3} + 12l\alpha \beta \delta + 12Hl\beta ^{2} \delta + 8\alpha \beta ^{2} \delta + 8H\beta ^{3} \delta + 16l\alpha \beta \rho + 18Hl\beta ^{2} \rho \\ & + 16\alpha \beta ^{2} \rho + 12H\beta ^{3} \rho + 16l\alpha \beta \delta \rho + 18Hl\beta ^{2} \delta \rho + 16\alpha \beta ^{2} \delta \rho + 12H\beta ^{3} \delta \rho + 6l\alpha \beta \rho ^{2} + 6Hl\beta ^{2} \rho ^{2} + 8\alpha \beta ^{2} \rho ^{2} \\ & + 4H\beta ^{3} \rho ^{2} + 6l\alpha \beta \delta \rho ^{2} + 6Hl\beta ^{2} \delta \rho ^{2} + 8\alpha \beta ^{2} \delta \rho ^{2} + 4H\beta ^{3} \delta \rho ^{2} + 12l\alpha \beta \lambda _{a} + 12Hl\beta ^{2} \lambda _{a} + 8\alpha \beta ^{2} \lambda _{a} + 8H\beta ^{3} \lambda _{a} \\ & - 6l^{2} \alpha \delta \lambda _{a} - 6Hl^{2} \beta \delta \lambda _{a} - 28l\alpha \beta \delta \lambda _{a} - 28Hl\beta ^{2} \delta \lambda _{a} - 16\alpha \beta ^{2} \delta \lambda _{a} - 16H\beta ^{3} \delta \lambda _{a} + 16l\alpha \beta \rho \lambda _{a} + 18Hl\beta ^{2} \rho \lambda _{a} \\ & + 16\alpha \beta ^{2} \rho \lambda _{a} + 12H\beta ^{3} \rho \lambda _{a} - 6l^{2} \alpha \delta \rho \lambda _{a} - 9Hl^{2} \beta \delta \rho \lambda _{a} - 32l\alpha \beta \delta \rho \lambda _{a} - 42Hl\beta ^{2} \delta \rho \lambda _{a} - 24\alpha \beta ^{2} \delta \rho \lambda _{a} - 24H\beta ^{3} \delta \rho \lambda _{a} \\ & + 6l\alpha \beta \rho ^{2} \lambda _{a} + 6Hl\beta ^{2} \rho ^{2} \lambda _{a} + 8\alpha \beta ^{2} \rho ^{2} \lambda _{a} + 4H\beta ^{3} \rho ^{2} \lambda _{a} - l^{2} \alpha \delta \rho ^{2} \lambda _{a} - 3Hl^{2} \beta \delta \rho ^{2} \lambda _{a} - 8l\alpha \beta \delta \rho ^{2} \lambda _{a} - 14Hl\beta ^{2} \delta \rho ^{2} \lambda _{a} \\ & - 8\alpha \beta ^{2} \delta \rho ^{2} \lambda _{a} - 8H\beta ^{3} \delta \rho ^{2} \lambda _{a} + \left( {1 + \rho } \right)c_{b} \\ & *\left( \begin{gathered} 2\beta \left( {1 + \delta } \right)\left( {2\beta ^{2} \left( {1 + \rho } \right) + l^{2} \left( {4 + 3\rho } \right) + l\beta \left( {7 + 6\rho } \right)} \right) \hfill \\ + \left( {l^{3} \delta \rho + 4\beta ^{3} \left( {1 + \rho } \right) + l^{2} \beta \left( {8 - 5\delta + 6\rho } \right) - 2l\beta ^{2} \left( { - 7 - 6\rho + \delta \left( {3 + \rho } \right)} \right)} \right)\lambda _{a} \hfill \\ \end{gathered} \right) \\ & - \left( {1 + \rho } \right)c_{a} (2\beta \left( {1 + \delta } \right)\left( {2\beta ^{2} \left( {3 + 2\rho } \right) + l^{2} \left( {4 + 3\rho } \right) + l\beta \left( {13 + 9\rho } \right)} \right) + (l^{3} \delta \rho + 4\beta ^{3} \left( {3 + 2\rho - 2\delta \left( {2 + \rho } \right)} \right) \\ & + l^{2} \beta \left( {8 + 6\rho - \delta \left( {11 + 3\rho } \right)} \right) - 2l\beta ^{2} \left( { - 13 - 9\rho + \delta \left( {17 + 8\rho } \right)} \right))\lambda _{a} ) \\ \end{aligned}$

2.6 Proof of Proposition 6

We can see from proposition 4 that $\frac{{\partial^{2} U_{b} }}{{\partial^{2} p_{b} }} = \frac{{2\left( {l + \beta } \right)\left( {1 + \rho } \right)\left( {1 + \lambda_{b} } \right)\left( { - 1 - \delta + \left( { - 1 + \delta } \right)\lambda_{b} } \right)}}{{1 + \delta + \lambda_{b} }} < 0$, indicating that $U_{b}$ is a concave function about the variable $p_{b}$, and there is a unique solution.

2.7 Proof of Proposition 7

Substitute $p_{t}^{N}$ and $p_{b}^{N}$ into Eq. (9), and let $\frac{{\partial U_{b} }}{{\partial p_{b} }}{ = }0$ to obtain the optimal solution of the offline recycler:

$$p_{b}^{*} = - \frac{C}{{4\beta \left( {l + 2\beta } \right)\left( {3l + 2\beta } \right)\left( {1 + \rho } \right)\left( {2 + \rho } \right)\left( { - 1 - \delta + \left( { - 1 + \delta } \right)\lambda_{b} } \right)}}$$

$$\pi_{b}^{*} = - \frac{{\left( {l + \beta } \right)\left( {1 + \lambda_{b} } \right)D^{2} }}{{16\beta^{2} \left( {l + 2\beta } \right)^{2} \left( {3l + 2\beta } \right)^{2} \left( {1 + \rho } \right)\left( {2 + \rho } \right)^{2} \left( {1 + \delta + \lambda_{b} } \right)\left( { - 1 - \delta + \left( { - 1 + \delta } \right)\lambda_{b} } \right)}}$$

Here the simplification for C and D are as follows:

$$\begin{aligned} C = & 2\left( {1 + \delta } \right)\left( \begin{gathered} 2\beta^{2} \left( {H\beta \left( {1 + \rho } \right)\left( {2 + \rho } \right) - 2\alpha \left( {3 + 2\rho } \right)} \right) + l^{2} \left( {3H\beta \left( {1 + \rho } \right)\left( {2 + \rho } \right) - \alpha \left( {6 + \rho \left( {6 + \rho } \right)} \right)} \right) \hfill \\ + l\beta \left( {5H\beta \left( {1 + \rho } \right)\left( {2 + \rho } \right) - \alpha \left( {22 + \rho \left( {20 + 3\rho } \right)} \right)} \right) \hfill \\ \end{gathered} \right) \\ & + ( - 4\beta^{2} \left( {H\beta \left( { - 1 + 2\delta } \right)\left( {1 + \rho } \right)\left( {2 + \rho } \right) + \alpha \left( {6 + 4\rho - 2\delta \left( {2 + \rho } \right)} \right)} \right) + l^{2} \left( \begin{gathered} - 3H\beta \left( { - 2 + 3\delta } \right)\left( {1 + \rho } \right)\left( {2 + \rho } \right) \hfill \\ + \alpha \left( { - 2 + \delta } \right)\left( {6 + \rho \left( {6 + \rho } \right)} \right) \hfill \\ \end{gathered} \right) \\ & + 2l\beta \left( { - H\beta \left( { - 5 + 9\delta } \right)\left( {1 + \rho } \right)\left( {2 + \rho } \right) + \alpha \left( { - 22 - \rho \left( {20 + 3\rho } \right) + 2\delta \left( {7 + \rho \left( {6 + \rho } \right)} \right)} \right)} \right))\lambda_{b} + \left( {1 + \rho } \right)c_{a} \\ & \left( { - 2\left( {l + \beta } \right)\left( {1 + \delta } \right)\left( {\left( {l - 2\beta } \right)\beta + l\left( {l + 3\beta } \right)\rho } \right) + \left( \begin{gathered} \beta \left( {4\beta^{2} + l^{2} \left( { - 2 + 5\delta } \right) + 2l\left( {\beta + 3\beta \delta } \right)} \right) \hfill \\ + l\left( {l + \beta } \right)\left( { - 6\beta + l\left( { - 2 + \delta } \right) + 4\beta \delta } \right)\rho \hfill \\ \end{gathered} \right)\lambda_{b} } \right) + \left( {1 + \rho } \right)c_{b} \\ & \left( {2\left( {l + \beta } \right)\left( {1 + \delta } \right)\left( { - 5l\beta + l^{2} \rho - 2\beta^{2} \left( {3 + \rho } \right)} \right) + \left( \begin{gathered} - l^{3} \left( { - 2 + \delta } \right)\rho + l^{2} \beta \left( { - 10 + 13\delta + 2\rho + 4\delta \rho } \right) \hfill \\ + 2l\beta^{2} \left( { - 11 + 15\delta - 2\rho + 7\delta \rho } \right) + 4\beta^{3} \left( { - 3 - \rho + 2\delta \left( {2 + \rho } \right)} \right) \hfill \\ \end{gathered} \right)\lambda_{b} } \right) \\ \end{aligned}$$

$$\begin{aligned} D = & 12l\alpha \beta + 12Hl\beta^{2} + 8\alpha \beta^{2} + 8H\beta^{3} + 12l\alpha \beta \delta + 12Hl\beta^{2} \delta + 8\alpha \beta^{2} \delta + 8H\beta^{3} \delta + 8l\alpha \beta \rho + 18Hl\beta^{2} \rho + 12H\beta^{3} \rho \\ & + 8l\alpha \beta \delta \rho + 18Hl\beta^{2} \delta \rho + 12H\beta^{3} \delta \rho + 2l\alpha \beta \rho^{2} + 6Hl\beta^{2} \rho^{2} + 4H\beta^{3} \rho^{2} + 2l\alpha \beta \delta \rho^{2} + 6Hl\beta^{2} \delta \rho^{2} + 4H\beta^{3} \delta \rho^{2} \\ & + 12l\alpha \beta \lambda_{b} + 12Hl\beta^{2} \lambda_{b} + 8\alpha \beta^{2} \lambda_{b} + 8H\beta^{3} \lambda_{b} - 6l^{2} \alpha \delta \lambda_{b} - 6Hl^{2} \beta \delta \lambda_{b} - 28l\alpha \beta \delta \lambda_{b} - 28Hl\beta^{2} \delta \lambda_{b} - 16\alpha \beta^{2} \delta \lambda_{b} \\ & - 16H\beta^{3} \delta \lambda_{b} + 8l\alpha \beta \rho \lambda_{b} + 18Hl\beta^{2} \rho \lambda_{b} + 12H\beta^{3} \rho \lambda_{b} - 6l^{2} \alpha \delta \rho \lambda_{b} - 9Hl^{2} \beta \delta \rho \lambda_{b} - 24l\alpha \beta \delta \rho \lambda_{b} - 42Hl\beta^{2} \delta \rho \lambda_{b} \\ & - 8\alpha \beta^{2} \delta \rho \lambda_{b} - 24H\beta^{3} \delta \rho \lambda_{b} + 2l\alpha \beta \rho^{2} \lambda_{b} + 6Hl\beta^{2} \rho^{2} \lambda_{b} + 4H\beta^{3} \rho^{2} \lambda_{b} - l^{2} \alpha \delta \rho^{2} \lambda_{b} - 3Hl^{2} \beta \delta \rho^{2} \lambda_{b} - 4l\alpha \beta \delta \rho^{2} \lambda_{b} \\ & - 14Hl\beta^{2} \delta \rho^{2} \lambda_{b} - 8H\beta^{3} \delta \rho^{2} \lambda_{b} - \left( {1 + \rho } \right)c_{a} ( - 2\beta \left( {1 + \delta } \right)\left( {2\beta^{2} + l^{2} \left( {4 + \rho } \right) + l\beta \left( {7 + \rho } \right)} \right) \\ & + \left( { - 4\beta^{3} + l^{3} \delta \rho + l^{2} \beta \left( {5\delta \left( {1 + \rho } \right) - 2\left( {4 + \rho } \right)} \right) + 2l\beta^{2} \left( { - 7 - \rho + \delta \left( {3 + 2\rho } \right)} \right)} \right)\lambda_{b} ) + \left( {1 + \rho } \right)c_{b} ( - 2\beta \left( {1 + \delta } \right) \\ & \left( {2\beta^{2} \left( {3 + \rho } \right) + l^{2} \left( {4 + \rho } \right) + l\beta \left( {13 + 4\rho } \right)} \right) + \left( \begin{gathered} l^{3} \delta \rho + 4\beta^{3} \left( { - 3 - \rho + 2\delta \left( {2 + \rho } \right)} \right) + l^{2} \beta \hfill \\ \left( { - 2\left( {4 + \rho } \right) + \delta \left( {11 + 8\rho } \right)} \right) + 2l\beta^{2} \left( { - 13 - 4\rho + \delta \left( {17 + 9\rho } \right)} \right) \hfill \\ \end{gathered} \right)\lambda_{b} ) \\ \end{aligned}$$

2.8 Proof of Conclusion 1

From $0 < c_{a} < c_{b} < H$, we get

$\frac{{\partial p_{a} }}{{\partial \lambda_{a} }} = \frac{{\partial p_{b} }}{{\partial \lambda_{b} }} = - \frac{\begin{gathered} \delta \left( {1 + \delta } \right)(6l\alpha + 6Hl\beta + 4\alpha \beta + 4H\beta^{2} + 6l\alpha \rho + 9Hl\beta \rho + 4\alpha \beta \rho \hfill \\ + 6H\beta^{2} \rho + l\alpha \rho^{2} + 3Hl\beta \rho^{2} + 2H\beta^{2} \rho^{2} + \left( {1 + \rho } \right)\left( { - 2\beta^{2} + l\beta \left( { - 3 + \rho } \right) + l^{2} \rho } \right)c_{a} \hfill \\ - \left( {1 + \rho } \right)\left( {l^{2} \rho + 2\beta^{2} \left( {1 + \rho } \right) + l\beta \left( {3 + 4\rho } \right)} \right)c_{b} ) \hfill \\ \end{gathered} }{{4\beta \left( {3l + 2\beta } \right)\left( {1 + \rho } \right)\left( {2 + \rho } \right)\left( {1 + \delta - \left( { - 1 + \delta } \right)\lambda_{a} } \right)^{2} }} < 0$.

Since $- 2 < - 2 + \delta < - 1$ and $- 1 - \delta < 0, - 1 + \delta < 0$, we get

$$\frac{{\partial p_{a} }}{\partial \rho } = \frac{\begin{gathered} \left( {2l^{2} + 5l\beta + 2\beta^{2} } \right)\left( {1 + \rho } \right)^{2} \left( { - 2\left( {l + \beta } \right)\left( {1 + \delta } \right) + \left( { - 2\beta + l\left( { - 2 + \delta } \right)} \right)\lambda_{a} } \right)(c_{b} - c_{a} ) \hfill \\ + \alpha \left( \begin{gathered} - 2\left( {1 + \delta } \right)\left( {4\beta^{2} \left( {1 + \rho } \right)^{2} + l^{2} \left( {6 + 8\rho + 3\rho^{2} } \right) + 3l\beta \left( {6 + 8\rho + 3\rho^{2} } \right)} \right) \hfill \\ + \left( { - 8\beta^{2} \left( {1 + \rho } \right)^{2} + 4l\beta \delta \left( {5 + 6\rho + 2\rho^{2} } \right) - 6l\beta \left( {6 + 8\rho + 3\rho^{2} } \right) + l^{2} \left( { - 2 + \delta } \right)\left( {6 + 8\rho + 3\rho^{2} } \right)} \right)\lambda_{a} \hfill \\ \end{gathered} \right) \hfill \\ \end{gathered} }{{4\beta \left( {l + 2\beta } \right)\left( {3l + 2\beta } \right)\left( {1 + \rho } \right)^{2} \left( {2 + \rho } \right)^{2} \left( { - 1 - \delta + \left( { - 1 + \delta } \right)\lambda_{a} } \right)}} > 0$$

$\frac{{\partial p_{b} }}{\partial \rho } = \frac{\begin{gathered} \left( {2l^{2} + 5l\beta + 2\beta^{2} } \right)\left( {1 + \rho } \right)^{2} \left( { - 2\left( {l + \beta } \right)\left( {1 + \delta } \right) + \left( { - 2\beta + l\left( { - 2 + \delta } \right)} \right)\lambda_{b} } \right)(c_{b} - c_{a} ) \hfill \\ + \alpha ( - 2\left( {1 + \delta } \right)\left( {4\beta^{2} \left( {5 + 6\rho + 2\rho^{2} } \right) + l^{2} \left( {6 + 8\rho + 3\rho^{2} } \right) + l\beta \left( {26 + 32\rho + 11\rho^{2} } \right)} \right) \hfill \\ + \left( \begin{gathered} l^{2} \left( { - 2 + \delta } \right)\left( {6 + 8\rho + 3\rho^{2} } \right) + 8\beta^{2} \left( { - 5 - 6\rho - 2\rho^{2} + \delta \left( {2 + \rho } \right)^{2} } \right) \hfill \\ + 2l\beta \left( { - 26 - 32\rho - 11\rho^{2} + 2\delta \left( {9 + 10\rho + 3\rho^{2} } \right)} \right) \hfill \\ \end{gathered} \right)\lambda_{b} ) \hfill \\ \end{gathered} }{{4\beta \left( {l + 2\beta } \right)\left( {3l + 2\beta } \right)\left( {1 + \rho } \right)^{2} \left( {2 + \rho } \right)^{2} \left( { - 1 - \delta + \left( { - 1 + \delta } \right)\lambda_{b} } \right)}} > 0$.

2.9 Proof of Conclusion 2

Set $X = 2\beta \left( {1 + \rho } \right)\left( {2\alpha \left( {1 + \rho } \right) + H\beta \left( {2 + \rho } \right)} \right) + l\left( {3H\beta \left( {1 + \rho } \right)\left( {2 + \rho } \right) + \alpha \left( {6 + \rho \left( {8 + 3\rho } \right)} \right)} \right) - \left( {1 + \rho } \right)\left( {2\beta^{2} \left( {3 + 2\rho } \right) + l^{2} \left( {4 + 3\rho } \right) + l\beta \left( {13 + 9\rho } \right)} \right)c_{a} + \left( {1 + \rho } \right)\left( {2\beta^{2} \left( {1 + \rho } \right) + l^{2} \left( {4 + 3\rho } \right) + l\beta \left( {7 + 6\rho } \right)} \right)c_{b}$ $Y = 4\alpha \beta + 3Hl\beta \left( {1 + \rho } \right)\left( {2 + \rho } \right) + 2H\beta^{2} \left( {1 + \rho } \right)\left( {2 + \rho } \right) + l\alpha \left( {6 + \rho \left( {4 + \rho } \right)} \right) + \left( {1 + \rho } \right)(\left( {2\beta^{2} + l^{2} \left( {4 + \rho } \right) + l\beta \left( {7 + \rho } \right)} \right)c_{a} - \left( {2\beta^{2} \left( {3 + \rho } \right) + l^{2} \left( {4 + \rho } \right) + l\beta \left( {13 + 4\rho } \right)} \right)c_{b} )$,

from the simplification B and D in Proposition 5 and 7, we can get

$$\pi_{a}^{*} - \pi_{a}^{N} = \frac{{\left( {l + \beta } \right)( - 4\beta^{2} X^{2} \left( {1 + \delta + \lambda_{a} } \right)\left( { - 1 - \delta + \left( { - 1 + \delta } \right)\lambda_{a} } \right) - \left( {1 + \lambda_{a} } \right)B^{2} )}}{{16\beta^{2} \left( {l + 2\beta } \right)^{2} \left( {3l + 2\beta } \right)^{2} \left( {1 + \rho } \right)^{2} \left( {2 + \rho } \right)^{2} \left( {1 + \delta + \lambda_{a} } \right)\left( { - 1 - \delta + \left( { - 1 + \delta } \right)\lambda_{a} } \right))}} > 0$$

$\pi_{b}^{*} - \pi_{b}^{N} = \frac{{\left( {l + \beta } \right)( - 4\beta^{2} Y^{2} \left( {1 + \delta + \lambda_{b} } \right)\left( { - 1 - \delta + \left( { - 1 + \delta } \right)\lambda_{b} } \right) - \left( {1 + \lambda_{b} } \right)D^{2} )}}{{16\beta^{2} \left( {l + 2\beta } \right)^{2} \left( {3l + 2\beta } \right)^{2} \left( {1 + \rho } \right)\left( {2 + \rho } \right)^{2} \left( {1 + \delta + \lambda_{b} } \right)\left( { - 1 - \delta + \left( { - 1 + \delta } \right)\lambda_{b} } \right))}} > 0$.

2.10 Proof of Conclusion 3

When $\lambda_{a} { = }\lambda_{b}$, we get $p_{a}^{*} - p_{b}^{*} = \frac{{ - \alpha \rho - \left( {l + \beta } \right)\left( {1 + \rho } \right)(c_{a} - c_{b} )}}{{\left( {3l + 2\beta } \right)\left( {1 + \rho } \right)}}$.

So it can be seen that when $\beta > - 1 - \frac{\alpha \rho }{{(1 + \rho )(c_{a} - c_{b} )}}$ we get $p_{a}^{*} - p_{b}^{*} > 0$, namely $p_{a}^{*} > p_{b}^{*}$.