Loss-averse preferences in a two-echelon supply chain with yield risk and demand uncertainty

Abstract

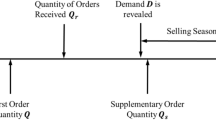
We consider a one-period two-echelon supply chain composed of a loss-averse supplier with yield randomness and a loss-averse retailer with demand uncertainty. At the beginning of the selling season, the retailer orders from the supplier via the wholesale price contract, and then the supplier makes his production decision. We derive the loss-averse retailer’s optimal ordering policy and the loss-averse supplier’s optimal production policy under these conditions. In addition, we discuss the effect of loss aversion on both parties’ decision making and show how loss aversion contributes to decision bias. Furthermore, we find that the loss-averse retailer’s optimal order quantity may increase in wholesale price and decrease in retail price which is differ from the risk-neutral case where the optimal order quantity is always decreasing in wholesale price and increasing in retail price. Finally, numerical examples are presented to illustrate how loss aversion and yield variance contribute to the supply chain performance.

Appendices

Appendix A

Proof of Theorem 3.2

(1) From Eq. (3), we obtain

$$\begin{aligned} \frac{{dE\left[ {U\left( {{\Pi ^S}(Q)} \right) } \right] }}{{dQ}}= \,& {} \left( {w + {\beta _s} + {h_s}} \right) \int _0^{q/Q} {uf\left( u \right) du - } {h_s}\mu - c + \left( {{\lambda _s} - 1} \right) \nonumber \\&\times \left( {\int _0^{{S_1}(Q)} {\left( {\left( {w + {\beta _s}} \right) u - c} \right) f\left( u \right) du - \int _{{S_2}(Q)}^\infty {\left( {{h_s}u + c} \right) f\left( u \right) } du} } \right) \end{aligned}$$

and

$$\begin{aligned} \frac{{{d^2}E\left[ {U\left( {{\Pi ^S}(Q)} \right) } \right] }}{{d{Q^2}}}= \,& {} - \left( {w + {\beta _s} + {h_s}} \right) \frac{{{q^2}}}{{{Q^3}}}f\left( {\frac{q}{Q}} \right) \nonumber \\&- \left( {{\lambda _s} - 1} \right) \left( {\frac{{\beta _s^2{q^2}}}{{\left( {w + {\beta _s}} \right) {Q^3}}}f\left( {{S_1}(Q)} \right) + \frac{{{{\left( {w + {h_s}} \right) }^2}{q^2}}}{{{h_s}{Q^3}}}f\left( {{S_2}(Q)} \right) } \right) < 0 \end{aligned}$$

Hence, there exists a unique optimal order quantity that satisfies the first-order condition Eq. (4).

(2) From the first-order condition Eq. (4), we can find that $Q_\lambda ^*$ is related to the retailer’s ordered quantity q. Therefore, by the implicit function theorem,

$$\begin{aligned} \frac{{dQ_\lambda ^*}}{{dq}} = - \frac{{{d^2}E\left[ {U\left( {{\Pi ^S}(Q_\lambda ^*)} \right) } \right] /dQdq}}{{{d^2}E\left[ {U\left( {{\Pi ^S}(Q_\lambda ^*)} \right) } \right] /d{Q^2}}} = \frac{{Q_\lambda ^*}}{q} > 0 \end{aligned}$$

and

$$\begin{aligned} \frac{{{d^2}Q_\lambda ^*}}{{d{q^2}}} = \left( {\frac{{dQ_\lambda ^*}}{{dq}}q - Q_\lambda ^*} \right) /{q^2} = 0 \end{aligned}$$

We can prove that $Q_\lambda ^*$ is a linear function on the retailer’s ordered quantity q, denoted as $Q_\lambda ^*(q) = Kq$. $\square$

Proof of Theorem 3.3

After substituting $Q_1^*$ into $dE\left[ {U\left( {{\Pi ^S}(Q)} \right) } \right] /dQ$, we get

$$\begin{aligned}&dE\left[ {U\left( {{\Pi ^S}(Q_1^*)} \right) } \right] /dQ\\&\quad = \left( {w + {\beta _s} + {h_s}} \right) \int _0^{q/Q_1^*} {uf\left( u \right) du - } {h_s}\mu - c + \left( {{\lambda _s} - 1} \right) \nonumber \\&\qquad \times \left( {\int _0^{{S_1}(Q_1^*)} {\left( {\left( {w + {\beta _s}} \right) u - c} \right) f\left( u \right) du - \int _{{S_2}(Q_1^*)}^\infty {\left( {{h_s}u + c} \right) f\left( u \right) } du} } \right) \end{aligned}$$

According to Eq. (5), $dE\left[ {U\left( {{\Pi ^S}(Q_1^*)} \right) } \right] /dQ$ reduces to

$$\begin{aligned}&dE\left[ {U\left( {{\Pi ^S}(Q_1^*)} \right) } \right] /dQ\nonumber \\&\quad = \left( {{\lambda _s} - 1} \right) \left( {\int _0^{{S_1}(Q_1^*)} {\left( {\left( {w + {\beta _s}} \right) u - c} \right) f\left( u \right) du - \int _{{S_2}(Q_1^*)}^\infty {\left( {{h_s}u + c} \right) f\left( u \right) } du} } \right) \end{aligned}$$

Therefore, if ${L_1}(Q_1^*) > {L_2}(Q_1^*)$, then $dE\left[ {U\left( {{\Pi ^S}(Q_1^*)} \right) } \right] /dQ > 0$. Since $E\left[ {U\left( {{\Pi ^S}(Q)} \right) } \right]$ is concave, so $Q_\lambda ^* > Q_1^*$, which in turn implies $\left( {w + {\beta _s} + {h_s}} \right) \int _0^{q/Q_\lambda ^*} {uf\left( u \right) du - } {h_s}\mu - c < 0$ based on Eq. (5). Thus, from the first-order condition Eq. (4),

$$\begin{aligned} \int _0^{{S_1}(Q_\lambda ^*)} {\left( {\left( {w + {\beta _s}} \right) u - c} \right) f\left( u \right) du - \int _{{S_2}(Q_\lambda ^*)}^\infty {\left( {{h_s}u + c} \right) f\left( u \right) } du} > 0 \end{aligned}$$

Therefore, by the implicit function theorem,

$$\begin{aligned} \frac{{dQ_\lambda ^*}}{{d{\lambda _s}}}= \,& {} - \frac{{{d^2}E\left[ {U\left( {{\Pi ^S}(Q_\lambda ^*)} \right) } \right] /dQd{\lambda _s}}}{{{d^2}E\left[ {U\left( {{\Pi ^S}(Q_\lambda ^*)} \right) } \right] /d{Q^2}}}\nonumber \\= \,& {} \frac{{\int _0^{{S_1}(Q_\lambda ^*)} {\left( {\left( {w + {\beta _s}} \right) u - c} \right) f\left( u \right) du - \int _{{S_2}(Q_\lambda ^*)}^\infty {\left( {{h_s}u + c} \right) f\left( u \right) } du} }}{{ - {d^2}E\left[ {U\left( {{\Pi ^S}(Q_\lambda ^*)} \right) } \right] /d{Q^2}}} > 0 \end{aligned}$$

Furthermore, as

$$\begin{aligned} \frac{{{d^2}E\left[ {U\left( {{\Pi ^S}(Q)} \right) } \right] }}{{dQdK}}= \,& {} - \left( {w + {\beta _s} + {h_s}} \right) \frac{1}{{{K^3}}}f\left( {\frac{1}{K}} \right) \nonumber \\&- \left( {{\lambda _s} - 1} \right) \left( {\frac{{Q\beta _s^2}}{{{{\left( {w + {\beta _s}} \right) }^2}{K^3}}}f\left( {{S_1}(Q)} \right) + \frac{{{{\left( {w + {h_s}} \right) }^2}{q^2}}}{{Qh_s^2{K^2}}}f\left( {{S_2}(Q)} \right) } \right) < 0 \end{aligned}$$

Therefore,

$$\begin{aligned} \frac{{dK}}{{d{\lambda _s}}}= \,& {} - \frac{{{d^2}E\left[ {U\left( {{\Pi ^S}(Q_\lambda ^*)} \right) } \right] /dQd{\lambda _s}}}{{{d^2}E\left[ {U\left( {{\Pi ^S}(Q_\lambda ^*)} \right) } \right] /dQdK}}\nonumber \\= \,& {} \frac{{\int _0^{{S_1}(Q_\lambda ^*)} {\left( {\left( {w + {\beta _s}} \right) u - c} \right) f\left( u \right) du - \int _{{S_2}(Q_\lambda ^*)}^\infty {\left( {{h_s}u + c} \right) f\left( u \right) } du} }}{{ - {d^2}E\left[ {U\left( {{\Pi ^S}(Q_\lambda ^*)} \right) } \right] /dQdK}} > 0 \end{aligned}$$

Similarly, we can prove that if ${L_1}(Q_1^*) \le {L_2}(Q_1^*)$, then $Q_\lambda ^* \le Q_1^*$, $dQ_\lambda ^*/d{\lambda _s} \le 0$ and $dK/d{\lambda _s} \le 0$. $\square$

Proof of Theorem 3.4

(1) According to the implicit function theorem,

$$\begin{aligned} \frac{{dQ_\lambda ^*}}{{d{\beta _s}}} = - \frac{{{d^2}E\left[ {U\left( {{\Pi ^S}(Q_\lambda ^*)} \right) } \right] /dQd{\beta _s}}}{{{d^2}E\left[ {U\left( {{\Pi ^S}(Q_\lambda ^*)} \right) } \right] /d{Q^2}}} \end{aligned}$$

where,

$$\begin{aligned}&{d^2}E\left[ {U\left( {{\Pi ^S}(Q_\lambda ^*)} \right) } \right] /dQd{\beta _s} \\&\quad = \int _0^{q/Q_\lambda ^*} {uf\left( u \right) du + \left( {{\lambda _s} - 1} \right) \left( {\int _0^{{S_1}(Q_\lambda ^*)} {uf\left( u \right) } du + \frac{{{\beta _s}q\left( {wq - cQ_\lambda ^*} \right) }}{{{{\left( {w + {\beta _s}} \right) }^2}{{\left( {Q_\lambda ^*} \right) }^2}}}f\left( {{S_1}(Q_\lambda ^*)} \right) } \right) } \\&\quad > 0 \end{aligned}$$

So, $dQ_\lambda ^*/d{\beta _s} > 0$.

(2) According to the implicit function theorem,

$$\begin{aligned} \frac{{dQ_\lambda ^*}}{{d{h_s}}} = - \frac{{{d^2}E\left[ {U\left( {{\Pi ^S}(Q_\lambda ^*)} \right) } \right] /dQd{h_s}}}{{{d^2}E\left[ {U\left( {{\Pi ^S}(Q_\lambda ^*)} \right) } \right] /d{Q^2}}} \end{aligned}$$

where,

$$\begin{aligned}&{d^2}E\left[ {U\left( {{\Pi ^S}(Q_\lambda ^*)} \right) } \right] /dQd{h_s} \nonumber \\&\quad = \int _0^{q/Q_\lambda ^*} {uf\left( u \right) du - \mu - \left( {{\lambda _s} - 1} \right) \left( {\int _{{S_2}(Q_\lambda ^*)}^\infty {uf\left( u \right) } du + \frac{{\left( {w + {h_s}} \right) q\left( {wq - cQ_\lambda ^*} \right) }}{{h_s^2{{\left( {Q_\lambda ^*} \right) }^2}}}f\left( {{S_2}(Q_\lambda ^*)} \right) } \right) } \nonumber \\&< 0 \end{aligned}$$

So, $dQ_\lambda ^*/d{h_s} < 0$. $\square$

Proof of Theorem 3.6

From Eq. (8), we get

$$\begin{aligned}&\frac{{dE\left[ {U\left( {{\Pi ^R}(q)} \right) } \right] }}{{dq}}\\&\quad =\int _0^{1/K} {\left[ {\left( {p - w + {\beta _r}} \right) uK - \left( {p + {h_r} + {\beta _r}} \right) uK\int _0^{uKq_\lambda ^*} {g\left( x \right) dx} } \right] f\left( u \right) du} \nonumber \\&\qquad + \int _{1/K}^\infty {\left[ {\left( {p - w + {\beta _r}} \right) - \left( {p + {h_r} + {\beta _r}} \right) \int _0^{q_\lambda ^*} {g\left( x \right) dx} } \right] f\left( u \right) du} \nonumber \\&\qquad + \left( {{\lambda _r} - 1} \right) \int _0^{1/K} {\left[ {uK\left( {\left( {p - w + {\beta _r}} \right) \int _{{R_4}(q_\lambda ^*)}^\infty {g\left( x \right) dx} - \left( {w + {h_r}} \right) \int _0^{{R_3}(q_\lambda ^*)} {g\left( x \right) } dx} \right) } \right] f\left( u \right) du} \nonumber \\&\qquad + \left( {{\lambda _r} - 1} \right) \int _{1/K}^\infty {\left[ {\left( {p - w + {\beta _r}} \right) \int _{{R_2}(q_\lambda ^*)}^\infty {g\left( x \right) dx} - \left( {w + {h_r}} \right) \int _0^{{R_1}(q_\lambda ^*)} {g\left( x \right) } dx} \right] f\left( u \right) du} \end{aligned}$$

and

$$\begin{aligned}&\frac{{{d^2}E\left[ {U\left( {{\Pi ^R}(q)} \right) } \right] }}{{d{q^2}}}\\&\quad = - \int _{1/K}^\infty {\left[ {\left( {p + {h_r} + {\beta _r}} \right) g\left( q \right) } \right] f\left( u \right) du} \nonumber \\&\qquad - \int _0^{1/K} {\left[ {\left( {p + {h_r} + {\beta _r}} \right) {{\left( {uK} \right) }^2}g\left( {uKq} \right) } \right] f\left( u \right) du} \nonumber \\&\qquad - \left( {{\lambda _r} - 1} \right) \int _{1/K}^\infty {\left[ {\left( {p - w + {\beta _r}} \right) g\left( {{R_2}(q)} \right) R_2^{'}(q) + \left( {w + {h_r}} \right) g\left( {{R_1}(q)} \right) R_1^{'}(q)} \right] f\left( u \right) du} \nonumber \\&\qquad - \left( {{\lambda _r} - 1} \right) \int _0^{1/K} {uK\left[ {\left( {p - w + {\beta _r}} \right) g\left( {{R_4}(q)} \right) R_4^{'}(q) + \left( {w + {h_r}} \right) g\left( {{R_3}(q)} \right) R_3^{'}(q)} \right] f\left( u \right) du} \nonumber \\&\quad < 0 \end{aligned}$$

Hence, there exists a unique optimal order quantity $q_\lambda ^*$ that satisfies the first-order condition Eq. (9). $\square$

Proof of Theorem 3.8

Proof is similar to the proof of Theorem 3.3.

After substituting $q_1^*$ into $dE\left[ {U\left( {{\Pi ^R}(q)} \right) } \right] /dq$, we get

$$\begin{aligned}&dE\left[ {U\left( {{\Pi ^R}(q_1^*)} \right) } \right] /dq\\&\quad = \int _0^{1/K} {\left[ {\left( {p - w + {\beta _r}} \right) uK - \left( {p + {h_r} + {\beta _r}} \right) uK\int _0^{uKq_1^*} {g\left( x \right) dx} } \right] f\left( u \right) du} \\&\qquad + \int _{1/K}^\infty {\left[ {\left( {p - w + {\beta _r}} \right) - \left( {p + {h_r} + {\beta _r}} \right) \int _0^{q_1^*} {g\left( x \right) dx} } \right] f\left( u \right) du} \\&\qquad + \left( {{\lambda _r} - 1} \right) \int _0^{1/K} {\left[ {uK\left( {\left( {p - w + {\beta _r}} \right) \int _{{R_4}(q_1^*)}^\infty {g\left( x \right) dx} - \left( {w + {h_r}} \right) \int _0^{{R_3}(q_1^*)} {g\left( x \right) } dx} \right) } \right] f\left( u \right) du} \\&\qquad + \left( {{\lambda _r} - 1} \right) \int _{1/K}^\infty {\left[ {\left( {p - w + {\beta _r}} \right) \int _{{R_2}(q_1^*)}^\infty {g\left( x \right) dx} - \left( {w + {h_r}} \right) \int _0^{{R_1}(q_1^*)} {g\left( x \right) } dx} \right] f\left( u \right) du} \end{aligned}$$

According to Eq. (10), $dE\left[ {U\left( {{\Pi ^R}(q_1^*)} \right) } \right] /dq$ reduces to

$$\begin{aligned}&dE\left[ {U\left( {{\Pi ^R}(q_1^*)} \right) } \right] /dq\\&\quad = \left( {{\lambda _r} - 1} \right) \int _0^{1/K} {\left[ {uK\left( {\left( {p - w + {\beta _r}} \right) \int _{{R_4}(q_1^*)}^\infty {g\left( x \right) dx} - \left( {w + {h_r}} \right) \int _0^{{R_3}(q_1^*)} {g\left( x \right) } dx} \right) } \right] f\left( u \right) du} \\&\qquad + \left( {{\lambda _r} - 1} \right) \int _{1/K}^\infty {\left[ {\left( {p - w + {\beta _r}} \right) \int _{{R_2}(q_1^*)}^\infty {g\left( x \right) dx} - \left( {w + {h_r}} \right) \int _0^{{R_1}(q_1^*)} {g\left( x \right) } dx} \right] f\left( u \right) du} \end{aligned}$$

Therefore, if ${l_1}(q_1^*) > {l_2}(q_1^*)$, then $dE\left[ {U\left( {{\Pi ^R}(q_1^*)} \right) } \right] /dq > 0$. Since $E\left[ {U\left( {{\Pi ^R}(q)} \right) } \right]$ is concave, so $q_\lambda ^* > q_1^*$, which in turn implies

$$\begin{aligned}&\int _0^{1/K} {\left[ {\left( {p - w + {\beta _r}} \right) uK - \left( {p + {h_r} + {\beta _r}} \right) uK\int _0^{uKq_\lambda ^*} {g\left( x \right) dx} } \right] f\left( u \right) du} \nonumber \\&\quad + \int _{1/K}^\infty {\left[ {\left( {p - w + {\beta _r}} \right) - \left( {p + {h_r} + {\beta _r}} \right) \int _0^{q_\lambda ^*} {g\left( x \right) dx} } \right] f\left( u \right) du} \nonumber \\&\quad < 0 \end{aligned}$$

Thus, from the first-order condition Eq. (9), ${l_1}(q_\lambda ^*) > {l_2}(q_\lambda ^*)$. Therefore, by the implicit function theorem,

$$\begin{aligned} \frac{{dq_\lambda ^*}}{{d{\lambda _r}}}= \,& {} - \frac{{{d^2}E\left[ {U\left( {{\Pi ^R}(q_\lambda ^*)} \right) } \right] /dqd{\lambda _r}}}{{{d^2}E\left[ {U\left( {{\Pi ^R}(q_\lambda ^*)} \right) } \right] /d{q^2}}} \\= \,& {} \frac{{{l_1}(q_\lambda ^*) - {l_2}(q_\lambda ^*)}}{{ - {d^2}E\left[ {U\left( {{\Pi ^R}(q_\lambda ^*)} \right) } \right] /d{q^2}}} > 0 \end{aligned}$$

Similarly, we can prove that if ${l_1}(q_1^*) \le {l_2}(q_1^*)$, then $dq_\lambda ^*/d{\lambda _r} \le 0$. $\square$

Proof of Theorem 3.9

(1) According to the implicit function theorem,

$$\begin{aligned} \frac{{dq_\lambda ^*}}{{d{\beta _r}}} = - \frac{{{d^2}E\left[ {U\left( {{\Pi ^R}(q_\lambda ^*)} \right) } \right] /dqd{\beta _r}}}{{{d^2}E\left[ {U\left( {{\Pi ^R}(q_\lambda ^*)} \right) } \right] /d{q^2}}} \end{aligned}$$

where,

$$\begin{aligned}&{d^2}E\left[ {U\left( {{\Pi ^R}(q_\lambda ^*)} \right) } \right] /dqd{\beta _r}\\&\quad = \int _0^{1/K} {uK\overline{G} \left( {uKq_\lambda ^*} \right) } f\left( u \right) du + \int _{1/K}^\infty {\overline{G} \left( {q_\lambda ^*} \right) f\left( u \right) du} \\&\qquad + \left( {{\lambda _r} - 1} \right) \int _0^{1/K} {uK\left[ {\overline{G} \left( {{R_4}\left( {q_\lambda ^*} \right) } \right) + \frac{{\left( {p - w} \right) \left( {p - w + {\beta _r}} \right) }}{{\beta _r^2}}uKqg\left( {{R_4}\left( {q_\lambda ^*} \right) } \right) } \right] } f\left( u \right) du\\&\qquad + \left( {{\lambda _r} - 1} \right) \int _{1/K}^\infty {\left[ {\overline{G} \left( {{R_2}\left( {q_\lambda ^*} \right) } \right) + \frac{{\left( {p - w} \right) \left( {p - w + {\beta _r}} \right) }}{{\beta _r^2}}qg\left( {{R_2}\left( {q_\lambda ^*} \right) } \right) } \right] f\left( u \right) du} \\&\quad > 0 \end{aligned}$$

Thus, $dq_\lambda ^*/d{\beta _r} > 0$.

(2) According to the implicit function theorem,

$$\begin{aligned} \frac{{dq_\lambda ^*}}{{d{h_r}}} = - \frac{{{d^2}E\left[ {U\left( {{\Pi ^R}(q_\lambda ^*)} \right) } \right] /dqd{h_r}}}{{{d^2}E\left[ {U\left( {{\Pi ^R}(q_\lambda ^*)} \right) } \right] /d{q^2}}} \end{aligned}$$

where,

$$\begin{aligned}&{d^2}E\left[ {U\left( {{\Pi ^R}(q_\lambda ^*)} \right) } \right] /dqd{h_r}\\&\quad = - \int _0^{1/K} {uKG\left( {uKq_\lambda ^*} \right) } f\left( u \right) du - \int _{1/K}^\infty {G\left( {q_\lambda ^*} \right) f\left( u \right) du} \\&\qquad - \left( {{\lambda _r} - 1} \right) \int _0^{1/K} {uK\left[ {G\left( {{R_3}\left( {q_\lambda ^*} \right) } \right) + \frac{{\left( {p - w} \right) \left( {w + {h_r}} \right) }}{{{{\left( {p + {h_r}} \right) }^2}}}uKqg\left( {{R_3}\left( {q_\lambda ^*} \right) } \right) } \right] } f\left( u \right) du\\&\qquad - \left( {{\lambda _r} - 1} \right) \int _{1/K}^\infty {\left[ {G\left( {{R_1}\left( {q_\lambda ^*} \right) } \right) + \frac{{\left( {p - w} \right) \left( {w + {h_r}} \right) }}{{{{\left( {p + {h_r}} \right) }^2}}}qg\left( {{R_1}\left( {q_\lambda ^*} \right) } \right) } \right] f\left( u \right) du} \\&\quad < 0 \end{aligned}$$

Thus, $dq_\lambda ^*/d{h_r} < 0$.

(3) According to the implicit function theorem,

$$\begin{aligned} \frac{{dq_\lambda ^*}}{{dw}} = - \frac{{{d^2}E\left[ {U\left( {{\Pi ^R}(q_\lambda ^*)} \right) } \right] /dqdw}}{{{d^2}E\left[ {U\left( {{\Pi ^R}(q_\lambda ^*)} \right) } \right] /d{q^2}}} \end{aligned}$$

where,

$$\begin{aligned}&- {d^2}E\left[ {U\left( {{\Pi ^R}(q_\lambda ^*)} \right) } \right] /dqdw\\&\quad = \int _0^{1/K} {uKf\left( u \right) du + \overline{F} \left( {1/K} \right) } \\&\qquad + \left( {{\lambda _r} - 1} \right) \int _0^{1/K} {uK\left[ {\overline{G} \left( {{R_4}\left( {q_\lambda ^*} \right) } \right) - {R_4}\left( {q_\lambda ^*} \right) g\left( {{R_4}\left( {q_\lambda ^*} \right) } \right) } \right] } f\left( u \right) du\\&\qquad + \left( {{\lambda _r} - 1} \right) \int _0^{1/K} {uK\left[ {G\left( {{R_3}\left( {q_\lambda ^*} \right) } \right) + {R_3}\left( {q_\lambda ^*} \right) g\left( {{R_3}\left( {q_\lambda ^*} \right) } \right) } \right] } f\left( u \right) du\\&\qquad + \left( {{\lambda _r} - 1} \right) \int _{1/K}^\infty {\left[ {\overline{G} \left( {{R_2}\left( {q_\lambda ^*} \right) } \right) - {R_2}\left( {q_\lambda ^*} \right) g\left( {{R_2}\left( {q_\lambda ^*} \right) } \right) } \right] f\left( u \right) du} \\&\qquad + \left( {{\lambda _r} - 1} \right) \int _{1/K}^\infty {\left[ {G\left( {{R_1}\left( {q_\lambda ^*} \right) } \right) + {R_1}\left( {q_\lambda ^*} \right) g\left( {{R_1}\left( {q_\lambda ^*} \right) } \right) } \right] f\left( u \right) du} \end{aligned}$$

Let ${Z_1}\left( {q_\lambda ^*} \right) = - {d^2}E\left[ {U\left( {{\Pi ^R}(q_\lambda ^*)} \right) } \right] /dqdw$, thus, if ${Z_1}\left( {q_\lambda ^*} \right) < 0$, then $dq_\lambda ^*/dw > 0$; otherwise $dq_\lambda ^*/dw \le 0$.

(4) According to the implicit function theorem,

$$\begin{aligned} \frac{{dq_\lambda ^*}}{{dp}} = - \frac{{{d^2}E\left[ {U\left( {{\Pi ^R}(q_\lambda ^*)} \right) } \right] /dqdp}}{{{d^2}E\left[ {U\left( {{\Pi ^R}(q_\lambda ^*)} \right) } \right] /d{q^2}}} \end{aligned}$$

where,

$$\begin{aligned}&{d^2}E\left[ {U\left( {{\Pi ^R}(q_\lambda ^*)} \right) } \right] /dqdp\\&\quad = \int _0^{1/K} {uK\overline{G} \left( {uKq_\lambda ^*} \right) f\left( u \right) du + \overline{G} \left( {q_\lambda ^*} \right) \overline{F} \left( {1/K} \right) } \\&\qquad + \left( {{\lambda _r} - 1} \right) \int _0^{1/K} {uK\left[ {\overline{G} \left( {{R_4}\left( {q_\lambda ^*} \right) } \right) - {R_4}\left( {q_\lambda ^*} \right) g\left( {{R_4}\left( {q_\lambda ^*} \right) } \right) } \right] } f\left( u \right) du\\&\qquad + \left( {{\lambda _r} - 1} \right) \int _0^{1/K} {uK\left[ {\frac{{w + {h_r}}}{{p + {h_r}}}{R_3}\left( {q_\lambda ^*} \right) g\left( {{R_3}\left( {q_\lambda ^*} \right) } \right) } \right] } f\left( u \right) du\\&\qquad + \left( {{\lambda _r} - 1} \right) \left[ {\overline{G} \left( {{R_2}\left( {q_\lambda ^*} \right) } \right) - {R_2}\left( {q_\lambda ^*} \right) g\left( {{R_2}\left( {q_\lambda ^*} \right) } \right) } \right] \overline{F} \left( {1/K} \right) \\&\qquad + \left( {{\lambda _r} - 1} \right) \left[ {\frac{{w + {h_r}}}{{p + {h_r}}}{R_1}\left( {q_\lambda ^*} \right) g\left( {{R_1}\left( {q_\lambda ^*} \right) } \right) } \right] \overline{F} \left( {1/K} \right) \end{aligned}$$

Let ${Z_2}\left( {q_\lambda ^*} \right) = {d^2}E\left[ {U\left( {{\Pi ^R}(q_\lambda ^*)} \right) } \right] /dqdp$, thus, if ${Z_2}\left( {q_\lambda ^*} \right) < 0$, then $dq_\lambda ^*/dp < 0$, otherwise $dq_\lambda ^*/dp \ge 0$. $\square$

Appendix B

See Table 3.

Table 3 Summary of the problems and results