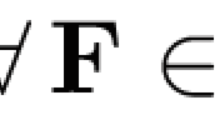Abstract
This article considers the influence of incompressibility on the compliance and stiffness constants that appear in the weakly nonlinear theory of elasticity. The formulation first considers the incompressibility constraint applied to compliances, which gives explicit finite limits for the second-, third-, and fourth-order compliance constants. The stiffness/compliance relationships for each order are derived and used to determine the incompressible behavior of the second-, third-, and fourth-order stiffness constants. Unlike the compressible case, the fourth-order compliances are not found to be dependent on the fourth-order stiffnesses.
Similar content being viewed by others
References
Saccomandi, G., Vergori, L.: Some remarks on the weakly nonlinear theory of isotropic elasticity. J. Elast. (2021). https://doi.org/10.1007/s10659-021-09865-1
Kostek, S., Sinha, B.K., Norris, A.N.: Third-order elastic constants for an inviscid fluid. J. Acoust. Soc. Am. 94, 3014–3017 (1993). https://doi.org/10.1121/1.407336
Hamilton, M.F., Iliinskii Zabolotskaya, Y.A.: Separation of compressibility and shear deformation in the elastic energy density (l). J. Acoust. Soc. Am. 116, 41–44 (2004). https://doi.org/10.1121/1.1736652
Ogden, R.W.: On isotropic tensors and elastic moduli. Proc. Camb. Philol. Soc. 75, 427–436 (1974). https://doi.org/10.1017/S0305004100048635
Gennisson, J.L., Rénier, M., Catheline, S., Barrière, C., Bercoff, J., Tanter, M., Fink, M.: Acoustoelasticity in soft solids: assessment of the nonlinear shear modulus with the acoustic radiation force. J. Acoust. Soc. Am. 122, 3211–3219 (2007). https://doi.org/10.1121/1.2793605
Gennisson, J.L., Deffieux, T., Fink, M., Tanter, M.: Ultrasound elastography: principles and techniques. Diagn. Interv. Imaging 94, 487–495 (2013). https://doi.org/10.1016/j.diii.2013.01.022
Destrade, M., Ogden, R.W.: On the third- and fourth-order constants of incompressible isotropic elasticity. J. Acoust. Soc. Am. 128, 3334–3343 (2010). https://doi.org/10.1121/1.3505102
Destrade, M., Martin, P., Ting, T.: The incompressible limit in linear anisotropic elasticity, with applications to surface waves and elastostatics. J. Mech. Phys. Solids 50, 1453–1468 (2002). https://doi.org/10.1016/S0022-5096(01)00121-1
Ignaczak, J., Rao, C.R.A.: A tensorial classification of elastic waves. J. Acoust. Soc. Am. 93, 17–21 (1993). https://doi.org/10.1121/1.405642
Ostoja-Starzewski, M.: Ignaczak equation of elastodynamics. Math. Mech. Solids 24, 3674–3713 (2018). https://doi.org/10.1177/1081286518757284
Kube, C.M., Norris, A.N.: Stress formulation of elastic wave motion. JASA Express Lett. 1, 064,001 (2021). https://doi.org/10.1121/10.0004317
Norris, A.N.: Finite-amplitude waves in solids. In: Hamilton, M.F., Blackstock, D. (eds.) Nonlinear Acoustics, pp. 263–278. Academic Press, San Diego (1998)
Kube, C.M., Roy, A., Jensen, D.S., Branch, D.W.: A unifying model of weakly nonlinear elastic waves; large on large theory. J. Acoust. Soc. Am. 151, 1294–1310 (2022). https://doi.org/10.1121/10.0009376
Brugger, K.: Pure modes for elastic waves in crystals. J. Appl. Phys. 36, 759–768 (1965). https://doi.org/10.1063/1.1714215
Landau, L.D., Lifshitz, E.M.: Theory of elasticity. In: Course of Theoretical Physics, 3rd edn. vol. 7, pp. 1–186. Butterworth-Heinemann, Oxford (1986)
Kube, C.M.: Scattering of harmonic waves from a nonlinear elastic inclusion. J. Acoust. Soc. Am. 141, 4756 (2017). https://doi.org/10.1121/1.4986747
Acknowledgements
The author thanks Prof. Andy Norris of Rutgers University for helpful suggestions and discussion at the early stages of this work.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher’s Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendices
Appendix A: Isotropic Tensors of Elastic Stiffness and Compliance
For isotropic materials, the elastic modulus tensors are linear combinations of products of Kronecker delta functions,
where \({I_{\mathit{ijkl}}} = \left ( {{\delta _{ik}}{\delta _{jl}} + {\delta _{il}}{ \delta _{jk}}} \right )/2\) is the fourth-rank identity tensor. The form of \(c_{\mathit{ijkl}}\) is well-known whereas the forms for \(c_{\mathit{ijklmn}}\) and \(c_{\mathit{ijklmnpq}}\) are less common. Using Eqs. (36), it is easy to show that [16] \(c_{11}=c_{12}+2c_{44}\), \(c_{111}=c_{123}+6c_{144}+8c_{456}\), \(c_{112}=c_{123}+2c_{144}\), \(c_{155}=c_{144}+2c_{456}\), \(c_{1111}=c_{1123}+10c_{1255}+12c_{1456}+12c_{4455}\), \(c_{1112}=c_{1123}+4c_{1255}\), \(c_{1155}=c_{1255}+2c_{1456}+2c_{4455}\). Note that the analogous isotropic tensors for compliance constants take the same form as Eqs. (36), thus, only a notation change from \(c\rightarrow s\) is needed.
Conversions to Landau-Lifshitz notation follow [12, 15, 16] \(c_{11}=\lambda +2\mu \), \(c_{12}=\lambda \), \(c_{44}=\mu \), \(c_{111}=2\mathcal{A}+6\mathcal{B}+2\mathcal{C}\), \(c_{112}=2\mathcal{B}+2\mathcal{C}\), \(c_{123}=2\mathcal{C}\), \(c_{144}=\mathcal{B}\), \(c_{155}=\mathcal{A}/2+\mathcal{B}\), \(c_{456}=\mathcal{A}/4\), \(c_{1111}=24(\mathcal{E}+\mathcal{F}+\mathcal{G}+\mathcal{H})\), \(c_{1112}=6\mathcal{E}+12\mathcal{F}+24\mathcal{H}\), \(c_{1122}=8\mathcal{F}+8\mathcal{G}+24\mathcal{H}\), \(c_{1123}=4\mathcal{F}+24\mathcal{H}\), \(c_{1255}=3\mathcal{E}/2+2\mathcal{F}\), \(c_{1456}=3\mathcal{E}/4\), \(c_{1144}=2\mathcal{F}+4\mathcal{G}\), \(c_{1155}=3\mathcal{E}+2\mathcal{F}+4\mathcal{G}\), \(c_{1244}=3\mathcal{E}/2+2\mathcal{F}\), \(c_{1266}=3\mathcal{E}+2\mathcal{F}\), \(c_{4455}=2\mathcal{G}\), \(c_{4444}=6\mathcal{G}\). Thus, in terms of the [15] convention,

Appendix B: Stiffness/Compliance Relationships
The derivative of \(\boldsymbol{S}\) defined in Eq. (1) with respect to itself is
where \(I_{\mathit{ijkl}}=\left (\delta _{ik}\delta _{jl}+\delta _{il}\delta _{jk} \right )/2\) is the fourth-rank identity. The term \(\partial E_{mn}/\partial S_{kl}\) is found by taking the derivative of \(\boldsymbol{E}\) in Eq. (8) with respect to \(\boldsymbol{S}\),
which was obtained by using \(\partial S_{ij}/\partial S_{kl}=I_{\mathit{ijkl}}\). Substituting Eqs. (8) and (39) into Eq. (38) and keeping terms up to second-order in \(\boldsymbol{S}\) gives
The relationships between the elastic stiffness and compliance tensors are found by equating constant, linear, and quadratic terms of \(\boldsymbol{S}\),
The stiffness/compliance relations for isotropic materials follow from Eqs. (41),
where \(a_{1}=3s_{12}+2s_{44}\).
Rights and permissions
About this article
Cite this article
Kube, C.M. On the Incompressible Behavior in Weakly Nonlinear Elasticity. J Elast 148, 129–140 (2022). https://doi.org/10.1007/s10659-022-09886-4
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10659-022-09886-4