Abstract
We examine how international transfers affect the welfare levels of a donor with a higher marginal propensity to save and a recipient with a lower marginal propensity to save when both countries adopt a pay-as-you-go (PAYG) pension system using a one-sector overlapping generations model. We demonstrate that in a dynamically efficient economy, except at the golden rule, when a per capita PAYG pension contribution of either a donor or a recipient increases marginally, the effect of the transfer on the donor’s welfare can be reduced, whereas whether the effect of the transfer on the recipient’s welfare is reduced is ambiguous. These results imply that the existence of a PAYG pension might hinder the effectiveness of the transfer on the donor’s welfare, and the adoption of a PAYG pension system is likely to cause a weak transfer paradox in which both a donor and a recipient immiserize. Our results also suggest that the introduction of a PAYG pension system, which is used as a domestic policy instrument for intergenerational income redistribution, reduces the donor’s incentive to make an international transfer to a recipient, which is a form of international income redistribution.
Similar content being viewed by others
Notes
In China, there are two types of pension system. The PAYG pension system is adopted in cities, and a fully funded scheme is adopted in rural areas.
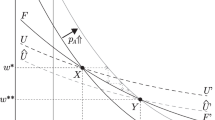
Following Galor and Polemarchakis (1987), Haaparanta (1989) demonstrated the occurrence of a transfer paradox even if only a temporary transfer occurs, involving the government issuing bonds. Yanagihara (1998) incorporated public goods that affected productivity in the private production sector and examined transfers in the form of lump-sum, debt-relief, and public goods. Yanagihara (2006) provided a visualization of the transfer paradox set out in Galor and Polemarchakis (1987). Cremers and Sen (2005) investigated the possibility of transfer paradoxes in the transitional path to the steady state as well as in the steady state.
In an OLG model, a fully funded pension cannot be distinguished from a system involving private savings only. Thus, introducing a fully funded pension into the model does not affect our results.
References
Bruce N, Turnovsky SJ (2013) Social security, growth, and welfare in overlapping generations economies with or without annuities. J Public Econ 101:12–24
Cipriani G (2014) Population aging and PAYG pensions in the OLG model. J Popul Econ 27(1):251–256
Cremers ET, Sen P (2005) The transfer paradox in a one-sector overlapping generations model. J Econ Dyn Control 32(6):1995–2012
Fanti L, Gori L (2012) Fertility and PAYG pensions in the overlapping generations model. J Popul Econ 25(3):955–961
Galor O, Polemarchakis H (1987) International equilibrium and the transfer paradox. Rev Econ Stud 54(1):147–56
Haaparanta P (1989) The intertemporal effects of international transfers. J Int Econ 26(3–4):371–382
Kaganovich M, Zilcha I (2012) Pay-as-you-go or funded social security? A general equilibrium comparison. J Econ Dyn Control 36(4):455–467
Keynes JM (1929a) The German transfer problem. Econ J 39(153):1–7
Keynes JM (1929b) The reparation problem: a discussion II. A rejoinder. Econ J 39(154):179–82
Ohlin B (1929) The reparation problem: a discussion I. Transfer difficulties, real and imagined. Econ J 39(154):172–78
Orszag PR, Stiglitz JE (2001) Rethinking pension reform: ten myths about social security systems. In: Holzmann R, Stiglitz JE (eds) New ideas about old age security: toward sustainable pension systems in the 21st century. The World Bank
Roberts MA (2003) Can Pay-as-You-Go pensions raise the capital stock? Manch Sch 71(s1):1–20
The World Bank (1994) Averting the old age crisis: policies to protect the old and promote growth. Oxford University Press, Oxford
Yanagihara M (1998) Public goods and the transfer paradox in an overlapping generations model. J Int Trade Econ Dev 7(2):175–206
Yanagihara M (2006) The strong transfer paradox in an overlapping generations framework. Econ Bull 6(3):1–8
Acknowledgments
We acknowledge financial support from Waseda Univsesity Tokutei Kadai (Kiso Grant) No. 2014K-6007 (Akihiko Kaneko), MEXT/JSPS Grant-in-Aid for Scientific Research (C) No. 25380286 (Kojun Hamada), and No. 26380360 (Mitsuyoshi Yanagihara). We also would like to thank participants at 70th Annual Congress of the International Institute of Public Finance (IIPF) at Università della Svizzera italiana (USI) in Lugano, Switzerland, 2014 China Meeting of the Econometric Society in Xiamen University, China, and Waseda University lunchtime seminar, and 2015 annual meeting of Japan Association of Political Economy. Above all, the authors are deeply indebted to an anonymous referee for his helpful suggestions and insightful comments.
Author information
Authors and Affiliations
Corresponding author
Appendices
Appendix A: The proof of Lemma 1
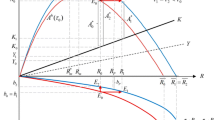
From Eq. 7 and r≡r t = r t+1, the world capital market equilibrium in the steady state is rewritten as follows:
-
(i)
By the definition of a transfer from a donor to a recipient, \(\frac {dI^{D}}{dT}=-1\) and \(\frac {dI^{R}}{dT}=1\). By differentiating Eq. A.1 with respect to r and T, we obtain the following equation:
$$ {\Gamma} dr =-{s^{D}_{I}}dT+{s^{R}_{I}}dT \Leftrightarrow \frac{dr}{dT} =-\frac{{s^{D}_{I}}-{s^{R}_{I}}}{\Gamma}. $$(A.2)Γ<0 holds in the steady state under the dynamically stable condition, Eq. 11. Thus, if \({s^{D}_{I}}>{s^{R}_{I}}\), \(\frac {dr}{dT}>0\).
-
(ii)
By the definition of lifetime income I i, \(\frac {dI^{i}}{dP^{i}}= -\frac {r-n}{1+r}<0\). By differentiating Eq. A.1 with respect to r and P i, we obtain the following equation:
$$ {\Gamma} dr ={s^{i}_{I}}(-\frac{r-n}{1+r})dP^{i} \Leftrightarrow \frac{dr}{dP^{i}} =-\frac{(r-n){s^{i}_{I}}}{(1+r){\Gamma}}>0. $$(A.3)
Appendix B: The proof of Lemma 2
Differentiating \(\frac {dr}{dT}\), which is given by Eq. A.2, with respect to P i, we obtain the following equation:
When we evaluate Eqs. A.4 and A.5 at P D = P R=0, and noting that \(\frac {r-n}{1+r} =-\frac {\Gamma }{{s_{I}^{i}}}\frac {dr}{dP^{i}}\) and \(w^{\prime }=-k\), the following equations are obtained:
If \(s^{D}_{II}= s^{R}_{II}\) and \(s^{D}_{Ir}= s^{R}_{Ir}\), \(\frac {d^{2}r}{dTdP^{D}}\left |{\vphantom {\frac {d^{2}r}{dTdP^{R}}}}{~}_{P^{D}=P^{R}=0}\right .\) is negative from Eq. A.6, while only when \(\frac {d{\Gamma }} {dP^{R}} < \frac {(r-n)s_{II}^{R}{\Gamma }} {(1+r)({s_{I}^{D}}-{s_{I}^{R}})}(<0)\), \(\frac {d^{2}r}{dTdP^{R}}\left |{\vphantom {\frac {d^{2}r}{dTdP^{R}}}}{~}_{P^{D}=P^{R}=0}\right .\) is negative from Eq. A.7.
Appendix C: The derivation of \(\frac {d{\Gamma }}{dP^{D}}\)
From Eq. 11, the dynamic stability condition in the steady state can be rewritten as follows:
Differentiating Eq. A.8 with respect to P D, we obtain the following equation:
When marginally evaluating at P D=0 and P R=0, Eq. A.9 is arranged as follows:
By arranging Eq. 10 with \(\frac {dr}{dP^{D}} =-\frac {(r-n){s^{D}_{I}}}{(1+r){\Gamma }}>0\), we obtain the following equation:
The signs follow from \(k^{\prime }=\frac {1}{f^{\prime \prime }}<0\), Γ<0, and \(\frac {dr}{dP^{D}}>0\), from Lemma 1(ii). The sufficient condition under which \(\frac {d{\Gamma }}{d P^{D}}\left |{\vphantom {\frac {d^{2}r}{dTdP^{R}}}}{~}_{P^{D}=P^{R}=0}\right .\) is negative is that the large bracket of the left-hand side of Eq. A.11 is negative. A similar procedure can be used to derive \(\frac {d{\Gamma }}{dP^{R}}\).
Appendix D: The proof of Proposition 2
The proof procedure is similar to that of Proposition 1. By rearranging Eq. 20, we obtain the following equation:
By differentiating Eq. A.12 with respect to P R and P D, respectively, we obtain the second-order cross-partial derivatives. Evaluating them at P D = P R=0, we obtain the following equations:
Note that \(\frac {dr}{dT}>0\), \(\frac {dr}{dP^{R}}>0\), and \(\frac {d^{2}r}{dTdP^{i}}\left |{\vphantom {\frac {d^{2}r}{dTdP^{R}}}}{~}_{P^{D}=P^{R}=0}\right .<0\) from Lemmas 1 and 2, respectively. By the capital market equilibrium and the dynamically efficient condition, s R<(1 + n)k≤(1 + r)k. Thus, (1 + r)k−s R>0 always holds irrespective of the size of s R. Therefore, the second terms of Eqs. A.13 and A.14 are necessarily positive, while the sign of the first terms is not determined. For example, when \({s^{R}_{I}}\ge \frac {{s^{R}_{r}}-(1+r)k^{\prime }}{k}\), which is a similar condition to that used in Proposition 1, the first terms of Eqs. A.13 and A.14 are negative and therefore the signs of both Eqs. A.13 and A.14 depend on the relative sizes of the first term and the second term.
Rights and permissions
About this article
Cite this article
Hamada, K., Kaneko, A. & Yanagihara, M. The transfer paradox in a pay-as-you-go pension system. Int Econ Econ Policy 14, 221–238 (2017). https://doi.org/10.1007/s10368-016-0338-2
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10368-016-0338-2