Piezo-viscous-polar lubrication of hybrid journal bearing under misaligned operation

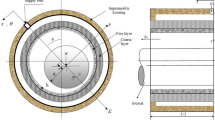
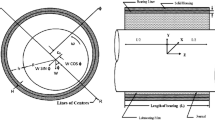
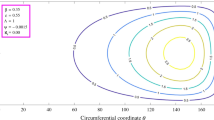
Abstract

Polymer based additives with long chain of suspended particles in oil retain polarity effect in the presence of couple stresses. Further, at high speed of journal bearing, the oil film experiences substantial agitation due to pressure-viscosity interaction which evolve piezo-viscous dependency of the lubricant. The present article addresses the combined effects of piezo-viscous dependency and couple stresses of lubricant on the characteristics of multi-recessed hybrid journal bearing under misaligned operation. To govern the flow of piezo-viscous-polar lubricant in clearance space of the journal bearing, a piezo-polar function is used to obtain the modified form of Reynolds equation. Galerkin’s technique is employed to obtain the finite element formulation in the form of algebraic equation, which is solved to determine the unknown pressure field in the bearing system. The present results reveal that the use of piezo-viscous-polar lubricant offers an enhancement in performance characteristics of bearing system, i.e., a significant hike of 13–36%, 27–30% and 7–10% in the values of $\overline{S}_{ij}$, $\overline{C}_{ij}$ and $\overline{\omega }_{th}$ respectively vis-à-vis Newtonian lubricant. The numerically simulated non-dimensional results presented in the article may be useful to generate the bearing design data of the hybrid journal baring system functioning in realistic and stringent operating conditions.

Appendices

Appendix A

Derivation of modified form of Reynold’s equation

The flow of a piezo-viscous-polar lubricant is governed by continuity equation (Eq. 1), momentum balance equation (Stokes theory of couple stress fluid) (Eq. 2) and Barus’s law (Eq. 3) [2, 30, 49].

The following assumptions incorporated in the derivation of generalized Reynolds equation governing the flow PVP lubricant in a finite width journal bearing system [66]:

• 1. The lubricant is incompressible, i.e., $\nabla .\vec{u}$ = 0

• 2. The flow is two dimensional, i.e., $w$ = 0; $\vec{u}$ = $u\hat{i} + v\hat{j}$

• 3. The flow is laminar.

• 4. Inertia forces are negligible i.e., $\rho \frac{{D\vec{u}}}{Dt}$ = 0

• 5. Body forces per unit mass are negligible i.e., $\vec{B}_{f}$ = 0

• 6. Body couples per unit mass are neglected i.e., $\vec{C}$ = 0

• 7. The axial length of the bearing system is sufficient that results no change in the flow field with respect to axial dimension i.e., $\frac{{\partial \vec{u}}}{\partial y} = 0$ which means $u$ = $u\left( {x,z} \right)$, $v$ = $v\left( {x,z} \right)$

• 8. Above assumptions results in pressure variation across the fluid film thickness is zero, i.e., $\frac{\partial p}{{\partial z}}$ = 0

• 9. The velocity gradients in X direction are very small as compared to Z direction across the fluid film thickness, i.e., $\frac{\partial u}{{\partial x}}$ <  <  <  < $\frac{\partial u}{{\partial z}}$ and $\frac{\partial v}{{\partial x}}$ <  <  <  < $\frac{\partial v}{{\partial z}}$

Incorporating the assumptions of lubricant flow in clearance space of bearing system in Eqs. (2) and (3) in X and Y direction can be expressed as follows:

$$\frac{{\partial^{4} u}}{{\partial z^{4} }} - \frac{{\mu_{0} e^{{\alpha_{pv} p}} }}{\eta }\frac{{\partial^{2} u}}{{\partial z^{2} }} = - \frac{1}{\eta }\frac{\partial p}{{\partial x}}$$

(A.1a)

$$\frac{{\partial^{4} v}}{{\partial z^{4} }} - \frac{{\mu_{0} e^{{\alpha_{pv} p}} }}{\eta }\frac{{\partial^{2} v}}{{\partial z^{2} }} = - \frac{1}{\eta }\frac{\partial p}{{\partial y}}$$

(A.1b)

Further, these Eqs. (A.1a, A.1b) can also be modified as:

$$\frac{{\partial^{4} u}}{{\partial z^{4} }} - \frac{{e^{{\alpha_{pv} p}} }}{{l_{cs}^{2} }}\frac{{\partial^{2} u}}{{\partial z^{2} }} = - \frac{1}{\eta }\frac{\partial p}{{\partial x}}$$

(A.2a)

$$\frac{{\partial^{4} v}}{{\partial z^{4} }} - \frac{{e^{{\alpha_{pv} p}} }}{{l_{cs}^{2} }}\frac{{\partial^{2} v}}{{\partial z^{2} }} = - \frac{1}{\eta }\frac{\partial p}{{\partial y}}$$

(A.2b)

where, couple stress parameter, $l_{cs} = \sqrt {\frac{\eta }{{\mu_{0} }}}$.

Solving the differential Eqs. (A.2a, A.2b), the generalized solution for velocity components ($u$ and $v$) may be expressed as:

$$u = A_{0} + A_{1} z + A_{2} \cosh \left( {\frac{{ze^{{\frac{{\alpha_{pv} p}}{2}}} }}{{l_{cs} }}} \right) + A_{3} \sinh \left( {\frac{{ze^{{\frac{{\alpha_{pv} p}}{2}}} }}{{l_{cs} }}} \right) + \frac{1}{{2\mu_{0} e^{{\alpha_{pv} p}} }}\frac{\partial p}{{\partial x}}z^{2}$$

(A.3)

$$v = B_{0} + B_{1} z + B_{2} \cosh \left( {\frac{{ze^{{\frac{{\alpha_{pv} p}}{2}}} }}{{l_{cs} }}} \right) + B_{3} \sinh \left( {\frac{{ze^{{\frac{{\alpha_{pv} p}}{2}}} }}{{l_{cs} }}} \right) + \frac{1}{{2\mu_{0} e^{{\alpha_{pv} p}} }}\frac{\partial p}{{\partial y}}z^{2}$$

(A.4)

where, $A_{0} ,A_{1} ,A_{2} ,A_{3} ,B_{0} ,B_{1} ,B_{2} {\text{ and }}B_{3}$ are constants.

Applying the boundary conditions [10, 67, 68] in Eqs. (A.3-A.4), the constants of generalized velocity expression yield as:

Constants	Computed value of constants
$A_{0}$	$\frac{{l^{2} }}{{\mu_{0} }}\frac{\partial p}{{\partial x}}e^{{ - 2\alpha_{pv} p}}$
$A_{1}$	$\frac{U}{h} - \frac{1}{2\mu_{0}}\frac{\partial p}{\partial x}\frac{h}{e^{\alpha_{pv}p}}$
$A_{2}$	$- \frac{{l_{cs}^{2} }}{{\mu_{0} }}\frac{\partial p}{{\partial x}}e^{{ - 2\alpha_{pv} p}}$
$A_{3}$	$\left[ {\cosh \left( {\frac{{he^{{\frac{{\alpha_{pv} p}}{2}}} }}{{l_{cs} }}} \right) - 1} \right]\left( {\frac{{l_{cs}^{2} }}{{\mu_{0} }}\frac{\partial p}{{\partial x}}} \right)\frac{{e^{{ - 2\alpha_{pv} p}} }}{{\sinh \left( {\frac{{he^{{\frac{{\alpha_{pv} p}}{2}}} }}{{l_{cs} }}} \right)}}$
$B_{0}$	$\frac{{l^{2} }}{{\mu_{0} }}\frac{\partial p}{{\partial y}}e^{{ - 2\alpha_{pv} p}}$
$B_{1}$	$- \frac{1}{2\mu_{0}}\frac{\partial p}{\partial y}\frac{h}{e^{\alpha_{pv}p}}$
$B_{2}$	$- \frac{{l_{cs}^{2} }}{{\mu_{0} }}\frac{\partial p}{{\partial y}}e^{{ - 2\alpha_{pv} p}}$
$B_{3}$	$\left[ {\cosh \left( {\frac{{he^{{\frac{{\alpha_{pv} p}}{2}}} }}{{l_{cs} }}} \right) - 1} \right]\left( {\frac{{l_{cs}^{2} }}{{\mu_{0} }}\frac{\partial p}{{\partial y}}} \right)\frac{{e^{{ - 2\alpha_{pv} p}} }}{{\sinh \left( {\frac{{he^{{\frac{{\alpha_{pv} p}}{2}}} }}{{l_{cs} }}} \right)}}$

Using the computed values of constants of generalised velocity expressions, the simplified expression of velocity components u and v in X and Y directions respectively are derived as follows (A.5, A.6):

$$u = \frac{Uz}{h} + \frac{{e^{{ - 2\alpha_{pv} p}} }}{{2\mu_{0} }}\frac{\partial p}{{\partial x}}\left\{ {\frac{{z\left( {z - h} \right)}}{{e^{{ - \alpha_{pv} p}} }} + 2l_{cs}^{2} \left[ {1 - \frac{{\cosh \left( {\frac{2z - h}{{2l_{cs} e^{{ - \frac{1}{2}\alpha_{pv} p}} }}} \right)}}{{\cosh \left( {\frac{h}{{2l_{cs} e^{{ - \frac{1}{2}\alpha_{pv} p}} }}} \right)}}} \right]} \right\}$$

(A.5)

$$v = \frac{{e^{{ - 2\alpha_{pv} p}} }}{{2\mu_{0} }}\frac{\partial p}{{\partial y}}\left\{ {\frac{{z\left( {z - h} \right)}}{{e^{{ - \alpha_{pv} p}} }} + 2l_{cs}^{2} \left[ {1 - \frac{{\cosh \left( {\frac{2z - h}{{2l_{cs} e^{{ - \frac{1}{2}\alpha_{pv} p}} }}} \right)}}{{\cosh \left( {\frac{h}{{2l_{cs} e^{{ - \frac{1}{2}\alpha_{pv} p}} }}} \right)}}} \right]} \right\}$$

(A.6)

The integration of Eqs. (A.5, A.6) across the oil film thickness direction yields the flow rates per unit width in $X and Y$ direction which maybe expressed as:

$$q_{x} = \int\limits_{0}^{h} {udz} = \frac{Uh}{2} - \frac{1}{{12\mu_{0} }}\frac{\partial p}{{\partial x}}\Psi \left( {h,l_{cs} ,\alpha_{pv} ,p} \right)$$

(A.7)

$$q_{y} = \int\limits_{0}^{h} {vdz} = - \frac{1}{{12\mu_{0} }}\frac{\partial p}{{\partial y}}\Psi \left( {h,l_{cs} ,\alpha_{pv} ,p} \right)$$

(A.8)

where,

$$\Psi \left( {h,l_{cs} ,\alpha_{pv} ,p} \right) = h^{3} e^{{ - \alpha_{pv} p}} - 12l_{cs}^{2} \left[ {he^{{ - 2\alpha_{pv} p}} - 2l_{cs} e^{{ - \frac{5}{2}\alpha_{pv} p}} \tanh \left( {\frac{{he^{{\frac{1}{2}\alpha_{pv} p}} }}{{2l_{cs} }}} \right)} \right]$$

For 2D incompressible flow of the lubricant, integrating the continuity Eq. (2.1) across the oil the film thickness transforms as:

$$\frac{\partial h}{{\partial t}} + \frac{{\partial q_{x} }}{\partial x} + \frac{{\partial q_{y} }}{\partial y} = 0$$

(A.9)

Substituting the expressions for fluid flow rates (A.7, A.8) in integrated continuity equation (A.9), the modified form of Reynold’s equation yields as:

$$\frac{\partial }{\partial x}\left( {\frac{{\Psi \left( {h,l_{cs} ,\alpha_{pv} ,p} \right)}}{{12\mu_{0} }}\frac{\partial p}{{\partial x}}} \right) + \frac{\partial }{\partial y}\left( {\frac{{\Psi \left( {h,l_{cs} ,\alpha_{pv} ,p} \right)}}{{12\mu_{0} }}\frac{\partial p}{{\partial y}}} \right) = \frac{U}{2}\frac{\partial h}{{\partial x}} + \frac{\partial h}{{\partial t}}$$

(A.10)