Normal Indentation of Rock Specimens with a Blunt Tool: Role of Specimen Size and Indenter Geometry

Abstract

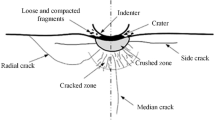
Indentation testing has been widely used in laboratory environments to investigate the processes leading to rock fragmentation in drilling, mechanized tunneling, and mining. Rock specimens for laboratory testing are limited to finite size, potentially causing size effects that have to be accounted for when transferring results to in situ applications. We present an integrated experimental and theoretical investigation of the specimen size effect in indentation testing (a) to address the limited understanding of its causes and the lack of tools to analyze tests on variable specimen sizes and (b) to identify to what extent an indenter mimicking the shape of a cutter on a tunneling machine can be approximated by a conventional indenter geometry. We performed indentation tests on cylindrical specimens of a porous sandstone with aspect ratios (diameter/height) ranging from 0.3 to 1.7, using a blunt-truncated indenter and monitoring the fracturing process by the acoustic emission technique. A damage zone, enclosing a zone of crushed grains immediately below the indenter tip, forms and grows due to tool penetration. Eventually, all specimens failed as a result of the propagation of a sub-vertical fracture, initiated close to peak indentation pressure. Peak force, its corresponding penetration depth, and peak indentation pressure increase with specimen size, more significantly with specimen diameter than with height. We developed a semi-analytical model based on cavity-expansion theory and linear elastic fracture mechanics for the formation of the damage zone and the nucleation and propagation of the macroscopic vertical fracture, respectively, whose predictions are in good agreement with our experimental data. The observed increases of peak indentation pressure with specimen size can be explained by the effect of the free surfaces on damage zone growth rather than on fracture propagation. The model permits evaluating the specimen size effect through the ratio between two geometrical parameters, specimen diameter and tip width of the truncated indenter, which has to be larger than around 10² for the size effect to be insignificant. The model permits upscaling of experimental results to in situ conditions based on geometrical indenter parameters and commonly used material parameters.

Highlights

• The series of indentation tests performed with the aid of acoustic emission technique reveals the significant influence of specimen size on test results.

• The proposed novel semi-analytical model is proved useful to analyse indentation tests on variable specimen sizes and indenter geometries including indenters with a truncated tip.

• The presented detailed mechanistic analysis for indentation tests identifies key parameters controlling rock fragmentation process and permits transferring laboratory results to in-situ applications.

Appendices

Appendix 1

1.1 Cavity Expansion in Mohr–Coulomb Rock of Finite Radial Extent

Previous applications of cavity-expansion theory to rock indentation, for example, with a regular indenter (Huang et al. 1998; Huang 1999) or a blunt tool (Alehossein et al. 2000), did not incorporate the specimen size. We modify the solutions of Huang et al. (1998) and Alehossein et al. (2000) by incorporating the stresses and displacements around a cavity in a finite specimen, introduced in previous studies (Pournaghiazar et al. 2013; Cheng et al. 2018; Cheng and Yang 2019).

1.1.1 Definition of the Problem and Notation

The indentation process is idealized by describing the contact surface between the indenter and the rock as a hemispherical or a semicircular dent of radius a, which is surrounded by a hemispherical or a semicircular damage zone with radius $R$ (Fig.

Fig. 14

Approximation of the tangential or hoop stress from cavity-expansion analysis using Eqs. (47) and (48) outside of the damage zone

14). The semicircular dent corresponds to plane strain conditions and models a wedge indenter, while the hemi-spherically symmetric conditions apply to a cone indenter. The damage region, in turn, is surrounded by elastically deforming rock to boundary $B$, laterally determined by the specimen radius. We use spherical (r, ω, θ) and cylindrical (r, z, θ) coordinates matching hemispherical and semicircular dent geometry, respectively, and introduce a dimensionless radius $\xi = r/a$. Radial stress $\sigma_{r}$ and tangential stress $\sigma_{\theta }$ represent major and minor principal stress. We follow the engineering sign convention, i.e., compressive stresses are negative. Model parameters are shear modulus $G$, Poisson’s ratio $\mu$, uniaxial compressive strength $\sigma_{{{\text{uni}}}}$, angle of internal friction $\phi$, dilation angle $\psi$, a parameter $k$ varying with cavity geometry, $k = 1$ and 2 for cylindrical and hemispherical cavity, respectively (e.g., Collins and Stimpson 1994), and the various parameters of indenter geometry, such as the inclination angle $\alpha$ with respect to the indented specimen surface and the width of the blunt tip $2a_{0}$.

1.1.2 Finite Elastic Region

The finite elastic region $\xi_{{\text{R}}} \le \xi \le \xi_{{\text{B}}} \, \left( {R \le r \le B} \right)$ requires modifications of the stresses and displacements in Alehossein et al. (2000). We use the solutions by Cheng et al. (2018) for the stresses and displacements around an expanding cavity:

$$\sigma_{r} = \sigma_{{\text{R}}} \frac{{\left( {\frac{{\xi_{{\text{R}}} }}{{\xi_{{}} }}} \right)^{k + 1} - m}}{1 - m},$$

(9)

$$\sigma_{\theta } = - \frac{{\sigma_{{\text{R}}} }}{k}\frac{{\left( {\frac{{\xi_{{\text{R}}} }}{\xi }} \right)^{k + 1} + km}}{1 - m},$$

(10)

and

$$u(r,\xi ) = - \frac{{\sigma_{{\text{R}}} r}}{2kG}\frac{{\left( {\frac{{\xi_{{\text{R}}} }}{\xi }} \right)^{k + 1} + mC}}{1 - m},$$

(11)

respectively, where

$$m = \left( \frac{R}{B} \right)^{k + 1} = \left( {\frac{{\xi_{{\text{R}}} }}{{\xi_{{\text{B}}} }}} \right)^{k + 1} ,$$

(12)

and

$$C = \frac{{k\left( {1 - 2\mu } \right)}}{{1 + \mu \left( {k - 1} \right)}}.$$

(13)

1.1.3 Plastic Region and Elastic-Plastic Boundary

In the plastic region $1 \le \xi \le \xi_{{\text{R}}} \, \left( {a \le r \le R} \right)$, the stress states satisfy the Mohr–Coulomb failure criterion:

$$\sigma_{r} - h = K_{{\text{p}}} \left( {\sigma_{\theta } - h} \right),$$

(14)

where

$$K_{{\text{p}}} = \frac{1 + \sin \phi }{{1 - \sin \phi }}$$

(15)

and

$$h = \frac{{\sigma_{{{\text{uni}}}} }}{{K_{{\text{p}}} - 1}}.$$

(16)

The stress state has to satisfy the equilibrium equation

$$\frac{{\partial \sigma_{r} }}{\partial \xi }{ + }k\frac{{\sigma_{r} - \sigma_{\theta } }}{\xi } = 0.$$

(17)

Using the boundary condition at the cavity wall $\sigma_{r} = - p$ ($p$ compressive) at $r = a$ and combining Eqs. (14) and (17) gives the stresses in the plastic region as

$$\sigma_{r} = h - \left( {p + h} \right)\xi^{ - \beta }$$

(18)

and

$$\sigma_{\theta } = h - \frac{{\left( {p + h} \right)}}{{K_{{\text{p}}} }}\xi^{ - \beta } ,$$

(19)

where

$$\beta = \frac{{k\left( {K_{{\text{p}}} - 1} \right)}}{{K_{{\text{p}}} }}.$$

(20)

Stress continuity across the elastic–plastic boundary yields

$$\sigma_{r} = h\left[ {1 - f\left( m \right)\left( {\frac{{\xi_{{\text{R}}} }}{\xi }} \right)^{\beta } } \right]$$

(21)

and

$$\sigma_{\theta } = h\left[ {1 - \frac{f\left( m \right)}{{K_{{\text{p}}} }}\left( {\frac{{\xi_{{\text{R}}} }}{\xi }} \right)^{\beta } } \right],$$

(22)

where

$$f\left( m \right) = \frac{{K_{{\text{p}}} \left( {k + 1} \right)}}{{K_{{\text{p}}} + k\left[ {1 - m\left( {1 - K_{{\text{p}}} } \right)} \right]}} \ge 1.$$

(23)

Since $f\left( m \right)$ decreases with increasing $R/B$, i.e., decreasing distance between the elastic–plastic boundary and the specimen’s free surface, the absolute values of radial and tangential stresses at the elastic–plastic boundary, Eqs. (21) and (22), are reduced by finite specimen size.

For strains in the plastic region 1 ≤ ξ ≤ ξ_R (a ≤ r ≤ R), the conventional non-associative flow rule for the Mohr–Coulomb model is assumed, i.e.,

$$\frac{{\dot{\varepsilon }_{r}^{{\text{p}}} }}{{\dot{\varepsilon }_{\theta }^{{\text{p}}} }} = - \frac{k}{{K_{{\text{d}}} }}$$

(24)

with

$$\dot{\varepsilon }_{r}^{{}} = \frac{\partial v}{{\partial r}}{, }\dot{\varepsilon }_{\theta }^{{}} = \frac{v}{r},$$

(25)

where $v = {{{\text{d}}u} \mathord{\left/ {\vphantom {{{\text{d}}u} {{\text{d}}t}}} \right. \kern-\nulldelimiterspace} {{\text{d}}t}}$ denotes the radial particle velocity, and

$$K_{{\text{d}}} = \frac{1 + \sin \psi }{{1 - \sin \psi }}.$$

(26)

Decomposing the total strain into elastic and plastic strains and combining Eqs. (24) and (25), Hook’s law for elastic radial and tangential strains, and the yield function (14) lead to

$$K_{{\text{d}}} \frac{\partial v}{{\partial \xi }} + k\frac{v}{\xi } = \frac{{a\lambda \dot{\sigma }_{r} }}{2G},$$

(27)

where $\lambda$ is given by

$$\lambda = \frac{{\left( {K_{{\text{p}}} - 1} \right)\left( {K_{{\text{d}}} - 1} \right) + \left( {1 - 2\mu } \right)\left[ {\left( {K_{{\text{p}}} + 1} \right)\left( {K_{{\text{d}}} + 1} \right) - \left( {\mu - 1} \right)K_{{\text{d}}} K_{{\text{p}}} } \right]}}{{\left( {3 - k} \right)\left( {1 + \mu } \right)^{k - 1} K_{{\text{p}}} }}.$$

(28)

The material derivative of radial stress Eq. (21) is

$$\begin{gathered} \dot{\sigma }_{r} = \frac{{{\text{d}}\sigma_{r} }}{{{\text{d}}t}} = \frac{{\partial \sigma_{r} }}{\partial t} + v\frac{{\partial \sigma_{r} }}{\partial r} = \left( {\frac{{\partial \sigma_{r} }}{\partial R}\frac{\partial R}{{\partial t}} + \frac{{\partial \sigma_{r} }}{\partial a}\frac{\partial a}{{\partial t}}} \right) + \frac{v}{a}\frac{{\partial \sigma_{r} }}{\partial \xi } \\ = hf\left( m \right)\left( {\frac{{\xi_{{\text{R}}} }}{\xi }} \right)^{\beta } \left[ {\frac{\beta }{a}\frac{{v - \xi \dot{a}}}{\xi } - \frac{\beta }{{\xi_{{\text{R}}} }}\left( {1 + f\left( m \right)m} \right)\frac{{{\text{d}}\xi_{{\text{R}}} }}{{{\text{d}}a}}\dot{a}} \right]. \\ \end{gathered}$$

(29)

Substituting Eq. (29) into Eq. (37) yields

$$\begin{gathered} K_{{\text{d}}} \frac{\partial v}{{\partial \xi }} + k\frac{v}{\xi }\left[ {1 - \frac{\lambda \beta f\left( m \right)}{{2k}}\frac{h}{G}\left( {\frac{{\xi_{{\text{R}}} }}{\xi }} \right)^{\beta } } \right] = \hfill \\ \, - \frac{\lambda \beta f\left( m \right)}{2}\frac{h}{G}\left[ {1 + \frac{a}{{\xi_{{\text{R}}} }}\left( {1 + f\left( m \right)m} \right)\frac{{{\text{d}}\xi_{{\text{R}}} }}{{{\text{d}}a}}} \right]\left( {\frac{{\xi_{{\text{R}}} }}{\xi }} \right)^{\beta } \dot{a}. \hfill \\ \end{gathered}$$

(30)

To solve this inhomogeneous differential equation for the radial particle velocity, a boundary condition is required. The material derivative of the radial displacement component, i.e., ${{{\text{d}}u} \mathord{\left/ {\vphantom {{{\text{d}}u} {{\text{d}}t}}} \right. \kern-\nulldelimiterspace} {{\text{d}}t}} = v = {{\partial u} \mathord{\left/ {\vphantom {{\partial u} {\partial t}}} \right. \kern-\nulldelimiterspace} {\partial t}} + v{{\partial u} \mathord{\left/ {\vphantom {{\partial u} {\partial r}}} \right. \kern-\nulldelimiterspace} {\partial r}}$ yields

$$v = \frac{{\frac{\partial u}{{\partial a}}\dot{a}}}{{1 - \frac{\partial u}{{\partial r}}}}.$$

(31)

Substituting (A.13) with $r = R$ into Eq. (11) gives the displacement in the elastic region as

$$u = \frac{\gamma r}{{\left( {k + 1} \right)}}\frac{{\left( {\frac{{\xi_{{\text{R}}} }}{\xi }} \right)^{k + 1} + Cm}}{1 - m}$$

(32)

with

$$\gamma = \left( {k + 1} \right)\frac{h}{2kG}\left( {f\left( m \right) - 1} \right).$$

(33)

Combining Eqs. (32) and (31) gives

$$v\left( {\xi_{{\text{R}}} } \right) = \frac{{\left( {k + 1} \right)\gamma }}{{\left( {k + 1} \right)\left( {1 - m} \right) + \gamma k\left( {1 - \frac{mC}{k}} \right)}}\left( {\xi_{{\text{R}}} + a\frac{{{\text{d}}\xi_{{\text{R}}} }}{{{\text{d}}a}}} \right) = C_{{\text{v}}} \left( {\xi_{{\text{R}}} + a\frac{{{\text{d}}\xi_{{\text{R}}} }}{{{\text{d}}a}}} \right)\dot{a}$$

(34)

Now, Eq. (30) can be solved as an initial value problem with the boundary condition given by Eq. (34). We omit the non-linear part in the brackets on the left-hand side of Eq. (30) because, dominated by the ratio $h/G \propto \sigma_{{{\text{uni}}}} /G$ that is of magnitude 10^–2, it is much smaller than 1 for relevant material parameters. Then, Eq. (30) reduces to a linear inhomogeneous differential equation of the form:

$y^{\prime} + a\left( \xi \right)y = f\left( \xi \right)$, Eq. (35).

with the general solution

$$y = \frac{{\int {\exp \left( {\int {a\left( \xi \right){\text{d}}\xi } } \right)f\left( \xi \right){\text{d}}\xi + {\text{const}}} }}{{\exp \left( {\int {a\left( \xi \right){\text{d}}\xi } } \right)}}.$$

(35)

Accordingly, the solution of Eq. (30) is

$$\begin{gathered} \frac{v(\xi )}{{\dot{a}}} = \left( {\frac{\chi \beta f\left( m \right)}{{2k}}\frac{h}{G}\xi_{{\text{R}}} + C_{v} \xi_{{\text{R}}} } \right)\left( {\frac{{\xi_{{\text{R}}} }}{\xi }} \right)^{{\frac{k}{{K_{{\text{d}}} }}}} - \frac{\chi \beta f\left( m \right)}{{2k}}\frac{h}{G}\xi_{{\text{R}}} \left( {\frac{{\xi_{{\text{R}}} }}{\xi }} \right)^{\beta - 1} \hfill \\ \, + \left\{ {\left[ {\frac{\chi \beta f\left( m \right)}{{2k}}\frac{h}{G}\left( {1 + f\left( m \right)m} \right) + C_{v} } \right]\left( {\frac{{\xi_{{\text{R}}} }}{\xi }} \right)^{{\frac{k}{{K_{{\text{d}}} }}}} - \frac{\chi \beta f\left( m \right)}{{2k}}\frac{h}{G}\left( {\frac{{\xi_{{\text{R}}} }}{\xi }} \right)^{\beta - 1} \left( {1 + f\left( m \right)m} \right)} \right\}a\frac{{{\text{d}}\xi_{{\text{R}}} }}{{{\text{d}}a}}. \hfill \\ \end{gathered}$$

(36)

where χ is given by

$$\chi = \frac{{k\lambda K_{{\text{p}}} }}{{k\left( {K_{{\text{p}}} + K_{{\text{d}}} } \right) + \left( {1 - k} \right)K_{{\text{p}}} K_{{\text{d}}} }}.$$

(37)

1.1.4 Size of Damage Zone and Indentation Pressure

We apply the developed cavity-expansion model to the indentation problem. The displacements produced by a blunt indenter are approximately radial and the plastic strain contours are hemispherical or semicircular in shape. An increment of penetration of the indenter is accompanied by an increment in the radial displacement of the cavity of Δa. Following Johnson (1970), we impose volume conversion requiring that the volume of the material displaced by the indenter, $\Delta V_{{{\text{IND}}}} = 2^{2 - k} \pi^{k - 1} a^{k} \tan \alpha \Delta a$, corresponds to the radial expansion of the hemispherical cavity, $\Delta V_{{{\text{CEM}}}} = 2^{k - 1} \pi a^{k} \Delta a$. The velocity at the cavity wall, i.e., ξ = 1, reads

$$\frac{v\left( 1 \right)}{{\dot{a}}} = \frac{{\Delta V_{{{\text{IND}}}} }}{{\Delta V_{{{\text{CEM}}}} }} = \frac{{2^{3 - 2k} }}{{\pi^{2 - k} }}\tan \alpha .$$

(38)

Combining Eqs. (37) and (39) gives a general expression for the evolution of the normalized damage zone size:

$$\begin{gathered} a\frac{{{\text{d}}\xi_{{\text{R}}} }}{{{\text{d}}a}} = \hfill \\ \, \frac{{\frac{f\left( m \right)}{h}\frac{2kG}{\beta }\frac{{2^{3 - 2k} }}{{\pi^{2 - k} }}\tan \alpha - \left( {\chi + C_{v} \frac{2kG}{{f\left( m \right)h\beta }}} \right)\xi_{{\text{R}}}^{{\frac{k}{{K_{{\text{d}}} }} + 1}} + \chi \xi_{{\text{R}}}^{\beta } }}{{\left[ {\chi \left( {1 + mf\left( m \right)} \right) + C_{{\text{v}}} \frac{2kG}{{f\left( m \right)h\beta }}} \right]\xi_{{\text{R}}}^{{\frac{k}{{K_{{\text{d}}} }}}} - \chi \left( {1 + mf\left( m \right)} \right)\xi_{{\text{R}}}^{\beta - 1} }}. \hfill \\ \end{gathered}$$

(39)

For a blunt-truncated indenter with a flat tip of width 2a₀, the contact length becomes

$$a = a_{0} + \frac{d}{\tan \alpha }.$$

(40)

Introducing the ratio of penetration depth $d$ and the length of the truncated tip

$$\delta = \frac{d}{{2a_{0} }},$$

(41)

the evolution of the damage zone size Eq. (40) becomes

$$\begin{gathered} \left( {\frac{\tan \,\alpha }{2} + \delta } \right)\frac{{d\xi_{R} }}{d\delta } = \hfill \\ \, \frac{{\frac{2kG}{{f\left( m \right)h\beta }}\frac{{2^{3 - 2k} }}{{\pi^{2 - k} }}\tan \alpha - \left( {\chi + C_{v} (\xi_{R} )\frac{2kG}{{f\left( m \right)h\beta }}} \right)\xi_{R}^{{\frac{k}{{K_{d} }} + 1}} + \chi \xi_{R}^{\beta } }}{{\left[ {\chi \left( {1 + mf\left( m \right)} \right) + C_{v} (\xi_{R} )\frac{2kG}{{f\left( m \right)h\beta }}} \right]\xi_{R}^{{\frac{k}{{K_{d} }}}} - \chi \xi_{R}^{\beta - 1} \left( {1 + m(\xi_{R} )f\left( m \right)} \right)}} \hfill \\ \end{gathered}$$

(42)

with the initial condition given by

$$\xi_{{\text{R}}} = 1\,\,{\text{at}}\,\,\delta = 0.$$

(43)

Equation (43) reduces to the solution of Alehossein et al. (2000) for m = 0, i.e., infinite specimen size. An analytical solution of Eq. (43) is hindered by the fact that $f_{{\text{m}}}$ and $C_{{\text{v}}}$ depend on $m$ introduced in Eq. (12), which is a function of $\xi_{R}$ and $\delta$:

$$m = \left[ {\frac{{a_{0} }}{B}\left( {1 + \frac{2\delta }{{\tan \alpha }}} \right)\xi_{{\text{R}}} } \right]^{k + 1} .$$

(44)

Therefore, Eq. (43) is solved numerically as an initial value problem using fourth Runge–Kutta method as in Yang and Russell (2015). Once $\xi_{R}$ is known, the indentation pressure is determined by Eq. (21) as:

$$\frac{p}{{\sigma_{{{\text{uni}}}} }} = \frac{{f\left( m \right)\xi_{{\text{R}}}^{\beta } - 1}}{{K_{{\text{p}}} - 1}}.$$

(45)

Appendix 2

2.1 Linear Elastic Fracture Mechanics

The growth of a single tensile fracture in the wake of indentation is treated within the framework of linear elastic fracture mechanics. The model is based on the milestone work of Lawn and Evans (1977) and is extended to incorporate specimen size effect and indenter geometry for frictional materials like rocks. The stress intensity factor for an axially symmetric penny-shaped crack of length 2c is given as (Tada et al. 2000)

$$ K_{{\text{I}}} = \frac{2}{{\sqrt {\pi c} }}\int_{0}^{c} {\frac{r\sigma \left( x \right)}{{\sqrt {c^{2} - x^{2} } }}{\text{d}}x} , $$

(46)

where the position $x$ corresponds to a coordinate axis aligned with the crack and with its origin at the center of the crack. We identify the tensile stress $\sigma (x)$ with the hoop stress at the elastic–plastic boundary from the cavity-expansion analysis Eq. (22). To simplify the following analysis, the power function Eq. (22) is approximated by a linear function (see Fig. 15):

Fig. 15

Effect of indenter geometry and geometrical factors, $R/B$ and $\xi_{{\text{R}}}$, on stress intensity factor according to Eq. (49) using material parameters as discussed in Sec. 3.2 and used for the cavity-expansion model, too

$$\sigma_{\theta } \left( x \right) = \sigma_{{\theta {\text{,EP}}}} \left( {1 - \frac{x}{b}} \right) = \frac{{\sigma_{{{\text{uni}}}} \left( {1 + mk} \right)}}{{k\left( {1 - m} \right) + K_{{\text{p}}} \left( {1 + mk} \right)}}\left( {1 - \frac{x}{b}} \right)$$

(47)

with the characteristic distance, over which the hoop stress decays in the elastic region:

$$b = C_{{\text{b}}} \xi_{{\text{R}}} a,$$

(48)

where $C_{{\text{b}}}$ is a dimensionless factor expected to be of order unity (Fig. 15). Equation (48) reflects the rule-of-thumb that a source perturbs stresses in a region with the source’s size and corresponds to the scaling argument made by Lawn and Evans (1977).

Precisely, C_b can be obtained by comparing the gradient of σ_θ at the elastic-plastic boundary, i.e., $\sigma _{\theta } (x) = \sigma _{{\theta ,{\text{EP}}}} + \left. {{{d\sigma _{\theta } } \mathord{\left/ {\vphantom {{d\sigma _{\theta } } {dx}}} \right. \kern-\nulldelimiterspace} {dx}}} \right|_{{x = R}} x$ with Eq. (47), so that

$$ C_{b} = \frac{{K_{{\text{p}}} - f\left( m \right)}}{{K_{{\text{p}}} }}\frac{{1 - m}}{{f\left( m \right) - 1}}\frac{k}{{k + 1}} .$$

Insertion of Eq. (47) into Eq. (46) and subsequent direct integration for $b > c$ lead to

$$K_{{\text{I}}} = \frac{{2\left( {1 + mk} \right)\sigma_{{{\text{uni}}}} }}{{k\left( {1 - m} \right) + K_{{\text{p}}} \left( {1 + mk} \right)}}\sqrt {\frac{c}{\pi }} \left( {1 - \frac{\pi c}{{4b}}} \right).$$

(49)

The stress intensity Eqs. (49) depends on cavity shape and specimen dimensions. Evaluation with the scaling relation Eq. (48) reveals that the stress intensity for a given crack size $c$ is larger for a cylindrical cavity than for a spherical cavity (Fig. 16). Furthermore, cracks of a given size experience larger stress intensities in smaller specimens (larger $R/B$) corresponding to a smaller $\xi_{{\text{R}}}$.

Fig. 16

Estimation of the angle of internal friction, one of the two parameters of the Mohr–Coulomb failure criterion

The stress intensity Eq. (49) exhibits a maximum for a crack length

$$c_{\max } = \frac{4}{3\pi }b,$$

(50)

i.e., a flaw that extends over ~ 40% of the distance range, for which a significant tensile hoop stress occurs in the elastic region (Fig. 15). The existence of this maximum is of key importance for the failure evolution. When a pre-existing flaw with $c_{{{\text{ini}}}} < c_{\max }$ reaches the critical stress intensity, i.e., $K_{{\text{I}}} (c_{{{\text{ini}}}} ) = K_{{{\text{IC}}}}$, its growth is associated with increasing stress intensity and thus indentation pressure decreases until the initial flaw reaches a length $c_{\max }$. As a consequence of the reduction in indentation pressure, the damage zone will exhibit limited growth during fracture growth and thus we can approximate $\xi_{{\text{R}}} (c_{{{\text{ini}}}} ) \simeq \xi_{{\text{R}}} (c_{\max } )$. Then also the penetration depths hold $d(c_{{{\text{ini}}}} ) \simeq d(c_{\max } )$, as supported by our experimental observation that the load decrease after the peak pressure occurs over a small range in penetration compared to the penetration associated with reaching the peak pressure, and thus, evaluating Eq. (46) for the maximum indentation pressure $p_{{{\text{max}}}} = p(c_{{{\text{ini}}}} )$, we arrive at

$$\frac{{p_{\max } }}{{\sigma_{{{\text{uni}}}} }} = \frac{1}{{K_{{\text{p}}} - 1}}\left\{ {\frac{{f_{{\text{m}}} }}{{B_{g}^{2\beta } }}\left[ {\frac{{\left( {\frac{{K_{{{\text{IC}}}} }}{{\sigma_{{{\text{uni}}}} }}} \right)^{2} }}{{\left( {a_{0} + \frac{{d_{{p{\text{m}}ax}} }}{\tan \alpha }} \right)C_{{\text{b}}} }}} \right]^{\beta } - 1} \right\}{ ,}$$

(51)

where

$$B_{{\text{g}}} = \frac{8}{3\sqrt 3 \pi }\frac{{\left( {1 + mk} \right)}}{{k\left( {1 - m} \right) + K_{p} \left( {1 + mk} \right)}}$$

(52)

is a parameter depending on indenter and specimen geometry.

Appendix 3

3.1 Failure Envelope of the Tested Rock

We use the results of triaxial compression tests, performed at confining pressures up to 150 MPa on specimens from a different block than the one used to prepare our specimens for the indentation tests, to estimate the parameters of the Mohr–Coulomb failure criterion. The failure envelope is slightly curved (Fig. 16). The internal friction angle used in this study is estimated based on the initial part of the curved failure envelope, covering mean stresses up to 200 MPa consistent with the stress state of the damage zone and is, therefore, larger than the angle obtained from the overall fitting.