X-FEM Modeling of Multizone Hydraulic Fracturing Treatments Within Saturated Porous Media

Abstract

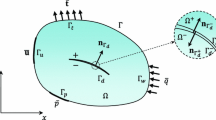
In this paper, a fully coupled hydro-mechanical model is presented for the study of multizone hydraulic fracturing. The momentum balance equation of the bulk together with the mass balance and momentum balance equation of the fluid phase are employed in order to derive the hydro-mechanical coupled system of governing equations of the porous media known as the $({\mathbf{u}} - p)$ formulation. The hydro-fracture inflow is modeled based on the Darcy law, where the fracture permeability is determined by using the cubic law. Provisions are made for the plausible closure as well as the frictional resistance of the fracture edges in the solid phase by means of Kuhn–Tucker inequalities embedded in an X-FEM penalty method. In addition, for the fluid phase, the zero leak-off constrain is imposed through the application of the large time increment-based contact algorithm in the case of crack closure. The cohesive crack model is employed to account for the nonlinear fracturing process at the hydro-fracture tips. Multiple crack growth patterns are determined by means of energy based cohesive stress functions. Based on the X-FEM, the strong discontinuities in the displacement field due to fracture opening as well as the weak discontinuities within the pressure field due to leak-off flow are incorporated by using the Heaviside and modified level-set enrichment functions, respectively. A consistent computational algorithm is proposed for the determination of the fracturing fluid flow distribution across the existing perforations. Finally, several numerical examples are presented to demonstrate the robustness of the proposed X-FEM framework in the study of multizone hydraulic fracturing treatments through saturated porous media. The results appear to accord with the field observations reporting numerous failed attempts of multistage multizone fracturing treatments, which provide a great insight into the complexities encountered in practice.

Appendix

The Jacobian matrix appearing in Newton–Raphson solution of the fully coupled nonlinear equation system (40) is denoted by

$${\mathbf{J}} = \left[ {\begin{array}{*{20}c} {{\mathbf{J}}_{uu} } & {{\mathbf{J}}_{{u\tilde{u}}} } & {{\mathbf{J}}_{up} } & {{\mathbf{J}}_{{u\tilde{p}}} } \\ {{\mathbf{J}}_{{\tilde{u}u}} } & {{\mathbf{J}}_{{\tilde{u}\tilde{u}}} } & {{\mathbf{J}}_{{\tilde{u}p}} } & {{\mathbf{J}}_{{\tilde{u}\tilde{p}}} } \\ {{\mathbf{J}}_{pu} } & {{\mathbf{J}}_{{p\tilde{u}}} } & {{\mathbf{J}}_{pp} } & {{\mathbf{J}}_{{p\tilde{p}}} } \\ {{\mathbf{J}}_{{\tilde{p}u}} } & {{\mathbf{J}}_{{\tilde{p}\tilde{u}}} } & {{\mathbf{J}}_{{\tilde{p}p}} } & {{\mathbf{J}}_{pp} } \\ \end{array} } \right],$$

(45)

in which

$$\begin{aligned} &{\mathbf{J}}_{uu} = \frac{{\partial {\varvec{\Psi}}^{u} }}{{\partial {\mathbf{U}}}} = {\mathbf{K}}_{uu} ,\quad {\mathbf{J}}_{{u\tilde{u}}} = \frac{{\partial {\varvec{\Psi}}^{u} }}{{\partial {\tilde{\mathbf{U}}}}} = {\mathbf{K}}_{{u\tilde{u}}} , \hfill \\ &{\mathbf{J}}_{up} = \frac{{\partial {\varvec{\Psi}}^{u} }}{{\partial {\mathbf{P}}}} = - {\mathbf{Q}}_{up} ,\quad {\mathbf{J}}_{{u\tilde{p}}} = \frac{{\partial {\varvec{\Psi}}^{u} }}{{\partial {\tilde{\mathbf{P}}}}} = - {\mathbf{Q}}_{{u\tilde{p}}} , \hfill \\ &{\mathbf{J}}_{{\tilde{u}u}} = \frac{{\partial {\varvec{\Psi}}^{{\tilde{u}}} }}{{\partial {\mathbf{U}}}} = {\mathbf{K}}_{{\tilde{u}u}} ,\quad {\mathbf{J}}_{{\tilde{u}\tilde{u}}} = \frac{{\partial {\varvec{\Psi}}^{{\tilde{u}}} }}{{\partial {\tilde{\mathbf{U}}}}} = {\mathbf{K}}_{{\tilde{u}\tilde{u}}} + {\mathbf{K}}_{\text{Solid}}^{\text{cont}} + {\mathbf{K}}^{\text{cohs}} - \frac{{\partial \varvec{f}^{\text{intf}} }}{{\partial {\tilde{\mathbf{U}}}}}, \hfill \\ &{\mathbf{J}}_{{\tilde{u}p}} = \frac{{\partial {\varvec{\Psi}}^{{\tilde{u}}} }}{{\partial {\mathbf{P}}}} = - {\mathbf{Q}}_{{\tilde{u}p}} - \frac{{\partial \varvec{f}^{\text{intf}} }}{{\partial {\mathbf{P}}}},\quad {\mathbf{J}}_{{\tilde{u}\tilde{p}}} = \frac{{\partial {\varvec{\Psi}}^{{\tilde{u}}} }}{{\partial {\tilde{\mathbf{P}}}}} = - {\mathbf{Q}}_{{\tilde{u}\tilde{p}}} - \frac{{\partial \varvec{f}^{\text{intf}} }}{{\partial {\tilde{\mathbf{P}}}}}, \hfill \\ &{\mathbf{J}}_{pu} = \frac{{\partial {\varvec{\Psi}}^{p} }}{{\partial {\mathbf{U}}}} = \frac{\theta }{\Delta t}{\mathbf{Q}}_{up}^{T} - \frac{{\partial \varvec{q}_{p}^{\text{intf}} }}{{\partial {\mathbf{U}}}},\quad {\mathbf{J}}_{{p\tilde{u}}} = \frac{{\partial {\varvec{\Psi}}^{p} }}{{\partial {\tilde{\mathbf{U}}}}} = \frac{\theta }{\Delta t}{\mathbf{Q}}_{{\tilde{u}p}}^{T} - \frac{{\partial \varvec{q}_{p}^{\text{intf}} }}{{\partial {\tilde{\mathbf{U}}}}} \hfill \\ &{\mathbf{J}}_{pp} = \frac{{\partial {\varvec{\Psi}}^{p} }}{{\partial {\mathbf{P}}}} = {\mathbf{H}}_{pp} + \frac{1}{{\bar{\theta }\Delta t}}{\mathbf{S}}_{pp} - \frac{{\partial \varvec{q}_{p}^{\text{intf}} }}{{\partial {\mathbf{P}}}},\quad {\mathbf{J}}_{{p\tilde{p}}} = \frac{{\partial {\varvec{\Psi}}^{p} }}{{\partial {\tilde{\mathbf{P}}}}} = {\mathbf{H}}_{{p\tilde{p}}} + \frac{1}{{\bar{\theta }\Delta t}}{\mathbf{S}}_{{p\tilde{p}}} - \frac{{\partial \varvec{q}_{p}^{\text{intf}} }}{{\partial {\tilde{\mathbf{P}}}}} \hfill \\ &{\mathbf{J}}_{{\tilde{p}u}} = \frac{{\partial {\varvec{\Psi}}^{{\tilde{p}}} }}{{\partial {\mathbf{U}}}} = \frac{\theta }{\Delta t}{\mathbf{Q}}_{{u\tilde{p}}}^{T} - \frac{{\partial \varvec{q}_{{\tilde{p}}}^{\text{intf}} }}{{\partial {\mathbf{U}}}},\quad {\mathbf{J}}_{{\tilde{p}\tilde{u}}} = \frac{{\partial {\varvec{\Psi}}^{{\tilde{p}}} }}{{\partial {\tilde{\mathbf{U}}}}} = \frac{\theta }{\Delta t}{\mathbf{Q}}_{{\tilde{u}\tilde{p}}}^{T} - \frac{{\partial \varvec{q}_{{\tilde{p}}}^{\text{intf}} }}{{\partial {\tilde{\mathbf{U}}}}} \hfill \\ &{\mathbf{J}}_{{\tilde{p}p}} = \frac{{\partial {\varvec{\Psi}}^{{\tilde{p}}} }}{{\partial {\mathbf{P}}}} = {\mathbf{H}}_{{\tilde{p}p}} + \frac{1}{{\bar{\theta }\Delta t}}{\mathbf{S}}_{{\tilde{p}p}} - \frac{{\partial \varvec{q}_{{\tilde{p}}}^{\text{intf}} }}{{\partial {\mathbf{P}}}},\quad {\mathbf{J}}_{{\tilde{p}\tilde{p}}} = \frac{{\partial {\varvec{\Psi}}^{{\tilde{p}}} }}{{\partial {\tilde{\mathbf{P}}}}} = {\mathbf{H}}_{{\tilde{p}\tilde{p}}} + \frac{1}{{\bar{\theta }\Delta t}}{\mathbf{S}}_{{\tilde{p}\tilde{p}}} - \frac{{\partial \varvec{q}_{{\tilde{p}}}^{\text{intf}} }}{{\partial {\tilde{\mathbf{P}}}}} + {\mathbf{K}}_{\text{fluid}}^{\text{cont}} . \hfill \\ \end{aligned}$$

(46)

The matrixes ${\mathbf{K}}$, ${\mathbf{Q}}$, ${\mathbf{H}}$, and ${\mathbf{S}}$ are defined through relation (34). The contact stiffness matrix associated with the solid and fluid phases ${\mathbf{K}}_{\text{solid}}^{\text{cont}}$ and ${\mathbf{K}}_{\text{fluid}}^{\text{cont}}$ are, respectively, defined as

$$\begin{aligned} {\mathbf{K}}_{\text{solid}}^{\text{cont}} &= \int_{{\Gamma_{c} }} {({\mathbf{N}}_{u}^{\text{std}} )^{\text{T}} {\mathbf{D}}^{\text{cont}} {\mathbf{N}}_{u}^{\text{std}} } {\text{d}}\Gamma , \hfill \\ {\mathbf{K}}_{\text{fluid}}^{\text{cont}} &= \int_{{\Gamma_{c} }} {({\mathbf{N}}_{p}^{\text{std}} )^{\text{T}} {{\bar{\varvec{k}}_{f} } \mathord{\left/ {\vphantom {{\bar{\varvec{k}}_{f} } {\mu_{f} }}} \right. \kern-0pt} {\mu_{f} }}{\mathbf{\mathcal{N}}}_{{\Gamma_{d} }} {\mathbf{N}}_{p}^{\text{std}} } {\text{d}}\Gamma , \hfill \\ \end{aligned}$$

(47)

where ${\mathbf{\mathcal{N}}}_{{\Gamma_{d} }} = {\mathbf{n}}_{{\Gamma_{d} }} ({\mathbf{n}}_{{\Gamma_{d} }} )^{\text{T}}$. Moreover, the stiffness matrix ${\mathbf{K}}^{\text{cohs}}$ corresponding to the cohesive forces is expressed as,

$${\mathbf{K}}^{\text{cohs}} = \int_{{\Gamma_{d} }} {({\mathbf{N}}_{u}^{\text{std}} )^{\text{T}} {\mathbf{D}}^{\text{cohs}} \,{\mathbf{N}}_{u}^{\text{std}} } {\text{d}}\Gamma$$

(48)

where ${\mathbf{D}}^{\text{coh}} = \partial {\mathbf{t}}_{\text{coh}} /\partial \left[\kern-0.15em\left[ {\mathbf{u}} \right]\kern-0.15em\right]$.It is beneficial to symmetrize the non-symmetrical Jacobian matrix deduced in Newton–Raphson solution algorithm in favor of core storage and performance speed. The partial derivatives of the interfacial force vector $\varvec{f}^{\text{intf}}$ can be evaluated as

$$\begin{aligned} \frac{{\partial \varvec{f}^{\text{intf}} }}{{\partial {\mathbf{P}}}} = {\mathbf{Q}}_{{\tilde{u}p}}^{\text{intf}} = \int_{{\Gamma_{d} }} {{\mathbf{(N}}_{u}^{\text{std}} )^{T} {\mathbf{n}}_{{\Gamma_{d} }} {\mathbf{N}}_{p}^{\text{std}} {\text{d}}\Gamma } , \hfill \\ \frac{{\partial \varvec{f}^{\text{intf}} }}{{\partial {\tilde{\mathbf{P}}}}} = {\mathbf{Q}}_{{\tilde{u}\tilde{p}}}^{\text{intf}} = \int_{{\Gamma_{d} }} {{\mathbf{(N}}_{u}^{\text{std}} )^{T} {\mathbf{n}}_{{\Gamma_{d} }} {\mathbf{N}}_{p}^{{\text{ridge}}} {\text{d}}\Gamma } . \hfill \\ \end{aligned}$$

(49)

The terms arising from the partial derivatives of the interfacial flux vector $\varvec{q}^{\text{intf}}$ with respect to ${\mathbf{U}}$, i.e., ${{\partial \varvec{q}_{p}^{\text{intf}} } \mathord{\left/ {\vphantom {{\partial \varvec{q}_{p}^{\text{intf}} } {\partial {\mathbf{U}}}}} \right. \kern-0pt} {\partial {\mathbf{U}}}}$ and ${{\partial \varvec{q}_{{\tilde{p}}}^{\text{intf}} } \mathord{\left/ {\vphantom {{\partial \varvec{q}_{{\tilde{p}}}^{\text{intf}} } {\partial {\mathbf{U}}}}} \right. \kern-0pt} {\partial {\mathbf{U}}}}$, are omitted from the Jacobian matrix in order to retain the symmetry of the Jacobian matrix with respect to the above mentioned components. Meanwhile, the partial derivatives with respect to ${\tilde{\mathbf{U}}}$, i.e., ${{\partial \varvec{q}_{p}^{\text{int}} } \mathord{\left/ {\vphantom {{\partial \varvec{q}_{p}^{\text{int}} } {\partial {\tilde{\mathbf{U}}}}}} \right. \kern-0pt} {\partial {\tilde{\mathbf{U}}}}}$ and ${{\partial \varvec{q}_{{\tilde{p}}}^{\text{int}} } \mathord{\left/ {\vphantom {{\partial \varvec{q}_{{\tilde{p}}}^{\text{int}} } {\partial {\tilde{\mathbf{U}}}}}} \right. \kern-0pt} {\partial {\tilde{\mathbf{U}}}}}$, are approximated by

$$\frac{{\partial \varvec{q}_{p}^{\text{intf}} }}{{\partial {\tilde{\mathbf{U}}}}} \simeq - \frac{\theta }{\Delta t}\left( {{\mathbf{Q}}_{{\tilde{u}p}}^{\text{intf}} } \right)^{\text{T}} ,\quad \frac{{\partial \varvec{q}_{{\tilde{p}}}^{\text{intf}} }}{{\partial {\tilde{\mathbf{U}}}}} \simeq - \frac{\theta }{\Delta t}\left( {{\mathbf{Q}}_{{\tilde{u}\tilde{p}}}^{\text{intf}} } \right)^{\text{T}} .$$

(50)

The partial derivatives of the internal flux vector $\varvec{q}^{\text{intf}}$ with respect to ${\mathbf{P}}$ and ${\tilde{\mathbf{P}}}$ are computed as

$$\begin{aligned} \frac{{\partial \varvec{q}_{p}^{\text{intf}} }}{{\partial {\mathbf{P}}}} = {\mathbf{H}}_{pp}^{\text{intf}} + \frac{1}{\theta \Delta t}{\mathbf{S}}_{pp}^{\text{intf}} ,\quad \frac{{\partial \varvec{q}_{p}^{\text{intf}} }}{{\partial {\tilde{\mathbf{P}}}}} = {\mathbf{H}}_{{p\tilde{p}}}^{\text{intf}} + \frac{1}{\theta \Delta t}{\mathbf{S}}_{{p\tilde{p}}}^{\text{intf}} , \hfill \\ \frac{{\partial \varvec{q}_{{\tilde{p}}}^{\text{intf}} }}{{\partial {\mathbf{P}}}} = {\mathbf{H}}_{{\tilde{p}p}}^{\text{intf}} + \frac{1}{\theta \Delta t}{\mathbf{S}}_{{\tilde{p}p}}^{\text{intf}} ,\quad \frac{{\partial \varvec{q}_{{\tilde{p}}}^{\text{intf}} }}{{\partial {\tilde{\mathbf{P}}}}} = {\mathbf{H}}_{{\tilde{p}\tilde{p}}}^{\text{intf}} + \frac{1}{\theta \Delta t}{\mathbf{S}}_{{\tilde{p}\tilde{p}}}^{\text{intf}} , \hfill \\ \end{aligned}$$

(51)

in which

$${\mathbf{H}}_{\delta \gamma }^{\text{intf}} = - \int_{{\Gamma_{d} }} {(\nabla {\mathbf{N}}_{p}^{ \, \delta } )^{\text{T}} k_{d} {\mathbf{\mathcal{M}}}_{d} \, (\nabla {\mathbf{N}}_{p}^{ \, \gamma } ) \, w{\text{d}}\Gamma }$$

(52)

$${\mathbf{S}}_{\delta \gamma }^{\text{intf}} = - \int_{{\Gamma_{d} }} {({\mathbf{N}}_{p}^{ \, \delta } )^{\text{T}} \frac{{(1 - S_{c} )}}{{K_{f} }}{\mathbf{N}}_{p}^{ \, \gamma } w\,{\text{d}}\Gamma }$$

where $(\delta ,\gamma ) \in \{ {\text{std , }}\,\text{ridge}\} \equiv \{ p,\,\tilde{p}\}$. Final step is carried out by multiplying the first two rows of the Jacobian matrix by the scaler factor − θ/Δt. Provided that the contributions related to contact in the solid and fluid phases are symmetric, the symmetrized approximation of the Jacobian matrix ${\mathbf{J}}$ is expressed as

$${\mathbf{J}} \simeq \left[ {\begin{array}{*{20}c} { - \frac{\theta }{\Delta t}({\mathbf{K}}_{uu} )} \hfill & { - \frac{\theta }{\Delta t}({\mathbf{K}}_{{u\tilde{u}}} )} \hfill \\ { - \frac{\theta }{\Delta t}({\mathbf{K}}_{{\tilde{u}u}} )} \hfill & { - \frac{\theta }{\Delta t}({\mathbf{K}}_{{\tilde{u}\tilde{u}}} + {\mathbf{K}}^{\text{cont}} + {\mathbf{K}}^{\text{cohs}} )} \hfill \\ {\frac{\theta }{\Delta t}({\mathbf{Q}}_{up}^{\rm T} )} \hfill & {\frac{\theta }{\Delta t}({\mathbf{Q}}_{{\tilde{u}p}}^{\rm T} + {\mathbf{Q}}_{{\tilde{u}p}}^{\text{intf}} )} \hfill \\ {\frac{\theta }{\Delta t}({\mathbf{Q}}_{{u\tilde{p}}}^{\rm T} )} \hfill & {\frac{\theta }{\Delta t}({\mathbf{Q}}_{{\tilde{u}\tilde{p}}}^{\rm T} + {\mathbf{Q}}_{{\tilde{u}\tilde{p}}}^{\text{intf}} )} \hfill \\ \end{array} \quad \mathop {\left. {\begin{array}{*{20}c} {\frac{\theta }{\Delta t}({\mathbf{Q}}_{up} )\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,} & {\frac{\theta }{\Delta t}({\mathbf{Q}}_{{u\tilde{p}}} )\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,} \\ {\frac{\theta }{\Delta t}({\mathbf{Q}}_{{\tilde{u}p}} + {\mathbf{Q}}_{{\tilde{u}p}}^{\text{intf}} )\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,} & {\frac{\theta }{\Delta t}({\mathbf{Q}}_{{\tilde{u}\tilde{p}}} + {\mathbf{Q}}_{{\tilde{u}\tilde{p}}}^{\text{intf}} )\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,} \\ {({\mathbf{H}}_{pp} + {\mathbf{H}}_{pp}^{\text{intf}} ) + \frac{1}{{\bar{\theta }\Delta t}}({\mathbf{S}}_{pp} + {\mathbf{S}}_{pp}^{\text{intf}} )} & {({\mathbf{H}}_{{p\tilde{p}}} + {\mathbf{H}}_{{p\tilde{p}}}^{\text{intf}} ) + \frac{1}{{\bar{\theta }\Delta t}}({\mathbf{S}}_{{p\tilde{p}}} )\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,} \\ {({\mathbf{H}}_{{\tilde{p}p}} + {\mathbf{H}}_{{\tilde{p}p}}^{\text{intf}} ) + \frac{1}{{\bar{\theta }\Delta t}}({\mathbf{S}}_{pp} + {\mathbf{S}}_{pp}^{\text{intf}} )} & {\,\,({\mathbf{H}}_{{\tilde{p}\tilde{p}}} + {\mathbf{H}}_{{\tilde{p}\tilde{p}}}^{\text{intf}} ) + \frac{1}{{\bar{\theta }\Delta t}}({\mathbf{S}}_{{\tilde{p}\tilde{p}}} + {\mathbf{S}}_{{\tilde{p}\tilde{p}}}^{\text{intf}} ) + {\mathbf{K}}_{\text{fluid}}^{\text{cont}} } \\ \end{array} } \right].}\limits^{{}} } \right.$$

(53)