The practice of principles: Planck’s vision of a relativistic general dynamics

Abstract

Planck’s pioneering contributions to special relativity have received less consideration than one might expect in the historiography and philosophy of physics. Although they are celebrated in isolation, they are mostly not understood as integral to an overarching project. This paper aims (a) to provide a historically accurate overview of Planck’s contributions to the early history of relativity that is reasonably accessible to today’s reader, (b) to demonstrate how these contributions can be presented against the background of Planck’s ‘Helmholtzian’ vision of relativistic general dynamics based on the principle of relativity and principle of least action, and (c) to argue that Planck’s general dynamics serves as an illuminating example of the use of ‘principles’ in physics.

1 Introduction

As the well-worn quip goes, Max Planck made two significant discoveries during his lifetime: the quantum of energy and Albert Einstein (qtd. in Miller 1981, xxiv). Indeed, Planck was one of the first to recognize the profound significance of Einstein’s 1905 Annalen paper on the electrodynamics of moving systems and contributed more than any other to its promotion and early development.¹ However, while Planck’s introduction of ‘quantum discontinuity’ gave rise to an enormous debate among historians (see Darrigol 2008), his contributions to the early history of special relativity can be counted on one hand. In a path-breaking paper, Stanley Goldberg (1976) shows that Planck’s work on relativity should be understood against the ‘philosophical’ background of Planck’s search for a unified world picture. A more technical account of how relativity was supposed to bring this unification project to completion was provided only nearly 2 decades later in Chuang Liu’s (1991) dissertation. However, Liu focuses on the history of relativistic thermodynamics (Liu 1992) rather than providing a comprehensive account of Planck’s contribution to relativity theory.² Such an account has been provided more recently by Hubert Goenner (2010) in a paper for a volume celebrating Planck’s 150th birthday. Nevertheless, Goenner does not explore the philosophical underpinning of Planck’s interest in relativity. In the same volume, Michael Stöltzner (2010) emphasizes the significance of the principle of least action in Planck’s epistemology, but he does not address its role in Planck’s relativistic work.

To the best of my knowledge, there is otherwise a dearth of scholarly literature on this topic. Even authoritative general histories of relativity only mention Planck in passing (eg., Miller 1981; Darrigol 2022). This is surprising, as not only was a significant portion of Planck’s scientific production after 1905 dedicated to relativity, but it was also the topic on which most of his doctoral students worked during the same period. The reason for this oversight is, fundamentally, a failure to see the forest through the trees. Planck’s contributions to relativity are celebrated in isolation: the introduction of the concept of relativistic momentum, the first treatment of thermodynamics in a relativistic framework, and the establishment of the universal relationship between energy flow and momentum density. However, they are mostly not understood as integral to an overarching project.

This paper aims to address what I perceive as a gap in the historical–philosophical literature. It examines Planck’s work on relativity between 1905 and 1910, contextualizing it within Planck’s vision of a general dynamics, the grand unification of the three classical physics fields—mechanics, thermodynamics, and electrodynamics—under a single principle, the principle of least action. Section 1 discusses Planck’s treatment of the relativistic dynamics of point particles and its empirical verification.³ Section 2 explores Planck’s dynamics of extended thermo-mechanical systems. Finally, Sect. 3 delves into Planck’s articulation of the philosophical background of his general dynamics. Through this analysis, the paper aims to achieve the following objectives: (a) to provide a historically accurate overview of Planck’s contributions to special relativity, (b) to demonstrate how these contributions can be properly understood within the context of Planck’s project of a relativistic general dynamics, and (c) to illustrate how general dynamics serves as a perspicuous example of the use of ‘principles’ in physics.

Suman Seth (2010) has effectively portrayed Planck’s foundational style of doing physics as the ‘practice of principles,’ as opposed to Arnold Sommerfeld’s shut-up-and-calculate ‘physics of problems’ (Seth 2010, ch. 4). Planck was the kind of physicist who does not simply try to guess various particular mathematical formulas that fit the phenomena but aims to uncover the fundamental, general principles that lurk behind all those apparently disparate equations. These principles, possibly converging into one fundamental principle, encapsulate what Planck referred to as the ‘unity of the physical world picture’. Seth primarily focuses on Planck’s early contributions to thermodynamics, in which his methodological stance is rooted. This paper suggests that Planck’s work on relativity offers another, possibly even more illuminating example of his ‘practice of principles’.

In the legacy of his Berlin mentor Hermann von Helmholtz (1886), Planck emerged as one of the protagonists of a well-established tradition in the history of physics that, from Poincaré’s (1904) ‘physics of principles’, found its most celebrated expression in Einstein’s (1919) ‘theories of principles’ (see Giovanelli 2020). Within this tradition, Planck shows a particularly clear epistemological awareness that physical theories can be broken down into two components: (a) a general framework entailing the principles that provide for treating specific interactions–what Planck used to call a ‘general dynamics ’; (b) the various dynamical laws governing those interactions. This ‘stratification’ of physical knowledge, with its Kantian overtones (Friedman 2000, ch. 3), has garnered renewed attention in recent philosophical literature (Flores 1998; Lange 2016). The paper argues that Planck’s work on relativity serves as a historical example of how ‘principles’ are used in concrete physics practice to generate new exact laws starting from old well-established laws that are only approximately valid.

2 Planck’s relativistic dynamics of point particles

The 26-year-old Einstein (1905b) submitted his first paper on relativity at the end of June 1905; it appeared in September in volume 17 of the fourth series of the Annalen der Physik, of which Planck had been a scientific adviser since 1895 (Hoffmann 2008). According to Einstein’s sister’s recollections, Planck initiated correspondence shortly afterward (see Winteler-Einstein 1924, 23–24). At the beginning of the winter semester, he presented Einstein’s paper at the Berlin Physics Colloquium, inspiring the interests of some of his students, like Max Laue (1961b)⁴ and Kurd von Mosengeil. However, Einstein found that the initial reaction to the paper was underwhelming (see Winteler-Einstein 1924, 23–24).

It was only in November that Einstein’s relativity work was cited for the first time by Walter Kaufmann (1905) in his preliminary report of his $\beta$-rays experiments measuring the dependence of the electron mass on the velocity. Kaufmann (1906, 530f.) seemed to be the first to note that, in the final §10 of his 1905 paper, Einstein, relying solely on the principle of relativity, derived the same formula⁵ for the velocity dependence of the electron’s mass as Lorentz had obtained by introducing a model of a ‘deformable’ electron. However, Kaufmann (1906, 495) concluded that the data he had collected were not compatible with Lorentz and Einstein’s assumption that motion through the ether is undetectable. In contrast, his data set supported Max Abraham’s ‘rigid’ spherical electron and Alfred Bucherer’s ‘deformable’ electron at constant volume without allowing a choice between these two models (Kaufmann 1906, 495; see Janssen 2006).

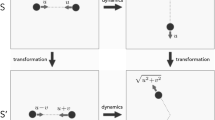
It did not look good for the relativity principle. However, Planck, backed by his weighty authority, cautioned against making hasty judgments. He intervened publicly in this debate by presenting his first paper on relativity at the meeting of the German Physical Society on March 23, 1906. Opening the paper, Planck (1906a) introduces the ‘principle of relativity’ as it was put forward recently by Lorentz and, “in a still more general version”, by Einstein (Planck 1906a, 136). Planck’s formulation of the principle goes like this. If the two reference frames (x, y, z, t) and $(x', y', z', t')$ in uniform relative translation are to be considered equivalent for the formulations of equations in both mechanics and electrodynamics, the space–time coordinates must be related by the so-called Lorentz transformations

$$\begin{aligned} \begin{aligned}x^{\prime }=\frac{c}{\sqrt{c^2-v^2}}(x-v t). \quad y^{\prime }=y, \quad z^{\prime }=z. \\ t^{\prime }=\frac{c}{\sqrt{c^2-v^2}}\left( t-\frac{v}{c^2} x\right) \,,\end{aligned} \end{aligned}$$

(1.1)

where Planck’s preferred form of the Lorentz factor is utilized

$$\begin{aligned} \frac{c}{\sqrt{c^2-v^2}}=\sqrt{\frac{c^2}{c^2-v^2}}=\frac{1}{\sqrt{1-\frac{v^2}{c^2}}}. \end{aligned}$$

Planck’s version of the principle of relativity differs from Einstein’s. Indeed, Planck incorporates the Lorentz transformations directly into the definition of the principle, without introducing the light postulate separately (see Liu 1991, 22–24). Planck conceded that the acceptability of principle of relativity “appears to have already been addressed by the latest important measurements by W. Kaufmann, and indeed in the negative sense” (Planck 1906a, 136). Still, he insists, “further investigation remains to be done” (Planck 1906a, 136). Planck regarded the results of Kaufmann’s experiments as the primary obstacle to the acceptance of relativity. However, he probably already questioned their reliability, a point he would elaborate in detail in a separate paper (Planck (1906b); see below, Sect. 1.2).

Planck alludes somewhat cryptically to another objection against the Lorentz ‘deformable’ electron raised by his former student Abraham (1904). If the principle of conservation of energy has to hold, one must attribute to the moving Lorentz electron, in addition to the electromagnetic energy of its external field, a certain internal potential energy to account for the work required for its deformation.⁶ Thus, the Lorentz electron appeared to be incompatible with a purely electromagnetic foundation of mechanics, in which the electron’s ‘inertia’ is exclusively due to the resistance of its own field.⁷ Abraham considered this to be a serious drawback of the Lorentz electron. However, Planck points out quite dismissively that one should “attach no decisive importance” to such an objection (Planck 1906a, 137). He suggests that, as Einstein did, one could treat the electron as a moving point having the properties of electric charge and inertia, ignoring the question of internal constitution and shape.⁸ In this way, the hypothesis of no internal change is implicitly assumed and the deformation works “can be included in the kinetic energy of the electron” (Planck 1906a, 137). The work of external forces is done only by the ‘translational work’ on the electron to accelerate it from rest to speed v.

The fact that Planck addresses this point might suggest that he was already aware that the clear-cut separation between ‘deformation work’ and ‘translational work’, internal and kinetic energy, was not obvious in the general case of extended systems (see below, Sect. 2.3). However, by treating the electron as a point particle, this problem could be provisionally swept under the rug. Planck concedes that, in this way, “the question of an electromagnetic explanation of inertia remains open” (Planck 1906a, 137). However, at the same time, “the advantage arises that one does not need to attribute a spherical shape or any specific form to the electron to arrive at a particular dependence of inertia on velocity” (Planck 1906a, 137).

2.1 Planck and the notion of relativistic momentum

Ultimately, Planck considers both common objections against the relativity principle as insufficient to impede further investigation. The relativity principle appears to embody a physical idea of such “simplicity and generality” that it deserves thorough examination: “and there is no better way to do this than by examining the consequences it leads to” (Planck 1906a). To evaluate the validity of the principle of relativity from its consequences, Planck addresses the task of determining the form of the equations of motion of a free point mass that must replace the usual Newtonian equations if the relativity principle must have general validity. In the Cartesian coordinate system $x,y,z,t$, Newton’s equations of motion take the form

$$\begin{aligned} m \ddot{x}=F _{x}, \quad m \ddot{y}=F _{y}, \quad m \ddot{z} = F _{z}. \end{aligned}$$

(1.2)

Planck aims to show that if the relativity principle has to hold, these equations apply only to a material point at rest but fail for the case of finite velocity q, where

$$\begin{aligned} q=\sqrt{\dot{x}^2+\dot{y}^2+\dot{z}^2}. \end{aligned}$$

(1.3)

Indeed, applying the Lorentz transformation, one obtains the mathematical expression Eq. (1.2) with respect to the coordinates $x', y',z',t'$; however, the two expressions are not identical. As a consequence, the principle of relativity is not fulfilled and the expression of the law is not acceptable (Planck 1906a, 138). If the relativity principle has to hold, Eq. (1.2) must be modified. For this purpose, Planck suggests starting from a specific case in which the connection between the components of the force in both reference systems is known. One such case is the effect of vacuum electric and magnetic fields, $\mathbf{{E}}$ and $\mathbf{{B}}$, on a mass point of mass m and charge $e$. The relationships between the components of $\mathbf{{E}}$ and $\mathbf{{B}}$ in vacuum in both reference systems were derived by Einstein (and Lorentz)

$$\begin{aligned} \begin{array}{ll}E_{x^{\prime }}^{\prime }=E_x &{} B_{x^{\prime }}^{\prime }=B_x, \\ E_{y^{\prime }}^{\prime }=\dfrac{c}{\sqrt{c^2-v^2}}\left( E_y-\dfrac{v}{c} B_z\right) &{} B_{y^{\prime }}^{\prime }=\dfrac{c}{\sqrt{c^2-v^2}}\left( E_y+\dfrac{v}{c} E_z\right) , \\ E_{z^{\prime }}^{\prime }=\dfrac{c}{\sqrt{c^2-v^2}}\left( E_z+\dfrac{v}{c} B_y\right) &{} B_{z^{\prime }}^{\prime }=\dfrac{c}{\sqrt{c^2-v^2}}\left( E_z-\dfrac{v}{c} E_y\right) \end{array}. \end{aligned}$$

(1.4)

Planck, like Einstein, supposed that a charged mass point particle is located at the origin of the coordinate system $x,y,z,t$ and moves along the x-axis with velocity components $\dot{x}=q,\dot{y},\dot{z}$ with respect to it. To find the equations of motion, Planck placed the particle at the origin of the coordinate system $x', y',z',t'$, comoving with the particle with speed q along the x-axis, with respect to which the mass point is at rest. In this coordinate system, the equations of motion take the form of Eq. (1.2), where $\mathbf{{F}} '=q \mathbf{{E}} '_{x}$

$$\begin{aligned} m\ddot{x}'=qE'_x, \quad m \ddot{y}'=qE'_y, \quad m \ddot{z}'=qE'_z, \end{aligned}$$

(1.5)

where $x', y',z',t'$ are the coordinates of the electron in the comoving coordinate system. Departing from Einstein’s derivation, Planck introduces a third coordinate system $x'', y'', z'', t''$, with the spatial axes oriented like the axis of $x', y',z',t'$. Using the transformation Eq. (1.1) for the acceleration components and Eq. (1.4) for the force components, he transformed Eq. (1.5) written in $x', y',z',t'$ the one written in $x'', y'', z'', t''$. The x-axis of the latter coordinate system is parallel the velocity q, but the system is at rest with respect to $x,y,z,t$. In this way, Planck obtained the same equations that Einstein had obtained in §10 of his relativity paper,⁹ but as functions of the variables $x'', y'', z'', t''$

$$\begin{aligned}&\frac{m \ddot{x}''}{\sqrt{1-\frac{q^{2}}{c^{2}}} ^3}=e E''_x, \end{aligned}$$

(1.6a)

$$\begin{aligned}&\frac{m \ddot{y}''}{\sqrt{1-\frac{q^{2}}{c^{2}}}}=e\left( E''_y-\frac{e}{c} B''_z\right) , \end{aligned}$$

(1.6b)

$$\begin{aligned}&\frac{m \ddot{z}''}{\sqrt{1-\frac{q^{2}}{c^{2}}}}=e\left( E''_z+\frac{e}{c} B''_y\right) . \end{aligned}$$

(1.6c)

From Eq. (1.6), one can infer that the same force acting on the same electron (due to the cube of the Lorentz factor) experiences greater resistance to the change of velocity (i.e., greater inertia) along the longitudinal axis compared to the transverse direction. Thus, if one defines the mass m as the ratio of force to acceleration, one obtains two different notions of ‘mass’. Indeed, following the terminology used at the time, Einstein (1905b) distinguished the longitudinal mass as the force/acceleration ratio when the latter is in the same direction as the line of motion, Eq. (1.6b), and the transverse mass as the force/acceleration ratio when the latter is perpendicular to the line of motion, Eqs. (1.6b) and (1.6c). Einstein could conclude that, according to the new dynamics, the mass is variable and the longitudinal mass grows more quickly with velocity than the transverse mass.

However, Planck avoids this parlance altogether and moves forward. He factorizes the cube of the Lorentz factor out of the left-hand side of Eq. (1.6a), and, after some “elementary calculations” (see appendix A), reshuffles Eq. (1.6) into the equivalent form

$$\begin{aligned} \begin{aligned} \frac{m \ddot{x}''}{\sqrt{1-\frac{q^{2}}{c^{2}}}}&=e E''_{x}-\frac{e\dot{x}''}{c^{2}} \left( \dot{x} E''_{x}+\dot{y}'' E''_{y}+\dot{z}'' E''_{z}\right) , \\ \frac{m \ddot{y}''}{\sqrt{1-\frac{q^{2}}{c^{2}}}}&= e E''_{y} + \frac{e}{c}\left( \dot{z}'' B''_{x}-\dot{x} B''_{z}\right) ,\\ \frac{m \ddot{z}''}{\sqrt{1-\frac{q^{2}}{c^{2}}}}&= e E''_{z} + \frac{e}{c}\left( \dot{x} B''_{y}-\dot{y} B''_{x}\right) . \end{aligned} \end{aligned}$$

(1.7)

These equations apply to the case in which the electron moves in the $x''$-direction with respect to $x'', y'', z'', t''$ with velocity $\dot{x}''$, where the longitudinal component, the electric field, vanishes in the last two equations. Through “a simple rotation of the coordinate axes”, (Planck 1906a, 139) returns to the original coordinate system $x,y,z,t$, and obtains equations that are valid in the general case of arbitrary orientation and between velocity and force, not only when $\dot{y}=\dot{z}=0$

$$\begin{aligned} \frac{m \ddot{x}}{\sqrt{1-\frac{q^{2}}{c^{2}}}}= e E_{x}-\frac{e\dot{x}}{c^{2}} \left( \dot{x} E_{x}+\dot{y} E_{y}+\dot{z} E_{z}\right) + \frac{e}{c}\left( \dot{y} B_{z}-\dot{z} B_{y}\right) ,\nonumber \\ \frac{m \ddot{y}}{\sqrt{1-\frac{q^{2}}{c^{2}}}}=e E_{y}-\frac{e\dot{y}}{c^{2}} \left( \dot{x} E_{x}+\dot{y} E_{y}+\dot{z} E_{z}\right) + \frac{e}{c}\left( \dot{z} B_{x}-\dot{x} B_{z}\right) ,\\ \frac{m \ddot{z}}{\sqrt{1-\frac{q^{2}}{c^{2}}}}=e E_{z}-\frac{e\dot{z}}{c^{2}} \left( \dot{x} E_{x}+\dot{y} E_{y}+\dot{z} E_{z}\right) + \frac{e}{c}\left( \dot{x} B_{y}-\dot{y} B_{x}\right) .\nonumber \end{aligned}$$

(1.8)

The equations are now symmetric to the three spatial variables. Planck simplifies them further. By scalar multiplication of Eq. (1.8) by $\dot{x}, \dot{y}, \dot{z}$ and summing, one obtains¹⁰

$$\begin{aligned} e\left( \dot{x} E_x+\dot{y} E_y+\dot{z} E_z\right) =\frac{m(\dot{x} \ddot{x}+\dot{y} \ddot{y}+\dot{z} \ddot{z})}{\sqrt{1-\frac{q^2}{c^2}}^{3}}. \end{aligned}$$

(1.9)

The product of force $e\mathbf{{E}}$ and velocity $\dot{x}, \dot{y}, \dot{z}$ is the work per unit time or ‘power’ delivered by $e\mathbf{{E}}$, which, as in classical mechanics, corresponds to the product of mass, acceleration, and velocity. Plugging Eqs. (1.9) into (1.8), one obtains

$$\begin{aligned} \begin{aligned} \frac{m\ddot{x}}{\sqrt{1-\frac{q^2}{c^2}}} +\frac{m(\dot{x} \ddot{x}+\dot{y} \ddot{y}+\dot{z} \ddot{z})}{\sqrt{1-{\frac{q^2}{c^2}}}^3} {\frac{\dot{x}}{c^{2}}} = e E_x+\frac{e}{c}\left( \dot{y} B_z-\dot{z} B_y\right) ,&\\ \frac{m\ddot{y}}{\sqrt{1-\frac{q^2}{c^2}}} +\frac{m(\dot{x} \ddot{x}+\dot{y} \ddot{y}+\dot{z} \ddot{z})}{\sqrt{1-{\frac{q^2}{c^2}}}^3} {\frac{\dot{x}}{c^{2}}} = e E_{y}+ \frac{e}{c} \left( \dot{z} B_{x}-\dot{x} B_{z}\right) , \\ \frac{m\ddot{z}}{\sqrt{1-\frac{q^2}{c^2}}} +\frac{m(\dot{x} \ddot{x}+\dot{y} \ddot{y}+\dot{z} \ddot{z})}{\sqrt{1-{\frac{q^2}{c^2}}}^3} {\frac{\dot{x}}{c^{2}}} =eE_{z}+\frac{e}{c}\left( \dot{x} B_{y}-\dot{y} B_{x}\right) . \end{aligned} \end{aligned}$$

(1.10)

It can be shown (see appendix A) that the sum of the two terms on the left-hand side corresponds (via the chain rule) to the time derivatives of the term on the left-hand side of the following equation:

$$\begin{aligned} \begin{aligned} \frac{{\textrm{d}}}{{\textrm{d}}t}\frac{m \dot{x}}{\sqrt{1-\frac{q^{2}}{c^{2}}}}&= e E_x+\frac{e}{c}\left( \dot{y} B_z-\dot{z} B_y\right) , \\ \frac{{\textrm{d}}}{{\textrm{d}}t}\frac{m \dot{y}}{\sqrt{1-\frac{q^{2}}{c^{2}}}}&= e E_{y}+ \frac{e}{c} \left( \dot{z} B_{x}-\dot{x} B_{z}\right) , \\ \frac{{\textrm{d}}}{{\textrm{d}}t}\frac{m \dot{z}}{\sqrt{1-\frac{q^{2}}{c^{2}}}}&= eE_{z}+\frac{e}{c}\left( \dot{x} B_{y}-\dot{y} B_{x}\right) . \end{aligned} \end{aligned}$$

(1.11)

As can be seen, the components of the ponderomotive force exerted by an electromagnetic field (that is, the Lorentz force) on a charged particle are now defined as the time derivatives of the components of the quantity on the left side of Eq. (1.11). The latter then plays the same role as the momentum in classical mechanics. “These equations,” Planck concluded his derivation, “contain the solution to the problem”: “they form that generalization of Newton’s equations of motion”, which is required by the principle of relativity (Planck 1906a, 137; my emphasis). Still, Planck considers only one force law here, namely the Lorentz force law. One might want to generalize this result to non-electromagnetic forces (see Einstein 1908, 433ff.). Possibly, Planck felt that this generalization was not justified at this stage. I speculate that it is deferred to the last part of the paper (see below, Sect. 1.2).

Nevertheless, the general methodological gist of Planck’s derivation is already worth emphasizing. Electron theorists retained the old Newtonian dynamics and introduced a suitable electron model to explain why the electron mass turns out to be variable, in contrast to the constant Newtonian mass. Planck appears to have been the first to appreciate Einstein’s different strategy. Like Einstein, Planck modified Newton’s equation of motion for charged point particles to comply with the relativity principle, thereby obtaining Eq. (1.11). No hypothesis about the structure of the electron (its nature, mass shape, and charge distribution) is required, and Eq. (1.11) applies to any charged point particle, microscopic or macroscopic. Electrons forming the so-called $\beta$-rays emitted by a radioactive material are required only to test the theory, as one needs charged particles moving at extremely high speeds (see below, Sect. 1.2).

Indeed, Einstein arrived at the same formulas for the variability of the electron mass as Lorentz on the basis of his electron model. However, for Planck, the superiority of Einstein’s approach was that he unveiled the general principle lurking behind those formulas. As is well known, Planck advanced beyond Einstein on a significant point by defining the force as the rate of change of momentum rather than the product of mass and acceleration. This simple change allows the introduction of a single ‘relativistic’ variable mass

$$\begin{aligned} \frac{m_{0}}{{\sqrt{1- \frac{v^2}{c^2}}}}\,\, \text {(where}\,m_{0}\hbox { is the so-called `rest mass')}, \end{aligned}$$

instead of two different masses—although Planck often switched back to the more common language of the two variable masses in subsequent writings. However, in hindsight, Planck’s result sets the stage for the more radical step of abolishing the very distinction between the ‘constant’ mass $m_{0}$ and the ‘variable’ mass m. The mass m in relativity is a constant independent of the velocity. Relativity introduced a novel relativistic definition of ‘momentum’, which, in contrast to classical ‘momentum’ that increases with velocity, but more rapidly than the latter, and would become infinite for $q=c$.

2.2 The derivation of the equations of motion form the principle of least action

Somewhat in passing, concluding the paper, Planck shows how the relativistic equations of motion for a free point particle can also be derived using ‘Hamilton’s principle’, i.e., the principle of least action. Planck formulates the latter in a modified form with the presence of additional terms

$$\begin{aligned} \int _{t_0}^{t_1}(\delta H+ \delta A) {\textrm{d}}t=0, \end{aligned}$$

(1.12)

where the time t coordinate, as well as the initial and final positions, remain unchanged. The integral $\int _{t_0}^{t_1}\delta H$ is called the ‘action’ W Wirkung, and the integrand H is the so-called ‘kinetic potential ’. The term ‘kinetic potential ’ goes back to von Helmholtz (1886). As we shall see, Planck’s choice of this terminology is essential to understand the conceptual framework he was working in. For the time being it suffices to say, that, in the case of particle dynamics, the kinetic potential H corresponds to the ‘Lagrangian’ L: the difference in kinetic and potential energy expressed as a function of the Cartesian coordinates $x,y,z$ indicating the particle position, and the velocities $\dot{x}, \dot{y}, \dot{z}$. $\delta A$ is the ‘virtual work’ done by the external forces $\mathbf{{F}}$ to change those coordinates

$$\begin{aligned} \delta A=F _x \delta x + F _y \delta y +F _y \delta y = \delta E. \end{aligned}$$

Planck considered Hamilton’s principle in this form, with the inclusion of external work, particularly advantageous. This formulation not only implicitly contains the equation of motion but also explicitly separates the difference between the kinetic and potential energy of the system, on the one hand, and the work of external forces, on the other. This separation allows for the inclusion of forces that are not derivable from a potential (see Witte 1906b, 5). From the principle of least action, Lagrange’s equation with external terms can be derived

$$\begin{aligned} \frac{{\textrm{d}}}{{\textrm{d}}t}\left( \frac{\partial H}{\partial \dot{x}}\right)&= F _x&\frac{{\textrm{d}}}{{\textrm{d}}t}\left( \frac{\partial H}{\partial \dot{y}}\right)&= F _y&\frac{{\textrm{d}}}{{\textrm{d}}t}\left( \frac{\partial H}{\partial \dot{z}}\right)&= F _z. \end{aligned}$$

From this set of equations, one can derive the equations of motion once one knows the form of H. For the simple case of a free particle (not inserted in a potential field), Planck introduces the kinetic potential

$$\begin{aligned} H=-m c^2 \sqrt{1-\frac{q^2}{c^2}} + \textrm{const} \end{aligned}$$

(1.13)

A derivation of the laws of motion for electrons from the Lagrangian was common in electron theoretical literature. However, Planck’s kinetic potential given in Eq. (1.13) is not the electrodynamic Lagrangian adopted by electron theorists,¹¹ but rather the Lagrangian for a point particle as in classical mechanics. Indeed, by Taylor expanding Eq. (1.13), one obtains, in the first approximation, the classical Lagrangian $L=\frac{1}{2}mq^{2}$ of a free particle with the term $-mc^{2}+\text {const}$. The presence of an additive constant in Eq. (1.13) is somewhat puzzling. In classical mechanics, the additive constant is inconsequential. However, within relativistic mechanics, the existence of any non-zero additive constant would disrupt the Lorentz invariance of the associated action.¹² At this point, Planck does not appear to be aware or interested in this issue.¹³ He proceeds to derive the components of the so-called generalized momentum $\mathbf{{G}}$ as the derivative of H, Eq. (1.13) with respect to the velocity

$$\begin{aligned} \begin{aligned} G_x&= \frac{\partial H}{\partial \dot{x}} = \frac{m \dot{x}}{{\sqrt{1- \frac{v^2}{c^2}}}},&G_y&= \frac{\partial H}{\partial \dot{y}} = \frac{m \dot{y}}{{\sqrt{1- \frac{v^2}{c^2}}}},&G_z&= \frac{\partial H}{\partial \dot{z}} = \frac{m \dot{z}}{{\sqrt{1- \frac{v^2}{c^2}}}}. \end{aligned} \end{aligned}$$

If we consider a single free particle and use rectangular coordinates, the three quantities $G_x, G_y, G_z$ are identical to the rectangular components of the relativistic momentum

$$\begin{aligned} \mathbf{{G}} = \frac{m q}{\sqrt{1-\frac{q^{2}}{c^{2}}}}. \end{aligned}$$

(1.14)

Thus, Planck’s choice of H is justified by the fact that it allows one to recover the definition of relativistic momentum introduced in the first part of the paper.¹⁴ It is worth noting that, as was common in the literature of that time, from the momentum, one can derive both transverse and longitudinal masses—although Planck intentionally avoided using this language here. If the force acts perpendicular to the velocity, it changes the direction but not the magnitude of the relativistic momentum $\mathbf{{G}}$. Thus, the transverse mass is simply the ratio between the constant momentum and the velocity. In contrast, a longitudinal force acting in the direction of motion changes the magnitude of the relativistic momentum $\mathbf{{G}}$. Using the definition of mass as the ratio between force and acceleration, one obtains two distinct variable masses with respect to velocity

(1.15)

where the cube of the Lorentz factor is the consequence of the fact that one has to take the derivative of the latter with respect to speed and apply the chain rule.

Finally, as is customary in Lagrangian mechanics, the generalized momentum can be used to express the generalized energy E

$$\begin{aligned} E _{k} =\dot{x} \frac{\partial H}{\partial \dot{x}}+\dot{y} \frac{\partial H}{\partial \dot{y}}+\dot{z} \frac{\partial H}{\partial \dot{z}}-H=\frac{m c^2}{\sqrt{1-\frac{q^2}{c^2}}}+\text {const}\end{aligned}$$

(1.16)

The quantity $E$ is referred to by Planck as lebendige Kraft, which translates to kinetic energy.¹⁵ In Newtonian mechanics, the zero point of the kinetic energy is arbitrary, and the constant term in Eq. (1.16) is typically set to 0. However, in special relativity, this choice has significant consequences. When the constant is set to $-m c^2$, Eq. (1.16) becomes identical to the expression introduced by Einstein (1905b, §10). Choosing this zero point of energy implies that as the speed becomes zero (and the kinetic energy vanishes), the particle still has a residual energy $=mc^{2}$. Planck does not address this issue at this juncture. Instead, he expresses the relativistic kinetic energy in terms of the relativistic momentum $\mathbf{{G}}$, that is, the ‘Hamiltonian function’, as follows:

$$\begin{aligned} E=m c^2 \sqrt{1+\frac{G^2}{m c^2}}+\text {const}\end{aligned}$$

(1.17)

It can be demonstrated that only by setting the constant $=0$, the usual relation between relativistic momentum and energy is recovered.¹⁶ However, Planck was, of course, not aware of the proper relativistic form of the momentum–energy relation.¹⁷ Instead, he uses Eq. (1.17) as a stepping stone to transform the equations of motion into the so-called ‘canonical’ form. In this form, the momenta $G_x, G_y, G_z$ can be treated as a new set of mechanical variables alongside $x,y,z$, and t

$$\begin{aligned} \frac{d \dot{x}}{d t}=\frac{\partial E}{\partial G_x}, \quad \frac{d G_x}{d t}=-\frac{\partial E}{\partial \dot{x}}\, \\ \frac{d \dot{y}}{d t}=\frac{\partial E}{\partial G_y}, \quad \frac{d G_y}{d t}=-\frac{\partial E}{\partial \dot{y}}, \\ \frac{d \dot{x}}{d t}=\frac{\partial E}{\partial G_z}, \quad \frac{d G_z}{d t}=-\frac{\partial E}{\partial \dot{z}}. \\ \end{aligned}$$

In this manner, Planck was able to demonstrate that the relativistic momentum $\mathbf{{G}}$, as defined in Eq. (1.14), plays the same role in relativistic mechanics as the Newtonian momentum in classical mechanics. Consequently, he could justify interpreting the time derivative of the relativistic momentum $\mathbf{{G}}$ as the general-relativistic definition of ‘force’, extending beyond the electromagnetic forces discussed in the first part of the paper.

Young Einstein did not conceal his satisfaction that, at last, his work received the attention it deserved (Einstein to Solovine, Apr. 27, 1906; CPAE, Vol. 5, Doc. 36). By introducing the notion of relativistic momentum and relying on the Lagrange–Helmholtz formalism, Planck brought Einstein’s derivation to a higher level of abstraction, thereby further freeing it from the reference to electrodynamics. The significance of this formalism becomes more pronounced in Planck’s presentation at the meeting of the Physical Division of the 78th Congress of German Natural Scientists and Physicians held in Stuttgart on September 19, 1906. Planck conducted a meta-analysis of the data collected by Kaufmann’s experiments (Potters 2019) to decide between the two most promising theories of the ‘electron’: the Abraham theory and the Lorentz-Einstein theory. Abraham theory, which assumes the electron as a charged rigid sphere, was labeled the Kugeltheorie (sphere theory) by Planck. On the other hand, the Lorentz–Einstein approach, characterized by the assumption that absolute motion cannot be detected, was labeled Relativtheorie.¹⁸

By presenting the equations of motion in the Lagrange–Helmholtz formalism, Planck is able to compare different electron theories with the empirical data within a common mathematical framework. Initially, Planck derives the observed values of fundamental quantities, such as the magnitude of the electron’s momentum, from observed data (the magnetic and electric deflections) independently of the specific form of the kinetic potential (Planck 1906b, §§1-4). Subsequently, Planck computes the theoretical values of the corresponding quantities using Abraham’s and Lorentz–Einstein’s kinetic potentials (Planck 1906b, §5). Planck gave great importance to the Lagrange–Helmholtz formalism. During the ensuing discussion, Planck indicated that he did not take into account Bucherer’s deformable ‘electron’ model, because the theory’s equations were not presented “in the Lagrangian form”, and Bucherer had not determined “the value of the Lagrangian function H” (Planck 1906b, 760) for his theory.

Finally, Planck compares the theoretical results with the observed data (Planck 1906b, §6). The intricacies of Planck’s ‘meta-analysis’ of Kaufmann’s data have been discussed elsewhere (Cushing 1981, sec. IV; Zahar 1989, sec. 6.3). Planck, albeit reluctantly, concedes that the comparison of theoretical values with observed ones favors the spherical theory over the theory of relativity (Planck 1906b, 757). However, he also highlights that, according to the observed values, electrons may be moving with a velocity higher than that of light, contradicting both theories (Planck 1906b, 757f.). Thus, during the discussion after his lecture, Planck concluded that “the mere fact that the deviations of one theory are smaller does not imply that it is preferable” (Planck 1906b, 760). However, the question was not purely empirical. Planck agreed with his former assistant Abraham that the choice between Kugeltheorie and Relativtheorie is ultimately a choice between the electromagnetic worldview and the principle of relativity. Indeed, only Abraham’s ‘rigid electron’ is entirely electromagnetic; Lorentz’s ‘deformable electron’, as explicitly emphasized by Bucherer, requires additional internal potential energy to be stable (Planck 1906b, 760).

However, as we have seen, the question of the nature of the electron’s mass was fundamentally irrelevant for Planck. He had lost faith in the mechanical worldview (Witte 1906b). However, he considered the electromagnetic approach to be excessively speculative, involving unjustified hypotheses about the electron’s structure. Such assumptions, he believed, could be avoided by adopting the more ‘sober’ relativistic approach, based on the validity of a general principle. In contrast, Arnold Sommerfeld did not share the “pessimistic viewpoint of Mr. Planck” (Planck 1906b, 761). The 38-year-old Sommerfeld famously remarked that he expected physicists under 40 years of age to prefer the “electrodynamics postulate,” while those over 40 the, “mechanical-relativistic postulate” (Planck 1906b, 761).

This tongue-in-cheek comment vividly reflects the hopes generated by the electrodynamic worldview in late nineteenth-century physics. On the contrary, Relativtheorie ’s silence on the nature of the electron’s inertia seemed to be a concession to the old-fashioned mechanical worldview (Planck 1906b, 761). However, for Planck, framing the problem in this way was misleading. As one of his students noted around the same time, Planck did not believe in reducing all physics to mechanics or electrodynamics. His ambition was to establish “both mechanics and electrodynamics on a common ground” that could not be characterized as either purely mechanical or purely electrodynamic (Witte 1906a, 784; my emphasis).

3 Planck’s relativistic dynamics of extended systems

To appreciate the motivation behind Planck’s interest in relativity, one must understand how Planck conceived this ‘common ground’. A valuable source is Planck’s concise summary of Hermann von Helmholtz’s impact on theoretical physics for the Allgemeine Deutsche Biographie—the authoritative reference on prominent figures in German history—that was published around the same time. In Planck’s assessment, Helmholtz’s remarkable ability to engage across various branches of physics stemmed from his reliance on ‘general principles’ that appeared to be valid in all those diverse domains. The principle of conservation of energy, which Helmholtz contributed to establishing (von Helmholtz 1847), dominated the first part of his career. After his later works on thermodynamics (von Helmholtz 1884), it was replaced by principle of least action as the supreme principle in physics, encompassing the energy principle as a special case. Helmholtz’s merit was in demonstrating that, similar to the principle of conservation of energy, the principle of least action, if formulated in a sufficiently general manner, had significance beyond the motion of mechanical processes to which it was originally applied; it holds, “for all physical processes of which we have precise cognition”, including electrodynamic and reversible thermal processes (Planck 1906b, 472). Helmholtz generalized the notion of ‘Lagrangian’ encountered in mechanics to that of ‘kinetic potential ’.¹⁹ Once the ‘kinetic potential ’ H for a particular class of systems is known, Eq. (1.12) allows the unambiguous determination of its dynamical laws (von Helmholtz 1886).

Concluding his brief overview, Planck stated that, through these investigations, “Helmholtz has paved the way for a unified understanding of all natural forces. The realization of his ideas must be brought forth by the future” (Planck 1906b, 472; my emphasis). Planck’s ambition was, indeed, to bring ‘Helmholtz’s ideas’ to completion. At the turn of the century, Planck applied his expertise in thermodynamics to the case of heat radiation. His goal was to explore the relations between thermodynamics and electrodynamics, in the hope of building a bridge to the final unification of the three branches of mechanics, thermodynamics, and electrodynamics under the banner of a single principle, the principle of least action. However, around 1904/1905, Planck probably realized that recent research on moving thermal radiation (Hasenöhrl 1904, 1905) suggested that the principle of least action was only valid in the low-speed approximation.

This realization explains Planck’s early engagement with relativity. In contrast to most of his fellow physicists, Planck’s interest in Einstein’s Annlen paper was not motivated by the problem of the structure of the electron. Instead, it was driven by the assumption that the principle of relativity would allow him to reestablish the exact validity of the principle of least action. Planck felt compelled to address Kaufmann’s results only to remove what he perceived as the only serious obstacle to the acceptance of the exact validity of the principle of relativity (Cushing 1981, 1146). Following the meeting in Stuttgart in 1906, Planck was optimistic: “[c]oncerning the relativity principle, I really see no difficulty yet” (Planck to Wien, Oct. 15, 1906; WN). The contours of Planck’s plan start to become more defined as we follow his research path in the ensuing months.

3.1 The Von Mosengeil–Planck paper

In January 1907, Planck wrote to Wien, the new main editor of the Annalen der Physik, urging him to agree to publish an excerpt of the dissertation of one of Planck’s students, Kurd von Mosengeil (1906), who had tragically died in an Alps accident in September (Planck to Wien, Jan. 26, 1907; WN). Following Planck’s suggestion, von Mosengeil addressed the problem of stationary radiation trapped inside a moving hollow enclosure or cavity (Hohlraum), a topic first explored by Fritz Hasenöehrl (1904; 1905).²⁰ On February of 1907, Planck, with the help of his assistant Laue, finished preparing von Mosengeil ’s work for publication in the Annalen der Physik (Planck to Wien, Feb. 1, 1907; Planck to Wien, Feb. 4, 1907; WN). It was published in the spring of the same year with the disclaimer “abridged and with a correction by M. Planck”.

Von Mosengeil, following Planck’s lead, introduces an arbitrarily shaped cavity of volume V enclosed by perfectly reflecting walls, so that radiation cannot escape. If the walls are maintained at the same temperature T, the introduction of a small carbon particle leads to the gradual establishment of a stationary state of radiation (Planck 1906c, §51). A small opening in a large cavity of this kind acts as a perfect absorber: radiation that passes through the hole bounces around inside but has little chance of ever escaping again (Fig. 1). Thus, the hole serves as a good approximation of a ‘black body’. Due to the second principle of thermodynamics, a perfect absorber must also be a perfect emitter. Therefore, the ‘cavity radiation’ that manages to escape from the hole must be a good approximation of ‘black’ or ‘black-body radiation’ (see Planck 1906c).

Fig. 1

Schematic diagram of a radiation-filled cavity; adapted from Mosengeil 1907, fig. 1

If the cavity is at rest in the ether system, in which the velocity of light is c independent of directions, all rays, regardless of their direction of propagation, have the same specific intensity K(0) or brightness. The total energy density $w =\frac{E}{V}$ (the energy per unit volume)²¹ of radiation at a point can be calculated by integrating the contributions of rays emitted in all directions within a cone centered at that point, with solid angle ${\textrm{d}}\Omega = \sin \vartheta {\textrm{d}}\vartheta {\textrm{d}}\varphi$ expressed in spherical coordinates $\vartheta , \varphi$. Since cavity radiation is uniform, integrating gives

$$\begin{aligned} w =\int _{\vartheta =0}^\pi \int _{\varphi =0}^{2 \pi } \frac{1}{c} K(0) \sin \vartheta {\textrm{d}}\vartheta {\textrm{d}}\varphi =\frac{4 \pi }{c} K(0). \end{aligned}$$

(2.1)

An important result of nineteenth-century physics was that, if the second principle of thermodynamics holds, the total energy density $w$ of black body radiation must be a universal function of its temperature (Kirchhoff’s law)

$$\begin{aligned} \frac{4\pi }{c} K(0) = w (T), \end{aligned}$$

independent of the material composition and shape of the cavity (Planck 1906c, ch. I.2). By further assuming that heat radiation is electromagnetic, it could be proven (Stefan–Boltzmann law) that this function has the following form:

$$\begin{aligned} w =\frac{4\pi }{c} K(0) = aT^4\, \quad \quad p= \frac{4\pi }{3c} K(0) = \frac{1}{3}aT^4, \end{aligned}$$

where p is the mechanical pressure excerpts by electromagnetic radiation against the walls of the cavity (Planck 1906c, ch. II.2). The factor $\frac{1}{3}$ is the consequence of the isotropy of hohlraum radiation at rest.²²

A new set of problems emerged when considering the case of cavity radiation in motion (Hasenöhrl 1904, 1905) at a certain speed q. Then, rays that form an acute angle $\vartheta$ with the direction of velocity become more intense. By the Doppler principle, von Mosengeil determines the dependence of the specific intensity K on velocity and direction $({\vartheta }, {q})$

$$\begin{aligned} K(\vartheta , q)=K(0) \frac{\left( 1-\frac{q^{2}}{c^{2}}\right) ^{\frac{8}{3}}}{\left( 1-\frac{v}{c} \cos \vartheta \right) ^4}, \end{aligned}$$

(2.2)

where $\vartheta$ is the angle with the direction of velocity q. Since K is not uniform, the radiation pressure p against the reflecting walls becomes anisotropic (Mosengeil 1907, 870). Due to the resistance of radiation, a greater force will be required to set the cavity into motion than in the absence of radiation. Matter-free, confined electromagnetic radiation behaves as if it had an ‘apparent mass’ that increases with velocity, so that the momentum of radiation is not proportional to the velocity. Bearing in mind Eq. (2.2), von Mosengeil derives the following expressions for the dependence of the energy density $w$ and momentum density $\mathbf{{g}}$,²³ and pressure of radiation on direction and velocity

$$\begin{aligned} w _{q}&= \int _{\vartheta =0}^\pi \int _{\varphi =0}^{2\pi } \frac{1}{c} K(\vartheta , q) \sin \vartheta {\textrm{d}}\vartheta {\textrm{d}}\varphi , \\ g _{q}&= \int _{\vartheta =0}^\pi \int _{\varphi =0}^{2\pi } \frac{1}{c^{2}} K(\vartheta , q) \sin \vartheta {\textrm{d}}\vartheta {\textrm{d}}\varphi \cos \vartheta ,\\ p_{q}q&= \int _{\vartheta =0}^\pi \int _{\varphi =0}^{2\pi } \frac{1}{c} K(\vartheta , q) \sin \vartheta \textrm{d} \vartheta \textrm{d} \varphi (\cos \vartheta -q)\, \end{aligned}$$

where the extra factor of $\cos \vartheta$ specifies the normal component of momentum. Performing the integration and multiplying by the cavity’s volume V, he obtains the total momentum $\mathbf{{G}}$ and energy $E$ of moving hohlraum radiation

$$\begin{aligned} E _{q}&= \frac{4 \pi }{c} K(\vartheta , q) \cdot \frac{1+\frac{1}{3} \frac{q^{2}}{c^{2}}}{\left( 1-\frac{q^{2}}{c^{2}}\right) ^3} \cdot V = aT^{4}V \frac{1+\frac{1}{3} \frac{q^{2}}{c^{2}}}{\left( 1-\frac{q^{2}}{c^{2}}\right) ^3}\, \end{aligned}$$

(2.3a)

$$\begin{aligned} G _{q}&= \frac{4}{3}\frac{ 4\pi }{ c} K(\vartheta , q) \cdot \frac{\frac{q}{c}}{c\left( 1-\frac{q^{2}}{c^{2}}\right) ^3} \cdot V = \frac{4}{3} \frac{aT^{4}V}{\left( 1-\frac{q^{2}}{c^{2}}\right) ^3} \frac{q}{c^{2}} , \end{aligned}$$

(2.3b)

$$\begin{aligned} p _{q}&= \frac{1}{3} \frac{4 \pi }{ c} K(\vartheta , q) \cdot \frac{1}{\left( 1-\frac{q^{2}}{c^{2}}\right) ^2} = \frac{1}{3} \frac{T^{4}}{\left( 1-\frac{q^{2}}{c^{2}}\right) ^2}. \end{aligned}$$

(2.3c)

The electromagnetic momentum is equal to zero when the cavity is at rest; if the cavity moves slowly (compared to c), the momentum takes the form

$$\begin{aligned} G _{q} =\frac{4}{3} \frac{4\pi }{c}K(0)q, \quad \text {thus} \quad m = \frac{G}{q} = \frac{4}{3} \frac{4\pi }{c}K(0)=\frac{4}{3}aT^{4}V. \end{aligned}$$

(2.4)

Hence, as already Hasenöhrl (1905) showed, the apparent mass of cavity radiation at rest is $\frac{4}{3}$ its energy, just like the mass of the purely electromagnetic spherical electron.²⁴ Cavity radiation at constant volume V behaves like a sort of massive rigid body; however, since its mass depends temperature T it does not behave as classical rigid body even not in first approximation.

Von Mosengeil derived these results through brute-force calculations based on the well-known properties of radiation, without explicitly using the principle of relativity. However, in the last §10 of the paper, Mosengeil (1907) shows how the same dependence of radiation momentum and energy on velocity could be obtained using Einstein’s transformation rules for radiation pressure exerted on perfect mirrors (Einstein 1905b, §8). This agreement is not surprising, since electrodynamic radiation is, so to speak, ‘already relativistic’. Thus, von Mosengeil could maintain an agnostic position on the universal validity of the relativity principle (Mosengeil 1907, 904). However, as one might already guess, Planck believed that it was possible to take the opposite approach. Assuming the universal validity of the relativity principle, von Mosengeil ’s result emerges as a specific instance of a relation that holds for any mechanical-thermal system. Once again, according to his general methodological attitude, Planck was not satisfied with von Mosengeil ’s formula but searched for the principle hidden behind it. The ‘physics of problems’ had to evolve into a ‘physics of principles’.

3.2 The impasse of the project of a relativistic dynamics

Von Mosengeil ’s paper appeared in the Annalen der Physik on May 2, 1907. In the following months, Planck was actively “working on the Lorentz–Einstein relativity theory” (Planck to Wien, May 24, 1907; WN). He compiled the results of his research in a paper presented to the Prussian Academy on June 13 (Planck 1907b). Planck’s motivation for his interest in the problem of moving cavity radiation is outlined in the opening paragraphs. Usually, as Planck pointed out, the energy of a moving ponderable system is conceived as additively composed of two components:

• the kinetic energy, which varies solely with the velocity q of the system as a whole (regardless of its internal state);

• the internal energy, which (regardless of the speed) only depends on the internal state (density, temperature, chemical composition etc.).

However, the case of moving cavity radiation shows that this decomposition of the total energy is generally no longer permissible.

In von Mosengeil ’s formula for the energy of moving radiation, Eq. (2.3a), the decomposition into kinetic energy dependent only on velocity q and internal energy dependent only on the parameters T and V is no longer possible. This implication becomes significant when one considers that any massive body contains within its bounds a certain finite amount of energy in the form of radiant heat. Planck conceded that in most systems, other forms of energy will be predominant with respect to radiation energy. However, the latter is always present. Thus, a separation between internal and translational energy is stricto sensu never possible in a moving system (Planck 1907b, 542f.). A change in T and V would affect the kinetic energy of the body, and, vice versa, a change in the kinetic energy might lead to a change in T and V. This result was particularly concerning for Planck’s Helmholtzian research program.

As we have mentioned, Planck aimed to develop what he called a ‘general dynamics ’ in which the equations of mechanics and equilibrium thermodynamics were derived from the principle of least action. However, the application of the principle of least action requires the separation of the internal energy from the translational energy of the system as a whole. For example, a possible derivation of thermo-mechanical dynamics uses V and S as generalized coordinates; in this case, the internal energy E plays the role of a thermodynamic potential.²⁵ That is, for a system at rest, the kinetic potential takes the form $H = -E$. In classical physics, the value of $H'$ for the system in motion can be calculated simply by adding the kinetic energy term: $H' = \frac{1}{2}mv^{2} - E$ where m is constant. However, in the presence of heat radiation, the separation of these two terms no longer seems feasible. When the system is in motion, the internal energy E of the moving system might differ from that when the system is at rest in an unknown manner. Thus, it is not possible to determine $H'$ simply by adding the kinetic energy term. As we shall see (see below, Sect. 2.3), Planck prefers an equivalent derivation of thermodynamics from the principle of least action in which V and T are used as generalized coordinates, and the so-called ‘Helmholtz free energy’ $F=E - TS$ plays the role of the thermodynamic potential (see Helmholtz 1902).²⁶ However, this does not affect the problem at hand, as F depends on the internal energy E.

One is forced to conclude that, since one does not know how to calculate the value of $H'$ starting from H in the general case, principle of least action loses its status as a fundamental principle. It is true that in most circumstances, the decomposition of kinetic/internal energy is still valid in the first approximation (Planck 1907b, 545). However, to develop a general dynamics, one should be able to distinguish between exactly and approximately valid statements (Planck 1907b, 545). In fact, Planck perceptively pointed out that “profound upheavals” in science mostly “originated from the discovery of almost imperceptible inaccuracies in a theory previously considered exact by general consensus” (Planck 1907b, 545). Planck, however, envisaged a possible solution, suggesting that the principle of relativity would come to the rescue:

When we consider [...] the real exact foundations of general dynamics, we find in all laws known to us, above all, the principle of least action, which, as H. von Helmholtz has proven, embraces mechanics, electrodynamics, and the two laws of thermodynamics in their application to reversible processes. [...] But the principle of least action is not enough to serve as the foundation of a complete dynamics of ponderable bodies; for it alone provides no replacement for the separation of the energy of a body into a progressive energy and an internal energy that turned out to be impossible. On the contrary, the a more primising prospect of such a replacement lies in another theorem: the principle of relativity stated by H. A. Lorentz and, in its most general form, by A. Einstein. (Planck 1907b, 545f.)

Planck concedes that the only single direct confirmation of the validity of this principle was the experiment of Michelson and Morley, which some might still not consider sufficiently compelling (Planck 1907b, 546). However, the burden of proof was on the accuser. Planck’s analysis of Kaufmann’s results showed so far “no fact is known that directly hinders attributing general and absolute accuracy to this principle” (Planck 1907b, 546). Thus, given its great heuristic power, it was legitimate to assume the exact validity of the principle of relativity from the outset and draw possible consequences from it. In particular, Planck could show how principle of relativity allows one to resolve the issue of the inseparability of the two terms in the total energy of a system and construct a general dynamics applicable to any thermo-mechanical system.

In this endeavor, Planck could use moving cavity radiation as a sort of ‘theoretical test body’, whose dynamics is fully known independently (via von Mosengeil ’s work) of the relativity principle: “a system devoid of ponderable matter and consisting only of electromagnetic radiation, obeys the basic laws of mechanics as well as the two laws of thermodynamics in such a complete way, that for all consequences drawn from it one has nothing left to wish for” (Planck 1907b, 542). Thus, Planck could use cavity radiation (a) to obtain specific results he could not achieve directly via the relativity principle alone (e.g., the transformation rules for temperature and pressure) (b) to verify the correctness of the derivations based on the relativity principle against an independent, albeit purely theoretical, standard.²⁷ The privileged role that Planck attributed to this system is consistent with his style of doing physics.

The question of the structure of the electron, on which most of the contemporary debate was focused, appeared to Planck as the result of speculative guessing. By making ultimately arbitrary hypothesis²⁸ about its form, charge distribution, and nature of its mass, one can calculate how its momentum and energy vary as a function of q and possibly compare the results with experience via Kaufmann-like experiments. In von Mosengeil ’s cavity radiation at constant volume, Planck could find an example of a moving ‘rigid body’ displaying non-classical mechanical properties: “However, there is absolutely nothing hypothetical about those properties” (Planck 1907b, 545; my emphasis). The pressure, energy, momentum etc. of cavity radiation are completely determined by known physical laws, given a few measurable parameters: velocity $q<c$, volume V, and temperature T: “The black cavity radiation in pure vacuum is, among all physical systems, the only one whose thermodynamic, electromagnetic, and mechanical properties can be specified with absolute precision, independently of the conflicts of specific theories. Its treatment, therefore, takes precedence over that of the other systems” (Planck 1907b, 546).

3.3 Principle of least action

After introducing von Mosengeil ’s dynamics of hohlraum radiation (Planck 1907b, pp. 547–549), the second part of the paper outlines a general dynamics of thermo-mechanical systems (Planck 1907b, pp. 547–549). Planck considers a system consisting of a large number of molecules n with negligible interaction. The translational motion of the system as a whole is determined by its velocity components $\dot{x}$, $\dot{y}$, and $\dot{z}$ with respect to the unprimed coordinate system $x,y,z,t$, while its internal state depends on its volume V and temperature T. Following Helmholtz’s (1886), in §2 of the paper, Planck shows how, in pre-relativistic physics, by a suitable choice of the kinetic potential H, the dynamics of such a system can be derived from the principle of least action, Eq. (1.12). As usual, the kinetic potential H is a function of the generalized coordinates. Following Helmholtz, Planck chooses as generalized coordinates volume V, temperature T, and the magnitude q of the velocity vector $\dot{x}, \dot{y}, \dot{z}$ given by Eq. (1.3). Thus, the kinetic potential takes the general form

$$\begin{aligned} H = H(q, V, T). \end{aligned}$$

Lagrange’s equations derived from the principle of least action are as follows:

$$\begin{aligned} \frac{{\textrm{d}}}{{\textrm{d}}t} \frac{\partial H}{\partial \dot{x}}=F _x, \quad \frac{{\textrm{d}}}{{\textrm{d}}t} \frac{\partial H}{\partial \dot{y}}=F _y, \quad \frac{{\textrm{d}}}{{\textrm{d}}t} \frac{\partial H}{\partial \dot{z}}=F _z, \end{aligned}$$

(2.5)

where $\mathbf{{F}}$ is the external moving force acting on the system. The so-called Maxwell relations are given by

$$\begin{aligned} \frac{\partial H}{\partial V}=p, \quad \frac{\partial H}{\partial T}=S. \end{aligned}$$

(2.6)

Planck observes that Eqs. (2.5) and (2.6) can be regarded as an implicit definition of the kinetic potential H. However, this definition is not univocal. Indeed, given a certain force $\mathbf{{F}}$, pressure p, and entropy S, the value of H is fixed only up to an additive constant (Planck 1907b, 549). As we shall see, Planck, in section §9 of the paper, shows that it is possible to set the constant equal to $=0$ (see below, Sect. 2.4.2). As customary, the generalized momentum is given by

$$\begin{aligned} \mathbf{{G}} = \frac{\partial H}{\partial q}, \end{aligned}$$

with components

$$\begin{aligned} {G}_x= \mathbf{{G}} \cdot \frac{\dot{x}}{q} = \frac{\partial H}{\partial \dot{x}}, \quad {G}_y= \mathbf{{G}} \cdot \frac{\dot{y}}{q}=\frac{\partial H}{\partial \dot{y}}, \quad {G}_z=\mathbf{{G}} \cdot \frac{\dot{z}}{q}=\frac{\partial H}{\partial \dot{z}}. \end{aligned}$$

(2.7)

The generalized total energy of the system is

$$\begin{aligned} E=q \frac{\partial H}{\partial q}+T \frac{\partial H}{\partial T}-H=\dot{x} G_x+\dot{y} G_y+\dot{z} G_z+T S-H. \end{aligned}$$

(2.8)

As is well known, the first law of thermodynamics for an infinitesimal change of state is $\delta E = Q + A$. Combining equations representing the first and second laws provides the expression for the change in entropy as $\delta S = \delta E - \frac{A}{T}$. For any reversible energy transformation in a closed system, we can substitute these into the equation to obtain that the total work done by external forces in producing a virtual change of state:

(2.9)

If the system is at rest, the translational work can be neglected, and the only way the system can do work on its surroundings is by a change in volume under pressure $p\delta V$. The application of the first principle of thermodynamics then gives us simply the virtual change in internal energy of a system: the difference between mechanical work $\delta A=p\delta V$ and the supplied heat $\delta Q=T \delta S$. If such a system is in motion, one should take into account the action of the translational work determining the virtual change in the kinetic energy of the system as a whole .

As Planck points out, for a system at constant pressure p, instead of energy E, it is useful to introduce Gibbs’ ‘thermal function at constant pressure’ R, which we now call the ‘enthalpy’²⁹ of the system

For a system at rest (neglecting the term $qG$), the enthalpy of the system is defined as the sum of its internal energy and the product of its pressure and volume

$$\begin{aligned} R=E+pV=E+A. \end{aligned}$$

(2.10)

From the first law of thermodynamics, $\delta E=\delta Q+\delta A$, one can infer that, under constant pressure, the change in enthalpy of a system is equal to the heat added $\delta R=\delta Q$. Depending on the nature of the process, additional quantities called ‘free energy’ that serve as ‘thermodynamic potentials’ can be defined. In particular at constant temperature, one can introduce ‘Helmholtz free energy’ $F=E-TS$, which is mostly used in physics; at constant temperature and under constant pressure, Gibbs’ free energy $G=R-TS$, which is mostly used in chemistry.

Helmholtz’s system of thermo-mechanics becomes complete by making explicit the implicit definition of H given by Eqs. (2.5) and (2.6). As we have mentioned, Helmholtz adopted the following form of the kinetic potential H:

(2.11)

With this type of dependence of the function H on q, Eq. (2.5) becomes the equations of ordinary mechanics and Eq. (2.6) becomes the equations of ordinary thermodynamics. Helmholtz achieved a unification of both branches of physics by demonstrating that both sets of equations can be derived from the principle of least action, Eq. (1.12), in the form

$$\begin{aligned} \int _{t_{1}}^{t_2}\left[ \delta \left( -F+\frac{1}{2}mq^{2}\right) +\delta A\right] {\textrm{d}}t=0. \end{aligned}$$

As one can see, in Helmholtz’s thermodynamics, the kinetic potential H is composed of two terms: the kinetic energy, which depends only on q, and the free energy F, which depends only on the ‘internal’ variables V and T. A change in the kinetic energy of the system does not affect the variables T, V and vice versa, a change in T, V does not affect the kinetic energy of the system, since the mass is supposed to be constant. As we have seen, Planck realized that the case of cavity radiation challenged Helmholtz’s derivation at its core (Planck 1907b, 550f.). For such a system, the decomposition between mechanical variables and the thermodynamic variables is strictly speaking not allowed. Mosengeil’s (1907) work shows that the momentum and energy of the cavity radiation depend on both the velocity q of the whole system and its internal states as determined by T, V. Thus, a strict separation of a term proportional to $q^{2}$ and a term depending only on T, V becomes impossible. As a consequence, in the general case, starting from $H=-F$ the value of the kinetic potential of the moving system $H'$ cannot be determined by simply adding the term $\frac{1}{2}mq^{2}$. This means that the laws of mechanics for a thermo-mechanical system cannot be derived from the least action principle alone.

3.4 The principle of relativity

The Planck–Helmholtz project of unifying mechanics and thermodynamics on the basis of the principle of least action seems to have reached a dead end. As we have conjectured, Planck must have perceived this issue early on by reading Hasenoehrl’s (1904) work. Therefore, he might have instructed his doctoral student von Mosengeil to work on the topic. When he read Einstein’s (1905b) relativity paper in the Annalen, Planck must have quickly realized that the principle of relativity could come to the rescue. The Lorentz transformation, Eq. (1.1), could allow establishing the dependence of the kinetic potential H on velocity q in the general case, even when the decomposition between internal and external energy is not possible: “We do not want to carry out that decomposition here; instead, we want to rely on the principle of relativity and develop its consequences for the case under consideration” (Planck 1907b, 551).

3.4.1 Transformations for T and V

For this purpose, Planck needed to derive the Lorentz transformations for the ‘internal’ variables T and V. Planck introduces a thermo-mechanical system moving with velocity q with respect to the unprimed frame. The system is brought in a reversible, adiabatic process to rest in the unprimed reference frame. To establish a general relation between the unprimed variables T and V and the primed variables $T'$ and $V'$, in §§4-7, Planck proceeds roughly as follows. Due to the Lorentz contraction, it is relatively straightforward for Planck (§5) to show that the volume $V'$ of a moving system is related to the volume V of an identical system at rest by

$$\begin{aligned} V'=V \sqrt{1-\frac{q^2}{c^2}}. \end{aligned}$$

(2.12)

A more challenging task is to derive the transformation for T. To this purpose, in §4 Planck first shows that the entropy is invariant. Planck relies on (a) a statistical argument based on the relation between the entropy of a system in a given state and the probability of that state, which is supposed to be frame-independent; (b) a more rigorous thermodynamic reduction ad absurdum (Planck 1907b, 552). The cogency these arguments cannot be addressed here (see Liu 1991, 17f.). For the scope of this paper, we readily acknowledge that

$$\begin{aligned} S'=S. \end{aligned}$$

(2.13)

Planck (1907b, 548) calculates the entropy S for the special case of cavity radiation from the relation ${\textrm{d}}S=q{\textrm{d}}G-p{\textrm{d}}V$. By borrowing von Mosengeil ’s values for pressure p and momentum $G$ as function of the energy density $w =aT^{4}$, he derives the following expression:

$$\begin{aligned} S =\frac{4}{3} \frac{aT^3 V}{\left( 1-\frac{q^{2}}{c^{2}}\right) ^{2}}. \end{aligned}$$

(2.14)

From the value of S, one can infer that in an adiabatic-isochoric process, where S and V are kept constant, the Lorentz contraction must be compensated, and accordingly, the cavity radiation must expand. Thus, as von Mosengeil argued, the temperature must decrease in the ratio $T'=T\sqrt{1-\frac{q^{2}}{c^{2}}}^{3}$. However, imposing the conditions $S'=S$ and $V'=V \cdot \sqrt{1-\frac{q^{2}}{c^{2}}}$, Planck concludes in §6 that the relationship between the temperature $T'$ of the moving system and the temperature T of an identical system at rest must be, the following:

$$\begin{aligned} T'=T \sqrt{1-\frac{q^2}{c^2}}. \end{aligned}$$

(2.15)

Thus, radiation in a moving cavity is cooler³⁰ than an identical cavity at rest. Under the same constraints, starting once again from von Mosengeil ’s value for the pressure Eq. (2.3c), Planck shows that p is invariant

$$\begin{aligned} p'=p. \end{aligned}$$

(2.16)

Planck concludes that the relations thereby obtained for the special case of cavity radiation can be generalized for a thermo-mechanical system.³¹ The condition $V=V' \cdot \sqrt{1-\frac{v^2}{c^2}}$ can be then substituted with the condition $p=\text {const.}$. Thus, for the case in which the acceleration process is adiabatic-isobaric, with S and p constant, one obtains the following relations for the primed and unprimed quantities:³²

$$\begin{aligned} \frac{V^{\prime }}{V}=\frac{T^{\prime }}{T}=\sqrt{\frac{c^2-q^2}{c^2-q^2}}, \quad p^{\prime }=p, \quad S^{\prime }=S. \end{aligned}$$

3.4.2 Transformation for H

After obtaining the transformation rules for T and V, in §9 of the paper, Planck investigates the values of the kinetic potential H at rest and $H'$ for the kinetic potential of an identical system moving with velocity q. Planck writes down Lagrange’s equations for the moving system in terms of $H'$. After some tedious calculations, he shows how the equations of thermodynamics, Eq. (2.6), can be rewritten using $T'$ and $V'$ as generalized coordinates. Planck does the same for the equations of mechanics, Eq. (2.5), relying on the already known case of the Lorentz force. Planck finds that the difference between H and $H'$ reduces to

$$\begin{aligned} H^{\prime } \sqrt{\frac{c^2-q^2}{c^2-q^{\prime 2}}}=H+\text {const}\end{aligned}$$

Planck has now realized that the presence of the additive constant is an issue (see above, Sect. 1.2) and provides a quite cumbersome argument to get rid of it. He shows that, in the case of a system moving in the x direction, the constant does not depend on V, T, nor on $\dot{y}$ and $\dot{z}$. However, it can still depend on $\dot{x}$. Given the Lorentz transformations for $\dot{x}$, Planck concludes that the additive constant must be a function of the form

$$\begin{aligned} H^{\prime } \sqrt{\frac{c^2-q^2}{c^2-q^{\prime 2}}}=H+f\left( \frac{c^2-q^2}{c^2-q'^2}\right) . \end{aligned}$$

Planck argues that, since H and $H'$ depend only on q, V, T, and V, T are related to $V',T'$ by Eqs. (2.12) and (2.15), the difference depends only q, and it ultimately simplifies to

$$\begin{aligned} \frac{H^{\prime }}{\sqrt{c^2-q^2}}-\frac{H}{\sqrt{c^2-q^2}} = \frac{C}{\sqrt{c^2-q^{\prime 2}}}-\frac{C}{\sqrt{c^2-q^2}}. \end{aligned}$$

This allows Planck to write the relation between $H^{\prime }$ and H in the Lorentz-invariant form

$$\begin{aligned} \frac{H^{\prime }-C}{\sqrt{c^2-q^2}}=\frac{H-C}{\sqrt{c^2-q^{\prime 2}}}, \end{aligned}$$

(2.17)

where C is a pure constant that does not depend on any other variables of the system. H and $H-C$ satisfy the same equations Eqs. (2.6) and (2.5). Thus, writing both sets of equations in terms of H or $H-C$ makes no difference. Planck concludes that, setting $C=0$, “represents no physical limitation, but a useful supplement to the definition of the kinetic potential” (Planck 1907b, 558), which is not univocally determined by Eqs. (2.6) and (2.5). Thus, one can write

$$\begin{aligned} \frac{H^{\prime }}{\sqrt{c^2-q^{\prime 2}}}=\frac{H}{\sqrt{c^2-q^2}}. \end{aligned}$$

(2.18)

In this way, Planck suppresses the constant which, in his 1906 paper, prevented the action from being Lorentz invariant.³³ In the subsequent section §10, Planck explicitly emphasized the importance of searching for such invariants, and that the differential expression $Hdt=H'dt'$ is among them.³⁴ As a consequence, the value for the time integral, which is characteristic for the principle of least action, that is the action

$$\begin{aligned} W'=\int _{t'_0}^{t'_1} \delta H^{\prime } \textrm{d}t^{\prime }=\int _{t_0}^{t_1} \delta H \textrm{d}t=W, \end{aligned}$$

is also an invariant of the Lorentz transformation, that is has the same value in the primed and unprimed coordinate systems.

However, Planck does not appear to be fully satisfied with this ‘formal’ argument and introduces a further ‘physical’ argument to justify the requirement of the invariance of W. As is well known, to account for the spectral distribution of energy in black-body radiation, Planck (1900) assumed the existence of quanta of energy $w =h \nu$. However, in his 1906 lectures on heat radiation, Planck noticed that the constant h ($6.35 \times 10^{-27}$ erg-secs) has dimension $\text {energy} \times \text {time}$, that is, it has “the same dimensions as the quantity to which the principle of least action owes its name” (Planck 1906c, 155). Thus, Planck started to speak of the elementary ‘quantum of action’ Wirkungsquantum or the ‘element of action’ Wirkungselement. If the existence of this quantum of action was accepted, this would justify the assumption of the invariance of the kinetic potential with respect to Lorentz transformations, since any discrete number of elements is obviously invariant with respect to Lorentz transformations. Thus, it would be possible to maintain that “each and every change in nature corresponds to a definite number of action elements completely independent of the choice of coordinate system” (Planck 1907b, 561).

3.5 General dynamics of moving thermo-mechanical systems

At this point, Planck has gathered all the elements to construct his general dynamics based on two fundamental principles: the principle of least action in Helmholtz’s version (with the free energy F as the thermodynamic potential) and the principle of relativity. The outline is presented in §§10-12 of the paper. Let us introduce the following notation: for the system in motion, we use $H, p, S, E, \ldots$ to denote functions of the three variables q, V, T; and for the system at rest, we use $H_0, p_0, S_0, E_0, \ldots$ to denote functions of $T_{0}$ and $V_{0}$:³⁵

$$\begin{aligned} V_0=\frac{cV}{\sqrt{q^{2}-c^{2}}} \quad \quad T_0=\frac{cT}{\sqrt{q^{2}-c^{2}}}. \end{aligned}$$

(2.19)

As we have seen, for the thermo-mechanical system, Helmholtz has shown that $H_{0}$ can be defined as the negative of the free energy $F_{0}$. The relations between the $H_{0}$ and H are then

$$\begin{aligned} H=\frac{\sqrt{c^2-q^2}}{c} H_{0}, \quad H_{0} =-F_{0}=-(E_{0}-T_{0}S_{0}). \end{aligned}$$

(2.20)

Equation (2.20) allows determining the kinetic potential H of the moving system, provided that the kinetic potential $H_0$ of the stationary system is known. Thus, this equation achieves the same outcome as the corresponding Eq. (2.11) in classical theory. However, in the relativistic context, separating the two terms—one as a function of q and the other as a function of T, V—is no longer tenable, except in the low-speed approximation. Nevertheless, if $F_{0}$ is provided as a function of $V_{0}$ and $T_{0}$, Eq. (2.20) allows for the calculation of the value of H. Once H is known, one can derive, as usual, all state variables of the moving system from Eqs. (2.5) and (2.6) by setting $H=\sqrt{1-\frac{q^{2}}{c^{2}}} F_{0}$.

In a classical setting, Helmholtz obtained both the equations of mechanics and thermodynamics from the principle of least action; in the new relativistic setting, Planck obtained these two sets of equations using two principles, the principle of least action and the principle of relativity. In both cases, one might argue that a unification of thermodynamics and mechanics is achieved within a more overarching general dynamics, since both the equations of thermodynamics and mechanics are derived from the same kinetic potential. However, there is a profound difference. Helmholtz’s unification between the mechanical and thermodynamic variables was still a juxtaposition: the variable q appears only in mechanical equations and the variables T and V only in thermodynamic equations. This is no longer the case in Planck’s general dynamics. In this case, there is a veritable coupling of both types of variables (see de Broglie 1995, 159). The ‘internal’ energy depends on the velocity, and there is no separate kinetic energy anymore.

The other fundamental quantities are obtained by taking the partial derivatives of the kinetic potential H(q, V, T) with respect to the independent variables q, T, and V. Differentiating $T_{0}$ and $V_{0}$ with respect to q and keeping, respectively, T and V constant, one obtains the following:

$$\begin{aligned} \left( \frac{\partial V_{0}}{\partial q}\right) _{V}= & {} \frac{cqV_{0}}{\sqrt{c^2-q^2}^3} \frac{{\sqrt{c^2-q^2}}}{c} =\frac{V_{0}q}{c^2-q^2}, \\ \left( \frac{\partial T_{0}}{\partial q}\right) _T= & {} \frac{cqT_{0}}{\sqrt{c^2-q^2}^3} \frac{{\sqrt{c^2-q^2}}}{c} = \frac{T_{0} q}{c^2-q^2}. \end{aligned}$$

By differentiating $H=\sqrt{1-\frac{q^{2}}{c^{2}}}F_{0}$ with respect to volume and temperature and keeping the subscript variables constant, one obtains the entropy and pressure

$$\begin{aligned} \left( \frac{\partial H}{\partial V}\right) _{q, T} = p = p_{0}, \quad \quad \left( \frac{\partial H}{\partial T}\right) _{q, V}= S=S_{0}. \end{aligned}$$

By differentiating $H=\sqrt{1-\frac{q^{2}}{c^{2}}}H_{0}(T_{0},V_{0})$ with respect to q and keeping V and T constant, Planck derives the total generalized momentum (using the product rule and the chain rule)

(2.21)

From the generalized momentum, through Eq. (2.8), one can derive the generalized energy

(2.22)

In this manner, Planck establishes for the first time the transformation equations of the total energy and momentum of a system in the presence of external pressure. From this, it further follows that:

(2.23)

The importance of this result is difficult to overstate. At constant pressure, the heat flow equals an increase in enthalpy R. Thus, according to Eq. (2.23), it turns out that the momentum of a moving system can be calculated by taking the heat energy transferred at constant pressure R and dividing by $c^{2}$. As can be seen, Eq. (2.23) makes the momentum dependent on the energy content, thus a function of q, V, and T, and eliminates the existence of a ‘constant’ rest mass (Planck 1907b, 544).

3.6 Mass and energy: adiabatic-isobaric dynamics

In the general case of a thermo-mechanical system, not only the transverse and longitudinal masses are different, but—because of the coupling between thermodynamical and mechanical variables—the dependence of the longitudinal mass on q is contingent upon the quantities that remain constant throughout the acceleration process.³⁶ If the pressure $p_{0}$ is constant (isobaric acceleration), then a change in $R_{0}$ is a measure of the supplied heat; with constant entropy $S_{0}$ (adiabatic acceleration), $R_{0}$ remains unchanged. Thus, in the case of an isobaric-adiabatic transformation (${\textrm{d}}S_{0}={\textrm{d}}p_{0}=0$), the internal state of the system remains unchanged, and the transverse and longitudinal are universal functions of velocity (see also below 2.7). However, this statement does not hold in the general case. If, instead of S, the temperature T is constant (isobaric–isothermal process), heat must be supplied to the body during acceleration to counteract the temperature decrease established by Eq. (2.15). Consequently, $R_{0}$ increases with q, and the mass increases faster with q than with constant entropy. One may consider adiabatic-isochoric, isothermal-isochoric processes, and so forth. Only at zero speed ($q = 0$), the transverse and longitudinal masses of all types converge to the same value

$$\begin{aligned} m_{0}=\frac{G}{q}=\frac{R_{0}}{c^2}=\frac{E_{0}+p_{0} V}{c^2}. \end{aligned}$$

(2.24)

This essentially brings back the rest mass $m_{0}$ to the energy of the body, because the work term $p_{0}V_{0}/c^{2}$ is usually very small (e.g., for a system at constant atmospheric pressure). Since one can provide an absolute measure of $m_{0}$ (say, with a weighing scale) Eq. (2.24), it is also possible to fix not only the difference, but also the absolute measure of the rest energy $E _{0}$. However, Planck concedes that this value cannot be measured by thermodynamic means, since an additive constant remains undetermined (Planck 1907b, 567). What could be measured in principle is the change in enthalpy $R_{0}$. Equation (2.24) implies that, by adding or subtracting heat under constant pressure ($dp_{0}=0$), the increase or decrease in mass is exactly the difference in heat divided by $c^{2}$. However, these energy changes are so small that they are unlikely to be measurable in practice, even in the context of enthalpy changes due to strong chemical reactions (Planck 1907b, 566). Thus, any body must store an enormous amount of latent energy that is not observed in heating and cooling processes (Planck 1907b, 567f.). In these processes, atoms change their arrangement and state of motion, but remain unchanged themselves. Therefore, a large proportion of the latent energy $E_{0}$ must reside within the interior of the atoms. Planck suggests that confirmation of this hypothesis might arise from heat production associated with radioactive phenomena. However, this effect is also still too small to be experimentally accessible (Planck 1907b, 568).

As revealed by a footnote, Planck (1907b, 566; fn.) is aware that Einstein (1905a) essentially drew the same conclusion by applying the relativity principle to a special radiation process—the case of a rigid body emitting two radiation pulses in opposite directions. However, he questions Einstein’s derivation (see Ives 1952, 543). According to Planck, Einstein tacitly assumed that the total energy of the rigid body he considered is simply the sum of its internal and kinetic energy. However, as Planck’s paper abundantly shows, this assumption is “permissible only as a first approximation” (Planck 1907b, 566; fn.). Planck does not elaborate on his objection further. However, he seems to have realized that Einstein took for granted that the kinetic energy of the rigid body’s center of mass depends on velocity like the relativistic kinetic energy of a particle with constant rest mass.³⁷ This assumption is natural in classical dynamics, but it is generally not true in relativistic dynamics. As a matter of fact, von Mosengeil ’s cavity radiation in adiabatic–isochoric motion provides an example of a rigid body whose kinetic energy, even at low speeds, does not reduce to a product of constant mass and variable squared velocity (Planck 1907b, 545). Planck seems to suggest that Einstein should have first proven whether and under what circumstances his assumption holds.

As far as I can see, it has not been pointed out that in the last §19 of his paper, Planck (1907b, 569f.) attempts to provide such a proof. The dynamics of a material point developed in his previous publication (Planck 1906a; see above Sect. 1), he explains, is nothing but a special case of adiabatic–isobaric dynamics. In fact, a material point moves “without external heat supply and under constant zero external pressure” (Planck 1907b, 569f.). Planck can show that the kinetic potential of any extended thermo-mechanical system in a reversible adiabatic–isobaric motion takes the kinetic potential of a point particle. Roughly, Planck’s proof goes as follows. He demonstrates that his general dynamics can be derived in an equivalent form using q, p, S instead of q, T, V as generalized coordinates. In other words, instead of an isochoric–isothermal dynamics, an adiabatic–isobaric dynamics is considered. In this case, the enthalpy $R_{0}$ serves as a thermodynamic potential, and the kinetic potential $K (S,p,q)$ takes the form:³⁸

$$\begin{aligned} K =-\sqrt{1-\frac{q^{2}}{c^{2}}} R_{0}. \end{aligned}$$

According to Eq. (2.24), $R_{0}=m_{0}c^{2}$; then, we have

$$\begin{aligned} K =-m_{0}c^{2}\sqrt{1-\frac{q^{2}}{c^{2}}}. \end{aligned}$$

(2.25)

As one can see, Eq. (2.25) is nothing, but, Eq. (1.13) without the additive constant.³⁹ Then, the adiabatic–isochoric dynamics is derived according to the usual scheme, with the differentiation of K by the independent variables q, p, S

$$\begin{aligned}&\displaystyle \left( \frac{\partial K}{\partial p}\right) _S=-V, \quad \quad \left( \frac{\partial K}{\partial S}\right) _p=-T.\nonumber \\&\displaystyle \mathbf{{G}} =\left( \frac{\partial K}{\partial q}\right) _{S,p}=-\frac{q K _0}{c \sqrt{c^2-q^2}}, \quad \quad E = q\frac{\partial K}{\partial q}-K =-\frac{K _0}{c \sqrt{c^2-q^2}}.\qquad \quad \end{aligned}$$

(2.26)

In the case of adiabatic–isobaric processes, the transformation laws for T and V with velocity have the same form as the relativistic transformation law of these quantities $T=T_{0}\sqrt{1-\frac{q^{2}}{c^{2}}}$ and $V=V_{0}\sqrt{1-\frac{q^{2}}{c^{2}}}$, and the the quantities marked with the index 0 remain constant. In particular, $R_{0}$ is independent of q, and the masses are universal functions of velocities

$$\begin{aligned} m_t=-\frac{K _0}{c\sqrt{c^2-q^2}}, \quad m_l=-\frac{K _0}{c\sqrt{c^2-q^2}^{3}}. \end{aligned}$$

If the system is at rest, $q=0$, one recovers Eq. (2.24)

$$\begin{aligned} m_{0}=\frac{-K _{0}}{c^{2}}=\frac{R_{0}}{c^{2}}=\frac{E_{0}+p_{0}V_{0}}{c^{2}}, \end{aligned}$$

where $m_{0}$ might depend on $T_{0}$ and $V_{0}$. The dynamics of a mass point that Planck had developed in his first 1906 paper (see above, 1) becomes the special case of a reversible adiabatic-isobaric process of an extended system where, however, $m_{0}$ is an absolute constant. In turn, the kinetic potential of any extended system in reversible adiabatic-isobaric motion is analogous to that of a point particle with mass $m_{0}={R}/{c^{2}}$. At constant external zero pressure, $m_{0}={E}/{c^{2}}$. In this way, Planck made an important, but ultimately rarely mentioned, step toward a general ‘proof’⁴⁰ of the energy–mass equivalence.⁴¹

3.7 The dynamics of the cavity radiation

Once the main lines of his relativistic general dynamics of thermo-mechanical systems have been established, the third part of the Planck’s paper considers its applications. In particular, in §15, he deals with the special matter-free case ($n=0$) of the hohlraum radiation in thermodynamical equilibrium. The significance of this system stems from the fact that, although it may not be physically realizable, the value of $F_{0}$ can be determined on the basis of a few macroscopic parameters without making hypothesis. As we have mentioned, on thermodynamic grounds, it can be shown that the total energy density $w = E/ V$ of radiation at thermal equilibrium within a cavity of volume V is independent of the shape and material of the cavity. It is a universal function of the temperature T of the cavity walls alone, $u=u(T)$. Maxwell electrodynamics allows one to establish a relation between the radiation pressure and the energy of radiation falling on a certain surface element in a time element (see Planck 1906c, §59). It can be shown that, in the case of isotropic radiation in a three-dimensional cavity, there is a straightforward relation between the radiation pressure and the energy density of radiation

$$\begin{aligned} p=\frac{1}{3} \frac{E}{V}=\frac{1}{3}w. \end{aligned}$$

Note that Maxwell’s radiation pressure is a special feature of the electromagnetic theory and cannot be deduced from general thermodynamic considerations alone (see Planck 1906c, ch. II.1). Together, these relations permit the direct application of thermodynamics to cavity radiation. As we have mentioned, the energy density, $w = E/ V$, depends only on the temperature, T. By relying only on the fundamental thermodynamic relation $\delta E =\delta S-P \delta V$ and integrating, one obtains the so-called Stefan–Boltzmann law. According to the latter, the radiation energy density $w$ is proportional to the fourth power of the temperature T

$$\begin{aligned} \frac{E}{V}=w = aT^4, \end{aligned}$$

where a is the Stefan–Boltzmann constant (see Planck 1906c, ch. 2). Substituting these values into the thermodynamic relation $\delta S={(\delta E+p \delta V)}/{T}$, and integrating, one can determine the energy $E$, entropy $S$, and the free energy F of the cavity radiation

$$\begin{aligned} E =a T^4 V, \quad S=\frac{4}{3} aT^3 V, \quad F = a T^4 V - T \left( \frac{4}{3} a T^3 V\right) = - \frac{1}{3} aT^4 V. \end{aligned}$$

As can be seen, the result has been obtained only using the laws of electrodynamics and thermodynamics, without resorting to any microscopic assumption (Planck 1907b, 563). Using the definition of E, S, and F, one can finally find that the kinetic potential $H_0$ for the cavity at rest using the appropriate transformation formulas for $T_{0}$ and $V_{0}$. Since, as we have seen, $H=H_{0}\sqrt{1-\frac{q^{2}}{c^{2}}}$, one can calculate H for the cavity in motion if one knows the value of $H_{0}$

(2.27)

Given Eq. (2.20), one only needs to determine the free energy $F_{0}$ of the radiation at rest to calculate the value of the kinetic potential H of the moving system. Notice that in the kinetic potential of cavity radiation, there is no separation between the internal energy of the system at rest and its kinetic energy when in motion. However, the kinetic potential of the moving cavity can be unequivocally determined. Planck has achieved his goal. All remaining thermo-mechanical properties of the cavity at arbitrary velocity—pressure p, energy $E$, and momentum $\mathbf{{G}}$, etc.—can be computed provided that $F_{0}$ is known for the cavity at rest.

As expected, Planck obtains the same results, Eqs. (2.3c), (2.3a), (2.3b) and (2.14), that von Mosengeil derived from purely electrodynamic considerations (Planck 1907b, 564). In this sense, Planck provides a sort of external confirmation of his derivation based on principle of relativity, and at the same time shows von Mosengeil ’s formulas as a special consequences of that universal principle.⁴² For $q=0$, Planck found the following values:

$$\begin{aligned} \begin{aligned} E_{0}= aT_{0}^{4}V_{0}= a \frac{c V}{\sqrt{c^2-q^2}}\ \left( \frac{c T}{\sqrt{c^2-q^2}}\right) ^4 = \frac{a c^5 T^4 V}{\left( c^2-q^2\right) ^{\frac{5}{2}}}, \\ p=p_{0}=\left( \frac{\partial {H}}{\partial V}\right) _{q, T}=\frac{1}{3} \frac{aT^4 c}{\sqrt{c^2-q^2}}\, \quad S=S_{0}=\left( \frac{\partial H}{\partial T}\right) _{q, V}=\frac{4 }{3} \frac{aT^3 Vc}{\sqrt{c^2-q^2}}. \end{aligned} \end{aligned}$$

The energy and momentum in the moving cavity at any velocity q are

$$\begin{aligned} \begin{aligned} \mathbf{{G}}&=\left( \frac{\partial H}{\partial q}\right) _{V, T}= \frac{E_{0}+p_{0}V_{0}}{c^{2}} \frac{1}{\sqrt{1-\frac{q^{2}}{c^{2}}}} = \frac{E_{0}+\frac{1}{3}E_{0}}{c^{2}} \frac{1}{\sqrt{1-\frac{q^{2}}{c^{2}}}}\\&=\frac{4}{3} aT^4 V \frac{1}{\left( 1-\frac{q^{2}}{c^{2}}\right) ^3} \frac{q}{c^{2}}, \\ E&=q \frac{\partial H}{\partial q}+T \frac{\partial H}{\partial T}-H = \frac{E_0+ p_0V_0\beta ^2}{\sqrt{\left( 1-\beta ^2\right) }} = E_0 \frac{1+\frac{1}{3} \beta ^2}{\sqrt{\left( 1-\beta ^2\right) }}= aT^4 V \frac{1+\frac{1}{3} \frac{q^2}{c^2}}{\left( 1-\frac{q^2}{c^2}\right) ^3}. \end{aligned} \end{aligned}$$

In the formula for energy, the three independent variables q, T, and V appear, ruling out once again any decomposition into a kinetic energy dependent only on q and an internal energy dependent only on T and V in the theory of relativity. Mechanical quantities, such as momentum $\mathbf{{G}}$, are determined not only by velocity but also by temperature and volume, as are the masses. The transverse mass of radiation is

$$\begin{aligned} m_t\!=\!\frac{G}{q}\!=\! \frac{E_{0}+p_{0} V_{0}}{c^2} \frac{1}{\sqrt{1-\frac{q^{2}}{c^{2}}}} \!=\! \frac{4}{3c^2}\frac{a c^5 T^4 V}{\left( c^2-q^2\right) ^{\frac{5}{2}}} \frac{c}{(c^2-q^2)^{\frac{1}{2}}} \!=\!\frac{4c^{4}}{3} \frac{aT^4 V}{\left( c^2-q^2\right) ^3}.\nonumber \\ \end{aligned}$$

(2.28)

Since the magnitude of the momentum does not change in the case of a transverse acceleration, one can simply plug ${E}_{0}$ into the relativistic formula for the transverse mass, Eq. (1.15). In contrast, in the context of longitudinal acceleration, as discussed earlier, the mass is fully determined only when the manner in which the change of state occurs is specified. If temperature T and volume V are held constant, one obtains the isothermal–isochoric mass

$$\begin{aligned} m_{l}=\frac{\partial G}{\partial q}=\frac{4 a c^4}{3} T^4 V \frac{c^2+5 q^2}{\left( c^2-q^2\right) ^4}, \end{aligned}$$

where a heat supply must occur to prevent temperature T and volume V from decreasing according to Eqs. (2.12) and (2.15). The longitudinal adiabatic–isochoric mass is

$$\begin{aligned} m_{l}=\left( \frac{\partial G}{\partial q}\right) _{S, V} = \frac{4 a c^4\left( 3 c^2-q^2\right) }{9\left( c^2-q^2\right) ^4} T^4 V, \end{aligned}$$

and so forth. Once again, a particularly significant situation arises in the case of the longitudinal adiabatic–isobaric process, where quantities with index 0 do not change during the acceleration process. Consequently, one only needs to use the relativistic formula for the longitudinal mass Eq. (1.15) and plug in the value $E_{0}$

$$\begin{aligned} m_{l}= & {} \left( \frac{\partial G}{\partial q}\right) _{S, p} = \frac{E_{0}+p_{0} V_{0}}{c^2} \frac{1}{\sqrt{1-\frac{q^{2}}{c^{2}}} ^{3}} =\frac{4}{3c^2}\frac{a c^5 T^4 V}{\left( c^2-q^2\right) ^{\frac{5}{2}}} \frac{c}{(c^2-q^2)^{\frac{3}{2}}}\nonumber \\= & {} \frac{4 a c^4 T^4 V}{3\left( c^2-q^2\right) ^4}. \end{aligned}$$

(2.29)

It is apparent that, according to eqs. (2.28) and (2.29), the mass of cavity radiation in adiabatic–isobaric motion at constant $T_{0}$ behaves like that of a relativistic point particle, Eq. (1.15). Of course, for the case of the cavity at rest, one always obtains the same value for the rest mass

Planck can then conclude that pure radiation (without matter) has a ‘mass’⁴³ which amounts to $\frac{4}{3}$ of its rest energy: $m_{0}=\frac{4}{3} E_{0}/c^2$. This formula for the ‘apparent’ mass of cavity radiation was, of course, already obtained by Hasenöhrl (1905).⁴⁴ However, once again, Planck sought the principle beyond the formula and arrived at a result that applies to any thermo-mechanical system (see Hasenöhrl 1907, 1908).⁴⁵

Still, as is evident, also Planck does not recover the relativistic relation $m_{0}={E_{0}}/{c^2}$. Surprisingly, he does not address the issue explicitly. A solution to the $\frac{4}{3}$ paradox for the case of hohlraum radiation seems to have been first suggested by Einstein (1907, §2; 1908, §14) at around the same time. Cavity radiation can exist only within the walls of a container; otherwise, the radiation would fly apart. The factor $\frac{4}{3}$ arises from the radiation pressure $p_{0}$ on the walls, which, as we have seen, is $\frac{1}{3}$ of the energy density $w _{0}$. Thus, the total energy is $E_{0}+p_{0}V_{0}=E_{0}+\frac{1}{3}E_{0}$. However, if the system is in equilibrium, that is, if the rest volume $V_{0}$ is to be kept constant, there must be an inward pressure, exerted by forces not belonging to the system, that exactly balances the outward pressure of radiation. Such external forces do not perform net work; yet they contribute to the total energy of the moving system, by $-p_{0}V_{0}=-\frac{1}{3}E_{0}$, reducing the $\frac{4}{3}E_{0}$ to $E_{0}$. Planck did not appear to have emphasized an implication of his own result: the inward pressure p of the cavity material walls must also negatively contribute to its total energy even without performing any ‘deformation work’. With this addition, the pressure term vanishes and the complete system ‘cavity radiation + walls’ in adiabatic–isobaric motion behaves like a point particle of mass

$$\begin{aligned} m_{0}= \frac{E_{0}}{c^{2}} = \frac{a T_0^4 V_0}{c^{2}}. \end{aligned}$$

However, also Einstein was only able to apply this reasoning to particular case studies without providing a universal proof valid for all physical systems.⁴⁶ As we shall see, Planck soon made an important step in this direction.⁴⁷

4 Planck’s relativistic general dynamics

On June 28, 1907, a few days after presenting his paper on general dynamics, Planck intervened at the German Physical Society, offering further reasons to question the reliability of Kaufmann’s results (Planck 1907a). At this point, Kaufmann’s experiments no longer presented a ‘stumbling block’ to the acceptance of principle of relativity (Cushing 1981, 1146). The issue of the compatibility of the principle of least action and the principle of relativity came to the forefront. In a letter written a few days later, Planck sought Einstein’s opinion on this ‘burning’ question (Planck to Einstein, Jul. 6, 1907; CPAE, Vol. 5, Doc. 47). Planck complained about Bucherer’s opposition to his general dynamics because he did not believe the two principles to be compatible. However, Einstein seemed to have been on Planck’s side: “It is all the more delightful for me, therefore, to gather from your esteemed card that you do not currently share the views of Mr. B.” (Planck to Einstein, Jul. 6, 1907; CPAE, Vol. 5, Doc. 47). As Planck continued, “the proponents of the principle of relativity still constitute such a small bunch [Häuflein]”, and it was of great importance that they form a common front (Planck to Einstein, Jul. 6, 1907; CPAE, Vol. 5, Doc. 47).

However, the ‘small bunch’ was quickly gaining new acolytes. In September, Einstein accepted Johannes Stark’s request to write a review paper on relativity (Einstein to Stark, Sep. 25, 1907; CPAE, Vol. 5, Doc. 58). Despite the difficulty in obtaining the literature, Einstein was already aware of Planck’s two contributions to relativity. In the final version of the paper, Einstein included both Planck’s results, however, without giving much emphasis to the principle of least action (Einstein 1908). In October, Hermann Minkowski wrote to Einstein requesting a copy of his relativity papers (Minkowski to Einstein, Oct. 9, 1907; CPAE, Vol. 5, Doc. 62). In a lecture to the Göttingen Mathematical Society, delivered on November 7, 1907, Minkowski expressed his first thoughts on relativity and discussed Planck’s 1907 paper on the dynamics of moving systems. Specifically, Minkowski suggested that the invariance of the action could be directly derived within his four-dimensional formalism without the need to detour through thermodynamics (Minkowski 1907).

1908 marked a pivotal year for relativity. Einstein’s (1908) review paper appeared in January. In April, Minkowski (1908) published his paper on the electrodynamics of moving media with the first mature presentation of his four-dimensional matrix calculus. By July, Bucherer (1908) published the results of his experiments on electrons that were more favorable to relativity. Planck hoped to finally meet Einstein in person at the 80th meeting of the Gesellschaft Deutscher Naturforscher und Ärzte, which was going to be held from September 20, 1908 until September 26, 1908 in Cologne (Planck to Einstein, Sep. 8, 1908; CPAE, Vol. 5, Doc. 118). However, young Einstein, who was still working as a part-time physicist, was exhausted and did not attend.⁴⁸ Nevertheless, the meeting is commonly recognized as a key turning point in the reception of relativity theory in Germany. On September 21, Minkowski (1909) presented relativistic kinematics as a four-dimensional geometry. On On September 22, Bucherer (1909) presented his experimental results as a confirmation of relativity. Finally, on September 23, Planck (1908) delivered a seminal, although somewhat neglected, paper on relativistic dynamics.

4.1 The principle of action and reaction in general dynamics

In his Cologne talk, Planck addressed the role of the principle of equality of action and reaction in relativistic general dynamics, showing that the principle does not only apply to “mechanics in the narrower sense but also electrodynamics and thermodynamics” (Planck 1908, 828). Newton’s principle of action and reaction seems to be violated in Lorentz-Maxwell electrodynamics. The electromagnetic field produces the acceleration of the electrons, and the electrons gain momentum. However, it is unclear where the momentum comes from, as Lorentz ether, the seat of the electromagnetic field, has no movable parts (Poincaré 1900). It was Abraham (1902), Planck explained that suggested that the principle could be saved by introducing the notion ‘electromagnetic momentum’ in analogy to that of ‘electromagnetic energy’.

The energy principle is compromised when the electromagnetic energy is not taken into account. Similarly, the action and reaction principle is violated when relying exclusively on mechanical momentum, the momentum of the moving electrons. It can be reinstated once an ‘electromagnetic momentum’, the rather unintuitive notion of momentum of the field, is introduced (Planck 1908, 829). The gain of momentum by the electrons is compensated for by a loss of momentum stored in the field. After all, just as there are different kinds of energies in addition to mechanical energy, it is not surprising to introduce various types of momentum alongside mechanical momentum. In a field theory, one has to introduce the corresponding densities and fluxes. Thus, just as the electromagnetic field has an energy density $w = \frac{1}{8 \pi }\left( \mathbf{{E}} ^2+\mathbf{{B}} ^2\right)$, one can attribute to it a certain momentum per unit volume, a momentum density $\mathbf{{g}}$.

Planck sought to investigate whether, from the standpoint of relativistic general dynamics, a new definition of momentum was possible that includes both mechanical and electromagnetic momenta as special cases: “An affirmative answer to this question would, in any case, lead to an advance in the understanding of the true meaning of the principle of reaction” (Planck 1908, 829). As we have seen, Planck proved that in the theory of relativity the momentum of a thermo-mechanical system is defined by Eq. (2.23). Let us divide the expression Eq. (2.23) by the volume V to obtain the energy density $w = E/V$ and the momentum density $\mathbf{{g}} = G/V$. Of course, $pV/V=p$

$$\begin{aligned} \mathbf{{g}} = \frac{w +p}{c^2}q. \end{aligned}$$

(3.1)

As Planck suggests, Eq. (3.1) can be understood by considering a fluid flow through the surface S of the enclosed volume V. If the fluid has density $\varrho$, then $\varrho q$ can be termed the ‘mass flux’. Similarly, in Eq. (3.1), if $w$ is the energy density, then $w \frac{q}{c^{2}}$ corresponds to a flux of energy density, and $p\frac{q}{c^{2}}$ represents the additional energy flow resulting from external pressure. Equation (3.1) asserts that when the flow of energy is multiplied by $\frac{1}{c^{2}}$, it equals the momentum density $\mathbf{{g}}$, i.e., the momentum per unit volume in space. At constant pressure p, the energy density flux is nothing but the flux density of heat energy, which contributes to the momentum density.

Already in October 1907, Hasenöhrl (1907) noticed that Planck’s Eq. (3.1) resembles the relation introduced by Abraham in the context of electrodynamics. Whenever there is a flow of electromagnetic energy, the energy flowing through a unit area per unit time $\mathbf{{S}}_{{ \textsc {em}}}=\frac{c}{4 \pi } \mathbf{{E}} \times \mathbf{{B}}$, when multiplied by $1 / c^2$, is equal to the momentum per unit volume in space

(3.2)

For Abraham, the relation between momentum density and energy flux is valid only within electrodynamics; Planck transformed it into a general theorem of relativistic general dynamics. Whenever there is a flow of energy of any kind (field energy, heat energy, or any other type of energy), the energy passing through a unit area per unit time, when multiplied by $1/c^2$, always equals the momentum per unit volume in space. As Planck put it in a famous passage:

In the theory of relativity, momentum can now be traced back in general to the vector expressing the flow of energy, not only the Poynting electromagnetic energy flow but the energy flow in general. From the perspective of the theory of local action, each type of energy can only change its location in space through continuous propagation, not through abrupt changes. Therefore, the energy principle generally requires that the change in the total energy within a volume be equal to a surface integral, namely the algebraic sum of the energy flowing into the volume through its entire surface. The flow can occur through radiation, as in the Poynting vector, through conduction, as in pressure or impact and heat conduction, and through convection, when ponderable atoms or electrons enter the considered surface. In any case, the total energy flow at any point in space, per unit of surface and time, is a specific finite vector, and the quotient of this vector by the square of the speed of light c is generally the momentum per unit volume. (Planck 1908, 829)

The proportionality of energy flux density and momentum density has been established for the electromagnetic field through the correlation between the Poynting vector and momentum density. However, Planck can now assert its universal validity, presenting it as a consequence of the relativity principle. If the principle of relativity holds, then the momentum density of a system of any kind can be computed by dividing the energy flow in the desired direction by $c^2$. Planck famously labeled this generalized principle of equality of action and reaction as the ‘theorem of inertia of energy’

(3.3)

This theorem can be viewed as a generalized form of the principle of the equivalence of mass and energy. While the latter pertains solely to total energy, Planck’s theorem also addresses the localization of momentum and energy.⁴⁹

Proceeding further, Planck points out that, just as energy conservation implies the concept of energy flux and flux density, the conservation of momentum also implies the concept of ‘momentum flux’ and the corresponding ‘flux density’. Intuitively, flux is the total amount of a quantity passing through a surface. If the flux is non-uniform, it must be represented by a surface integral over the flux density. Since energy density $w$ is a scalar field, the energy flux is expressed by a single surface integral; thus, the energy flux density is a vector $\mathbf{{S}}$.⁵⁰ However, since momentum is a vector, momentum flux must be expressed by three surface integrals, corresponding to the three components of momentum density $\mathbf{{g}}$. Planck concludes that the momentum flux density must take the form of a ‘tensor triple’, a mathematical object introduced by Woldemar Voigt (1898) to describe the stresses and strains of crystals. In Voigt’s parlance, a ‘tensor’ is a two-sided vector indicating tension and compression along a line acting on the surface of an elastic body. Stresses can be characterized by three of these ‘tensors’ in three perpendicular directions, a ‘tensor triple’ (see Abraham 1902b, 28). In Planck’s notation, taken from Abraham (1902b)

$$\begin{aligned} X_n&=X_x \cos (n x)+X_y \cos (n y)+X_z \cos (n z), \nonumber \\ Y_n&=Y_x \cos (n x)+Y_y \cos (n y)+Y_z \cos (n z), \nonumber \\ Z_n&=Z_x \cos (n x)+Z_y \cos (n y)+Z_z \cos (n z), \end{aligned}$$

(3.4)

Fig. 2

Cauchy stress tetrahedron

where n is the normal to a plane of unit area inclined to all three coordinate axes $x,y,z$ (see Fig. 2); $\cos (n x), \cos (n y), \cos (n z)$ are the so-called direction cosines, the cosine of the angles between n and the coordinate axis $x,y,z$. It can be shown that $\cos (n x), \cos (n y), \cos (n z)$ are the projection areas of the unit area plane onto the coordinate planes.⁵¹${X}_n, {Y}_n, {Z}_n$ are components of a force acting on the sloping surface of unit area. In relation to the orientation of the plane, each component can be represented as the resultant of vectors acting across the projected areas with components: ${X}_x, {X}_y, {X}_z ; {Y}_x, {Y}_y, {Y}_z ; {Z}_x, {Z}_y, {Z}_z$. Since $X_x, Y_y, Z_z, X_y=Y_x, Y_z=Z_y, Z_x=X_z$, there are only six independent components. The capital letters indicate the direction of the respective force component, and the indices indicate the normal direction of the projected areas on which the respective component acts. A force parallel to x acting on the area $\cos (n x)$ will give a normal stress of $X_{x} \cos (n x)$. However, this is equivalent to saying that $X_x \cos (n x)$ is the rate of flow of the x-component of momentum through the unit area $\cos (n x)$ perpendicular to x.⁵²

In the case of electromagnetic momentum flux in a vacuum, the tensor triple corresponds to the so-called ‘Maxwell stresses’ used in electrodynamics; in the case of mechanical momentum flux, the tensor triple corresponds to the ‘elastic stresses’. This is a powerful result that will soon be used⁵³ to go beyond the case of scalar pressure p that Planck considered in his previous papers.⁵⁴ After the introduction of a non-mechanical ether, ‘Maxwell stresses’ in empty space lost their physical significance because, contrary to ‘elastic stresses’, they could not be considered as representing the state of a material medium. However, Planck argues that its physical meaning might reemerge by adopting the notion of ‘momentum flux density’ and ‘momentum density’. Needless to say, this does not imply that we should picture Maxwell stresses as elastic stresses of the ether, as in the old attempts to provide a mechanical interpretation of electromagnetic phenomena. From the point of view of Planck’s general dynamics, both the mechanical interpretation of electromagnetic phenomena and the electromagnetic interpretation of mechanical phenomena are equally misleading. Instead of trying to reduce mechanical momentum to electromagnetic momentum or vice versa, relativistic general dynamics introduced a new notion of ‘momentum’, of which both mechanical and electromagnetic momentum were only special cases. As we shall see, in the following months, Planck found an opportunity to delve into this notion of unification in greater detail.

4.2 Planck’s general dynamics: the philosophical outlook

In his Cologne lecture as well as in the discussion that followed, Planck refrained from making an unconditional commitment to the relativity principle, awaiting experimental verification and a comprehensive investigation of its consequences (Planck 1908, 829). However, in private correspondence with Lorentz—who could not attend the Cologne meeting (Planck to Lorentz, Oct. 7, 1908; SCHAL, Vol. 1, Doc. 176)—Planck did not conceal his satisfaction with Bucherer’s ‘confirmation’ of the Einstein–Lorentz theory (Planck to Lorentz, Nov. 21, 1908; SCHAL, Vol. 1, Doc. 178). Experiments on fast-moving electrons at the Berlin Institute of Physics⁵⁵ further reinforced Planck’s conviction of “victory of the deformable electron”: “Then it seems that the theory of relativity will need to be fully realized” (Planck to Lorentz, Nov. 21, 1908; SCHAL, Vol. 1, Doc. 178).

Around that time, Adriaan D. Fokker, a student of Lorentz’s, found himself in Berlin for family matters. Fokker carried a letter of invitation from Lorentz and managed to successfully convince Planck to give a lecture at Leiden.⁵⁶ Planck’s seminal lecture was held on December 9, 1908 in front of the Leiden student association. Planck seized the opportunity to make his first public statement regarding the philosophical underpinnings of his project on a general dynamics. “I did not take the lecture lightly”, Planck wrote to Lorentz a few days later. While he was accustomed to speaking in front of students, he remarked, it was quite challenging when there was a physicist of Lorentz’s stature among the audience. However, Planck was happy with Lorentz’s approval (Planck to Lorentz, Dec. 13, 1908; SCHAL, Vol. 2, Doc. 179) and consistently cherished the memories of his time in Leiden (see Planck 1929).

The lecture was published a year later in the Physikalische Zeitschrift and as separatum (Planck 1909). Planck’s stance on the principle of action and reaction reveals itself as a particular manifestation of his broader philosophical outlook on the challenge of unification in physics. Initially, Planck famously notes, physics had at least as many main branches as human beings have senses: mechanics corresponded to the sense of touch, optics to sight, acoustics to hearing, and thermodynamics to the perception of heat. However, this classification underwent a gradual reshuffle. Acoustics became fully integrated with mechanics, while magnetism and optics merged into electrodynamics. These unifications were accompanied by a noticeable detachment from the anthropomorphic aspects in all physical definitions (Planck 1909, 5–8). By the time Planck was delivering his lecture, there were ultimately two broad branches of physics remaining: “mechanics and electrodynamics, or, as they may also be called, physics of matter and physics of the ether” (Planck 1909, 8). However, Planck continues, this division is clearly provisional, as demonstrated by several processes that cannot be precisely classified as belonging to either mechanics or electrodynamics (Planck 1909, 8f.).

Ultimately, Planck sensed that “the original distinction between ether and matter is gradually fading” (Planck 1909, 9). The question was simply to decide which of the two branches of physics would ultimately survive and absorb the other (see also Witte 1909b). However, Planck was skeptical of the feasibility of both the ‘mechanical worldview’ and the ‘electromagnetic worldview’. Planck envisaged a different kind of unification of the ‘physical worldview’, a unification in which both mechanics and electrodynamics appear as special cases of a more encompassing framework: “A slightly more generalized view of mechanics might thus allow it to include electrodynamics, and, in fact, there are many indications that these two divisions, which are already encroaching upon one another, will be joined into one single general scheme of dynamics” (Planck 1909, 8; my emphasis).

In other terms, Planck did not seek a material unification, which would involve reducing all phenomena to the same type of interaction, whether it be electrodynamic or mechanical. Planck also rejected the so-called ‘energetics’ as an attempt to find in energy a common ‘substance’ of which both kinds of phenomena are made.⁵⁷ On the contrary, Planck aimed at a formal unification, where all the dynamical laws governing all interactions could be derived from a common principle (see also Witte 1909a). As we have mentioned, Planck’s stance was built on Helmholtz’s recognition that all dynamical laws governing mechanical and electromagnetic processes shared a common feature: they could all be derived from the principle of least action. It is true that the principle of energy conservation also applies to all of these processes. However, it does not unambiguously determine the laws that govern them. The principle of least action is much more powerful. It allows determining both mechano-dynamic and electrodynamic equations uniquely and entails the principle of conservation of energy as a special case, as the value of energy can be determined from the kinetic potential. As Helmholtz already recognized, the principle of least action proves to be insufficient in the domain of irreversible processes (Planck 1909, 18). Planck credited Boltzmann for having bridged the chasm between irreversible and reversible processes by establishing the relationship between entropy and disorder (Planck 1909, 22–29). The pursuit of general dynamics was back on track, as all elementary processes in nature could be considered as ultimately reversible.

4.3 Planck’s general dynamics: the mathematical structure

Planck entered into some technical details of the project of a general dynamics in a series of lectures delivered the following year at Columbia University’s physics department in New York City. The lectures were prepared in the winter of 1908–1909, just after the Leiden talk, and were intended to serve as its technical counterpart (Planck 1910a). They were delivered on four consecutive weekends in the spring of 1909, with a general lecture on Friday and a more detailed and mathematical lecture on Saturday (Needell 1980, 120). The problem of irreversibility dominated the first six lectures. In the final two lectures, held in May, Planck laid out his program of relativistic general dynamics, introducing the principle of least action and the principle of relativity. Indeed, in a postcard to Laue from Washington, where he had traveled to attend the annual meeting of the American Physical Society, Planck claimed that his ultimate goal was “making propaganda for the relativity principle” (Planck to Laue, Apr. 28, 1909; Deutsches Museum Bibliothek).

Once irreversibility is reduced to the concept of ‘disorder’, the general dynamics appeared to be capable of covering all fundamental natural processes. Whether these processes are mechanical, electromagnetic, or thermal in nature, the laws that govern them can all be derived from one principle—the principle of least action (Planck 1910a, 5). As Planck pointed out, providing a short historical sketch, Hamilton’s principle of least action emerged within the domain of mechanics, attaining equal status alongside numerous other principles: the principle of d’Alembert, the principle of virtual displacement, Gauss’s principle of least constraint, etc. (Planck 1910a, 97). All these principles ultimately represent equivalent formulations of the same laws of mechanics, and the choice of which to use depends on the specific problem at hand. However, according to Planck, Helmholtz had shown that Hamilton’s “principle of least action has the decided advantage over all the other principles mentioned” (Planck 1910a, 97). The former were a mere byproduct of mechanics, that is, they hold as long as the laws of mechanics are valid; Hamilton’s principle, on the contrary, is grounded at a deeper level than the equations of mechanics, which appears to be only one of its instantiations, alongside those of electrodynamics and the thermodynamics of reversible processes.

As we have seen, Planck credited von Helmholtz (1886) for providing a general formulation of the principle of least action. Consider a given physical system, subject to specified external forces, that passes from its initial configuration at time $t_1$ to a final configuration at time $t_2$. Out of all possible paths that the system might take, the one that is actually realized in nature is characterized by the condition

$$\begin{aligned} \int _{t_0}^{t_1}(\delta H+ \delta A) dt=0, \end{aligned}$$

(3.5)

where $\delta H$ denotes the variation in kinetic potential H at any point in time, corresponding to a slightly shifted path through which the system could move from the same initial to the same final state within the same time span. H is a function of a number of independent parameters, the generalized coordinates $\varphi _1,\varphi _2,\varphi _3\dots$ and their rate of change with time, the generalized velocities $\dot{\varphi _1},\dot{\varphi _2},\dot{\varphi _3},\dots$

$$\begin{aligned} H=f(\varphi _1,\varphi _2,\varphi _3\dots \dot{\varphi _1},\dot{\varphi _2},\dot{\varphi _3}). \end{aligned}$$

As we have seen, in classical point dynamics H corresponds to the ‘Lagrangian’ of the system, the difference between potential energy, as a function of the positions of mass points $\varphi _{i}$, and kinetic energy as a quadratic homogeneous function of their velocities, $\dot{\varphi }=\frac{{\textrm{d}}\varphi }{{\textrm{d}}t}$. However, in the general case $\varphi _{i}$ need not refer to positions of mass points and $\dot{\varphi _1},\dot{\varphi _2},\dot{\varphi _3},\dots$ to their velocities of particles, but may refer to macroscopic parameters. Moreover, in some cases, the separation of the two forms of energy might be no longer recognizable. The kinetic potential entering the modified principle of least action does not need to have its original form, in which the kinetic energy is a quadratic form in the velocities and the potential energy does not contain the velocities; in Helmholtz’s liberalized version of the kinetic potential, for example, the kinetic energy may depend on ‘gyroscopic terms’ linear in velocity (see Helmholtz 1898, §77).

In Helmholtz–Planck’s formulation, moreover, the ‘action’ includes besides $\delta H$ an external term $\delta A$, the total virtual work

$$\begin{aligned} \delta A = {\Phi }_1 \delta \varphi _1 + {\Phi }_2 \delta \varphi _2 + {\Phi }_2 \delta \varphi _2 \dots \end{aligned}$$

by ${\Phi }_1, {\Phi }_2, \dots$, the so-called generalized external forces which act in such a way as to increase the values of the corresponding generalized coordinates. In this generalized form, the principle applies to nonconservative systems acted upon by friction, electrical resistance, and other non-mechanical forces that are not derivable from a potential function (Planck 1910a, 5).

The mathematical expression of the kinetic potential H depends on the characteristics of the system under scrutiny. Uncovering the specific form of H in various classes of phenomena—might be of mechanical, electrical, and thermodynamic nature—is a fundamental task of general dynamics (Planck 1910a, 98). Once one has found experimentally or by educated guess what the form of H is for any particular class of physical systems, the calculus of variations yields Lagrange’s equations that in Helmholtz–Planck form are

$$\begin{aligned} \begin{aligned} \Phi _1-\frac{{\textrm{d}}}{{\textrm{d}}t}\left( \frac{\partial H}{\partial \dot{\varphi }_1}\right) +\frac{\partial H}{\partial \varphi _1}&=0,\\ \Phi _2-\frac{{\textrm{d}}}{{\textrm{d}}t}\left( \frac{\partial H}{\partial \dot{\varphi }_2}\right) +\frac{\partial H}{\partial \varphi _2}&=0,\\ \text {etc.}\dots ,&\end{aligned} \end{aligned}$$

(3.6)

where the external terms $\Phi _1,\Phi _2,\,\text {etc.}$ express the generalized forces acting on the system in such a sense as to tend to increase the values of the corresponding generalized coordinates (Planck 1910a, 99). The dynamical laws governing mechanical, thermodynamic, and electrodynamic systems can be derived as solutions to Eq. (3.6). In this sense, Planck considered his general dynamics as a ‘unification’ of these different branches of physics.

To understand the kind of unification Planck had in mind, one might take a closer look at an example he provides. Planck considers a class of theories in which the generalized coordinates form a continuous manifold, as in elasticity theory and electrodynamics (Planck 1910a, 105–109). In both cases, one might take the vector $\mathfrak {v}_x, \mathfrak {v}_y, \mathfrak {v}_z$ as generalized coordinates. The difference between elasticity theory and electrodynamics lies in the different dependence of the potential energy on the generalized coordinates $\mathfrak {v}$: in elasticity theory, the potential energy depends on the spatial derivative of displacement (the strain or deformation quantities), in electrodynamics it depends on the squared curl of the vector potential (squared magnetic field). However, in both cases, the field equations can be derived from the principle of least action. In particular, in the case of electrodynamics, one can derive Maxwell’s equations. In this sense, elasticity theory and electrodynamics are structurally similar. However, according to Planck, this structural analogy does not amount to a mechanical interpretation of electrodynamics:

Are, then, the electromagnetic processes thus referred back to mechanical processes? By no means; for the vector $\mathfrak {v}$ employed here is certainly not a mechanical quantity. It is, moreover, not possible in general to interpret $\mathfrak {v}$ as a mechanical quantity, for instance, $\mathfrak {v}$ as a displacement, $\dot{\mathfrak {v}}$ as a velocity, and the curl of $\dot{\mathfrak {v}}$ as a rotation. In fact, e.g., in an electrostatic field, $\dot{\mathfrak {v}}$ is constant. Therefore, $\mathfrak {v}$ increases with time beyond all limits, and the curl $\mathfrak {v}$ no longer can signify a rotation. However, from these considerations the [im]possibility of a mechanical explanation of electrical phenomena is not proven. It does appear, on the other hand, to be undoubtedly true that the significance of the principle of least action may be essentially extended beyond ordinary mechanics and that this principle can therefore also be utilized as the foundation for general dynamics, since it governs all known reversible processes (Planck 1910a, 109)

This passage illustrates Planck’s departure from the conventional approach of his contemporaries in understanding the interconnections among different branches of physics. Most of his peers pursued ‘horizontal’ unification, attempting to reduce one class of interactions to another, e.g., electrodynamics to mechanics. On the contrary, Planck embraced a ‘vertical’ unification, seeking unity through an overarching principle that governs all classes of interactions (see Liu 1997). Elasticity and vacuum electrodynamics are unified in the sense that the equations of both fields can be derived from the principle of least action. One can wonder whether this structural analogy deserves to be called a unification. Indeed, no attempt is made to derive both sets of equations from the same kinetic potential as in Helmholtz’s treatment of thermodynamics, and even less to establish a coupling between two sets of variables as in Planck’s relativistic thermodynamics.

Planck does not seem to have addressed this issue or possibly envisioned that relativity would have allowed for a stronger kind of unification. Indeed, after having sketched the mathematical framework of Helmholtz’s general dynamics in the 7th lecture, in the last, 8th lecture held on May 15, 1909, Planck outlines its relativistic completion. The lecture, albeit short, marks the convergence point for the entire lecture series at Columbia—and, ultimately, of Planck’s work on relativity. The last step of general dynamics, Planck pointed out, was to determine the dependence of H on the velocity based on the principle of relativity (Planck 1910a, 110). Since every field of physics is dominated by the principle of least action, Planck concludes that the meaning of the principle of relativity emerges from the specific form it prescribes to the kinetic potential H (Planck 1910a, 123). The latter prescription can be ultimately summarized by the demand that for every space element of a physical system $H\,dt=H'\,dt'$, in all coordinate system s related by the Lorentz transformations. This implies that the kinetic potential must satisfy the following relationship:

$$\begin{aligned} \frac{H}{\sqrt{c^2-q^2}}=\frac{H^{\prime }}{\sqrt{c^2-q^{\prime 2}}}. \end{aligned}$$

(3.7)

The additive constant that Planck removes by laborious calculations in his 1907 article (see above, Sect. 2.4.2) is now set equal to zero as a postulate. The theory of relativity requires the validity of Eq. (3.7) to ensure the invariance of the corresponding action. The task of relativistic general dynamics is to (I) determine the expression for the ‘classic’ kinetic potential H in a particular domain for the case of rest or low velocities; (II) make alterations when necessary, determining the ‘relativistic’ expression of H that (IIa) satisfies Eq. (3.7) and (IIb) reduces to the classical case in low-speed approximation. Planck briefly summarizes the results of his previous relativistic investigations, applying this reasoning to the case of point dynamics, vacuum electrodynamics, and thermodynamics; finally, he briefly introduces the case of cavity radiation (Planck 1910a, 124–126). However, in all these instances, the equations of motion are now obtained by imposing the condition Eq. (3.7) from the outset. In this manner, the results Planck had previously obtained in isolation clearly emerge as integral components of his project of a relativistic general dynamics.

Planck met Einstein for the first time in person a few months later, in September 1909, at the meeting of the Gesellschaft Deutscher Naturforscher und Aerzte in Salzburg, where they discussed the latter’s rather bold stance on the nature of radiation (Einstein 1909). However, it was in the keynote lecture at the following meeting of the Society in Königsberg on September of 1910 that Planck had the opportunity to celebrate the revolutionary nature of the relativity principle (Planck 1910b). The relativity principle, he insisted, did undercut the program of the mechanical worldview; however, it did not imply a postponement in the realization of the unified world picture. As far as I can see, for the first time, Planck alludes to the four-dimensional formulation of the principle of least action, $\int H d\tau$, where $\tau = t {\sqrt{1- \frac{v^2}{c^2}}}$ is the so-called ‘proper time’. He labeled it the crowning of the entire system of physics, unifying the conservation laws of momentum and energy, and of matter and energy (Planck 1910b, 27–28).

Thereby, Planck believed that he had established the general dynamics as a powerful research program, a formal method to find theories by searching for the appropriate Lorentz-invariant form of H. Also, in his last contribution to relativity, for example, Planck (1910c) reveals Planck’s commitment to this project. In a short note commenting on the so-called Ehrenfest (1909) paradox, Planck drew the conclusion that in relativity theory, there are only deformable bodies (see Giovanelli 2023). The task of determining the deformation of a body accelerated in any way is, therefore, essentially an ‘elastic’ problem in both relativity theory and ordinary mechanics (Planck 1910b, 294). The problem can be solved “once the kinetic potential of the elastic deformations is known”, under the condition that the kinetic potential for the unit volume of a material at a given point is Lorentz invariant (Planck 1910b, 294; my emphasis). The problem was addressed by Gustav Herglotz (1911) in the subsequent months.

The doctoral theses supervised by Planck during those years, as well as postdoctoral works written under his influence, exemplify his enduring interest and active endorsement of this program. Some students, such as Wilhelm Heil (1909) and Erich Hupka (1909), revisited the issue of the reliability of Kaufmann-like experiments on fast-moving electrons (Hupka 1910; Heil 1910b, a, 1911). Others were entrusted by Planck with actively developing and extending the general dynamics program to various branches of physics. Ferencz Jüettner (1911b; a) formulated a relativistic kinetic theory of gases, Ernst Lamla (1912a; b) delved into relativistic hydrodynamics, and Erich Henschke (1912) explored the electrodynamics of moving media. In all of these cases, the same methodological procedure was adopted. The classical kinetic potential of a certain class of systems valid for low velocities is established and then modified to comply with principle of relativity. The new relativistic equations are derived via the principle of least action as a solution of Lagrange’s equations. Although the relativistic implications of these new theories could not be empirically tested, their very existence served to demonstrate the possibility of developing general dynamics without encountering contradictions. Planck was confident that the meaning of the ‘quantum of action’ would nicely fit into this program (Planck 1914, 108).

5 Conclusion: the triumph of the great principle of physics

By the turn of the 1910s, while Planck’s scientific productivity slowed down, he assumed an increasingly prominent institutional role within the German physics community. In addition to his various responsibilities within the German Physical Society, Planck was elected as one of the four standing secretaries of the Berlin Academy’s Mathematical-Physical Class in June 1912. It was in this capacity that Planck played a crucial role in bringing Einstein to Berlin. In 1913, the last year before the war, Planck also took on the position of rector at the University of Berlin. He officially assumed his duties on October 15, 1913 and delivered an inaugural lecture on the new paths that physics had taken in the previous decade (Planck 1913). For the layperson, he pointed out, the recent history of physics might appear as a causal succession of unrealistic hopes and bitter disappointments. However, upon closer inspection, “the grand general principles of physics”—the principle of conservation of energy, the principle of conservation of momentum, the principle of least action, and the second principle of thermodynamics—had always held their ground. According to Planck, the latest developments in theoretical physics could be ultimately described as “the triumph of the great principles of physics” (Planck 1913, 30; my emphasis). By the end of the nineteenth century, two principles emerged as victorious: the principle of least action for reversible processes and the second principle of thermodynamics, which dominated irreversible processes.

In his famous 1914 address, at the Founder Day of the University of Berlin, Planck (1914) fully embraced Boltzmann’s explanation of irreversibility, accepting that entire domains of physics were dominated by purely statistical laws. However, Planck remained firmly convinced that physics could not evade postulating absolute dynamical laws for elementary reversible processes. The fact that all these laws can be derived from the principle of least action solidified the status of this principle as the supreme principle in physics. Planck returned to this issue in two contributions written for Kultur der Gegenwart, an ambitious encyclopedia project in more than 50 volumes directed by historian Paul Hinneberg (Stöltzner 2008). Planck contributed two articles to the last, more programmatic, chapter of the volume: Das Prinzip der kleinsten Wirkung (Planck 1915a) and Das Verhältnis der Theorien zueinander (Planck 1915b). Even though both papers were intended as encyclopedia entries, Planck utilized the opportunity to expand upon the key points of his programmatic Leyden lecture, providing a more detailed historical and philosophical framework for his overarching project to bring mechanodynamics, thermodynamics, and electro-dynamics “under the banner of the principle of relativity into a unified theory, which I shall call ‘dynamics’” (Planck 1915b, 735).

Ultimately, Planck’s three-dimensional generalized ‘dynamics’, based on the principle of least action and the principle of relativity, did not gain the widespread support he had hoped for. I guess that Planck’s project was superseded by his student Laue’s four-dimensional ‘relativistic continuum dynamics’ (see Darrigol 2022, 225–229), which relied on the principle of relativity, the principle of conservation of energy and momentum (Laue 1911c, a). However, the demise of Planck’s general dynamics was at the same time a confirmation of its methodological insight. Expounding Minkowski–Sommerfeld four-dimensional vector calculus, Laue substituted Newton’s definition of force as mass times acceleration $\mathbf{{F}} =m\mathbf{{a}}$, with the relativistic definition of the four-force-density $\mathbf{{F}}$ as the four-divergence of the ‘world tensor’ $\textbf{T}$, that is, in Laue’s notation

$$\begin{aligned} \mathbf{{F}} = - \Delta {\textrm{iv}}\textbf{T}. \end{aligned}$$

(4.1)

In the presence of the force-density $\mathbf{{F}} =F _{j}$, a ‘system’ undergoes a divergence in the 16-component world tensor ‘worldtensor’ $\textbf{T} =T_{j k}$; that is, it behaves like a ‘source’ or a ‘sink’ of the flow of energy and momentum at a space-time point. Thereby, the two aspects of ‘force’ as ‘the time rate of change of momentum’ and as ‘the space rate of change on energy’ are unified as the space and time components of the four-vector $\mathbf{{F}}$. The two local conservation laws of energy and momentum are encapsulated into the simple differential expression $-\Delta {\textrm{iv}}\textbf{T} =0$ that, when integrated over an appropriate volume, leads to the conservation laws in integral form (Laue 1911a, §15).

Within this formalism, all results that Planck obtained somewhat laboriously fall into place in one fell swoop. The nine spatial components $T_{xx}, T_{xy}, \ldots$ correspond to the components of Planck’s tensor-triple Eq. (3.4), representing the component stresses/momentum flux $\textbf{p} =p_{xy}$. The temporal component of $T_{ll}$ corresponds to the energy density $w$, the three components $T_{yl}$ to the momentum density $\mathbf{{g}}$, and the three components $T_{xl}$ to the energy flux $\mathbf{{S}}$. The symmetry $T_{jk}=T_{kj}$ elegantly encapsulates Planck’s ‘hypothesis’ of the inertia of energy Eq. (3.3), since it implies that $T_{xl}=T_{yl}$ (Laue 1911a, 149). Laue (1911a, 168–170) further shows that, for a complete static system at rest, all stress components vanish $\int \textbf{p} d V=0$, and the remaining components form a four-vector $E, G_{x}, G_{y}, G_{z}$ of invariant length m (in natural units with $c = 1$). Thus, any extended system in static equilibrium (whether it is an electron or cavity radiation) behaves like a structureless point particle, as Planck surmised Eq. (2.25). In the zero-momentum frame $\left( E_0, 0,0,0\right)$, and therefore, $E_{0}=m$ (or $mc^{2}$ in c.g.s. units). This is typically considered as a demonstration of the equivalence of mass and energy Eq. (2.24), which Planck could outline for the special case in which stresses reduce to scalar pressure (Laue 1911c, 534; Laue 1911a, 155f.).

Laue admits that Eq. (4.1) was first introduced by Minkowski (1908) in electrodynamics; however, this was a historical accident due to the higher precision of optical and electrodynamic experiments. In principle, Eq. (4.1) could have originated from any branch of physics. In fact, the universal validity of Eq. (4.1) has nothing to do with the reduction of all physics to electrodynamics. It lies at a deeper level than both mechanics and electrodynamics: “Overall, the unification of the two still separate areas of theoretical physics—electrodynamics and mechanics—does not seem to be achievable through the subordination of one to the other, but rather through the equal subordination of both under higher laws” (Laue 1911b, 186, first emphasis mine). There is little doubt that, in this passage, Laue had his mentor’s conception of unification in mind. His new relativistic dynamics stands over all branches of physics. Equation (4.1) applies to electromagnetic, mechanical, thermal systems or a combination thereof, provided that the proper interpretation of the components of the Welttensor $\textbf{T}$ for the system under consideration has been provided.

Planck and Laue appreciated that the relativistic revolution was possible by exploiting the existence of different levels of physical theories (Flores 1998). A physical theory entails (a) a general theory of motion, or, in Planck’s terminology, a general dynamics, a framework based on a set of principles that provide mathematical criteria to establish what counts as an allowable dynamical law, and (b) the actual dynamical laws describing a particular kind of interaction (Flores 1998; Lange 2016). In broad Kuhnian terms, one might venture to say that ‘normal science’ proceeds by searching for dynamical laws within a particular framework, aiming to reduce approximations to more fundamental interactions, possibly converging to one single type of interaction. On the contrary, ‘revolutionary science’ often addresses the principles that govern the framework itself, trying to establish a new scheme for treating physical theories.

However, these ‘frameworks’ only partially resemble Kuhnian ‘paradigms’. Using Planck’s work as a blueprint, one can try to point out some of their general features through contrast. (a) Frameworks are not simply disciplinary matrices; they consist of mathematically formulated principles that impose specific constraints that circumscribe the range of allowable lower level laws. As we have seen, Planck relied on the principle of relativity and the principle of least action. (b) The transition to a new framework is not an irrational jump. It is obtained by modifying an existing more abstract formulation of the old framework. Planck upgraded the Lagrange–Helmholtz formulation of classical mechanics and thermodynamics by making it Lorentz invariant. (c) Subsequent frameworks are not incommensurable. The transition to a new framework is achieved by introducing a new principle, like the relativity principle; however it presupposes principles that maintain transparadigmatic validity, such as the principle of least action. Ultimately, the success of a new framework is measured by the capacity of its principles to update the approximate laws of the old framework into laws that are exactly valid. Without the input of the old laws, the framework itself seems to be powerless.

Planck’s general dynamics failed as a unification program. However, it might be interesting to study it as a kind of heuristic routine: Put the classical equations in Lagrangian form; ‘relativize’ them by searching for the Lorentz-invariant form of the kinetic potential; find the new dynamical laws as solutions to Lagrange’s equations; if possible, compare the new dynamical laws with experience. I suggest that this routine encapsulates the core of the ‘practice of principles’. Extrapolating general methodological rules from the history of physics is always an unpromising endeavor, as we ultimately possess too few data points. However, it is legitimate to point out an interesting pattern. Even the other great theory of twentieth-century physics, quantum mechanics, seems to replicate a similar methodological routine. As Dirac (1925) has shown, quantum mechanics can be seen as an ‘upgrade’ of the classical Hamiltonian formulation through a modification of the algebra of Poisson brackets. A new general dynamics is established, a new recipe for generating laws: take a classical law, put it in Hamiltonian form, replace Poisson brackets with commutators, and test whether the new laws thus obtained are empirically adequate.

A Appendix: Planck’s ‘Elementary Calculation’

To understand Planck’s derivation of Eqs. (1.7) and (1.8), it is convenient to start with Planck’s result, namely the definition of force as the time derivative of relativistic momentum, Eq. (1.14). Consider the case of a force $\mathbf{{F}}$ that increases the velocity $\dot{x}=v, \dot{y}=0, \dot{z} =0$ of a particle along the x axis. One can split the force into two components and carry on the differentiation. Working with the x-component, one obtains the following:

$$\begin{aligned} F _{x}=\frac{{\textrm{d}}}{{\textrm{d}}t}\frac{m \dot{x}}{\sqrt{1-\frac{q^{2}}{c^{2}}}}=&\frac{m}{\sqrt{1-\frac{q^2}{c^2}}} \frac{{\textrm{d}}\dot{x}}{{\textrm{d}}t}+{m\dot{x}} \frac{{\textrm{d}}}{{\textrm{d}}t} \frac{1}{\sqrt{1-\frac{q^2}{c^2}}} \\ =&\frac{m}{\sqrt{1-\frac{q^2}{c^2}}} \frac{{\textrm{d}}\dot{x}}{{\textrm{d}}t}+\frac{m}{\sqrt{1-\frac{q^2}{c^2}}^{3}} \frac{q}{c^2} \frac{{\textrm{d}}\mathbf{{q}}}{{\textrm{d}}t} \dot{x} \\ =&\frac{m\ddot{x}}{\sqrt{1-\frac{q^2}{c^2}}} +\frac{m(\dot{x} \ddot{x}+\dot{y} \ddot{y}+\dot{z} \ddot{z})}{\sqrt{1-{\frac{q^2}{c^2}}}^3} {\frac{\dot{x}}{c^{2}}}. \end{aligned}$$

Since the component of the force acting in the direction of the velocity changes the magnitude of the relativistic momentum, one has to take into account the time derivative of the Lorentz factor by applying the chain rule

The product of mass, acceleration, and velocity $m(\mathbf{{q}}\cdot \mathbf{{a}})=m(\dot{x} \ddot{x}+\dot{y} \ddot{y}+\dot{z} \ddot{z})$ is the ‘power’ delivered by the force $\mathbf{{F}} \cdot q$. In the case $\mathbf{{F}} =q\mathbf{{E}}$, and by rearranging, one obtains the x-component of Eq. (1.7)

$$\begin{aligned} \frac{m\ddot{x}}{\sqrt{1-\frac{q^{2}}{c^{2}}}} = F _{x}-(\mathbf{{F}} \cdot q) \cdot {\frac{\dot{x}}{c^{2}}} = eE_{x} -e\left( \dot{x} E_x+\dot{y} E_y+\dot{z} E_z\right) \cdot {\frac{\dot{x}}{c^{2}}}. \end{aligned}$$

Abbreviations

CPAE:

Einstein, Albert. 1987-. The Collected Papers of Albert Einstein. Edited by John Stachel et al. 16 vols. Princeton: Princeton University Press.

SCHAL:

Lorentz, Hendrik Antoon. 2008-18. The Scientific Correspondence of H.A. Lorentz. Edited by A. J. Kox. 2 vols. New York: Springer.

WN:

Wien Nachlass. Manuscripts department of the Staatsbibliothek zu Berlin der Stiftung Preußischer Kulturbesitz, Nachlaß Wilhelm Wien.