Skip to main content
SpringerLink
Log in

Nonlinear resonance responses of size-dependent functionally graded cylindrical microshells with thermal effect and elastic medium

  • Original Article
  • Published:
Engineering with Computers Aims and scope Submit manuscript

Abstract

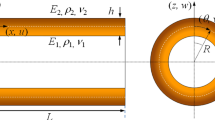
The nonlinear resonance responses of functionally graded (FG) cylindrical microshells with the elastic medium is investigated by considering thermal and scale effects. First, using the modified couple stress theory, the nonlinear dynamics model for FG microshell are established. Then the reduced nonlinear differential equations are derived by Galerkin’s method and static condensation. Finally, subharmonic, superharmonic and primary resonances of FG cylindrical microshells are analyzed by a perturbation method. In addition, the bifurcation characteristics of the nonlinear dynamic responses are investigated by some numerical examples. The effects of key parameters (modal damping, excitation frequency, foundation medium, scale parameter and thermal effect) on the nonlinear resonance responses are also discussed by numerical simulation.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12

Similar content being viewed by others

References

  1. Reddy JN (2011) Microstructure-dependent couple stress theories of functionally graded beams. J Mech Phys Solids 59:2382–2399

    MathSciNet  MATH  Google Scholar 

  2. Alizada AN, Sofiyev AH, Kuruoglu N (2012) Stress analysis of a substrate coated by nanomaterials with vacancies subjected to uniform extension load. Acta Mech 223:1371–1383

    MathSciNet  MATH  Google Scholar 

  3. Ebrahimi F, Barati MR, Civalek Ö (2020) Application of Chebyshev–Ritz method for static stability and vibration analysis of nonlocal microstructure- dependent nanostructures. Eng Comput 36:953–964

    Google Scholar 

  4. Anoop Krishnan NM, Ghosh D (2017) Buckling analysis of cylindrical thin-shells using strain gradient elasticity theory. Meccanica 52:1369–1379

    MathSciNet  MATH  Google Scholar 

  5. Ghayesh MH (2018) Functionally graded microbeams: simultaneous presence of imperfection and viscoelasticity. Int J Mech Sci 140:339–350

    Google Scholar 

  6. Ansari R, Faghih Shojaei M, Ebrahimi F, Rouhi H, Bazdid-Vahdati M (2016) A novel size-dependent microbeam element based on Mindlin’s strain gradient theory. Eng Comput 32:99–108

    Google Scholar 

  7. Zeighampour H, Tadi Beni Y, Dehkordi MB (2018) Wave propagation in viscoelastic thin cylindrical nanoshell resting on a visco-Pasternak foundation based on nonlocal strain gradient theory. Thin Wall Struct 122:378–386

    Google Scholar 

  8. Yang F, Chong A, Lam D, Tong P (2002) Couple stress based strain gradient theory for elasticity. Int J Solids Struct 39:2731–2743

    MATH  Google Scholar 

  9. Lam D, Yang F, Chong A, Wang J, Tong P (2003) Experiments and theory in strain gradient elasticity. J Mech Phys Solids 51:1477–1508

    MATH  Google Scholar 

  10. Asghari M, Kahrobaiyan M, Ahmadian M (2010) A nonlinear Timoshenko beam formulation based on the modified couple stress theory. Int J Eng Sci 48:1749–1761

    MathSciNet  MATH  Google Scholar 

  11. Ke LL, Wang YS, Yang J, Kitipornchai S (2012) Free vibration of size-dependent Mindlin microplates based on the modified couple stress theory. J Sound Vib 331:94–106

    Google Scholar 

  12. Krysko VA Jr, Awrejcewicz J, Dobriyan V, Papkova IV, Krysko VA (2019) Size-dependent parameter cancels chaotic vibrations of flexible shallow nano-shells. J Sound Vib 446:374–386

    Google Scholar 

  13. Veysi A, Shabani R, Rezazadeh G (2017) Nonlinear vibrations of micro-doubly curved shallow shells based on the modified couple stress theory. Nonlinear Dyn 87:2051–2065

    MATH  Google Scholar 

  14. Mehralian F, Tadi Beni Y, Ansari R (2016) On the size dependent buckling of anisotropic piezoelectric cylindrical shells under combined axial compression and lateral pressure. Int J Mech Sci 119:155–169

    Google Scholar 

  15. Ghayesh MH, Farokhi H (2017) Nonlinear mechanics of doubly curved shallow microshells. Int J Eng Sci 119:288–304

    MathSciNet  MATH  Google Scholar 

  16. Ghayesh MH, Farokhi H (2018) Nonlinear dynamics of doubly curved shallow microshells. Nonlinear Dyn 92:803–814

    MATH  Google Scholar 

  17. Gholami R, Darvizeh A, Ansari R, Sadeghi F (2016) Vibration and buckling of first-order shear deformable circular cylindrical micro-/nano-shells based on Mindlin’s strain gradient elasticity theory. Eur J Mech-A/Solids 58:76–88

    MathSciNet  MATH  Google Scholar 

  18. Hashemi SH, Sharifpour F, RezaIlkhani M (2016) On the free vibrations of size-dependent closed micro/nano-spherical shell based on the modified couple stress theory. Int J Mech Sci 115–116:501–515

    Google Scholar 

  19. Saini R, Lal R (2020) Axisymmetric vibrations of temperature-dependent functionally graded moderately thick circular plates with two-dimensional material and temperature distribution. Eng Comput. https://doi.org/10.1007/s00366-020-01056-1

    Article  Google Scholar 

  20. Sofiyev AH, Esencan Turkaslan B, Bayramov RP, Salamci MU (2019) Analytical solution of stability of FG-CNTRC conical shells under external pressures. Thin Wall Struct 144:106338

    Google Scholar 

  21. Sofiyev AH (2019) Review of research on the vibration and buckling of the FGM conical shells. Compos Struct 211:301–317

    Google Scholar 

  22. Pham QH, Pham TD, Trinh QV, Phan DH (2020) Geometrically nonlinear analysis of functionally graded shells using an edge-based smoothed MITC3 (ES-MITC3) finite elements. Eng Comput 36:1069–1082

    Google Scholar 

  23. Duc ND (2016) Nonlinear thermal dynamic analysis of eccentrically stiffened S-FGM circular cylindrical shells surrounded on elastic foundations using the Reddy’s third-order shear deformation shell theory. Eur J Mech-A/Solids 58:10–30

    MathSciNet  MATH  Google Scholar 

  24. Lal R, Saini R (2019) On the high-temperature free vibration analysis of elastically supported functionally graded material plates under mechanical in-plane force via GDQR. J Dyn Syst Meas Control 141:101003-1

    Google Scholar 

  25. Lal R, Saini R (2019) Vibration analysis of functionally graded circular plates of variable thickness under thermal environment by generalized differential quadrature method. J Vib Control 26(1–2):73–87

    MathSciNet  Google Scholar 

  26. Lal R, Saini R (2019) On radially symmetric vibrations of functionally graded non-uniform circular plate including non-linear temperature rise. Eur J Mech-A/Solids 77:103796

    MathSciNet  MATH  Google Scholar 

  27. Lal R, Saini R (2020) Vibration analysis of FGM circular plates under non-linear temperature variation using generalized differential quadrature rule. Appl Acoust 158:107027

    Google Scholar 

  28. Li L, Hu Y (2017) Post-buckling analysis of functionally graded nanobeams incorporating nonlocal stress and microstructure-dependent strain gradient effects. Int J Mech Sci 120:159–170

    Google Scholar 

  29. Attia MA, Mohamed SA (2020) Nonlinear thermal buckling and postbuckling analysis of bidirectional functionally graded tapered microbeams based on Reddy beam theory. Eng Comput. https://doi.org/10.1007/s00366-020-01080-1

    Article  Google Scholar 

  30. Gholami R, Ansari R (2016) A most general strain gradient plate formulation for size-dependent geometrically nonlinear free vibration analysis of functionally graded shear deformable rectangular microplates. Nonlinear Dyn 84:2403–2422

    MathSciNet  Google Scholar 

  31. Liu CC, Yu JG, Xu WJ, Zhang XM, Zhang B (2020) Theoretical study of elastic wave propagation through a functionally graded micro-structured plate base on the modified couple-stress theory. Meccanica 55:1153–1167

    MathSciNet  Google Scholar 

  32. Sahmani S, Ansari R, Gholami R, Darvizeh A (2013) Dynamic stability analysis of functionally graded higher-order shear deformable microshells based on the modified couple stress elasticity theory. Compos Part B Eng 51:44–53

    MATH  Google Scholar 

  33. Tadi Beni Y, Mehralian F, Razavi H (2015) Free vibration analysis of size-dependent shear deformable functionally graded cylindrical shell on the basis of modified couple stress theory. Compos Struct 120:65–78

    Google Scholar 

  34. Ansari R, Gholami R, Norouzzadeh A, Sahmani S (2015) Size-dependent vibration and instability of fluid-conveying functionally graded microshells based on the modified couple stress theory. Microfluid Nanofluid 19:509–522

    Google Scholar 

  35. Lou J, He L, Wu H, Du J (2016) Pre-buckling and buckling analyses of functionally graded microshells under axial and radial loads based on the modified couple stress theory. Compos Struct 142:226–237

    Google Scholar 

  36. Sheng GG, Wang X, Fu G, Hu H (2014) The nonlinear vibrations of functionally graded cylindrical shells surrounded by an elastic foundation. Nonlinear Dyn 78:1421–1434

    MathSciNet  Google Scholar 

  37. Sheng GG, Wang X (2018) Nonlinear vibrations of FG cylindrical shells subjected to parametric and external excitations. Compos Struct 191:78–88

    Google Scholar 

  38. Sheng GG, Wang X (2019) Nonlinear forced vibration of functionally graded Timoshenko microbeams with thermal effect and parametric excitation. Int J Mech Sci 155:405–416

    Google Scholar 

  39. Reddy JN (2004) Mechanics of laminated plates and shells, theory and analysis, 2nd edn. CRC Press, Boca Raton

    MATH  Google Scholar 

  40. Woo-Young J, Weon-Tae P, Sung-Cheon H (2014) Bending and vibration analysis of S-FGM microplates embedded in Pasternak elastic medium using the modified couple stress theory. Int J Mech Sci 87:150–162

    Google Scholar 

  41. Park KJ, Kim YW (2016) Vibration characteristics of fluid-conveying FGM cylindrical shells resting on Pasternak elastic foundation with an oblique edge. Thin Wall Struct 106:407–419

    Google Scholar 

  42. Pellicano F, Amabili M (2003) Stability and vibration of empty and fluid-filled circular cylindrical shells under static and periodic axial loads. Int J Solids Struct 40:3229–3251

    MATH  Google Scholar 

  43. Rougui M, Moussaoui F, Benamar R (2007) Geometrically non-linear free and forced vibrations of simply supported circular cylindrical shells: a semi-analytical approach. Int J Non-Linear Mech 42:1102–1115

    MATH  Google Scholar 

  44. Nayfeh AH, Mook DT (1979) Non-linear oscillation. Wiley, NewYork

    Google Scholar 

  45. Sarafraz A, Sahmani S, Aghdam MM (2019) Nonlinear secondary resonance of nanobeams under subharmonic and superharmonic excitations including surface free energy effects. Appl Math Model 66:195–226

    MathSciNet  MATH  Google Scholar 

  46. Javadi M, Noorian MA, Irani S (2019) Primary and secondary resonances in pipes conveying fluid with the fractional viscoelastic model. Meccanica 54:2081–2098

    MathSciNet  Google Scholar 

  47. Shah AG, Mahmood T, Naeem MN, Iqbal Z, Arshad SH (2010) Vibrations of functionally graded cylindrical shells within elastic foundations. Acta Mech 211:293–307

    MATH  Google Scholar 

  48. Sofiyev AH, Karaca Z, Zerin Z (2017) Non-linear vibration of composite orthotropic cylindrical shells on the non-linear elastic foundations within the shear deformation theory. Compos Struct 159:53–62

    Google Scholar 

  49. Paliwal DN, Pandey RK, Nath T (1996) Free vibrations of circular cylindrical shell on Winkler and Pasternak foundation. Int J Pres Ves Pip 69:79–89

    Google Scholar 

Download references

Acknowledgements

The authors thank the support of Natural Science Foundation of Hunan Province (CN) under no. 11JJ3013.

Author information

Authors and Affiliations

  1. School of Civil Engineering, Changsha University of Science and Technology, Changsha, 410114, Hunan, People’s Republic of China

    G. G. Sheng

  2. School of Naval Architecture, Ocean and Civil Engineering (State Key Laboratory of Ocean Engineering), Shanghai Jiaotong University, Shanghai, 200240, People’s Republic of China

    X. Wang

Authors
  1. G. G. Sheng
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. X. Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to G. G. Sheng.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Appendices

Appendix 1

$$(A_{ij} ,\;B_{ij} ,\;D_{ij} ) = \int_{{ - \frac{h}{2}}}^{{\frac{h}{2}}} {Q_{ij} } (1,z,z^{2} ){\text{d}}z\;(i,j = 1,2,6),\;E_{44} = \int_{{ - \frac{h}{2}}}^{{\frac{h}{2}}} {Q_{ 5 5} } {\text{d}}z,\;E_{55} = \int_{{ - \frac{h}{2}}}^{{\frac{h}{2}}} {Q_{ 4 4} } dz$$
(59)
$$\begin{aligned} \left\{ \begin{aligned} N_{x} \hfill \\ N_{\theta } \hfill \\ N_{x\theta } \hfill \\ M_{x} \hfill \\ M_{\theta } \hfill \\ M_{x\theta } \hfill \\ \end{aligned} \right\} &= \left[ {\begin{array}{*{20}c} {A_{11} } & {A_{12} } & 0 & {B_{11} } & {B_{12} } & 0 \\ {A_{21} } & {A_{22} } & 0 & {B_{21} } & {B_{22} } & 0 \\ 0 & 0 & {A_{66} } & 0 & 0 & {B_{66} } \\ {B_{11} } & {B_{12} } & 0 & {D_{11} } & {D_{12} } & 0 \\ {B_{21} } & {B_{22} } & 0 & {D_{21} } & {D_{22} } & 0 \\ 0 & 0 & {B_{66} } & 0 & 0 & {D_{66} } \\ \end{array} } \right]\left\{ \begin{aligned} \varepsilon_{x} \hfill \\ \varepsilon_{\theta } \hfill \\ \gamma_{x\theta } \hfill \\ \kappa_{x} \hfill \\ \kappa_{\theta } \hfill \\ \kappa_{x\theta } \hfill \\ \end{aligned} \right\} - \left\{ \begin{aligned} N_{x}^{T} \hfill \\ N_{\theta }^{T} \hfill \\ N_{x\theta }^{T} \hfill \\ M_{x}^{T} \hfill \\ M_{\theta }^{T} \hfill \\ M_{x\theta }^{T} \hfill \\ \end{aligned} \right\}, \hfill \\ \left\{ \begin{aligned} Q_{x} \hfill \\ Q_{\theta } \hfill \\ \end{aligned} \right\} &= \left[ {\begin{array}{*{20}c} {E_{44} } & 0 \\ 0 & {E_{55} } \\ \end{array} } \right]\left\{ {\begin{array}{*{20}c} {\gamma_{xz} } \\ {\gamma_{\theta z} } \\ \end{array} } \right\}, \hfill \\ \end{aligned}$$
(60)

where, the effective elasticity coefficients \(Q_{ij} (z)\) of the FG cylindrical microshell are given

$$\begin{aligned} Q_{11} = \varGamma_{11} ,\;Q_{12} = \varGamma_{12} /A,\;Q_{21} = \varGamma_{21} ,\;Q_{22} = \varGamma_{22} /A,\;Q_{66} = \varGamma_{66} /A \hfill \\ Q_{55} = \kappa_{G} \varGamma_{55} /A,\;Q_{44} = \kappa_{G} \varGamma_{44} ,\;A = 1 + z/R. \hfill \\ \end{aligned}$$
(61)

The shear correction factor is \(\kappa_{G} = \frac{5}{6}\) [39], and the nonzero stiffness coefficients are defined according to

$$\begin{aligned} \varGamma_{11} = \frac{{E_{\text{eff}} }}{{1 - \upsilon_{\text{eff}}^{2} }},\;\varGamma_{12} = \frac{{\nu_{\text{eff}} E_{\text{eff}} }}{{1 - \upsilon_{\text{eff}}^{2} }},\;\varGamma_{21} = \frac{{\upsilon_{\text{eff}} E_{\text{eff}} }}{{1 - \upsilon_{eff}^{2} }}, \hfill \\ \varGamma_{22} = \frac{{E_{\text{eff}} }}{{1 - \upsilon_{\text{eff}}^{2} }},\;\varGamma_{44} = \varGamma_{55} = \varGamma_{66} = \frac{{E_{\text{eff}} }}{{2(1 + \upsilon_{\text{eff}} )}}. \hfill \\ \end{aligned}$$
(62)

The thermal stress resultants

$$\left\{ {\begin{array}{*{20}c} {N_{x}^{T} } & {M_{x}^{T} } \\ {N_{\theta }^{T} } & {M_{\theta }^{T} } \\ {N_{x\theta }^{T} } & {M_{x\theta }^{T} } \\ \end{array} } \right\} = \int_{{ - \frac{h}{2}}}^{{\frac{h}{2}}} {\left\{ {\begin{array}{*{20}c} {Q_{11} (z)\alpha_{xxe} (z) + Q_{12} (z)\alpha_{\theta \theta e} (z)} \\ {Q_{12} (z)\alpha_{xxe} (z) + Q_{22} (z)\alpha_{\theta \theta e} (z)} \\ 0 \\ \end{array} } \right\}\Delta T(z)\left( {\begin{array}{*{20}c} 1 & z \\ \end{array} } \right)\text{d}z.}$$
(63)

The couple stress resultants can be defined in according to the higher-order stress \(m_{ij}\) (see Eq. (9))

$$\begin{aligned} (Y_{xx} ,Y_{\theta \theta } ,Y_{zz} ,Y_{x\theta } ,Y_{xz} ,Y_{\theta z} ) = \int_{{ - \frac{h}{2}}}^{{\frac{h}{2}}} {(m_{11} ,m_{22} ,m_{33} ,m_{12} ,m_{13} ,m_{23} )dz} \hfill \\ (T_{x\theta } ,T_{xz} ,T_{\theta z} ,T_{\theta \theta } ) = \int_{{ - \frac{h}{2}}}^{{\frac{h}{2}}} {(m_{12} ,m_{13} ,m_{23} ,m_{22} )z{\text{d}}z} . \hfill \\ \end{aligned}$$
(64)

Appendix 2

$$L_{11} = A_{11} \frac{{\partial^{2} }}{{\partial x^{2} }} + \frac{1}{{R^{2} }}A_{66} \frac{{\partial^{2} }}{{\partial \theta^{2} }},\;L_{12} = \frac{{A_{12} + A_{66} }}{R}\frac{{\partial^{2} }}{\partial x\partial \theta },\;L_{13} = \frac{{A_{12} }}{R}\frac{\partial }{\partial x},$$
$$L_{16} = L_{11} ,\;L_{14} = B_{11} \frac{{\partial^{2} }}{{\partial x^{2} }} + \frac{{B_{66} }}{{R^{2} }}\frac{{\partial^{2} }}{{\partial \theta^{2} }},\;L_{15} = \frac{{B_{12} + B_{66} }}{R}\frac{{\partial^{2} }}{\partial x\partial \theta },$$
$$L_{17} = \frac{{L_{12} }}{R},\;L_{21} = \frac{{A_{66} + A_{12} }}{R}\frac{{\partial^{2} }}{\partial x\partial \theta },\;L_{22} = A_{66} \frac{{\partial^{2} }}{{\partial x^{2} }} + \frac{{A_{22} }}{{R^{2} }}\frac{{\partial^{2} }}{{\partial \theta^{2} }} - \frac{{E_{55} }}{{R^{2} }},$$
$$L_{23} = \frac{{A_{22} + E_{55} }}{{R^{2} }}\frac{\partial }{\partial \theta },\;L_{24} = \frac{{B_{66} + B_{12} }}{R}\frac{{\partial^{2} }}{\partial x\partial \theta },\;L_{25} = B_{66} \frac{{\partial^{2} }}{{\partial x^{2} }} + \frac{{B_{22} }}{{R^{2} }}\frac{{\partial^{2} }}{{\partial \theta^{2} }} + \frac{{E_{55} }}{R},$$
$$L_{26} = L_{21} ,\;L_{27} = \frac{{A_{66} }}{R}\frac{{\partial^{2} }}{{\partial x^{2} }} + \frac{{A_{22} }}{{R^{3} }}\frac{{\partial^{2} }}{{\partial \theta^{2} }},\;L_{31} = - \frac{{A_{21} }}{R}\frac{\partial }{\partial x},\;L_{32} = - \frac{{E_{55} }}{{R^{2} }}\frac{\partial }{\partial \theta } - \frac{{A_{22} }}{{R^{2} }}\frac{\partial }{\partial \theta },$$
$$L_{33} = E_{44} \frac{{\partial^{2} }}{{\partial x^{2} }} + \frac{{E_{55} }}{{R^{2} }}\frac{{\partial^{2} }}{{\partial \theta^{2} }} - \frac{{A_{22} }}{{R^{2} }},\;L_{34} = E_{44} \frac{\partial }{\partial x} - \frac{{B_{21} }}{R}\frac{\partial }{\partial x},\;L_{35} = \frac{{E_{55} }}{R}\frac{\partial }{\partial \theta } - \frac{{B_{22} }}{{R^{2} }}\frac{\partial }{\partial \theta },$$
$$L_{36} = - \frac{{A_{21} }}{2R}\frac{\partial }{\partial x},\;L_{37} = - \frac{{A_{22} }}{{2R^{3} }}\frac{\partial }{\partial \theta },\;L_{41} = B_{11} \frac{{\partial^{2} }}{{\partial x^{2} }} + \frac{{B_{66} }}{{R^{2} }}\frac{{\partial^{2} }}{{\partial \theta^{2} }},\;L_{42} = \frac{{B_{12} + B_{66} }}{R}\frac{{\partial^{2} }}{\partial x\partial \theta },$$
$$L_{43} = \left( {\frac{{B_{12} }}{R} - E_{44} } \right)\frac{\partial }{\partial x},\;L_{44} = D_{11} \frac{{\partial^{2} }}{{\partial x^{2} }} + \frac{{D_{66} }}{{R^{2} }}\frac{{\partial^{2} }}{{\partial \theta^{2} }} - E_{44} ,\;L_{45} = \frac{{D_{12} + D_{66} }}{R}\frac{{\partial^{2} }}{\partial x\partial \theta },$$
$$L_{46} = L_{41} ,\;L_{47} = \frac{{L_{42} }}{R},\;L_{51} = \frac{{B_{66} + B_{12} }}{R}\frac{{\partial^{2} }}{\partial x\partial \theta },\;L_{52} = B_{66} \frac{{\partial^{2} }}{{\partial x^{2} }} + \frac{{B_{22} }}{{R^{2} }}\frac{{\partial^{2} }}{{\partial \theta^{2} }} + \frac{{E_{55} }}{R},$$
$$L_{53} = \frac{{B_{22} - E_{55} R}}{{R^{2} }}\frac{\partial }{\partial \theta },\;L_{54} = \frac{{D_{12} + D_{66} }}{R}\frac{{\partial^{2} }}{\partial x\partial \theta },\;L_{55} = \frac{{D_{22} }}{{R^{2} }}\frac{{\partial^{2} }}{{\partial \theta^{2} }} - E_{55} + D_{66} \frac{{\partial^{2} }}{{\partial x^{2} }},$$
$$L_{56} = L_{51} \;L_{57} = \frac{{B_{66} }}{R}\frac{{\partial^{2} }}{{\partial x^{2} }} + \frac{{B_{22} }}{{R^{2} }}\frac{{\partial^{2} }}{{\partial \theta^{2} }}.$$
$$\mu_{0} = \int_{{ - \frac{h}{2}}}^{{\frac{h}{2}}} {\mu (z){\text{d}}z} ,\;\mu_{1} = \int_{{ - \frac{h}{2}}}^{{\frac{h}{2}}} {\mu (z)z{\text{d}}z} ,\;\mu_{2} = \int_{{ - \frac{h}{2}}}^{{\frac{h}{2}}} {\mu (z)z^{2} {\text{d}}z} ,$$
$$L_{11}^{l} = \frac{{\mu_{0} }}{{R^{4} }}\frac{{\partial^{2} }}{{\partial \theta^{2} }} - \frac{{\mu_{0} }}{{4R^{2} }}\frac{{\partial^{4} }}{{\partial x^{2} \partial \theta^{2} }} - \frac{{\mu_{0} }}{{4R^{4} }}\frac{{\partial^{4} }}{{\partial \theta^{4} }},$$
$$L_{12}^{l} = - \frac{{\mu_{0} }}{{4R^{3} }}\frac{{\partial^{2} }}{\partial x\partial \theta } + \frac{{\mu_{0} }}{4R}\frac{{\partial^{4} }}{{\partial x^{3} \partial \theta }} + \frac{{\mu_{0} }}{{4R^{3} }}\frac{{\partial^{4} }}{{\partial x\partial \theta^{3} }},$$
$$L_{13}^{l} = \frac{{\mu_{0} }}{{2R^{3} }}\frac{{\partial^{3} }}{{\partial x\partial \theta^{2} }},\;L_{14}^{l} = - \frac{{5\mu_{0} }}{{4R^{3} }}\frac{{\partial^{2} }}{{\partial \theta^{2} }} - \frac{{\mu_{1} }}{{4R^{2} }}\frac{{\partial^{4} }}{{\partial x^{2} \partial \theta^{2} }} - \frac{{\mu_{1} }}{{4R^{4} }}\frac{{\partial^{4} }}{{\partial \theta^{4} }},$$
$$L_{15}^{l} = \left( {\frac{{\mu_{0} }}{{4R^{2} }} - \frac{{\mu_{1} }}{{2R^{3} }}} \right)\frac{{\partial^{2} }}{\partial x\partial \theta } + \frac{{\mu_{1} }}{{4R^{3} }}\frac{{\partial^{4} }}{{\partial x\partial \theta^{3} }} + \frac{{\mu_{1} }}{4R}\frac{{\partial^{4} }}{{\partial x^{3} \partial \theta }},$$
$$L_{21}^{l} = - \frac{{\mu_{0} }}{{4R^{3} }}\frac{{\partial^{2} }}{\partial x\partial \theta } + \frac{{\mu_{0} }}{{4R^{3} }}\frac{{\partial^{4} }}{{\partial x\partial \theta^{3} }} + \frac{{\mu_{0} }}{4R}\frac{{\partial^{4} }}{{\partial x^{3} \partial \theta }},$$
$$L_{22}^{l} = - \frac{{\mu_{0} }}{{4R^{4} }} + \frac{{\mu_{0} }}{{2R^{2} }}\frac{{\partial^{2} }}{{\partial x^{2} }} + \frac{{\mu_{0} }}{{4R^{4} }}\frac{{\partial^{2} }}{{\partial \theta^{2} }} - \frac{{\mu_{0} }}{{4R^{2} }}\frac{{\partial^{4} }}{{\partial x^{2} \partial \theta^{2} }} - \frac{{\mu_{0} }}{4}\frac{{\partial^{4} }}{{\partial x^{4} }},$$
$$L_{23}^{l} = \frac{{\mu_{0} }}{{4R^{4} }}\frac{\partial }{\partial \theta } - \frac{{3\mu_{0} }}{{4R^{2} }}\frac{{\partial^{3} }}{{\partial x^{2} \partial \theta }} - \frac{{\mu_{0} }}{{4R^{4} }}\frac{{\partial^{3} }}{{\partial \theta^{3} }},$$
$$L_{24}^{l} = \frac{{\mu_{0} }}{{2R^{2} }}\frac{{\partial^{2} }}{\partial x\partial \theta } + \frac{{\mu_{1} }}{{4R^{3} }}\frac{{\partial^{2} }}{\partial x\partial \theta } + \frac{{\mu_{1} }}{{4R^{3} }}\frac{{\partial^{4} }}{{\partial x\partial \theta^{3} }} + \frac{{\mu_{1} }}{4R}\frac{{\partial^{4} }}{{\partial x^{3} \partial \theta }},$$
$$L_{25}^{l} = \frac{{\mu_{0} }}{{4R^{3} }} + \frac{{3\mu_{0} }}{4R}\frac{{\partial^{2} }}{{\partial x^{2} }} + \frac{{\mu_{1} }}{{4R^{2} }}\frac{{\partial^{2} }}{{\partial x^{2} }} + \frac{{\mu_{0} }}{{4R^{3} }}\frac{{\partial^{2} }}{{\partial \theta^{2} }} - \frac{{\mu_{1} }}{{4R^{2} }}\frac{{\partial^{4} }}{{\partial x^{2} \partial \theta^{2} }} - \frac{{\mu_{1} }}{4}\frac{{\partial^{4} }}{{\partial x^{4} }},$$
$$L_{31}^{l} = - \frac{{\mu_{0} }}{{2R^{3} }}\frac{{\partial^{3} }}{{\partial x\partial \theta^{2} }},\;L_{32}^{l} = - \frac{{\mu_{0} }}{{4R^{4} }}\frac{\partial }{\partial \theta } + \frac{{3\mu_{0} }}{{4R^{2} }}\frac{{\partial^{3} }}{{\partial x^{2} \partial \theta }} + \frac{{\mu_{0} }}{{4R^{4} }}\frac{{\partial^{3} }}{{\partial \theta^{3} }},$$
$$L_{33}^{l} = \frac{{\mu_{0} }}{{4R^{2} }}\frac{{\partial^{2} }}{{\partial x^{2} }} + \frac{{\mu_{0} }}{{4R^{4} }}\frac{{\partial^{2} }}{{\partial \theta^{2} }} - \frac{{\mu_{0} }}{{2R^{2} }}\frac{{\partial^{4} }}{{\partial x^{2} \partial \theta^{2} }} - \frac{{\mu_{0} }}{4}\frac{{\partial^{4} }}{{\partial x^{4} }} - \frac{{\mu_{0} }}{{4R^{4} }}\frac{{\partial^{4} }}{{\partial \theta^{4} }},$$
$$L_{34}^{l} = - \frac{{\mu_{0} }}{{4R^{2} }}\frac{\partial }{\partial x} + \frac{{\mu_{0} }}{{4R^{2} }}\frac{{\partial^{3} }}{{\partial x\partial \theta^{2} }} + \frac{{\mu_{0} }}{4}\frac{{\partial^{3} }}{{\partial x^{3} }},$$
$$L_{35}^{l} = \frac{{\mu_{0} }}{{4R^{3} }}\frac{\partial }{\partial \theta } + \frac{{\mu_{0} }}{4R}\frac{{\partial^{3} }}{{\partial x^{2} \partial \theta }} + \frac{{\mu_{1} }}{{2R^{2} }}\frac{{\partial^{3} }}{{\partial x^{2} \partial \theta }} + \frac{{\mu_{0} }}{{4R^{3} }}\frac{{\partial^{3} }}{{\partial \theta^{3} }},$$
$$L_{41}^{l} = - \frac{{5\mu_{0} }}{{4R^{3} }}\frac{{\partial^{2} }}{{\partial \theta^{2} }} - \frac{{\mu_{1} }}{{4R^{2} }}\frac{{\partial^{4} }}{{\partial x^{2} \partial \theta^{2} }} - \frac{{\mu_{1} }}{{4R^{4} }}\frac{{\partial^{4} }}{{\partial \theta^{4} }},$$
$$L_{42}^{l} = \frac{{\mu_{0} }}{{2R^{2} }}\frac{{\partial^{2} }}{\partial x\partial \theta } + \frac{{\mu_{1} }}{{4R^{3} }}\frac{{\partial^{2} }}{\partial x\partial \theta } + \frac{{\mu_{1} }}{{4R^{3} }}\frac{{\partial^{4} }}{{\partial x\partial \theta^{3} }} + \frac{{\mu_{1} }}{4R}\frac{{\partial^{4} }}{{\partial x^{3} \partial \theta }},$$
$$L_{43}^{l} = \frac{{\mu_{0} }}{{4R^{2} }}\frac{\partial }{\partial x} - \frac{{\mu_{0} }}{{4R^{2} }}\frac{{\partial^{3} }}{{\partial x\partial \theta^{2} }} - \frac{{\mu_{0} }}{4}\frac{{\partial^{3} }}{{\partial x^{3} }},$$
$$L_{44}^{l} = - \frac{{\mu_{0} }}{{4R^{2} }} + \frac{{\mu_{0} }}{4}\frac{{\partial^{2} }}{{\partial x^{2} }} + \frac{{\mu_{0} }}{{R^{2} }}\frac{{\partial^{2} }}{{\partial \theta^{2} }} - \frac{{\mu_{1} }}{{2R^{3} }}\frac{{\partial^{2} }}{{\partial \theta^{2} }} - \frac{{\mu_{2} }}{{4R^{2} }}\frac{{\partial^{4} }}{{\partial x^{2} \partial \theta^{2} }} - \frac{{\mu_{2} }}{{4R^{4} }}\frac{{\partial^{4} }}{{\partial \theta^{4} }},$$
$$L_{45}^{l} = - \frac{{3\mu_{0} }}{4R}\frac{{\partial^{2} }}{\partial x\partial \theta } + \frac{{\mu_{1} }}{{2R^{2} }}\frac{{\partial^{2} }}{\partial x\partial \theta } + \frac{{\mu_{2} }}{{4R^{3} }}\frac{{\partial^{4} }}{{\partial x\partial \theta^{3} }} + \frac{{\mu_{2} }}{4R}\frac{{\partial^{4} }}{{\partial x^{3} \partial \theta }},$$
$$L_{51}^{l} = \frac{{\mu_{0} }}{{4R^{2} }}\frac{{\partial^{2} }}{\partial x\partial \theta } - \frac{{\mu_{1} }}{{2R^{3} }}\frac{{\partial^{2} }}{\partial x\partial \theta } + \frac{{\mu_{1} }}{{4R^{3} }}\frac{{\partial^{4} }}{{\partial x\partial \theta^{3} }} + \frac{{\mu_{1} }}{4R}\frac{{\partial^{4} }}{{\partial x^{3} \partial \theta }},$$
$$L_{52}^{l} = \frac{{\mu_{0} }}{{4R^{3} }} + \frac{{3\mu_{0} }}{4R}\frac{{\partial^{2} }}{{\partial x^{2} }} + \frac{{\mu_{1} }}{{4R^{2} }}\frac{{\partial^{2} }}{{\partial x^{2} }} + \frac{{\mu_{0} }}{{4R^{3} }}\frac{{\partial^{2} }}{{\partial \theta^{2} }} - \frac{{\mu_{1} }}{{4R^{2} }}\frac{{\partial^{4} }}{{\partial x^{2} \partial \theta^{2} }} - \frac{{\mu_{1} }}{4}\frac{{\partial^{4} }}{{\partial x^{4} }},$$
$$L_{53}^{l} = - \frac{{\mu_{0} }}{{4R^{3} }}\frac{\partial }{\partial \theta } - \frac{{\mu_{0} }}{4R}\frac{{\partial^{3} }}{{\partial x^{2} \partial \theta }} - \frac{{2\mu_{1} }}{{4R^{2} }}\frac{{\partial^{3} }}{{\partial x^{2} \partial \theta }} - \frac{{\mu_{0} }}{{4R^{3} }}\frac{{\partial^{3} }}{{\partial \theta^{3} }},$$
$$L_{54}^{l} = - \frac{{3\mu_{0} }}{4R}\frac{{\partial^{2} }}{\partial x\partial \theta } + \frac{{\mu_{1} }}{{2R^{2} }}\frac{{\partial^{2} }}{\partial x\partial \theta } + \frac{{\mu_{2} }}{{4R^{3} }}\frac{{\partial^{4} }}{{\partial x\partial \theta^{3} }} + \frac{{\mu_{2} }}{4R}\frac{{\partial^{4} }}{{\partial x^{3} \partial \theta }},$$
$$L_{55}^{l} = - \frac{{\mu_{0} }}{{4R^{2} }} + \mu_{0} \frac{{\partial^{2} }}{{\partial x^{2} }} + \frac{{\mu_{1} }}{2R}\frac{{\partial^{2} }}{{\partial x^{2} }} + \frac{{\mu_{2} }}{{2R^{2} }}\frac{{\partial^{2} }}{{\partial x^{2} }} + \frac{{\mu_{0} }}{{4R^{2} }}\frac{{\partial^{2} }}{{\partial \theta^{2} }} - \frac{{\mu_{2} }}{{4R^{2} }}\frac{{\partial^{4} }}{{\partial x^{2} \partial \theta^{2} }} - \frac{{\mu_{2} }}{4}\frac{{\partial^{4} }}{{\partial x^{4} }}.$$

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Sheng, G.G., Wang, X. Nonlinear resonance responses of size-dependent functionally graded cylindrical microshells with thermal effect and elastic medium. Engineering with Computers 38 (Suppl 1), 725–742 (2022). https://doi.org/10.1007/s00366-020-01176-8

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00366-020-01176-8

Keywords

Search

Navigation