Abstract
We consider social choice problems where the admissible set of preferences of each agent is single-peaked. First, we show that if all the agents have the same admissible set of preferences, then every unanimous and strategy-proof social choice function (SCF) is tops-only. Next, we consider situations where different agents have different admissible sets of single-peaked preferences. We show by means of an example that unanimous and strategy-proof SCFs need not be tops-only in this situation, and consequently provide a sufficient condition on the admissible sets of preferences of the agents so that unanimity and strategy-proofness guarantee tops-onlyness. Finally, we characterize all domains on which (i) every unanimous and strategy-proof SCF is a min–max rule, and (ii) every min–max rule is strategy-proof. As an application of our result, we obtain a characterization of the unanimous and strategy-proof social choice functions on maximal single-peaked domains (Moulin in Public Choice 35(4):437–455. https://doi.org/10.1007/BF00128122, 1980; Weymark in SERIEs 2(4):529–550. https://doi.org/10.1007/s13209-011-0064-5, 2011), minimally rich single-peaked domains (Peters et al. in J Math Econ 52:123–127. https://doi.org/10.1016/j.jmateco.2014.03.008. http://www.sciencedirect.com/science/article/pii/S0304406814000470, 2014), maximal regular single-crossing domains (Saporiti in Theor Econ 4(2):127–163, 2009, J Econ Theory 154:216–228. https://doi.org/10.1016/j.jet.2014.09.006. http://www.sciencedirect.com/science/article/pii/S0022053114001276, 2009), and distance based single-peaked domains.

Similar content being viewed by others
Notes
See, for instance, the domain restriction considered in models of voting (Tullock 1967; Arrow 1969), taxation and redistribution (Epple and Romer 1991), determining the levels of income redistribution (Hamada 1973; Slesnick 1988), and measuring tax reforms in the presence of horizontal inequity (Hettich 1979). Recently, Puppe (2018) shows that under mild conditions these domains form subsets of the maximal single-peaked domain.
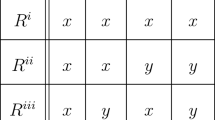
Chatterji and Sen (2011) provide a sufficient condition on a domain so that every unanimous and strategy-proof SCF on it is tops-only. However, an arbitrary single-peaked domain does not satisfy their condition.
A domain is regular if every alternative appears as the top-ranked alternative of some preference in the domain.
Single-crossing domains appear in models of taxation and redistribution (Roberts 1977; Meltzer and Richard 1981), local public goods and stratification (Westhoff 1977; Epple and Platt 1998; Epple et al. 2001), coalition formation (Demange 1994; Kung 2006), selecting constitutional and voting rules (Barberà and Jackson 2004), and designing policies in the market for higher education (Epple et al. 2006).
Saporiti (2014) provides a different but equivalent functional form of these SCFs which he calls augmented representative voter schemes.
A single-peaked preference is called left (or right) single-peaked if every alternative to the left (or right) of the peak is preferred to every alternative to its right (or left).
For details, see Weymark (2011).
A median rule is a min–max rule that is anonymous. More formally, a min–max rule with respect to parameters \((\beta _S)_{S \subseteq N}\) is a median rule if \(\beta _S=\beta _{\bar{S}}\) for all \(S,\bar{S} \subseteq N\) with \(|S|=|\bar{S}|\). For details see Moulin (1980).
Since \(f(P'_{i},P_{N {\setminus } i}) = y'\), if \(r_{1}(P'_i) \ne y'\), then by strategy-proofness, \(f(P''_{i},P_{N {\setminus } i}) = y'\) for some \(P''_{i} \in \mathcal {S}_{i}\) with \(r_{1}(P''_{i}) = y'\). Similarly, if \(r_{1}(P_{j}) < y'\) for some \(j \in N {\setminus } i\), then by strategy-proofness, \(f(P'_{i},P'_{j},P_{N {\setminus } \{i,j\}}) = y'\) for some \(P'_{j} \in \mathcal {S}_{j}\) with \(r_{1}(P'_{j}) = y'\).
References
Arribillaga RP, Massó J (2016) Comparing generalized median voter schemes according to their manipulability. Theor Econ 11(2):547–586. https://doi.org/10.3982/TE1910
Arrow KJ (1969) Tullock and an existence theorem. Public Choice 6:105–111. http://www.jstor.org/stable/30022601
Aswal N, Chatterji S, Sen A (2003) Dictatorial domains. Econ Theory 22(1):45–62. https://doi.org/10.1007/s00199-002-0285-8
Barberà S, Jackson MO (2004) Choosing how to choose: Self-stable majority rules and constitutions. Q J Econ 119(3):1011–1048. http://www.jstor.org/stable/25098708
Barberà S, Peleg B (1990) Strategy-proof voting schemes with continuous preferences. Soc Choice Welf 7(1):31–38. https://doi.org/10.1007/BF01832918
Barberà S, Gul F, Stacchetti E (1993) Generalized median voter schemes and committees. J Econ Theory 61(2):262–289. https://doi.org/10.1006/jeth.1993.1069. http://www.sciencedirect.com/science/article/pii/S0022053183710690
Barberà S, Massò J, Neme A (1999) Maximal domains of preferences preserving strategy-proofness for generalized median voter schemes. Soc Choice Welf 16(2):321–336. https://doi.org/10.1007/s003550050146
Barberà S, Berga D, Moreno B (2010) Individual versus group strategy-proofness: when do they coincide? J Econ Theory 145(5):1648–1674. https://doi.org/10.1016/j.jet.2010.03.006. http://www.sciencedirect.com/science/article/pii/S0022053110000566
Black D (1948) On the rationale of group decision-making. J Political Econ 56(1):23–34. http://www.jstor.org/stable/1825026
Chatterji S, Sen A (2011) Tops-only domains. Econ Theory 46(2):255–282. https://doi.org/10.1007/s00199-009-0509-2
Chatterji S, Zeng H (2018) On random social choice functions with the tops-only property. Games Econ Behav 109:413–435. https://doi.org/10.1016/j.geb.2017.11.011. http://www.sciencedirect.com/science/article/pii/S0899825617302427
Chatterji S, Sen A, Zeng H (2014) Random dictatorship domains. Games Econ Behav 86:212–236. https://doi.org/10.1016/j.geb.2014.03.017. http://www.sciencedirect.com/science/article/pii/S0899825614000700
Ching S (1997) Strategy-proofness and “median voters”. Int J Game Theory 26(4):473–490. https://doi.org/10.1007/BF01813886
Demange G (1982) Single-peaked orders on a tree. Math Soc Sci 3(4):389–396. https://doi.org/10.1016/0165-4896(82)90020-8
Demange G (1994) Intermediate preferences and stable coalition structures. J Math Econ 23(1):45–58. https://doi.org/10.1016/0304-4068(94)90035-3
Elkind E, Faliszewski P, Skowron P (2014) A characterization of the single-peaked single-crossing domain. In: AAAI, vol 14, pp 654–660
Epple D, Platt GJ (1998) Equilibrium and local redistribution in an urban economy when households differ in both preferences and incomes. J Urban Econ 43(1):23–51. https://doi.org/10.1006/juec.1996.2030. http://www.sciencedirect.com/science/article/pii/S0094119096920306
Epple D, Romer T (1991) Mobility and redistribution. J Political Econ 99(4):828–858. http://www.jstor.org/stable/2937782
Epple D, Romer T, Sieg H (2001) Interjurisdictional sorting and majority rule: an empirical analysis. Econometrica 69(6):1437–1465. https://doi.org/10.1111/1468-0262.00253
Epple D, Romano R, Sieg H (2006) Admission, tuition, and financial aid policies in the market for higher education. Econometrica 74(4):885–928. https://doi.org/10.1111/j.1468-0262.2006.00690.x
Gibbard A (1973) Manipulation of voting schemes: a general result. Econometrica 41(4):587–601. http://www.jstor.org/stable/1914083
Hamada K (1973) A simple majority rule on the distribution of income. J Econ Theory 6(3):243–264. https://doi.org/10.1016/0022-0531(73)90049-5
Hettich W (1979) A theory of partial tax reform. Can J Econ Revue canadienne d’Economique 12(4):692–712. http://www.jstor.org/stable/134874
Hotelling H (1929) Stability in competition. Econ J 39:41–57
Kung FC (2006) An algorithm for stable and equitable coalition structures with public goods. J Public Econ Theory 8(3):345–355. https://doi.org/10.1111/j.1467-9779.2006.00267.x
Meltzer AH, Richard SF (1981) A rational theory of the size of government. J Political Econ 89(5):914–927. http://www.jstor.org/stable/1830813
Moulin H (1980) On strategy-proofness and single peakedness. Public Choice 35(4):437–455. https://doi.org/10.1007/BF00128122
Nehring K, Puppe C (2007) The structure of strategy-proof social choice part i: general characterization and possibility results on median spaces. J Econ Theory 135(1):269–305. https://doi.org/10.1016/j.jet.2006.04.008. http://www.sciencedirect.com/science/article/pii/S0022053106001050
Nehring K, Puppe C (2007) Efficient and strategy-proof voting rules: a characterization. Games Econ Behav 59(1):132–153. https://doi.org/10.1016/j.geb.2006.05.002. http://www.sciencedirect.com/science/article/pii/S0899825606000674
Peters H, Roy S, Sen A, Storcken T (2014) Probabilistic strategy-proof rules over single-peaked domains. J Math Econ 52:123–127. https://doi.org/10.1016/j.jmateco.2014.03.008. http://www.sciencedirect.com/science/article/pii/S0304406814000470
Puppe C (2018) The single-peaked domain revisited: a simple global characterization. J Econ Theory 176:55–80. https://doi.org/10.1016/j.jet.2018.03.003. http://www.sciencedirect.com/science/article/pii/S0022053118300863
Rabinowitz G (1978) On the nature of political issues: insights from a spatial analysis. Am J Political Sci 22(4):793–817. http://www.jstor.org/stable/2110591
Rabinowitz G, Macdonald SE (1989) A directional theory of issue voting. Am Political Sci Rev 83(1):93–121. https://doi.org/10.2307/1956436
Rabinowitz G, Prothro JW, Jacoby W (1982) Salience as a factor in the impact of issues on candidate evaluation. J Politics 44(1):41–63. https://doi.org/10.2307/2130283
Roberts KW (1977) Voting over income tax schedules. J Public Econ 8(3):329–340. https://doi.org/10.1016/0047-2727(77)90005-6
Romer T, Rosenthal H (1979) Bureaucrats versus voters: on the political economy of resource allocation by direct democracy. Q J Econ 93(4):563–587. http://www.jstor.org/stable/1884470
Roy S, Storcken T (2016) Unanimity, pareto optimality and strategy-proofness on connected domains. Working Paper
Saporiti A (2009) Strategy-proofness and single-crossing. Theor Econ 4(2):127–163
Saporiti A (2014) Securely implementable social choice rules with partially honest agents. J Econ Theory 154:216–228. https://doi.org/10.1016/j.jet.2014.09.006. http://www.sciencedirect.com/science/article/pii/S0022053114001276
Satterthwaite MA (1975) Strategy-proofness and arrow’s conditions: existence and correspondence theorems for voting procedures and social welfare functions. J Econ Theory 10(2):187–217. https://doi.org/10.1016/0022-0531(75)90050-2
Schummer J, Vohra RV (2002) Strategy-proof location on a network. J Econ Theory 104(2):405–428. https://doi.org/10.1006/jeth.2001.2807. http://www.sciencedirect.com/science/article/pii/S0022053101928078
Slesnick D (1988) The political economy of redistribution policy. Unpublished University of Texas Working Paper
Stokes DE (1963) Spatial models of party competition. Am Political Sci Rev 57(2):368–377. https://doi.org/10.2307/1952828
Tullock G (1967) The general irrelevance of the general impossibility theorem. Q J Econ 81(2):256–270. http://www.jstor.org/stable/1879585
Westhoff F (1977) Existence of equilibria in economies with a local public good. J Econ Theory 14(1):84–112. https://doi.org/10.1016/0022-0531(77)90086-2
Weymark JA (2011) A unified approach to strategy-proofness for single-peaked preferences. SERIEs 2(4):529–550. https://doi.org/10.1007/s13209-011-0064-5
Acknowledgements
The authors would like to thank the Associate Editor and two anonymous referees for their insightful comments which significantly improved the results of this paper. Further, we would like to gratefully acknowledge Salvador Barberà, Somdatta Basak, Shurojit Chatterji, Indraneel Dasgupta, Jordi Massó, Debasis Mishra, Manipushpak Mitra, Hans Peters, Soumyarup Sadhukhan, Arunava Sen, Shigehiro Serizawa, Ton Storcken, John Weymark, and Huaxia Zeng for their invaluable suggestions which helped improve this paper. We are also thankful to the seminar audience of the International Conclave on Foundations of Decision and Game Theory, 2016 (held at the Indira Gandhi Institute of Development Research, Mumbai during March 14–19, 2016), the 13th Meeting of the Society for Social Choice and Welfare (held at Lund, Sweden during June 28–July 1, 2016) and the 11th Annual Winter School of Economics, 2016 (held at the Delhi School of Economics, New Delhi during December 13–15, 2016) for their helpful comments. The usual disclaimer holds.
Author information
Authors and Affiliations
Corresponding author
Appendix
Appendix
1.1 Proof of Theorem 2
Proof
Let \(P_{N} \in \mathcal {S}_{N}\), \(i \in N\), and \(P'_{i} \in \mathcal {S}\) be such that \(r_{1}(P_{i}) = r_{1}(P'_{i})\). It is enough to show that \(f(P_{N}) = f(P'_{i},P_{N {\setminus } i})\). Suppose not. Let \(r_{1}(P_{i}) = r_{1}(P'_{i}) = x\), \(f(P_{N}) = z\), and \(f(P'_{i},P_{N {\setminus } i}) = y\). By strategy-proofness, \(z P_{i} y\) and \(y P'_{i} z\). Since \(\mathcal {S}_{i}\) is single-peaked and \(r_{1}(P_{i}) = r_{1}(P'_{i})\), this means either \(y< x < z\) or \(z< x < y\). Assume without loss of generality that \(y< x < z\). Let \(\bar{N} = \{j \in N \mid r_{1}(P_{j}) \ge x\}\) and let \(\bar{P}_{N} \in \mathcal {S}_{N}\) be such that \(\bar{P}_{j} = P_{i}\) for all \(j \in \bar{N}\), and \(\bar{P}_{j} = P_{j}\) for all \(j \notin \bar{N}\).
Claim 1. \(f(P'_{i},P_{N {\setminus } i}) = y\) implies \(f(\bar{P}_{N}) = y\).
By Pareto optimality, \(f(\bar{P}_{N}) \le x\). If \(f(\bar{P}_{N}) \in (y,x]\) then agents in \(\bar{N}\) manipulates f at \((P'_{i},P_{N {\setminus } i})\) via \(\bar{P}_{N}\). On the other hand, if \(f(\bar{P}_{N}) < y\), then agents in \(\bar{N}\) manipulate f at \(\bar{P}_{N}\) via \((P'_{i},P_{N {\setminus } i})\). This completes the proof of Claim 1.
Claim 2. \(f(P_{N}) = z\) implies \(f(\bar{P}_{N}) \ne y\).
Because \(z \bar{P}_{j} y\) for all \(j \in \bar{N}\), if \(f(\bar{P}_{N}) = y\), then agents in \(\bar{N}\) manipulates f at \(\bar{P}_{N}\) via \(P_{N}\). This completes the proof of Claim 2.
However, Claim 2 contradicts Claim 1. This completes the proof of the theorem. \(\square \)
1.2 Proof of Theorem 3
Proof
We prove the theorem for the case where \(\mathcal {S}_{N}\) is right-connected, the proof is the same for the case where \(\mathcal {S}_{N}\) is left-connected.
It is sufficient to show that \(f(P_{N})=f(P'_{i},P_{N {\setminus } i})\) for all \(P_{i},P'_{i} \in \mathcal {S}_{i}\) and all \(P_{N {\setminus } i} \in \mathcal {S}_{N {\setminus } i}\) with \(r_{1}(P_{i}) = r_{1}(P'_{i})\). Assume for contradiction, \(f(P_{N}) \ne f(P'_{i},P_{N {\setminus } i})\). By strategy-proofness, we have \(f(P_{N}) P_{i} f(P'_{i},P_{N {\setminus } i})\) and \(f(P'_{i},P_{N {\setminus } i})P'_{i} f(P_{N})\). Assume without loss of generality, \(f(P'_{i},P_{N {\setminus } i})< r_{1}(P_{i}) < f(P_{N})\). Suppose \(f(P'_{i},P_{N {\setminus } i}) = y\), \(r_{1}(P_{i}) = x\), and \(f(P_{N}) = z\). Let \(N_{1} = \{j \in N \mid r_{1}(P_{j}) \ge z\}\). By Pareto optimality, \(N_{1} \ne \emptyset \). Consider \(P^{1}_{N} \in \mathcal {S}_{N}\) such that \(r_{1}(P^{1}_{j}) = z-1\) and \(r_{2}(P^{1}_{j}) = z\) for all \(j \in N_{1}\), and \(P^{1}_{k} = P_{k}\) for all \(k \notin N_{1}\). By group-strategy-proofness, \(f(P^{1}_{N}) \in \{z,z-1\}\). By Pareto optimality, \(f(P^{1}_{N}) \le z-1\). Combining, \(f(P^{1}_{N}) = z-1\). Now, let \(N_{2} = \{j \in N \mid r_{1}(P_{j}) = z-1\}\). Consider \(P^{2}_{N} \in \mathcal {S}_{N}\) such that \(r_{1}(P^{2}_{j}) = z-2\) and \(r_{2}(P^{2}_{j}) = z-1\) for all \(j \in N_{2}\), and \(P^{2}_{k} = P_{k}\) for all \(k \notin N_{2}\). Using similar logic as before, \(f(P^{2}_{N}) = z-2\). Continuing in this manner, we construct a profile \(\hat{P}_{N} \in \mathcal {S}_{N}\) such that \(r_{1}(\hat{P}_{j}) = x\) for all \(j \in N\) with \(r_{1}(P_{j}) \ge x\), \(\hat{P}_{k} = P_{k}\) for all \(k \in N\) such that \(r_{1}(\hat{P}_{k}) < x\), and \(f(\hat{P}_{N}) = x\).
Now, consider the profile \(\bar{P}_{N} \in \mathcal {S}_{N}\) such that \(\bar{P}_{i} = P'_{i}\), \(\bar{P}_{k} = P_{k}\) if \(k \in N\) such that \(r_{1}(P_{k}) < x\), and \(r_{1}(\bar{P}_{k}) = x\) if \(k \in N {\setminus } i\) such that \(r_{1}(P_{k}) \ge x\). By Pareto optimality, \(f(\bar{P}_{N}) \le x\). This, together with group-strategy-proofness from \((P'_{i},P_{N {\setminus } i})\) to \(\bar{P}_{N}\), implies \(f(\bar{P}_{N}) \ge y\). However, by group-strategy-proofness from \(\bar{P}_{N}\) to \((P'_{i},P_{N {\setminus } i})\), we \(f(\bar{P}_{N}) \le y\). Combining, \(f(\bar{P}_{N}) = y\).
Note that \(\hat{P}_{j} = \bar{P}_{j}\) for all \(j \in N\) with \(r_{1}(P_{j}) < x\) and \(r_{1}(\hat{P}_{j}) = r_{1}(\bar{P}_{j})\) for all \(j \in N\) with \(r_{1}(P_{j}) \ge x\). This means the group of agents \(\{j \in N \mid r_{1}(\bar{P}_{j}) = x\}\) manipulates at \(\bar{P}_{N}\) via \(\hat{P}_{N}\), a contradiction. This completes the proof of the theorem. \(\square \)
1.3 Proof of Theorem 4
Proof
(If part) Note that a min–max rule is unanimous by definition (on any domain). We show that such a rule is strategy-proof on \(\mathcal {S}_{N}\). For all \(i \in N\), let \(\bar{\mathcal {S}}_i\) be the maximal set of single-peaked preferences. By Weymark (2011), a min–max rule is strategy-proof on \(\bar{\mathcal {S}}_N\). Since \(\mathcal {S}_{i} \subseteq \bar{\mathcal {S}}_{i}\) for all \(i \in N\), a min–max rule must be strategy-proof on \(\mathcal {S}_N\). This completes the proof of the if part.
(Only-if part) Let \(\mathcal {S}_{i}\) be a top-connected set of single-peaked preferences for all \(i \in N\) and let \(f: \mathcal {S}_{N} \rightarrow X\) be a unanimous and strategy-proof SCF. We show that f is a min–max rule. First, we establish a few properties of f in the following sequence of lemmas.
By Corollary 1 and Theorem 3, f must satisfy Pareto property and tops-onlyness. Also, by Theorem 1, f is group strategy-proof. Our next lemma shows that f is uncompromising.
Lemma 3
The SCF f is uncompromising.
Proof
Let \(P_{N} \in \mathcal {S}_{N}\), \(i \in N\), and \(P'_{i} \in \mathcal {S}_{i}\) be such that \(r_{1}(P_{i})< f(P_{N})\) and \(r_{1}(P'_{i}) \le f(P_{N})\). It is sufficient to show \(f(P'_{i},P_{N {\setminus } i}) = f(P_{N})\). Suppose \(r_{1}(P_{i}) = x\), \(f(P_{N}) = y\), and \(f(P'_{i},P_{N {\setminus } i}) = y'\).
By strategy-proofness, we must have \(y' < x\). This is because, if \(y' \in [x,y)\), then agent i manipulates at \(P_{N}\) via \(P'_{i}\). On the other hand, if \(y' > y\), then by means of the fact that \(r_{1}(P'_{i}) \le y\), agent i manipulates at \((P'_{i},P_{N {\setminus } i})\) via \(P_{i}\).
Because \(y'<x\), we assume without loss of generality that \(r_1(P'_{i}) = y'\) and \(\min (\tau (P'_{i},P_{N {\setminus } i} ) ) = y'\).Footnote 13 Assume for contradiction that \(y \ne y'\).
Let \(T = \{j \in N \mid r_1(P_{j}) < x\}\). For \(j \in T\), let \(P'_{j} \in \mathcal {S}_{j}\) be such that \(r_1(P'_{j})=x\).
Claim 1. \(f(P_N)=y\) implies \(f(P'_{T},P_{N {\setminus } T})= y.\)
If T is empty, then there is nothing to show. Suppose T is non-empty. By Pareto property, \(f(P'_{T},P_{N {\setminus } T}) \ge x\). If \(f(P'_{T},P_{N {\setminus } T}) \in [x,y)\), then the agents in T manipulate f at \(P_N\) via \((P'_{T},P_{N {\setminus } T})\). On the other hand, if \(f(P'_{T},P_{N {\setminus } T}) > y\), then the agents in T manipulate f at \((P'_{T},P_{N {\setminus } T})\) via \(P_N\). This completes the proof of Claim 1.
Let \(T' = T \cup i\). For all \(j \in T'\), let \(\tilde{P}_{j} \in \mathcal {S}_j\) be such that \(r_{1}(\tilde{P}_{j}) = x\).
Claim 2. \(f(P_i', P_{N {\setminus } i})=y'\) implies \(f(\tilde{P}_{T'},P_{N {\setminus } T'}) = x\).
Let \(T''\) be the set of agents whose top-ranked alternative is \(y'\) at the profile \((P'_{i},P_{N {\setminus } i})\). More formally, \(T'' =i \cup \{j \in N \mid r_{1}(P_{j}) = y'\}\). Consider the profile \(\bar{P}_{N} \in \mathcal {S}_{N}\) such that \(r_{1}(\bar{P}_{j}) = y'+1\) for all \(j \in T''\) and \(\bar{P}_{j}=P_{j}\) for all other agents. As \(\mathcal {S}_N\) is top-connected, we can assume \(r_{2}(\bar{P}_{j}) = y'\) for all \( j \in T''\). However, since \(f(P_i', P_{N {\setminus } i})=y'\), by group strategy-proofness, \(f(\bar{P}_{N})\in \{y',y'+1\}\) as otherwise agents in \(T''\) manipulate f at \(\bar{P}_N\) via \((P_i',P_{N {\setminus } i})\). Since \(\min (\tau (\bar{P}_{N}))=y'+1\), by Pareto property,
Using similar logic, we can construct a profile \(\hat{P}_{N} \in \mathcal {S}_{N}\) where \(r_{1}(\hat{P}_{j}) = y'+2\) and \(r_{2}(\hat{P}_{j}) = y'+1\) for all agents j with \(r_{1}(\bar{P}_{j}) = y'+1\) and \(\hat{P}_{j}=\bar{P}_{j}\) for all other agents, and conclude that
Continuing in this manner, we move all the agents j in \(T'\) to a preference \(\tilde{P}_{j} \in \mathcal {S}_{j}\) with \(r_{1}(\tilde{P}_{j}) = x\) and \(r_2(\tilde{P}_j) = x-1\) while keeping the preferences of all other agents unchanged and conclude that
This and tops-onlyness completes the proof of Claim 2.
Now, we complete the proof of the lemma. Consider the profiles \((P'_{T},P_{N {\setminus } T})\) and \((\tilde{P}_{T'},P_{N {\setminus } T'})\). Note that for an agent j, if \(r_{1}(P_{j}) > x\), then her preference is the same in both the profiles \((P'_{T},P_{N {\setminus } T})\) and \((\tilde{P}_{T'},P_{N {\setminus } T'})\). Moreover, for an agent j, if \(r_{1}(P_{j}) \le x\), then her top-ranked alternative is x in both the profiles. Therefore, the top-alternatives of each agent in these two profiles are the same. However, since \(f(P'_{T},P_{N {\setminus } T}) \ne f(\tilde{P}_{T'},P_{N {\setminus } T'})\), Claim 1 and 2 contradict tops-onlyness of f. This completes the proof of the lemma. \(\square \)
The following lemma establishes that f is a min–max rule.
Lemma 4
The SCF f is a min–max rule.
Proof
For all \(S \subseteq N\), let \((P^{a}_{S},P^{b}_{N {\setminus } S}) \in \mathcal {S}_{N}\) be such that \(r_{1}(P^{a}_{i}) = a\) for all \(i \in S\) and \(r_{1}(P^{b}_{i}) = b\) for all \(i \in N {\setminus } S\). Define \(\beta _{S} = f(P^{a}_{S},P^{b}_{N {\setminus } S})\) for all \(S \subseteq N\). Clearly, \(\beta _{S} \in X\) for all \(S \subseteq N\). By unanimity, \(\beta _{\varnothing } = b\) and \(\beta _{N} = a\). Also, by strategy-proofness, \(\beta _{S} \le \beta _{T}\) for all \(T \subseteq S\).
Take \(P_{N} \in \mathcal {S}_{N}\). We show \(f(P_{N}) = \min \nolimits _{S \subseteq N}\{\max \nolimits _{i \in S}\{r_{1}(P_{i}),\beta _{S}\}\}\). Suppose \(S_{1} = \{i \in N \mid r_{1}(P_{i}) < f(P_{N})\}\), \(S_{2} = \{i \in N \mid f(P_{N}) < r_{1}(P_{i})\}\), and \(S_{3} = \{i \in N \mid r_{1}(P_{i}) = f(P_{N})\}\). By strategy-proofness and uncompromisingness, \(\beta _{S_{1} \cup S_{3}} \le f(P_{N}) \le \beta _{S_{1}}\). Consider the expression \(\min \nolimits _{S \subseteq N}\{\max \nolimits _{i \in S} \{r_{1}(P_{i}), \beta _{S}\}\}\). Take \(S \subseteq S_{1}\). Then, by Condition (iii) in Definition 9, \(\beta _{S_{1}} \le \beta _{S}\). Since \(r_{1}(P_{i}) < f(P_{N})\) for all \(i \in S\) and \(f(P_{N}) \le \beta _{S_{1}} \le \beta _{S}\), we have \(\max \nolimits _{i \in S} \{r_{1}(P_{i}), \beta _{S}\} = \beta _{S}\). Clearly, for all \(S \subseteq N\) such that \(S \cap S_{2} \ne \varnothing \), we have \(\max \nolimits _{i \in S} \{r_{1}(P_{i}), \beta _{S}\} > f(P_{N})\). Consider \(S \subseteq N\) such that \(S \cap S_{2} = \varnothing \) and \(S \cap S_{3} \ne \varnothing \). Then, \(S \subseteq S_{1} \cup S_{3}\), and hence \(\beta _{S_{1} \cup S_{3}} \le \beta _{S}\). Therefore, \(\max \nolimits _{i \in S} \{r_{1}(P_{i}), \beta _{S}\} = \max \{f(P_{N}), \beta _{S}\} \ge \max \{f(P_{N}), \beta _{S_{1} \cup S_{3}}\}\). Since \(\beta _{S_{1} \cup S_{3}} \le f(P_{N})\), we have \(\max \{f(P_{N}), \beta _{S_{1} \cup S_{3}}\} = f(P_{N})\). Combining all these, we have \(\min \nolimits \nolimits _{S \subseteq N}\{\max \nolimits _{i \in S} \{r_{1}(P_{i}), \beta _{S}\}\} = \min \{f(P_{N}),\beta _{S_{1}}\}\). Because \(f(P_{N}) \le \beta _{S_{1}}\), we have \(\min \{f(P_{N}),\beta _{S_{1}}\} = f(P_{N})\). This completes the proof of the lemma. \(\square \)
The proof of the only-if part of Theorem 4 follows from Lemmas 3 and 4. \(\square \)
1.4 Proof of Theorem 5
Proof
The proof of the if part follows from Theorem 4. We proceed to prove the only-if part. Let \(\mathcal {D}_{N}\) be a min–max domain. We show that \(\mathcal {D}_{i}\) is top-connected single-peaked for all \(i \in N\). We show this in two steps: in Step 1 we show that \(\mathcal {D}_i\) is single-peaked for all \(i \in N\), and in Step 2, we show that \(\mathcal {D}_i\) is top-connected for all \(i\in N\).
Step 1. Suppose that \(\mathcal {D}_{i}\) is not single-peaked for some \(i \in N\). Then, there is \(Q \in \mathcal {D}_{i}\) and \(x,y \in X\) such that \(x< y < r_{1}(Q)\) and xQy. Consider the min–max rule \(f^{\beta }\) with respect to \((\beta _{S})_{S \subseteq N}\) such that \(\beta _{S} = x\) for all \(\emptyset \subsetneq S \subsetneq N\). Take \(P_{N} \in {\mathcal {D}}_N\) such that \(P_{i} = Q\) and \(r_{1}(P_{j}) = y\) for all \(j \in N {\setminus } i\). By the definition of \(f^{\beta }\), \(f^{\beta }(P_{N}) = y\). Now, take \(P'_{i} \in \mathcal {D}_{i}\) with \(r_{1}(P'_{i}) = x\). Again, by the definition of \(f^{\beta }\), \(f^{\beta }(P'_{i},P_{N {\setminus } i}) = x\). This means agent i manipulates at \(P_{N}\) via \(P'_{i}\), which is a contradiction to the assumption that \(\mathcal {D}_{N}\) is a min–max domain. This completes Step 1.
Step 2. In this step, we show that \(\mathcal {D}_{i}\) satisfies top-connectedness for all \(i \in N\). Assume for contradiction that \(\mathcal {D}_{i}\) is not top-connected for some \(i \in N\). By definition, \(\mathcal {D}_{i}\) is regular. Since \(\mathcal {D}_{i}\) is single-peaked, for all \(P \in \mathcal {D}_{i}\), \(r_{1}(P)=a ~(\text{ or } b)\) implies \(r_{2}(P)=a+1 ~ (\text{ or } b-1)\). Again, because \(\mathcal {D}_{i}\) is single-peaked, for all \(P \in \mathcal {D}_{i}\) and all \(x \in X {\setminus } \{a,b\}\), \(r_{1}(P)=x\) implies \(r_{2}(P) \in \{x-1,x+1\}\). Since \(\mathcal {D}_{i}\) violates top-connectedness, assume without loss of generality that there exists \(x \in X {\setminus } \{a,b\}\) such that for all \(P \in \mathcal {D}_{i}\), \(r_{1}(P) = x\) implies \(r_{2}(P) = x-1\). Consider the following SCF:Footnote 14
It is left to the reader to verify that f is unanimous and strategy-proof. We show that f is not uncompromising, which in turn means that f is not a min–max rule. Let \(P_{N} \in \mathcal {D}_{N}\) be such that \(r_{1}(P_{i}) = x\) and \(r_{1}(P_{j}) = x-1\) for some \(j \ne i\), and let \(P'_{i} \in \mathcal {D}_{i}\) be such that \(r_{1}(P'_{i}) = x+1\). Then, by the definition of f, \(f(P_{N}) = x-1\) and \(f(P'_{i},P_{N {\setminus } i}) = x+1\). Therefore, because \(f(P_N)=x-1\) and \(x-1 \le r_1(P_i) \le r_1(P_i')\), the fact that \(f(P'_{i},P_{N {\setminus } i}) = x+1\) is a violation of uncompromisingness. This completes Step 2 and the proof of the only-if part. \(\square \)
Rights and permissions
About this article
Cite this article
Achuthankutty, G., Roy, S. On single-peaked domains and min–max rules. Soc Choice Welf 51, 753–772 (2018). https://doi.org/10.1007/s00355-018-1137-1
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00355-018-1137-1