Abstract
In 1901, Henri Poincaré discovered a new set of equations for mechanics. These equations are a generalization of Lagrange’s equations for a system whose configuration space is a Lie group which is not necessarily commutative. Since then, this result has been extensively refined through the Lagrangian reduction theory. In the present contribution, we apply an extended version of these equations to continuous Cosserat media, i.e. media in which the usual point particles are replaced by small rigid bodies, called microstructures. In particular, we will see how the shell balance equations used in nonlinear structural dynamics can be easily deduced from this extension of the Poincaré’s result. In future, these results will be used as foundations for the study of squid locomotion, which is an emerging topic relevant to soft robotics.






Similar content being viewed by others
Notes
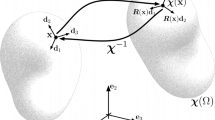
Here note that \(\varPhi \) is not a mechanical transformation of the body in the ambient space but rather a geometric transformation or more exactly a parameterization of \({\mathcal {B}}\). The mechanical transformation between the reference and the deformed configurations is in fact defined as \(\varPhi _{t}\circ \varPhi _{o}^{-1}\).
Abbreviations
- t :
-
Time
- \({\mathcal {E}}\) :
-
Three-dimensional geometric space of classical mechanics
- \({\mathcal {B}}\) :
-
Three-dimensional material space of a classical continuous medium
- \({\mathcal {D}}\) :
-
Material (p-dimensional, \(p<3\)) reference subspace
- \({\mathcal {M}}\) :
-
Rigid microstructure
- \({\mathcal {B}}={\mathcal {D}}\times {\mathcal {M}}\) :
-
Material space of a Cosserat medium
- \((O,E_{1},E_{2},E_{3})\) :
-
Material frame attached to \({\mathcal {B}}\)
- \((o,e_{1},e_{2},e_{3})\) :
-
Spatial frame attached to \({\mathcal {E}}\)
- \(x=x^{i}e_{i}\) :
-
Points of geometric space
- \(X=X^{i}E_{i}\) :
-
Material points of \({\mathcal {B}}\)
- \(\overline{X}=X^{\alpha }E_{\alpha }\) :
-
Material points of \({\mathcal {D}}\)
- \(\varPhi _{t}\) :
-
Transformation at time t from material to geometric space
- \(\varPhi _{t}({\mathcal {B}})\) :
-
Deformed configuration of \({\mathcal {B}}\)
- \(\varPhi _{o}({\mathcal {B}})\) :
-
Reference configuration of \({\mathcal {B}}\)
- \((\varPhi _{t}\circ e)({\mathcal {D}})\) :
-
Deformed configuration of \({\mathcal {D}}\)
- \((\varPhi _{o}\circ e)({\mathcal {D}})\) :
-
Reference configuration of \({\mathcal {D}}\)
- \(r(\overline{X})\) :
-
Position of \((\varPhi _{t}\circ e)(\overline{X})\)
- \(R(\overline{X})\in SO(3)\) :
-
Rotation tensor mapping \((E_{1},E_{2},E_{3})\) onto \((t_{1},t_{2},t_{3})(\overline{X})\)
- \((g_{1},g_{2},g_{3})(X)\) :
-
Convected basis on \(\varPhi _{t}({\mathcal {B}})\) at \(\varPhi _{t}(X)\)
- \((h_{1}, \ldots h_{p})(\overline{X})\) :
-
Convected basis on \((\varPhi _{t}\circ e)({\mathcal {D}})\) at \(r(\overline{X})\)
- \((t_{1},t_{2},t_{3})(\overline{X})\) :
-
Orthonormal spatial basis attached to the X-microstructure
- \((g_{ij}g^{i}\otimes g^{j})(X)\) :
-
Euclidean metric tensor in the convected basis of \(\varPhi _{t}({\mathcal {B}})\)
- \((h_{\alpha \beta }h^{\alpha }\otimes h^{\beta })(\overline{X})\) :
-
Euclidean metric induced on \((\varPhi _{t}\circ e)({\mathcal {D}})\) in its convected basis
- \(\nu \), \(\nu _{o}\), \(\nu _{t}\) :
-
Oriented unit normal vector to the material, reference and deformed surface element of \({\mathcal {D}}\)
- \(\textit{dS}\), \(\textit{dS}_{o}\), \(\textit{dS}_{t}\) :
-
Area of the material, reference and deformed surface element of \({\mathcal {D}}\)
- \({\mathcal {C}}\) :
-
Configuration space of a Cosserat medium \({\mathcal {D}}\times {\mathcal {M}}\)
- G and \(\texttt {g}\) :
-
Group of transformation and transformation of microstructure
- \(\mathfrak {g}\), \(\mathfrak {g}^{*}\) :
-
Lie algebra of G and its dual
- Ad and \(Ad^{*}\) :
-
Adjoint and co-adjoint action map of G on \(\mathfrak {g}\) and \(\mathfrak {g}^{*}\)
- ad and \(ad^{*}\) :
-
Adjoint and co-adjoint action map of \(\mathfrak {g}\) on \(\mathfrak {g}\) and \(\mathfrak {g}^{*}\)
- \(\eta \) and \(\xi _{\alpha }\) :
-
Left-invariant fields along time and space variables
- \(\mathfrak {L}\), \(\mathfrak {L}_{o}\) and \(\mathfrak {L}_{t}\) :
-
Density of left-reduced Lagrangian of a Cosserat medium per unit of its material, reference and deformed volume
- \(\frac{\partial \mathfrak {L}}{\partial \eta }\),\(\frac{\partial \mathfrak {L}_{o}}{\partial \eta }\) and \(\frac{\partial \mathfrak {L}_{t}}{\partial \eta }\) :
-
Densities of material t-conjugate (kinetic) momentum, per unit of material, reference and deformed volume
- \(\frac{\partial \mathfrak {L}}{\partial \xi _{\alpha }}\),\(\frac{\partial \mathfrak {L}_{o}}{\partial \xi _{\alpha }}\) and \(\left[ \frac{\partial \mathfrak {L}}{\partial \xi _{\alpha }}\right] _{t}\) :
-
Densities of material \(X^{\alpha }\)-conjugate (stress) momentum, per unit of material, reference and deformed volume
- \(F_\mathrm{ext}\), \(F_{\mathrm{ext},o}\) and \(F_{\mathrm{ext},t}\) :
-
Densities of material external forces per unit of material, reference and deformed volume
- \(\overline{F}_\mathrm{ext}\), \(\overline{F}_{\mathrm{ext},o}\) and \(\overline{F}_{\mathrm{ext},t}\) :
-
Densities of external forces per unit of material, reference and deformed boundary volume
- \({\mathcal {D}}\times {\mathbb {R}}^{+}\) :
-
Space–time of a p-dimensional Cosserat medium
- \(X^{0}\frac{\partial }{\partial t}+X^{\alpha }\frac{\partial }{\partial X^{\alpha }}\) :
-
Point in space–time with \(t=X^{0}\)
- \(\varUpsilon \) :
-
Space–time 1-form field with value in \(\mathfrak {g}\)
- \(\varLambda \), \(\varLambda _{o}\) and \(\varLambda _{t}\) :
-
Density of a space–time vector field with value in \(\mathfrak {g}^{*}\), per unit of material, reference and deformed volume
- \(\langle .,. \rangle \) and (., .):
-
Duality product in \(\mathfrak {g}\) and space–time
- \(Ad^{*}_{\texttt {g}^{-1}}\left( \sqrt{|h|}\left( \frac{\partial \mathfrak {L}_{t}}{\partial \eta }\right) \right) \) :
-
Densities of spatial (in the fixed frame) kinetic wrench, per unit of deformed volume
- \(Ad^{*}_{\texttt {g}^{-1}}\left( \sqrt{|h|}\left( \frac{\partial \mathfrak {L}_{t}}{\partial \xi _{\alpha }}\right) \right) \) :
-
Densities of spatial (in the fixed frame) stress wrench, per unit of deformed volume
- \(\textit{SE}(3)\) :
-
Special Euclidean group in \({\mathbb {R}}^{3}\) with Lie algebra \(\textit{se}(3)\)
- (R, r):
-
Transformation of \(\textit{SE}(3)\)
- \((\varOmega ^{T},V^{T})^{T}\in se(3)\) :
-
Material time rate of transformation (velocity) of the microstructure frames
- \((\omega ^{T},v^{T})^{T}\in se(3)\) :
-
Spatial time rate of transformation (velocity) of the microstructure frames
- \((\varSigma _{t}^{T},P_{t}^{T})^{T} \in se(3)^{*}\) :
-
Density of material kinetic wrench per unit of deformed volume
- \((\sigma _{t}^{T},p_{t}^{T})^{T}\in se(3)^{*}\) :
-
Density of spatial (in the microstructure frame) kinetic wrench per unit of deformed volume
- \((K_{\alpha }^{T},\varGamma _{\alpha }^{T})^{T}\in \textit{se}(3)\) :
-
Material \(X^{\alpha }\)-rate of transformation of the microstructure frames
- \((k_{\alpha }^{T},\gamma _{\alpha }^{T})^{T}\in \textit{se}(3)\) :
-
Spatial \(X^{\alpha }\)-rate of transformation of the microstructure frames
- \((M_{\alpha ,t}^{T},N_{\alpha ,t}^{T})^{T} \in \textit{se}(3)^{*}\) :
-
Density of material stress wrench per unit of deformed volume
- \((m_{\alpha ,t}^{T},n_{\alpha ,t}^{T})^{T}\in \textit{se}(3)^{*}\) :
-
Density of spatial stress wrench per unit of deformed volume
- \((\overline{\rho },\overline{\rho }_{o},\overline{\rho }_{t})\) and \((\overline{J},\overline{J}_{o},\overline{J}_{t})\) :
-
Densities of mass and of material angular inertia tensor per unit of material, reference and deformed volume
- \((\overline{I},\overline{I}_{o},\overline{I}_{t})\) :
-
Densities of spatial inertia tensor per unit of material, reference and deformed volume
- \(\epsilon _{\alpha \beta },\rho _{\alpha \beta },\tau _{\alpha }\) :
-
Effective strain measures (stretching, bending, transverse shearing) of a classical shell
- \({\mathcal {N}}_{t}^{\alpha \beta },{\mathcal {M}}_{t}^{\alpha \beta },{\mathcal {Q}}_{t}^{\alpha }\) :
-
Densities of effective stress of a classical shell per unit of deformed volume
References
Antman, S.S.: Nonlinear problems of elasticity. In: Mathematical Sciences, vol. 107. Springer, New York (2005)
Arnold, V.I.: Sur la geometrie differentielle des groupes de Lie de dimension infinie et ses applications a l’hydrodynamique des fluides parfaits. Ann. Inst. J. Fourier 16(1), 319–361 (1966)
Arnold, V.I.: Mathematical Methods in Classical Mechanics, 2nd edn. Springer, New-York (1988)
Boyer, F., Primault, D.: The Poincaré–Chetayev equations and flexible multibody systems. J. Appl. Math. Mech. 69(6), 925–942 (2005). http://hal.archives-ouvertes.fr/hal--00672477
Boyer, F., Porez, M., Khalil, W.: Macro-continuous computed torque algorithm for a three-dimensional eel-like robot. IEEE Trans. Robot. 22(4), 763–775 (2006)
Boyer, F., Porez, M., Leroyer, A., Visonneau, M.: Fast dynamics of an eel-like robot-comparisons with Navier–Stokes simulations. IEEE Trans. Robot. 24(6), 1274–1288 (2008)
Boyer, F., Porez, M., Leroyer, A.: Poincaré–Cosserat equations for the Lighthill three-dimensional large amplitude elongated body theory: Application to robotics. J. Nonlinear Sci. 20, 47–79 (2010)
Boyer, F., Ali, S., Porez, M.: Macro-continuous dynamics for hyper-redundant robots: application to kinematic locomotion bio-inspired by elongated body animals. IEEE Trans. Robot. 28(2), 303–317 (2012)
Castrillón López, M., Ratiu, T.S., Shkoller, S.: Reduction in principal fiber bundles: covariant Euler–Poincaré equations. Proc. Am. Math. Soc. 128(7), 2155–2164 (2000)
Cosserat, E., Cosserat, F.: Théorie des corps déformables. Hermann, Paris (1909)
Demoures, F., Gay-Balmaz, F., Kobilarov, M., Ratiu, T.S.: Multisymplectic lie group variational integrator for a geometrically exact beam in r3. Commun. Nonlinear Sci. Numer. Simul. 19(10), 3492–3512 (2014)
Ebin, D.G., Marsden, J.E.: Groups of diffeomorphism and the motion of an incompressible fluid. Ann. Math 92, 102–163 (1970)
Ellis, D.C.P., Gay-Balmaz, F., Holm, D.D., Putkaradze, V., Ratiu, T.S.: Symmetry reduced dynamics of charged molecular strands. Arch. Ration. Mech. Anal. 197(3), 811–902 (2010)
Eringen, A.C.: Microcontinuum Field Theories I: Foundations and Solids. Springer, New York (1998)
Fox, D.D., Simo, J.C.: A drill rotation formulation for geometrically exact shells. Comput. Methods Appl. Mech. Eng. 98, 329–343 (1992)
Gay-Balmaz, F., Holm, D.D., Ratiu, T.S.: Variational principles for spin systems and the Kirchhoff rod. J. Geom. Mech. 1(4), 417–444 (2009)
Green, A.E., Naghdi, P.M.: Non-isothermal theory of rods, plates and shells. Int. J. Solids Struct. 6, 209–244 (1970)
Green, A.E., Naghdi, P.M.: On the derivation of shell theories by direct approach. J. Appl. Mech. 41(1), 173–176 (1974)
Green, A.E., Zerna, W.: Theoretical Elasticity. Clarendon Press, Oxford (1960). end ed. edition
Holm, D.D., Marsden, J.E., Ratiu, T.S.: The Euler–Poincaré equations and semidirect products with applications to continuum theories. Adv. Math. 137, 1–81 (1998)
Holm, D.D., Putkaradze, V.: Nonlocal orientation-dependent dynamics of charged strands and ribbons. C. R. Acad. Sci. Paris Ser. I 347, 1093–1098 (2009)
Libai, A., Simmonds, J.G.: The Nonlinear Theory of Elastic Shells, 2nd edn. Cambridge University Press, Cambridge (1998)
Lichnerowicz, A.: Elements de Calcul Tensoriel. Jacques Gabay, Paris (1987)
Malvern, L.E.: Introduction to the Mechanics of a Continuous Medium. Prentice-Hall, New-Jersey (1969)
Marle, C.-M.: On Henri Poincaré’s note: “sur une forme nouvelle des equations de la mécanique”. J. Geom. Symmetry Phys. 29, 1–38 (2013)
Marsden, J.E., Montgomery, R., Ratiu, T.S.: Reduction, symmetry, and phases in mechanics. In: Memoirs of the American Mathematical Society, vol. 88 (436). American Mathematical Society (1990)
Marsden, J.E., Hughes, T.J.R.: Mathematical Foundations of Elasticity, 1st edn. Dover, Mineola (1994)
Marsden, J.E., Ratiu, T.S.: Introduction to Mechanics and Symmetry, 2nd edn. Springer, New York (1999)
Milne-Thomson, L.M.: Theoretical Hydrodynamics. Macmillan, London (1938)
Poincaré, H.: Sur une forme nouvelle des équations de la mécanique. Compte Rendu de l’Académie des Sciences de Paris 132, 369–371 (1901)
Pommaret, J.F.: Partial Differential Equations and Group Theory, 1st edn. Springer, Netherlands (1994)
Reissner, E.: The effect of transverse shear deformation on the bending of elastic plates. J. Appl. Mech. 12, 69–76 (1945)
Renda, F., Giorelli, M., Calisti, M., Cianchetti, M., Laschi, C.: Dynamic model of a multibending soft robot arm driven by cables. IEEE Trans. Robot. 30(5), 1109–1122 (2014)
Simmonds, J.G., Danielson, D.A.: Nonlinear shell theory with finite rotation and stress-function vectors. J. Appl. Mech. 39, 1085–1090 (1972)
Simo, J.C., Fox, D.D.: On a stress resultant geometrically exact shell model. Part I: formulation and optimal parametrization. Comput. Methods Appl. Mech. Eng. 72(3), 267–304 (1989)
Simo, J.C., Marsden, J.E., Krishnaprasad, P.S.: The hamiltonian structure of nonlinear elasticity: the material and convective representations of solids, rods, and plates. Arch. Ration. Mech. Anal. 104, 125–183 (1988)
Simo, J.C., Rifai, M.S., Fox, D.D.: On a stress resultant geometrically exact shell model. part vi: conserving algorithms for non-linear dynamics. Int. J. Numer. Methods Eng. 34, 117–164 (1992)
Simo, J.C., Vu-Quoc, L.: On the dynamics in space of rods undergoing large motions—a geometrically exact approach. Comput. Methods Appl. Mech. Eng. 66(2), 125–161 (1988)
Spencer, D.C.: Overdetermined systems of partial differential equations. Bull. Am. Math. Soc. 75, 1–114 (1965)
Thomas, J.R., Hughes, Brezzi, F.: On drilling degrees of freedom. Comput. Methods Appl. Mech. Eng. 72, 105–121 (1989)
Toupin, R.A.: Theories of elasticity with couple-stress. Arch. Rational Mech. Anal. 17, 85–112 (1964)
Verl, A., Albu-Schaeffer, A., Brock, O. (eds). Soft Robotics: Transferring Theory to Application. Springer, New York (2015)
Vu-Quoc, L.: On the algebra of two point tensors and their applications. Z. Angew. Math. Mech.: ZAMM 76(9), 540–541 (1996)
Weymouth, G.D., Triantafyllou, M.S.: Ultra-fast escape of a deformable jet-propelled body. J. Fluid Mech. 721, 367–385 (2013)
Author information
Authors and Affiliations
Corresponding author
Additional information
Communicated by Paul Newton.
Appendices
Appendix 1: Proof of (44) Through Direct Application of the Hamilton Principle
As evoked in Remark 5.2, Eqs. (43) and (44) can be derived directly by applying the Hamilton principle to an action defined in terms of the different definitions of the reduced Lagrangian of Sect. 2.6. In this “Appendix”, we apply this calculus to the deformed configuration. This can be achieved using Stokes theorem applied to differential forms or alternatively using the divergence theorem and vector analysis. We will follow the latter approach and will denote, according to the context of Sect. 2.4, \(\textit{dX}^{1}{} \textit{dX}^{2}\ldots \textit{dX}^{p}=d{\mathcal {D}}\) and \(|\overline{H}|^{1/2}dY^{1}dY^{2}\) \(\ldots dY^{p-1}=d\partial {\mathcal {D}}\) the material volumes of \({\mathcal {D}}\) and \(\partial {\mathcal {D}}\), which are assumed to be two manifolds consistently oriented according to the outward unit normal convention. We start from (19) and (20) in which we replace \(\mathfrak {L}\) by \(\sqrt{|h|}\mathfrak {L}_{t}\) and \((F_\mathrm{ext},\overline{F}_\mathrm{ext})\) by \((F_\mathrm{ext}|h|^{1/2},\overline{F}_\mathrm{ext}|\overline{h}|^{1/2})\). In this new formulation of Hamilton principle, \(\delta \) being achieved at fixed time and material parameters according to Sect. 2.6, this enables us to shift it under the integral. Then, since \(\delta (\mathfrak {L}_{t}\sqrt{|h|})=\delta \mathfrak {L}_{t}\sqrt{|h|}+\mathfrak {L}_{t}\delta \sqrt{|h|}\), where \(\sqrt{|h|}\) is configuration dependent through the invariant fields \(\xi _{\alpha }\) (see Sect. 2.6), we have:
where we used the notation \([\partial \mathfrak {L}/\partial \xi _{\alpha }]_{t}=\partial \mathfrak {L}_{t}/\partial \xi _{\alpha }\) \(+|h|^{-\frac{1}{2}}(\partial |h|^{\frac{1}{2}}/\partial \xi _{\alpha })\) \(\mathfrak {L}_{t}\), as it is introduced by (42). Then invoking (23) and applying a by-part time integration with fixed extreme times condition allows the left-hand side of (102) to be rewritten as:
Now let us remark that:
whose first right-hand side term is merely the divergence of a vector field of contravariant components \(v^{\alpha }=<[\partial \mathfrak {L}/\partial \xi _{\alpha }]_{t},\delta \zeta>\) in the convected basis \(\{h_{\alpha }\}_{\alpha =1,\ldots p}\). Applying the divergence theorem to this term gives:
where we introduce the metric volume element \(|\overline{h}|^{1/2}d\partial {\mathcal {D}}\) on \((\varPhi _{t}\circ e)(\partial {\mathcal {D}})\), and \(\nu _{t,\alpha } h^{\alpha }\) is the unit outward normal to the tangent planes of \((\varPhi _{t}\circ e)(\partial {\mathcal {D}})\) which, from (12), is related to the outward unit normal \(\nu _{\alpha }E^{\alpha }\) by \(\nu _{t,\alpha } |\overline{h}|^{1/2}d\partial {\mathcal {D}}=\nu _{\alpha } \sqrt{|h|}d\partial {\mathcal {D}}\). Then, inserting (105) into (104) and the result into (103) whose the last term is dualized, gives, with (20), a balance of two integral components, one over \({\mathcal {D}}\) with metric volume \(\sqrt{|h}|d{\mathcal {D}}\) and the second over \(\partial {\mathcal {D}}\), whose metric volume is \(|\overline{h}|^{1/2}d\partial {\mathcal {D}}\). This balance being satisfied for any variation \(\delta \zeta \in \mathfrak {g}\), it gives the set of Eq. (44), where due to (21), \(|\overline{h}|^{1/2}=1\) in the case of beams.
Appendix 2: Proof of (62)
The general expression (106) is stated in Simo et al. (1988) in the case of geometrically exact beams and plates, in this “Appendix” we prove it for shells. Since the medium \({\mathcal {B}}\) is classical (not micropolar), we have:
where \(g_{ij}(g^{i}\otimes g^{j})\) is the fundamental metric tensor in the convected basis of \(\varPhi _{t}({\mathcal {B}})\), with determinant |g|, and \(\sigma ^{ij}(g_{i}\otimes g_{j})\) is the Cauchy stress tensor in the same basis. Then introducing the Cosserat shell kinematics (64) into \(g_{ij}=g_{i}.g_{j}\) with \(g_{i}=\partial \varPhi _{t}/\partial X^{i}\) gives:
where, consistently with the first-order Cosserat kinematics (8), the \(X^{3}\)-quadratic terms are neglected in the expression of \(g_{\alpha \beta }\). In these conditions, we recognize in (107) the time-dependent components of the strain measures (86–88). Now, since expressions (107) depend on the medium configuration through the left-invariant fields \(\varGamma _{\alpha }\) and \(K_{\alpha }\) of (65) only, one can state:
in which the partial derivatives depend on the mid-surface coordinates through the left-invariant fields, and on \(X^{3}\), in a linear way. Then, introducing (108) into (106), and integrating the result along the \(X^{3}\)-thickness dimension, gives a general expression of the virtual work of internal forces similar to (62), along with the explicit expressions of the resultant of stress and couple stress \(N^{\alpha }_{t}\) and \(M^{\alpha }_{t}\) in terms of the three-dimensional Cauchy stress tensor:
where \(i<j\). \(\square \)
Rights and permissions
About this article
Cite this article
Boyer, F., Renda, F. Poincaré’s Equations for Cosserat Media: Application to Shells. J Nonlinear Sci 27, 1–44 (2017). https://doi.org/10.1007/s00332-016-9324-7
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00332-016-9324-7