Analytical detection of stationary and dynamic patterns in a prey–predator model with reproductive Allee effect in prey growth

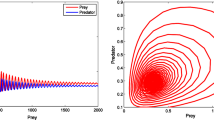
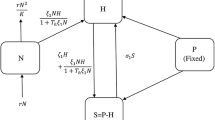
Abstract

Allee effect in population dynamics has a major impact in suppressing the paradox of enrichment through global bifurcation, and it can generate highly complex dynamics. The influence of the reproductive Allee effect, incorporated in the prey’s growth rate of a prey–predator model with Beddington–DeAngelis functional response, is investigated here. Preliminary local and global bifurcations are identified of the temporal model. Existence and non-existence of heterogeneous steady-state solutions of the spatio-temporal system are established for suitable ranges of parameter values. The spatio-temporal model satisfies Turing instability conditions, but numerical investigation reveals that the heterogeneous patterns corresponding to unstable Turing eigenmodes act as a transitory pattern. Inclusion of the reproductive Allee effect in the prey population has a destabilising effect on the coexistence equilibrium. For a range of parameter values, various branches of stationary solutions including mode-dependent Turing solutions and localized pattern solutions are identified using numerical bifurcation technique. The model is also capable to produce some complex dynamic patterns such as travelling wave, moving pulse solution, and spatio-temporal chaos for certain range of parameters and diffusivity along with appropriate choice of initial conditions. Judicious choices of parametrization for the Beddington–DeAngelis functional response help us to infer about the resulting patterns for similar prey–predator models with Holling type-II functional response and ratio-dependent functional response.

Appendices

Appendix A

Here we derive upper bounds for $I_1$, $I_2$ and $I_3$ used in Theorem 5. Using proposition 1, we find

$$\begin{aligned} I_1&= \int _\Omega (u-{\bar{u}})^2 \big (-(u^2+u {\bar{u}}+\bar{u}^2)+(u+{\bar{u}})(u_1+u_2)-u_1u_2 \big ) dx\\&\le \int _\Omega (u-{\bar{u}})^2 \left[ (u+{\bar{u}})(u_1+u_2)-u_1u_2 \right] dx\\&\le u_1(2-u_2) \int _\Omega (u-{\bar{u}})^2 dx,\\ I_2&= \frac{1}{\alpha +{\bar{u}}+\beta {\bar{v}}}\int _\Omega (u-\bar{u})\left( \frac{\alpha ({\bar{u}} {\bar{v}}-uv)+\beta v {\bar{v}}(\bar{u}-u)+u{\bar{u}} ({\bar{v}}-v)}{\alpha +u+\beta v}\right) dx\\&=\frac{1}{\alpha +{\bar{u}}+\beta {\bar{v}}}\int _\Omega (u-\bar{u})\left( \frac{ {\bar{v}}({\bar{u}}-u)(\alpha +\beta v)+u ({\bar{v}}-v) (\alpha + {\bar{u}})}{\alpha +u+\beta v}\right) dx \\&\le \frac{(\alpha + {\bar{u}})}{\alpha +{\bar{u}}+\beta {\bar{v}}}\int _\Omega (u-{\bar{u}})\frac{ u ({\bar{v}}-v) }{\alpha +u+\beta v}dx\\&\le \frac{u_1}{\alpha }\int _\Omega \vert (u-{\bar{u}})\,(v-{\bar{v}}) \vert dx\\&\le \frac{u_1}{2\alpha }\int _\Omega (u-\bar{u})^2dx+\frac{u_1}{2\alpha }\int _\Omega (v-{\bar{v}})^2dx, \end{aligned}$$

and

$$\begin{aligned} I_3&= \int _\Omega (v-{\bar{v}}){\bar{v}} \left( \frac{\gamma u}{\alpha +u+\beta v}-1\right) dx\\&=\int _\Omega (v-{\bar{v}}){\bar{v}} \left( \frac{\gamma u}{\alpha +u+\beta v}-\frac{\gamma {\bar{u}}}{\alpha +{\bar{u}}+\beta {\bar{v}}}\right) dx\\&=\frac{\gamma {\bar{v}}}{\alpha +{\bar{u}}+\beta {\bar{v}}}\int _\Omega (v-{\bar{v}}) \frac{\alpha (u-{\bar{u}})+\beta (u{\bar{v}}-{\bar{u}} v)}{\alpha +u+\beta v} \,dx\\&= \frac{\gamma {\bar{v}}}{\alpha +{\bar{u}}+\beta {\bar{v}}}\int _\Omega (v-{\bar{v}}) \frac{\beta u({\bar{v}}-v)+(u-{\bar{u}})(\alpha + \beta v)}{\alpha +u+\beta v}\, dx\\&\le \frac{\gamma {\bar{v}}}{\alpha +{\bar{u}}+\beta {\bar{v}}}\int _\Omega (v-{\bar{v}}) \frac{(u-{\bar{u}})(\alpha +\beta v)}{\alpha +u+\beta v} \,dx\\&\le \frac{\gamma }{\beta }\int _\Omega (v-{\bar{v}}) (u-{\bar{u}})\, dx \\&\le \frac{\gamma }{2 \beta }\int _\Omega (u-\bar{u})^2dx+\frac{\gamma }{2 \beta }\int _\Omega (v-{\bar{v}})^2dx. \end{aligned}$$

Appendix B

Fig. 11

Turing pattern solution of various modes: a 19- mode solution for $\sigma =1.939$, b 20- mode solution for $\sigma =1.867$, c 21- mode solution for $\sigma =1.812$. The color of a solution curve corresponds to the same color diamond point shown in Fig. 3b. Other parameter values are $\alpha =0.07,\;\beta =0.2,\; \gamma =1.2, \;\eta =0.1$ and $d=46.$

Fig. 12

Localized pattern solutions where the colour of a solution curve corresponds to the same color point shown in Fig. 3c. Other parameter values are $\alpha =0.07,\;\beta =0.2,\; \gamma =1.2, \;\eta =0.1,\; \sigma =2.36$ and $d=46.$

Here we show 19-, 20- and 30- mode Turing solutions in Fig. 11 and few localized solutions in Fig. 12.

Appendix C

Fig. 13

Periodic travelling wave solution at different times: a $t=100$, b $t=500,$ and c $t=605$. Here, other parameter values are $\alpha =0.07,\;\beta =0.2, \;\gamma =1.2,\;\eta =0.1,\;\sigma =1.82,$ and $d=10$

We consider the parameter set $\alpha =0.07,\;\beta =0.2,\; \gamma =1.2, \;\eta =0.1,\; \sigma =1.82,$ and $d=10$. We choose the same initial condition as in (33). Figure 13 shows the non-monotonic character of the travelling wave at an initial stage. However, as time progresses, new periodic peaks appear at the left side of the domain and these peaks oscillate with time. Note that, a stable limit cycle exists around the coexisting equilibrium point $E_*$ for the temporal system and the appearance of the periodic travelling wave is attributed to this stable limit cycle. However, the oscillation of the periodic peaks disappears resulting in the emergence of a localized pattern for larger values of d.

Appendix D

Fig. 14

a Bifurcation diagram in the $\sigma$-u plane for the ratio-dependent functional response. b A zoom version of Fig. (a) for $\sigma \in [3.9,3.98]$. The point marked SN denotes the saddle-node bifurcation threshold. Here, solid green, red dashed and black dashed curves represent stable, unstable and saddle branches of equilibria respectively. Further, solid red color curve represents the maximum and minimum of u of the unstable limit cycle. Other parameter values are $\alpha =0,\;\beta =0.2,\; \gamma =1.2$ and $\eta =0.1$ (color figure online)

Here we compare our findings with other functional responses. If we assume $\alpha =0$, then the resulting functional response is called a ratio-dependent functional response. The temporal dynamics of the system, keeping all other parameters the same with $\alpha =0$, changes significantly. Here, two coexisting equilibria $E_*^1$ and $E_*^2$ emerge through a saddle-node bifurcation, where $E_*^2$ is always a saddle-node and the stability of $E_*^1$ depends on the Hopf bifurcation threshold $\sigma _H.$ An unstable limit cycle is generated due to subcritical Hopf bifurcation at $\sigma =\sigma _H$ which vanishes due to a global homoclinic bifurcation. The temporal dynamics is summarized in the one-parameter bifurcation diagram shown in Fig. 14. The corresponding spatio-temporal model shows stationary Turing and localized patterns. It also exhibits dynamic patterns that include multiple moving pulse solution, spatio-temporal chaos, and travelling wave.

Fig. 15

Localized patterns for the system (12) with Holling type-II functional response: a $d=90$, b $d=100$, c $d=110$. Here, other parameter values are $\alpha =0.07,\;\beta =0,\; \gamma =1.11,\;\eta =0.1$ and $\sigma =2$

However, the chosen functional response becomes Holling type-II functional response for $\beta =0$. Then the system can have at most one coexisting equilibrium point $E_*$, whose feasibility comes from a transcritical bifurcation. However, the stability of $E_*$ is independent of the parameter $\sigma .$ If we take the parameter $\gamma =1.11,$ then the unique coexisting equilibrium point $E_*$ is asymptotically stable (whenever it exists) for all values of $\sigma$. Although the system does not produce Turing pattern but it exhibits localized pattern. We have shown few localized patterns for different values of diffusion parameter d in Fig. 15. Apart from the stationary pattern, the system also shows dynamic patterns that include multiple moving pulse solution, spatio-temporal chaos and travelling wave solution.