Theoretical growth rate of microalgae under high/low-flashing light

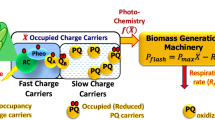
Abstract

Dynamic light regimes strongly impact microalgal photosynthesis efficiency. Finding the optimal way to supply light is then a tricky problem, especially when the growth rate is inhibited by overexposition to light and, at the same time, there is a lack of light in the deepest part of the culture. In this paper, we use the Han model to study the theoretical microalgal growth rate by applying periodically two different light intensities. Two approaches are considered depending on the period of the light pattern. For a large light period, we demonstrate that the average photosynthetic rate can be improved under some conditions. Moreover, we can also enhance the growth rate at steady state as given by the PI-curve. Although, these conditions change through the depth of a bioreactor. This theoretical improvement in the range of 10–15% is due to a recovery of photoinhibited cells during the high irradiance phase. We give a minimal value of the duty cycle for which the optimal irradiance is perceived by the algae culture under flashing light regime.

Appendices

Appendix A: Analytical computations

1.1 Case I: Large period T

For the large period case, the evolution system (7) can be approximated by (10) which can be solved explicitly for a constant irradiance I as

$$\begin{aligned} C(y,t)=\frac{\alpha (I(y))}{\alpha (I(y))+k_r}(1-e^{-(\alpha (I(y))+k_r)t})+e^{-(\alpha (I(y))+k_r)t}C(y,0), \quad t\in (0,T). \end{aligned}$$

Moreover, the system is assumed to be periodic (i.e. $C(y,0)=C(y,T)$). For the constant light regime $I_M(y)$, one has $C(y,t) = \frac{\alpha (I_M(y))}{\alpha (I_M(y))+k_r}$, $\forall t\in [0,T]$. Using (18), the T-average growth rate is given by $(1-C)\gamma (I_M(y)) = \frac{K\sigma I_M(y)}{1+\tau \sigma I_M(y)+\frac{k_d}{k_r}\tau (\sigma I_M(y))^2} = \mu _S(I_M(y))$. For the high/low light regime, one has

$$\begin{aligned}{} & {} C(y,t)\\{} & {} =\left\{ \begin{array}{ll} \frac{\alpha _H(y)}{\alpha _H(y)+k_r}(1-e^{-(\alpha _H(y)+k_r)t})+e^{-(\alpha _H(y)+k_r)t}C(y,0), &{} t\in (0,\eta T) \\ \frac{\alpha _L(y)}{\alpha _L(y)+k_r}(1-e^{-(\alpha _L(y)+k_r)(t-\eta T)})+e^{-(\alpha _L(y)+k_r)(t-\eta T)}C(y,\eta T), &{} t\in (\eta T,T) \end{array}\right. \end{aligned}$$

Using the periodic border condition of C, one has

$$\begin{aligned} C(y,0) = \frac{ \frac{\alpha _L(y)}{\alpha _L(y)+k_r}\big (1-e^{-(\alpha _L(y)+k_r)T(1-\eta )}\big ) + e^{-(\alpha _L(y)+k_r)T(1-\eta )}\frac{\alpha _H(y)}{\alpha _H(y)+k_r}\big (1-e^{-(\alpha _H(y)+k_r)T\eta }\big ) }{1-e^{-(\alpha _L(y)+k_r)T(1-\eta )-(\alpha _H(y)+k_r)T\eta }}. \end{aligned}$$

For a given local optical depth y, the T-average growth rate is given by

$$\begin{aligned} {\bar{\mu }}^{\text {T}}(y)= & {} \frac{1}{T}\int _0^T\mu (y,t)\textrm{d} t\\= & {} \frac{1}{T}\big (\int _0^{\eta T}\mu (y,t)\textrm{d} t + \int _{\eta T}^T\mu (y,t)\textrm{d} t\big )\\= & {} \eta \frac{\gamma _H(y)k_r}{\alpha _H(y)+k_r} + (1-\eta )\frac{\gamma _L(y)k_r}{\alpha _L(y)+k_r}\\{} & {} +\frac{\gamma _H(y)}{T(\alpha _H(y)+k_r)}\left( \frac{\alpha _H(y)}{\alpha _H(y)+k_r}-C(y,0)\right) \left( 1-e^{-(\alpha _H(y)+k_r)T\eta }\right) \\{} & {} +\frac{\gamma _L(y)}{T(\alpha _L(y)+k_r)}\left( \frac{\alpha _L(y)}{\alpha _L(y)+k_r}-C(y,T\eta )\right) \left( 1-e^{-(\alpha _L(y)+k_r)T(1-\eta )}\right) \\= & {} \eta \mu _S(I_{H}(y))+(1-\eta )\mu _S(I_{L}(y)) + \frac{\zeta _1(y,\eta ,T)\zeta _2(y)}{T k_r}, \end{aligned}$$

where $\gamma _H(y) := \gamma (I_H(y))$ and $\gamma _L(y) := \gamma (I_L(y))$.

1.2 Case II: Small period T

For small period case, the dynamics of C is negligible (i.e. C is a constant). Integrating (7) from 0 to T gives

$$\begin{aligned} 0 = \int _0^T \dot{C} \textrm{d} t = -k_r T C - k_d\sigma C\int _0^T I \textrm{d} t + k_d\sigma \int _0^T I \textrm{d} t - k_d\sigma \int _0^T I A \textrm{d} t. \end{aligned}$$

Let us denote by ${\bar{I}}:=\frac{1}{T}\int _0^T I \textrm{d} t$ and by ${\overline{IA}}:=\frac{1}{T}\int _0^T I A \textrm{d} t$, then one finds the constant value for C as

$$\begin{aligned} C = \frac{k_d\sigma ({\bar{I}} - {\overline{IA}})}{k_d\sigma {\bar{I}}+k_r}. \end{aligned}$$

(A1)

For high/low light regime, one has

$$\begin{aligned} A(y,t) = \left\{ \begin{array}{lr} e^{-\beta _H(y)t}A(0) + \frac{1-C}{\tau \beta _H(y)}(1-e^{-\beta _H(y)t}), &{} t\in [0,\eta T],\\ e^{-\beta _L(y)(t-\eta T)}A(\eta T) + \frac{1-C}{\tau \beta _L(y)}(1-e^{-\beta _L(y)(t-\eta T)}), &{} t\in [\eta T,T]. \end{array} \right. \end{aligned}$$

The periodicity on A gives

$$\begin{aligned} A(y,0) = \frac{e^{-\beta _L(y)(1 - \eta )T}\frac{1-C}{\tau \beta _H(y)}(1 - e^{-\beta _H(y)\eta T}) + \frac{1-C}{\tau \beta _L(y)}(1 - e^{-\beta _L(y)(1 - \eta ) T})}{1-e^{-\beta _L(y)(1 - \eta )T - \beta _H(y)\eta T}}. \end{aligned}$$

On the other hand, the average of IA can be computed by

$$\begin{aligned} T\overline{I A}= & {} \int _0^{\eta T}I_H(y) A(y,t)\textrm{d} t + \int _{\eta T}^T I_L(y) A(y,t)\textrm{d} t\\= & {} A(y,0)I_H(y)\frac{1 - e^{-\beta _H(y)\eta T}}{\beta _H(y)} + I_H(y)\frac{1-C}{\tau \beta _H(y)}(\eta T - \frac{1 - e^{-\beta _H(y)\eta T}}{\beta _H(y)}) \\{} & {} + A(y,\eta T)I_L(y)\frac{1 - e^{-\beta _L(y)(1-\eta )T}}{\beta _L(y)} + I_L(y)\frac{1-C}{\tau \beta _L(y)}\big ((1-\eta )T \\{} & {} - \frac{1 - e^{-\beta _L(y)(1-\eta )T}}{\beta _L(y)}\big )\\= & {} A(y,0)I_H(y)\frac{1 - e^{-\beta _H(y)\eta T}}{\beta _H(y)} + I_H(y)\frac{1-C}{\tau \beta _H(y)}(\eta T - \frac{1 - e^{-\beta _H(y)\eta T}}{\beta _H(y)})\\{} & {} + \big (e^{-\beta _H(y)\eta T}A(0) + \frac{1-C}{\tau \beta _H(y)}(1-e^{-\beta _H(y)\eta T})\big )I_L(y)\frac{1 - e^{-\beta _L(y)(1-\eta )T}}{\beta _L(y)}\\{} & {} + I_L(y)\frac{1-C}{\tau \beta _L(y)}\big ((1-\eta )T - \frac{1 - e^{-\beta _L(y)(1-\eta )T}}{\beta _L(y)}\big )\\= & {} \frac{A(y,0)}{\beta _H(y)\beta _L(y)}\big (I_H(y)\beta _L(y)(1-e^{-\beta _H(y)\eta T}) + I_L(y)\beta _H(y) e^{-\beta _H(y)\eta T}(1\\{} & {} - e^{-\beta _L(y)(1-\eta ) T})\big ) + \frac{1-C}{\tau \beta _H(y)\beta _L(y)}(I_H(y)\beta _L(y)\eta T \!+\! I_L(y)\beta _H(y)(1-\eta )T) \\{} & {} + \frac{1-C}{\tau \beta _L(y)^2\beta _H(y)^2}\big (I_L(y)\beta _H(y)\beta _L(y)(1 - e^{-\beta _H(y)\eta T})(1-e^{-\beta _L(y)(1-\eta ) T})\\{} & {} - I_H(y)\beta _L(y)^2(1-e^{-\beta _H(y)\eta T}) - I_L(y)\beta _H(y)^2(1-e^{-\beta _L(y)(1-\eta ) T})\big )\\= & {} \frac{1-C}{\tau \beta _L(y)^2\beta _H(y)^2}\frac{1}{1-e^{-\beta _L(y)(1 - \eta )T - \beta _H(y)\eta T}}\big (I_H(y)\beta _L(y)^2(1\\{} & {} - e^{-\beta _H(y)\eta T})^2e^{-\beta _L(y)(1-\eta ) T} + I_L(y)\beta _L(y)\beta _H(y) e^{-\beta _L(y)(1 - \eta )T - \beta _H(y)\eta T}(1\\{} & {} - e^{-\beta _H(y)\eta T})(1-e^{-\beta _L(y)(1-\eta ) T}) + I_H(y)\beta _L(y)\beta _H(y)(1\\{} & {} - e^{-\beta _H(y)\eta T})(1-e^{-\beta _L(y)(1-\eta ) T}) + I_L(y)\beta _H(y)^2e^{-\beta _H(y)\eta T}(1\\{} & {} - e^{-\beta _L(y)(1-\eta ) T})^2 + I_L(y)\beta _H(y)\beta _L(y)(1-e^{-\beta _H(y)\eta T})(1\\{} & {} - e^{-\beta _L(y)(1-\eta ) T})(1-e^{-\beta _L(y)(1 - \eta )T - \beta _H(y)\eta T}) - I_H(y)\beta _L(y)^2(1\\{} & {} - e^{-\beta _H(y)\eta T})(1-e^{-\beta _L(y)(1 - \eta )T - \beta _H(y)\eta T}) - I_L(y)\beta _H(y)^2(1\\{} & {} - e^{-\beta _L(y)(1-\eta ) T})(1-e^{-\beta _L(y)(1 - \eta )T - \beta _H(y)\eta T})\big )\\{} & {} + \frac{1-C}{\tau \beta _H(y)\beta _L(y)}(I_H(y)\beta _L(y)\eta T + I_L(y)\beta _H(y)(1-\eta )T).\\ \end{aligned}$$

To simplify the notations, let us denote by $a:=-(\sigma I_{\max } + \frac{1}{\tau })\eta T$ and by $i:=-(\sigma I_{\min } + \frac{1}{\tau })(1-\eta )T$, then $A(0)=\frac{1-C}{\tau \beta _L\beta _H(y)}\frac{1}{1-e^{a+i}}(\beta _L e^i(1-e^a) + \beta _L(1-e^i))$ and the previous computation can be written as (we omitted the dependence on y)

$$\begin{aligned} T\overline{I A}= & {} A(0)I_H\frac{1-e^a}{\beta _H} + I_H\frac{1-C}{\tau \beta _H}(\eta T -\frac{1-e^a}{\beta _H}) + e^a A(0)I_L\frac{1-e^i}{\beta _L} \\{} & {} + \frac{1-C}{\tau \beta _H}(1-e^a)I_L\frac{1-e^i}{\beta _L} + I_L\frac{1-C}{\tau \beta _L}((1-\eta )T - \frac{1-e^i}{\beta _L})\\= & {} \frac{A(0)}{\beta _H\beta _L}(I_H\beta _H(1-e^a) + I_L\beta _L e^a(1-e^i)) \\{} & {} + \frac{1-C}{\tau \beta _H\beta _L}(I_H\beta _L\eta T + I_L\beta _H(1-\eta )T)\\{} & {} +\frac{1-C}{\tau \beta _H^2\beta _L^2}\big (I_L\beta _H\beta _L(1-e^a)(1-e^i) - I_H\beta _L^2(1-e^a) - I_L\beta _H^2(1-e^i)\big )\\= & {} \frac{1-C}{\tau \beta _H^2\beta _L^2}\frac{1}{1-e^{a+i}}\big (I_H\beta _L^2(1-e^a)^2e^i + I_L\beta _H\beta _L e^{a+i}(1-e^a)(1-e^i) \\{} & {} + I_H\beta _H\beta _L(1-e^a)(1-e^i) + I_L\beta _H^2e^a(1-e^i)^2 + I_L\beta _H\beta _L(1-e^a)(1-e^i)\\{} & {} \times (1-e^{a+i})- I_H\beta _L^2(1-e^a)(1-e^{a+i}) - I_L\beta _H^2(1-e^i)(1-e^{a+i})\big ) \\{} & {} + \frac{1-C}{\tau \beta _H\beta _L}(I_H\beta _L\eta T + I_L\beta _H(1-\eta )T)\\= & {} \frac{1-C}{\tau \beta _H^2\beta _L^2}\frac{1}{1-e^{a+i}}\big (I_H\beta _L^2(1-e^a)(e^i-1) + I_H\beta _L^2(e^a-1)(1-e^i) \\{} & {} + I_H\beta _L\beta _L(1-e^a)(1-e^i) \\{} & {} + I_H\beta _L\beta _L(1-e^a)(1-e^i)\big ) + \frac{1-C}{\tau \beta _L\beta _L}(I_H\beta _L\eta T + I_H\beta _L(1-\eta )T)\\= & {} \frac{1-C}{\tau \beta _L^2\beta _L^2}\frac{(1-e^a)(1-e^i)}{1-e^{a+i}}\big (I_H\beta _L\beta _L + I_H\beta _L\beta _L - I_H\beta _L^2 - I_H\beta _L^2\big ) \\ {}{} & {} +\frac{1-C}{\tau \beta _L\beta _L}(I_H\beta _L\eta T + I_H\beta _L(1-\eta )T)\\= & {} \frac{1-C}{\tau \beta _L^2\beta _L^2}\frac{(1-e^a)(1-e^i)}{1-e^{a+i}}(I_H\beta _L-I_H\beta _L)(\beta _L-\beta _L)\\{} & {} + \frac{1-C}{\tau \beta _L\beta _L}(I_H\beta _L\eta T + I_H\beta _L(1-\eta )T). \end{aligned}$$

By using the definition of $I_H,I_H,\beta _L,\beta _L$, one has $(I_H\beta _L-I_H\beta _L)(\beta _L-\beta _L)=\frac{\sigma }{\tau }(I_{\max }-I_{\min })^2$ and $I_H\beta _L\eta T + I_H\beta _L(1-\eta )T=(\frac{I_{M}}{\tau }+\sigma I_{\max }I_{\min })T$. Replacing C by (A1) in the previous equation gives

$$\begin{aligned} \begin{aligned} T\overline{I A}&= \frac{k_d \sigma {\overline{IA}} + k_r}{\tau \beta _L^2\beta _L^2(k_d\sigma I_{\eta } + k_r)}\Big (\Delta (y,T)\frac{\sigma }{\tau }(I_{\max }-I_{\min })^2 \\&\quad + (\frac{I_{\eta }}{\tau }+\sigma I_{\max }I_{\min })\beta _L\beta _L T\Big ), \end{aligned} \end{aligned}$$

where $\delta (y,T)=\frac{(1-e^a)(1-e^i)}{1-e^{a+i}}$. In other words

$$\begin{aligned} \begin{aligned} {\overline{IA}} =\frac{k_r\Big (\frac{\Delta (y,T)}{T\beta _L\beta _L}\frac{\sigma }{\tau }(I_{\max }-I_{\min })^2+ \frac{I_{\eta }}{\tau }+\sigma I_{\max }I_{\min } \Big )}{\tau \beta _L\beta _L(k_d\sigma I_{\eta } + k_r) - k_d\sigma \Big (\frac{\Delta (y,T)}{T\beta _L\beta _L}\frac{\sigma }{\tau }(I_{\max }-I_{\min })^2+ \frac{I_{\eta }}{\tau }+\sigma I_{\max }I_{\min } \Big ) }. \end{aligned} \end{aligned}$$

We have that

$$\begin{aligned} \Theta :=\frac{\Delta (y,T)(1+\tau \sigma I_{\eta }(y))}{(1+\tau \sigma I_H(y))(1+\tau \sigma I_L(y))\eta (1-\eta )}\rightarrow 1 \text { as }T\rightarrow 0, \end{aligned}$$

and we can manipulate the value ${\overline{IA}}$ to get

$$\begin{aligned} {\overline{IA}} =\frac{\frac{k_rI_{\eta }}{(1+\tau \sigma I_{\eta })\beta _L\beta _L\tau ^2}\left( \tau ^2\beta _L\beta _L\right) +(\Theta -1)\tau \sigma (I_L-I_H)^2\eta (1-\eta )}{k_r+k_d\sigma I_{\eta }-k_d\sigma \frac{I_{\eta }}{(1+\tau \sigma )\tau ^2\beta _L\beta _L}\left( \tau ^2\beta _L\beta _L\right) +(\Theta -1)\tau \sigma (I_L-I_H)^2\eta (1-\eta )}, \end{aligned}$$

and rearranging this last equality, we get

$$\begin{aligned} {\bar{\mu }}^{\text {T}}(y) =\frac{K \sigma k_r I_M(y)\big (1+\xi _1(y)\xi _2(y,T)\big )}{k_r+k_r\tau \sigma I_M(y)+k_d\tau \big (\sigma I_M(y)\big )^2+k_d\sigma I_M(y)\xi _1(y)\xi _2(y,T)},\nonumber \\ \end{aligned}$$

(A2)

where

$$\begin{aligned} \xi _1(y)&= \frac{\sigma \big (I_H(y)-I_L(y)\big )^2\eta (1-\eta )}{\tau \beta _H(y)\beta _L(y)},\\ \xi _2(y,T)&= \frac{(1-e^{-\beta _H(y)\eta T})(1-e^{-\beta _L(y)(1-\eta )T})}{T(1-e^{-\beta _H(y)\eta T-\beta _L(y)(1-\eta )T})}\frac{\eta \beta _H(y)+(1-\eta )\beta _L(y)}{\eta (1-\eta )\beta _H(y)\beta _L(y)}-1. \end{aligned}$$

1.3 Proof of Lemma 1

We split equation (7) into the high/low-flashing light configuration

$$\begin{aligned} \left\{ \begin{array}{ll} \frac{\mathrm d}{dt}\begin{pmatrix} A(y,t)\\ C(y,t) \end{pmatrix}=-M_H(y)\begin{pmatrix} A(y,t)\\ C(y,t) \end{pmatrix}+N_H(y), &{}\text {if } t<\eta T,\\ \frac{\mathrm d}{\mathrm dt}\begin{pmatrix} A(y,t)\\ C(y,t) \end{pmatrix}=-M_L(y)\begin{pmatrix} A(y,t)\\ C(y,t) \end{pmatrix}+N_L(y),&\text {if } t>\eta T. \end{array}\right. \end{aligned}$$

One can solve this system by using the variation of parameters method and get

$$\begin{aligned} \begin{array}{ll} \begin{pmatrix} A(y,t)\\ C(y,t) \end{pmatrix}=\left( \mathop {\textrm{Id}}\limits -e^{-tM_H(y)}\right) M_H^{-1}(y)N_H(y)+e^{-tM_H(y)}\begin{pmatrix} A(y,0)\\ C(y,0) \end{pmatrix}, &{} \text {if } t<\eta T,\\ \begin{pmatrix} A(y,t)\\ C(y,t) \end{pmatrix}=\left( \mathop {\textrm{Id}}\limits -e^{-(t-\eta T)M_L(y)}\right) M_L^{-1}(y)N_L(y)+e^{-(t-\eta T)M_L(y)}\begin{pmatrix} A(y,\eta T)\\ C(y,\eta T) \end{pmatrix},&\text {if } t>\eta T, \end{array} \end{aligned}$$

where $\mathop {\textrm{Id}}\limits$ denotes the identity matrix in ${\mathbb {R}}^{2\times 2}$. Imposing then periodic conditions, i.e, $(A(y,0),C(y,0)) = (A(y,T),C(y,T))$, one can evaluate the values of (A(y, 0), C(y, 0)) and $(A(y,\eta T),C(y,\eta T))$:

$$\begin{aligned} \begin{pmatrix} A(y,\eta T)\\ C(y,\eta T) \end{pmatrix}&=\left( \mathop {\textrm{Id}}\limits -e^{-\eta TM_H(y)}\right) M_H^{-1}(y)N_H(y)+e^{-\eta TM_H(y)}\begin{pmatrix} A(y,0)\\ C(y,0) \end{pmatrix}, \end{aligned}$$

(A3)

$$\begin{aligned} \begin{pmatrix} A(y,0)\\ C(y,0) \end{pmatrix}&=\left( \mathop {\textrm{Id}}\limits -e^{-(1-\eta )TM_L(y)}\right) M_L^{-1}(y)N_L(y)+e^{-(1-\eta )TM_L(y)}\begin{pmatrix} A(y,\eta T)\\ C(y,\eta T) \end{pmatrix}. \end{aligned}$$

(A4)

Replacing finally (A4) in (A3) and vice versa, we obtain

$$\begin{aligned} \begin{aligned} \begin{pmatrix} A(y,\eta T)\\ C(y,\eta T) \end{pmatrix}&=\left( \mathop {\textrm{Id}}\limits -e^{-\eta TM_H(y)}e^{-(1-\eta )T M_L(y)}\right) ^{-1}\\&\quad \cdot \left[ \left( \mathop {\textrm{Id}}\limits -e^{-\eta T M_H(y)}\right) M_H^{-1} (y)N_H(y)\right. \\ {}&\quad \quad +\left. e^{-\eta TM_H(y)}\left( \mathop {\textrm{Id}}\limits -e^{-(1-\eta )T M_L(y)}\right) M^{-1}_L(y)N_L(y)\right] , \end{aligned}\nonumber \\ \end{aligned}$$

(A5)

$$\begin{aligned} \begin{aligned} \begin{pmatrix} A(y,0)\\ C(y,0) \end{pmatrix}&=\left( \mathop {\textrm{Id}}\limits -e^{-(1-\eta ) TM_L(y)}e^{-\eta T M_H(y)}\right) ^{-1}\\&\quad \cdot \left[ \left( \mathop {\textrm{Id}}\limits -e^{-(1-\eta ) T M_L(y)}\right) M_L^{-1}(y)N_L(y)\right. \\ {}&\quad \quad \left. +e^{-(1-\eta ) TM_L(y)}\left( \mathop {\textrm{Id}}\limits -e^{-\eta T M_H(y)}\right) M^{-1}_H(y)N_H(y)\right] . \end{aligned}\nonumber \\ \end{aligned}$$

(A6)

The inverse matrix $(Id-e^{-\eta TM_H}e^{-(1-\eta ) TM_L})$ exists because the matrix $e^{-\eta TM_H} e^{-(1-\eta ) TM_L}$ has no eigenvalue equal to 1. The solution founded it is then unique.

1.4 Exact growth rate

To calculate the T-average of the growth rate, we calculate the integral $\int _0^TI(y,t)(A(y, t),C(y,t))dt$:

$$\begin{aligned}&\int _0^TI(y,t)\begin{pmatrix} A(y,t)\\ C(y,t) \end{pmatrix}dt = I_H(y)\int _0^{\eta T}\begin{pmatrix} A(y,t)\\ C(y,t) \end{pmatrix}dt+I_L(y)\int _{\eta T}^{T}\begin{pmatrix} A(y,t)\\ C(y,t) \end{pmatrix}dt\nonumber \\&\begin{aligned}&\quad = \eta T I_H(y) M_H^{-1}(y)N_H(y)-I_H(y)M_H^{-1}(y)\left( \mathop {\textrm{Id}}\limits -e^{-\eta TM_H(y)}\right) \\ {}&\qquad \times \left[ M_H^{-1}(y)N_H(y)-\begin{pmatrix} A(y,0)\\ C(y,0) \end{pmatrix}\right] +I_L(y)(1-\eta ) TM_L^{-1}(y)N_L(y)\\ {}&\qquad -I_L(y)M_L^{-1}(y)\left( \mathop {\textrm{Id}}\limits -e^{-(1-\eta ) TM_L(y)}\right) \left[ M_L^{-1}(y)N_L(y)-\begin{pmatrix} A(y,\eta T)\\ C(y,\eta T) \end{pmatrix}\right] . \end{aligned}\nonumber \\ \end{aligned}$$

(A7)

Using (A5) we can compute

$$\begin{aligned}&M^{-1}_{L}(y)N_L(y)-\begin{pmatrix} A(y,\eta T)\\ C(y,\eta T) \end{pmatrix}\\&\begin{aligned}&= M^{-1}_{L}(y)N_L(y)-\left( \mathop {\textrm{Id}}\limits -e^{-\eta TM_H(y)}e^{-(1-\eta )T M_L(y)}\right) ^{-1}\\&\quad \cdot \left[ \left( \mathop {\textrm{Id}}\limits -e^{-\eta T M_H(y)}\right) M_H^{-1} (y)N_H(y)\right. \\ {}&\quad \quad +\left. e^{-\eta TM_H(y)}\left( \mathop {\textrm{Id}}\limits -e^{-(1-\eta )T M_L(y)}\right) M^{-1}_L(y)N_L(y)\right] \end{aligned}\\&\begin{aligned}&= \left( \mathop {\textrm{Id}}\limits -e^{-\eta TM_H(y)}e^{-(1-\eta )T M_L(y)}\right) ^{-1}\\&\quad \cdot \left[ (\mathop {\textrm{Id}}\limits -e^{-\eta TM_H(y)})M_L^{-1}(y)N_L(y) -(\mathop {\textrm{Id}}\limits -e^{-\eta TM_H(y)})M_H^{-1}(y)N_H(y) \right. \\&\quad \left. -e^{-\eta T M_H(y)}(\mathop {\textrm{Id}}\limits -e^{-(1-\eta )T M_H (y)})M^{-1}_L(y)N_L(y)\right] \end{aligned}\\&\begin{aligned}&=\left( \mathop {\textrm{Id}}\limits -e^{-\eta TM_H(y)}e^{-(1-\eta )T M_L(y)}\right) ^{-1}\left[ M_L^{-1}(y)N_L(y) \right. \\&\quad -e^{-\eta TM_H(y)}e^{-(1-\eta )TM_L(y)}M_L^{-1}(y)N_L(y) -M_H^{-1}(y)N_H(y)\\&\quad +e^{-\eta TM_H(y)}M_H^{-1}(y)N_H(y)-e^{-\eta TM_H(y)}M_L^{-1}(y)N_L(y)\\ {}&\left. \quad +e^{-e\eta TM_H(y)}e^{-(1-\eta )TM_L(y)}M_L^{-1}N_L(y)\right] \end{aligned}\\&\begin{aligned}&=\left( \mathop {\textrm{Id}}\limits -e^{-\eta TM_H(y)}e^{-(1-\eta )T M_L(y)}\right) ^{-1}\left[ (\mathop {\textrm{Id}}\limits -e^{-\eta T M_H(y)})M^{-1}_L(y)N_L(y)\right. \\&\left. \quad -(\mathop {\textrm{Id}}\limits -e^{-\eta T M_H(y)})M^{-1}_H(y)N_H(y)\right] \end{aligned}\\&\begin{aligned}&=\left( \mathop {\textrm{Id}}\limits -e^{-\eta TM_H(y)}e^{-(1-\eta )T M_L(y)}\right) ^{-1}\cdot \\&(\mathop {\textrm{Id}}\limits -e^{-\eta TM_H(y)})(M^{-1}_L(y)N_L(y)-M^{-1}_H(y)N_H(y)). \end{aligned} \end{aligned}$$

In the same way, using (A6) we can get

$$\begin{aligned} \begin{aligned} M_H^{-1}(y)N_H(y)-\begin{pmatrix} A(y,0)\\ C(y,0) \end{pmatrix}&= \left( \mathop {\textrm{Id}}\limits -e^{-(1-\eta )TM_L(y)}e^{-\eta TM_H(y)}\right) ^{-1}\\ {}&\quad \times (\mathop {\textrm{Id}}\limits -e^{-(1-\eta )TM_L(y)})\\ {}&\quad \cdot (M_H^{-1}N_H(y)-M_L^{-1}N_L(y)). \end{aligned} \end{aligned}$$

Replacing the above calculations on (A7), we have

$$\begin{aligned} \int _0^TI(y,t)\begin{pmatrix} A(y,t)\\ C(y,t) \end{pmatrix}&= \eta TI_H(y)M^{-1}_H(y)N_H(y)+(1-\eta )TI_L(y)\nonumber \\&\quad \times M_L^{-1}(y)N_L(y)+\Delta , \end{aligned}$$

(A8)

where

$$\begin{aligned} \begin{aligned} \Delta&= \left[ I_H(y)M^{-1}_H(y)\left( \mathop {\textrm{Id}}\limits -e^{-\eta T M_H(y)}\right) \left( \mathop {\textrm{Id}}\limits -e^{-(1-\eta )TM_L(y)}e^{-\eta TM_H(y)}\right) ^{-1}\right. \\&\quad \left. \times \left( \mathop {\textrm{Id}}\limits -e^{-(1-\eta )TM_L(y}\right) -I_L(y)M^{-1}_L(y)\left( \mathop {\textrm{Id}}\limits -e^{-(1-\eta )TM_L(y}\right) \right. \\&\quad \left. \times \left( \mathop {\textrm{Id}}\limits -e^{-\eta TM_H(y)}e^{-(1-\eta )TM_L(y)}\right) ^{-1}\left( \mathop {\textrm{Id}}\limits -e^{-\eta T M_H(y)}\right) \right] \\&\qquad \cdot \left( M^{-1}_H(y)N_H(y)-M^{-1}_L(y)N_L(y)\right) . \end{aligned} \end{aligned}$$

Finally, the average growth rate $\frac{1}{T}\int _0^TK\sigma IAdt$ is proportional to the first coordinate of (A8) multiplied by $\frac{K\sigma }{T}$. Denote $\delta$ the first coordinate of $\Delta$ and note that

$$\begin{aligned} M_H^{-1}(y)N_H(y)&= \begin{pmatrix} \dfrac{k_r}{\tau k_d(\sigma I_H(y))^2+\tau k_r\sigma I_H(y)+k_r}\\ \dfrac{\tau k_d(\sigma I_H(y))^2}{\tau k_d(\sigma I_H(y))^2+\tau k_r\sigma I_H(y)+k_r} \end{pmatrix}, \end{aligned}$$

(A9)

$$\begin{aligned} M_L^{-1}(y)N_L(y)&= \begin{pmatrix} \dfrac{k_r}{\tau k_d(\sigma I_L(y))^2+\tau k_r\sigma I_L(y)+k_r}\\ \dfrac{\tau k_d(\sigma I_L(y))^2}{\tau k_d(\sigma I_L(y))^2+\tau k_r\sigma I_L(y)+k_r} \end{pmatrix}, \end{aligned}$$

(A10)

where the first coordinate in (A9) and (A10) multiplied by $K\sigma$ is exactly $\mu _S(I_H(y))$ and $\mu _S(I_L(y))$, respectively. So, the T-average of the growth rate is

$$\begin{aligned} {\bar{\mu }}^{\text {T}}(y) = \eta \mu _s(I_H(y))+(1-\eta )\mu _S(I_L(y)) -\frac{K\sigma }{T}\delta (y,T). \end{aligned}$$

(A11)

1.5 Eigenvalues of matrix M

We will condense the analysis of the eigenvalues of $M_H$ and $M_L$ in the matrix

$$\begin{aligned} M(I) =\begin{pmatrix} \sigma I+\frac{1}{\tau } &{} \frac{1}{\tau }\\ k_d\sigma I &{} k_d\sigma I+k_r \end{pmatrix}. \end{aligned}$$

Denoting $\uplambda _1$ and $\uplambda _2$ the eigenvalues, then we have

$$\begin{aligned} \text {Tr}(M(I))=\uplambda _1+\uplambda _2&= \sigma I+\frac{1}{\tau }+k_d\sigma I+k_r, \end{aligned}$$

(A12)

$$\begin{aligned} \text {Det}(M(I))=\uplambda _1\uplambda _2&= k_d(\sigma I)^2+k_r\sigma I+\frac{k_r}{\tau }. \end{aligned}$$

(A13)

From (A13), $\uplambda _1$ and $\uplambda _2$ has the same sign, and since (A12) holds, the two eigenvalues are positive.

Appendix B: Proof of Lemma 3

The second derivative of the function $\mu _S$ can be computed as

$$\begin{aligned} \frac{\mathrm d^2}{\mathrm d I^2}\mu _S(I) = -\frac{2K\sigma \left[ \tau \sigma +\frac{k_d}{k_r}\tau \sigma ^2I\left( 3-\frac{k_d}{k_r}\tau \sigma ^2I^2\right) \right] }{\left( 1+\tau \sigma I+\frac{k_d}{k_r}\tau (\sigma I)^2\right) ^3}, \end{aligned}$$

and it is zero in the point $I_c$ which satisfies

$$\begin{aligned} \left( \frac{k_d}{k_r}\tau \sigma ^2\right) ^2I_c^3-3\frac{k_d}{k_r}\tau \sigma ^2I_c-\tau \sigma =0. \end{aligned}$$

This is a depressed cubic equation, where the determinant correspond to

$$\begin{aligned} \Delta = \left( \frac{1}{\frac{k_d}{k_r}\tau \sigma ^2}\right) ^4(\tau \sigma )^2-4\left( \frac{1}{\frac{k_d}{k_r}\tau \sigma ^2}\right) ^3 \end{aligned}$$

where if $\Delta \le 0$ all the roots are real. Note that

$$\begin{aligned} \Delta \le 0 \Longleftrightarrow \tau \le 4\frac{k_d}{k_r}. \end{aligned}$$

In this case, the solutions are given by (Zwillinger 2018):

$$\begin{aligned} l\cos {\frac{\theta }{3}}, \quad l\cos {\frac{\theta +2\pi }{3}}, \quad \text {and} \quad l\cos {\frac{\theta +4\pi }{3}} \end{aligned}$$

where

$$\begin{aligned} l = \frac{2}{\sigma \sqrt{\frac{k_d}{k_r}\tau }}, \quad \text {and} \quad \theta = \arccos \left( \frac{\sqrt{\tau }}{2\sqrt{\frac{k_d}{k_r}}}\right) . \end{aligned}$$

From the three possible solutions, only $l\cos {\frac{\theta }{3}}$ is positive due to $\theta /3\in (0,\pi /6)$. In the case that $\Delta > 0$ then the real solution can be written as (Holmes 2002)

$$\begin{aligned} l\cosh \left( \frac{1}{3}\mathop {\textrm{arccosh}}\limits \left( \frac{\sqrt{\tau }}{2\sqrt{\frac{k_d}{k_r}}}\right) \right) . \end{aligned}$$