Global Aspects of Moduli Spaces of 2d SCFTs

Abstract

The Bagger–Witten line bundle is a line bundle over moduli spaces of two-dimensional SCFTs, related to the Hodge line bundle of holomorphic top-forms on Calabi–Yau manifolds. It has recently been a subject of a number of conjectures, but concrete examples have proven elusive. In this paper we propose a new, intrinsically geometric definition of the Bagger–Witten line bundle, whose restriction to the moduli spaces of complex structures of Calabi–Yau manifolds we explicitly compute in some concrete examples. We also conjecture a new criterion for UV completion of four-dimensional supergravity theories in terms of properties of the Bagger–Witten line bundle.

Picard Groups of Moduli Spaces of Elliptic Curves with Level Structures

This paper uses results for moduli spaces of elliptic curves with two-torsion points and level two structures. Although this material is well-known in the mathematics community, it is much more obscure in the physics community, and in any event many of the results we need are scattered across different sources. To make this paper self-contained, we collect here a brief review of relevant results.

1.1 Ordinary moduli spaces of elliptic curves

First, recall the fundamental domain for $SL(2,{{\mathbb {Z}}})$, as shown in Fig. 1. Solid lines indicate the boundary for the usually-drawn fundamental domain for $PSL(2,{{\mathbb {Z}}})$; dashed lines indicate the boundaries of a few other nearby possible fundamental domains. Curved edges arise from circles of radius 1 centered at integer points along the real axis, and circles of radius 1/3 centered at points k/3 along the real axis, for k an integer not divisible by 3.

Fig. 1

Fundamental domain for $SL(2,{{\mathbb {Z}}})$

There are two points on the fundamental domain with nontrivial stabilizers (beyond the generic ${{\mathbb {Z}}}_2$). One is the point i (of j-invariant 1728), whose stabilizer is ${{\mathbb {Z}}}_4 \subset SL(2,{{\mathbb {Z}}})$, which is generated by

$$\begin{aligned} S = \left[ \begin{array}{rr} 0 &{} -1 \\ 1 &{} 0 \end{array} \right] . \end{aligned}$$

(A.1)

Of these, $-I$ acts trivially on the upper-half-plane, and so the point i represents a local ${{\mathbb {Z}}}_2$ singularity in the $PSL(2,{{\mathbb {Z}}})$ quotient. The other point with nontrivial stabilizer is $\exp (2 \pi i/3) = -1/2 + i \sqrt{3}/2$. This point has stabilizer ${{\mathbb {Z}}}_6$, generated by ST for S as above and

$$\begin{aligned} T \, = \, \left[ \begin{array}{cc} 1 &{} 1 \\ 0 &{} 1 \end{array} \right] . \end{aligned}$$

(A.2)

In principle, we can read off the Picard group of the moduli space from the two stabilizers above. Briefly, using the fact that $S^2 = (ST)^3 (=-I)$, we can identify the Picard group with

$$\begin{aligned} \frac{ {{\mathbb {Z}}}_4 \times {{\mathbb {Z}}}_6 }{ (g_1^2,1) \sim (1,g_2^3) } \end{aligned}$$

(A.3)

(for $g_1$, $g_2$ the generators of the ${{\mathbb {Z}}}_4$, ${\mathbb Z}_6$ factors) which can be shown to be isomorphic to ${\mathbb Z}_{12}$, with generator $(g_1,g_2)$, corresponding to the Hodge line bundle.

Alternatively, the Picard group can be understood as the group of $SL(2,{{\mathbb {Z}}})$-equivariant line bundles on the upper half plane, and since the upper half plane is simply connected, this is simply

$$\begin{aligned} {\mathrm{Hom}}(SL(2,{{\mathbb {Z}}}),{{\mathbb {C}}}^{\times }). \end{aligned}$$

(A.4)

This group is again ${{\mathbb {Z}}}_{12}$, corresponding to the abelianization of $SL(2,{{\mathbb {Z}}})$, as determined by the generators S, ST above. (See for example [40] for a description of this approach and [41] for a very readable discussion of the abelianization.)

In this language, the holonomy $\chi$ of the corresponding flat connection is encoded as follows [41]:

$$\begin{aligned} \chi (S) \, = \, -i, \, \, \, \chi (T) \, = \, \exp (2 \pi i/12) \, =\, -i \left( \frac{-1 + i \sqrt{3} }{ 2} \right) . \end{aligned}$$

(A.5)

In particular, for a compactification on an elliptic curve, it seems that under the mirror to $B \mapsto B + 1$ around large-radius on the complex structure moduli space, corresponding to the transformation T, we see that $\chi$ is nontrivial.

Extremely readable reviews of this case can be found in [19, 40, 42, 43].

In the next sections we will discuss the Picard groups of $\mathcal{M}_1(2) = [ {{\mathfrak {h}}}/\Gamma _1(2)]$ and ${{{\mathcal {M}}}}(2) = [ {{\mathfrak {h}}}/\Gamma (2)]$, where ${{\mathfrak {h}}}$ denotes the upper half plane, and their relatives in $PSL(2,{{\mathbb {Z}}})$ and $Mp(2,{{\mathbb {Z}}})$. To that end, it may be helpful to recall some basic relations between these subgroups of $SL(2,{{\mathbb {Z}}})$. First, $\Gamma (2)$ is a normal subgroup of $SL(2,{{\mathbb {Z}}})$ with cokernel $S_3$:

$$\begin{aligned} 1 \, \longrightarrow \, \Gamma (2) \, \longrightarrow \, SL(2,{{\mathbb {Z}}}) \, \longrightarrow \, S_3 \, \longrightarrow \, 1. \end{aligned}$$

(A.6)

In addition, $\Gamma (2)$ is also a normal subgroup of $\Gamma _1(2)$:

$$\begin{aligned} 1 \, \longrightarrow \, \Gamma (2) \, \longrightarrow \, \Gamma _1(2) \, \longrightarrow \, {{\mathbb {Z}}}_2 \, \longrightarrow \, 1. \end{aligned}$$

(A.7)

Finally, $\Gamma _1(2)$ is a non-normal subgroup of $SL(2,{\mathbb Z})$ of index three.

1.2 ${{{\mathcal {M}}}}_1(2)$

Define

$$\begin{aligned} \Gamma _1(m) \, \equiv \, \left\{ \left[ \begin{array}{cc} a &{} b \\ c &{} d \end{array} \right] \, \equiv \, \left[ \begin{array}{cc} 1 &{} * \\ 0 &{} 1 \end{array} \right] \text{ mod } m \right\} , \end{aligned}$$

(A.8)

a subgroup of $SL(2,{{\mathbb {Z}}})$. (In other words, $a, d \equiv 1$ mod m and $c \equiv 0$ mod m, but b is unconstrained.)

Define ${{{\mathcal {M}}}}_1(m) \equiv [ {\mathfrak {h}}/\Gamma _1(m) ]$. Then, ${{{\mathcal {M}}}}_1(m)$ can be interpreted as a moduli space of pairs (E, p), where E is an elliptic curve and p is a single m-torsion point [45, appendix C.13]. This is because for any $\gamma \in \Gamma _1(m)$,

$$\begin{aligned} \frac{1}{m} \, \mapsto \, \frac{1}{m (c \tau + d) } \, = \, \frac{1}{m} \, + \, \frac{ (c/m) \tau + (d-1)/m }{c \tau +d} \, \sim \, \frac{1}{m}, \end{aligned}$$

(A.9)

and so the m-torsion point 1/m is preserved. In particular, ${{{\mathcal {M}}}}_1(2)$ is then a moduli space of elliptic curves with a two-torsion point fixed.

A fundamental domain for $\Gamma _1(2) \subset SL(2,{{\mathbb {Z}}})$ is shown in¹² Fig. 2, where the left vertical edge has real part $-1/2$, the right vertical edge has real part $+1/2$, and the curved edges arise from circles of radius 1 centered at integer points along the real axis, and circles of radius 1/3 centered at points k/3 along the real axis, for k an integer not divisible by 3. Solid lines are the boundaries of the the fundamental domain of $\Gamma _1(2)$, whereas dashed lines indicate boundaries of fundamental domains of $PSL(2,{{\mathbb {Z}}})$. The vertex at the lower left is at $-1/2 + i /(2 \sqrt{3})$, and the vertex at the upper right is at $+1/2 + i \sqrt{3}/2$. The fundamental domain above is essentially one half of the fundamental domain for $\Gamma (2)$, as we shall see shortly.

Fig. 2

Fundamental domain for $\Gamma _1(2)$

The lower left corner, at $-1/2 + i/(2 \sqrt{3})$, and the upper right corner, at $+1/2 + i \sqrt{3}/2$, can be related by

$$\begin{aligned} \left[ \begin{array}{cc} 1 &{} 0 \\ 2 &{} 1 \end{array} \right] \, \in \, \Gamma (2) \, \subset \, \Gamma _1(2), \end{aligned}$$

(A.10)

and so define the same point. Similarly, the point $-1/2 + i \sqrt{3}/2$, at the intersection of the three fundamental domains for $SL(2,{{\mathbb {Z}}})$, is related to the upper right point $+1/2 + i \sqrt{3}/2$ by the action of

$$\begin{aligned} \left[ \begin{array}{cc} 1 &{} 1\\ 0 &{} 1 \end{array} \right] \, \in \, \Gamma _1(2). \end{aligned}$$

(A.11)

This particular matrix lies in $\Gamma _1(2)$ but not $\Gamma (2)$, and is the essential reason why the fundamental domain for $\Gamma (2)$ looks like two copies of the fundamental domain for $\Gamma _1(2)$.

The point i is no longer a singular point, since the only elements of $\langle S \rangle$ in $\Gamma _1(2)$ are the generic stabilizer $\pm I$. However, the image of i under $U = ST$ is the point

$$\begin{aligned} \alpha \, \equiv \, ST \cdot i \, = \, \frac{i}{2} - \frac{1}{2}, \end{aligned}$$

(A.12)

lying along the left edge of the fundamental domain above, and it has stabilizer ${{\mathbb {Z}}}_4 \subset \Gamma _1(2)$ generated by

$$\begin{aligned} K \, = \, U S U^{-1} \, = \, \left[ \begin{array}{rr} -1 &{} -1 \\ 2 &{} 1 \end{array} \right] . \end{aligned}$$

(A.13)

In this case, as there is one point with stabilizer ${{\mathbb {Z}}}_4$, for reasons similar to the ordinary moduli space, we see that Pic ${{{\mathcal {M}}}}_1(2) = {{\mathbb {Z}}}_4$, and it can be shown¹³ [44] that it is generated by the Hodge line bundle. Note in passing that this means the Hodge line bundle is fractional, a line bundle on the gerbe which is not a pullback from the underlying space.

We now turn to Picard groups and Hodge line bundles on $[ {\mathfrak h}/{\tilde{\Gamma }}_1(2) ]$ and $[ {{\mathfrak {h}}}/p^* \Gamma _1(2)]$, for ${\tilde{\Gamma }}_1(2) \subset PSL(2,{{\mathbb {Z}}})$ and $p: Mp(2,{{\mathbb {Z}}}) \rightarrow SL(2,{{\mathbb {Z}}})$.

First, note that there are projection maps

$$\begin{aligned} {[} {{\mathfrak {h}}}/p^* \Gamma _1(2)] \, \longrightarrow \, [ {\mathfrak h}/ \Gamma _1(2) ] \, \longrightarrow \, [ {\mathfrak h}/{\tilde{\Gamma }}_1(2) ] \, \longrightarrow \, {{\mathfrak {h}}} / {\tilde{\Gamma }}_1(2). \end{aligned}$$

(A.16)

We construct the last space, the variety ${\mathfrak h}/{\tilde{\Gamma }}_1(2)$, as follows. Begin with the variety ${{\mathfrak {h}}}/\Gamma (2)$, which is

$$\begin{aligned} {{{\mathcal {M}}}}_{0,4} \, = \, {{\mathbb {P}}}^1 \, - \, \text{(3 } \text{ points) }, \end{aligned}$$

(A.14)

where the three points are $\{ 0, 1, \infty \}$. We can now quotient this space by a transposition of order two, defined by an involution of $S_3$, to get the variety ${{\mathfrak {h}}}/{\tilde{\Gamma }}_1(2)$. We can describe possible actions on ${{\mathbb {P}}}^1$ explicitly as follows:

• 1. $\lambda \mapsto \lambda$, the identity,

• 2. $\lambda \mapsto 1/(1-\lambda )$, which sends $0 \rightarrow 1 \rightarrow \infty \rightarrow 0$ and has order three,

• 3. $\lambda \mapsto \lambda - 1/\lambda$, which sends $0 \rightarrow \infty \rightarrow 1 \rightarrow 0$ and has order three,

• 4. $\lambda \mapsto + 1/\lambda$, which sends $0 \leftrightarrow \infty$, has order two, and leaves the points $\pm 1$ fixed,

• 5. $\lambda \mapsto \lambda / (\lambda - 1)$, which sends $1 \leftrightarrow \infty$, has order two, and leaves fixed 0, 2,

• 6. $\lambda \mapsto 1-\lambda$, which sends $0 \leftrightarrow 1$, has order two, and leaves fixed $\infty$, 1/2.

To be definite, consider the quotient by the last involution. On the quotient, which is the variety ${{\mathfrak {h}}}/ {\tilde{\Gamma }}_1(2)$, there are now two excluded points (namely $\infty$ and $0=1$), and one marked point (1/2) of nontrivial stabilizer. Denote that marked point by y.

The stack $[ {{\mathfrak {h}}}/ {\tilde{\Gamma }}_1(2)]$ is isomorphic to the variety ${{\mathfrak {h}}}/ {\tilde{\Gamma }}_1(2)$ away from the marked point y. Over y, the stack $[ {\mathfrak h}/{\tilde{\Gamma }}_1(2)]$ has stabilizer ${{\mathbb {Z}}}_2$. Denote this stabilizer $Z^p$.

The stack $[ {{\mathfrak {h}}}/\Gamma _1(2)]$ is a ${{\mathbb {Z}}}_2$ gerbe over $[ {{\mathfrak {h}}}/{\tilde{\Gamma }}_1(2)]$, and over y has stabilizer ${{\mathbb {Z}}}_4$. Denote this stabilizer Z.

The stack $[ {{\mathfrak {h}}}/p^* \Gamma _1(2)]$ is a ${{\mathbb {Z}}}_4$ gerbe over $[ {{\mathfrak {h}}}/{\tilde{\Gamma }}_1(2)]$, and we will see below that over y it has stabilizer ${{\mathbb {Z}}}_8$. Let $Z^m$ denote the stabilizer over y, then from the sequence (A.15) we have the relation between stabilizers

$$\begin{aligned} Z^m \, \longrightarrow \, Z={{\mathbb {Z}}}_4 \, \longrightarrow \, Z^p = {{\mathbb {Z}}}_2. \end{aligned}$$

(A.17)

We know that $Z^m \rightarrow Z={{\mathbb {Z}}}_4$ is surjective, with kernel equal to the kernel of $p: Mp(2,{{\mathbb {Z}}}) \rightarrow SL(2,{{\mathbb {Z}}})$, namely ${{\mathbb {Z}}}_2$, and quotient ${\mathbb Z}_4$, hence we know $Z^m$ is given by an extension

$$\begin{aligned} 1 \, \longrightarrow \, {{\mathbb {Z}}}_2 \, \longrightarrow \, Z^m \, \longrightarrow \, Z={{\mathbb {Z}}}_4 \, \longrightarrow \, 1. \end{aligned}$$

(A.18)

It remains to determine whether the extension Z is ${{\mathbb {Z}}}_2 \times {{\mathbb {Z}}}_4$ or ${{\mathbb {Z}}}_8$. If we pullback along the center of $SL(2,{{\mathbb {Z}}})$, which preserves the kernel ${\mathbb Z}_2$ above, this becomes the nontrivial extension

$$\begin{aligned} 1 \, \longrightarrow \, {{\mathbb {Z}}}_2 \, \longrightarrow \, {\mathbb Z}_4 \, \longrightarrow \, {{\mathbb {Z}}}_2 \, \longrightarrow \, 1, \end{aligned}$$

(A.19)

hence the extension (A.18) must be nontrivial, and so $Z^m = {{\mathbb {Z}}}_8$. Finally, as these stabilizers live inside gerbe structures, they define twists by line bundles (on the gerbes), and so we see in each case that the Picard group matches the stabilizer at y, as summarized in Table 1.

Since the Hodge line bundle is acted upon by the center of $SL(2,{{\mathbb {Z}}})$, it does not exist over $[ {{\mathfrak {h}}} / {\tilde{\Gamma }}_1(2)]$, and its pullback to $[ {{\mathfrak {h}}}/p^* \Gamma _1(2)]$ is the square of the generator of the Picard group there.

1.3 ${{{\mathcal {M}}}}(2)$

Define ${{{\mathcal {M}}}}(m) = [ {\mathfrak {h}}/\Gamma (m) ]$, where $\Gamma (m)$ is the (“principal congruence”) subgroup of $SL(2,{{\mathbb {Z}}})$ consisting of matrices equivalent to the identity mod m:

$$\begin{aligned} \Gamma (m) \, \equiv \, \left\{ \left. \left[ \begin{array}{cc} a &{} b \\ c &{} d \end{array} \right] \right| a \equiv 1 \text{ mod } m, d \equiv 1 \text{ mod } m, b \equiv 0 \text{ mod } m, c \equiv 0 \text{ mod } m \right\} . \end{aligned}$$

(A.20)

(The notation $\Gamma (m)$ or $SL(2,{{\mathbb {Z}}})[m]$ is sometimes used instead; however, $\Gamma (m)$ is also sometimes used to refer to the analogous subgroup of $PSL(2,{{\mathbb {Z}}})$, so to try to remove ambiguity, we will use $\Gamma (m)$ or $SL(2,{{\mathbb {Z}}})[m]$ to denote this subgroup of $SL(2,{{\mathbb {Z}}})$.)

The moduli space ${{{\mathcal {M}}}}(m)$ defined above is the moduli stack of elliptic curves with a level m structure [19, section 4.2]. The idea is that $\Gamma (m)$ preserves the level m structure.

To gain a bit of intuition, note that for $m=2$, $\Gamma (2)$ preserves a choice of spin structure on $T^2$. If we let periodicities around two cycles be denoted $(-)^m$, $(-)^n$, then under the action of an element of $\Gamma (2)$ above,

$$\begin{aligned} \left[ \begin{array}{c} (-)^m \\ (-)^n \end{array} \right] \, \mapsto \, \left[ \begin{array}{c} (-)^{am+bn} \\ (-)^{cm_dn} \end{array} \right] \, = \, \left[ \begin{array}{c} (-)^m \\ (-)^n \end{array} \right] , \end{aligned}$$

(A.21)

where the last equality follows from the fact that for an element

$$\begin{aligned} \left[ \begin{array}{cc} a &{} b \\ c &{} d \end{array} \right] \, \in \, \Gamma (2), \end{aligned}$$

(A.22)

a and d are odd, while b and c are even.

We can also interpret ${{{\mathcal {M}}}}(m)$ as a moduli space of elliptic curves with a basis of m-torsion points [45, appendix C.13]. The basic point is that $\gamma \in \Gamma (m)$ will preserve the m-torsion points including 1/m and $\tau /m$. For example, for such $\gamma$,

$$\begin{aligned} \frac{1}{m} \, \mapsto \, \frac{1}{m (c \tau + d) }= & {} \frac{1}{m} \, + \, \frac{ (c/m) \tau + (d-1)/m }{c \tau +d} \, \sim \, \frac{1}{m}, \\ \frac{\tau }{m} \, \mapsto \, \frac{\tau }{m (c \tau + d)}= & {} \frac{1}{m} \frac{a \tau + b}{c \tau + d} \, + \, \frac{d-1}{m} \frac{a \tau + b}{c \tau + d} \, - \, \frac{b}{m} \, \sim \, \frac{\tau }{m}, \end{aligned}$$

where we have used the fact that $b, c \equiv 0$ mod m and $d-1 \equiv 0$ mod m. Similarly, one can show that k/m is invariant under $\gamma$ for integer k, as are other m-torsion points. For this reason, a level m structure is equivalent to a basis $\{ 1/m, \ldots , \tau /m \}$ for the m-torsion points.

In passing, the moduli space ${{{\mathcal {M}}}}(m)$ has automorphisms given by $SL(2,{{\mathbb {Z}}}) / \Gamma (m) \cong SL(2,{{\mathbb {Z}}}_m)$. For example, for $m=2$, these automorphisms form $S_3$, and permute the three nontrivial two-torsion points of a fixed elliptic curve.

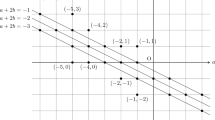
A fundamental domain for $\Gamma (2)$ is illustrated in Fig. 3 [46, section 5.5, fig. 28]. Solid lines indicate boundaries of the fundamental domain of $\Gamma (2)$. Dashed lines define boundaries between fundamental domains of $PSL(2,{{\mathbb {Z}}})$. The straight vertical boundaries lie along lines of real part $-1/2$, $+3/2$. The curved boundaries lie along circles of radius 1 centered at integer points along the real axis, and also circles of radius 1/3 centered at points k/3 along the real axis, for k an integer not divisible by 3. The vertices $\rho = -1/2+i \sqrt{3}/2$, $\rho +2$, $v = 1/2 + i/(2 \sqrt{3})$ are related by $\Gamma (2)$, and so define the same point. Clearly, the fundamental domain for $\Gamma (2)$ contains six copies of fundamental domains of $SL(2,{{\mathbb {Z}}})$. The one-point compactification of this domain is a sphere with three cusps, at 0, 1, and $\rho = \rho +2 = v$.

Fig. 3

Fundamental domain for $\Gamma (2)$

The reader should note that the fundamental domain above for $\Gamma (2)$ is precisely two copies of the fundamental domain for $\Gamma _1(2)$ given previously. The difference comes down to $T \in \Gamma _1(2)$ which is not also in $\Gamma (2)$.

The reader should also note that the point $\alpha$, which lies on the left edge of the fundamental domain previously discussed for $\Gamma _1(2)$ but is in the middle of the fundamental region above for $\Gamma (2)$, is no longer singular: the only elements of $\langle K \rangle$ which lie in $\Gamma (2)$ are $\pm I$, the stabilizer of generic points. We can also see this in another way. Recall that the fundamental domain of $SL(2,{{\mathbb {Z}}})$ had points with stabilizers $\langle S \rangle$ and $\langle S T \rangle$. It is straightforward to check that most elements of $\langle S \rangle$ and $\langle S T \rangle$ are not in $\Gamma (2)$, with the exception of the ${{\mathbb {Z}}}_2$ center of $SL(2,{{\mathbb {Z}}})$. As a result, at those potentially problematic points, the only stabilizer is the same as at every other point, the generic stabilizer defining the ${{\mathbb {Z}}}_2$ gerbe structure. The other elements of $\langle S \rangle$ and $\langle S T \rangle$ simply move between different copies of the fundamental domain of $SL(2,{{\mathbb {Z}}})$, within the fundamental domain of $\Gamma (2)$.

This is a ${{\mathbb {Z}}}_2$ gerbe over the space $M_{0,4} = {\mathbb C}-\{0,1\}$. More formally, we can describe elliptic curves as cubics in ${{\mathbb {P}}}^2 = \mathrm{Proj}\, {{\mathbb {C}}}[x,y,z]$ of the form

$$\begin{aligned} y^2 z \, = \, x (x-z) (x-\lambda z), \end{aligned}$$

(A.23)

where $\lambda$ parametrizes the family. The two-torsion points are then [47, chapter II.1]

$$\begin{aligned} (x,y,z) \, = \, (0,0,1), \, (1,0,1), \, (\lambda ,0,1), \, (0,1,0), \end{aligned}$$

(A.24)

where the point with $y=1$ corresponds to the origin and the remaining points, of $y=0$, correspond to the nonzero two-torsion points. ${{{\mathcal {M}}}}(2)$ is a moduli stack of elliptic curves with a basis of two-torsion points, and at $\lambda = 0, 1, \infty$, some of the two-torsion points collide, so we exclude those points. Hence, ${{{\mathcal {M}}}}(2)$ is a gerbe over

$$\begin{aligned} M_{0,4} \, = \, {{\mathbb {P}}}^1 - \{ 0, 1, \infty \} \, = \, {\mathbb C} - \{0, 1\}. \end{aligned}$$

(A.25)

Now, $H^2(M_{0,4}, {{\mathbb {Z}}}_k) = 0$, so any ${{\mathbb {Z}}}_2$ gerbe over $M_{0,4}$ is trivial, hence ${{{\mathcal {M}}}}(2)$ is the trivial ${{\mathbb {Z}}}_2$ gerbe over $M_{0,4}$.

Now we can compute the Picard group. Since ${{{\mathcal {M}}}}(2)$ is a trivial ${{\mathbb {Z}}}_2$ gerbe on $M_{0,4}$,

$$\begin{aligned} {\mathrm{Pic}}\, {{{\mathcal {M}}}}(2) \, = \, {{\mathbb {Z}}}_2 \times {\mathrm{Pic}}\, M_{0,4}. \end{aligned}$$

(A.26)

However, Pic $M_{0,4} = 0$, so we see that Pic ${{{\mathcal {M}}}}(2) = {{\mathbb {Z}}}_2$.

The Hodge line bundle on ${{{\mathcal {M}}}}(2)$ is the pullback¹⁴ of the Hodge line bundle on ${{{\mathcal {M}}}}$, and is necessarily nontrivial judging from the nontrivial action of the center of $SL(2,{{\mathbb {Z}}})$. (In passing, this means the pullback from Pic ${{{\mathcal {M}}}}$ to Pic ${{{\mathcal {M}}}}(2)$ is surjective.) Thus, we see that the Picard group of ${{{\mathcal {M}}}}(2)$ is generated by the Hodge line bundle, which is fractional (a line bundle on the gerbe which is not a pullback from the underlying space).

In passing, note that if we define ${\tilde{\Gamma }}(2)$ to be the analogous subgroup of $PSL(2,{{\mathbb {Z}}})$, then trivially $[ {{\mathfrak {h}}} / {\tilde{\Gamma }}(2) ] = M_{0,4}$, hence the Picard group of $[ {{\mathfrak {h}}} / {\tilde{\Gamma }}(2) ]$ is trivial.

Similarly, for p the projection $Mp(2,{{\mathbb {Z}}}) \rightarrow SL(2,{{\mathbb {Z}}})$, note $[ {{\mathfrak {h}}} / p^* \Gamma (2) ]$ is a ${{\mathbb {Z}}}_2$ gerbe over $[ {{\mathfrak {h}}} / \Gamma (2) ]$ and (since $Mp(2,{{\mathbb {Z}}})$ is a ${{\mathbb {Z}}}_4$ extension of $PSL(2,{{\mathbb {Z}}})$ a ${{\mathbb {Z}}}_4$ gerbe over $[ {\mathfrak h}/{\tilde{\Gamma }}(2)] = M_{0,4}$. Since there are no nontrivial root gerbes over $M_{0,4}$, it must be the trivial gerbe, hence

$$\begin{aligned} {\mathrm{Pic}}\, [ {{\mathfrak {h}}}/ p^* \Gamma (2) ] \, = \, {{\mathbb {Z}}}_4 \times {\mathrm{Pic}}\, M_{0,4}. \end{aligned}$$

(A.31)

Since the Picard group of $M_{0,4}$ is trivial, we then have that the Picard group of $[ {{\mathfrak {h}}}/ p^* \Gamma (2) ]$ is ${\mathbb Z}_4$.

1.4 Flat connections on moduli spaces

Although it is not directly relevant to the analyses of the rest of the paper, it seems appropriate to also list related results concerning flat U(1) connections over the moduli spaces of elliptic curves with level structures, which is what we shall do in this section.

The group ${\tilde{\Gamma }}(2) \subset PSL(2,{{\mathbb {Z}}})$ is a free group on 2 generators. (In fact, it can be identified with $\pi _1( {{\mathbb {C}}} -\{0,1\})$.) We can take the generators to be

$$\begin{aligned} \left[ \begin{array}{cc} 1 &{} 2 \\ 0 &{} 1 \end{array} \right] , \, \, \, \left[ \begin{array}{cc} 1 &{} 0 \\ 2 &{} 1 \end{array} \right] . \end{aligned}$$

(A.32)

As a result,

$$\begin{aligned} {\mathrm{Hom}}({\tilde{\Gamma }}(2), {{\mathbb {C}}}^{\times }) \, = \, {\mathbb C}^{\times } \times {{\mathbb {C}}}^{\times }. \end{aligned}$$

(A.33)

In $SL(2,{{\mathbb {Z}}})$, $\Gamma (2)$ contains $-I$, generating ${{\mathbb {Z}}}_2$, as well as the two matrices above, which generate a subgroup of $\Gamma (2)$ isomorphic to ${\tilde{\Gamma }}(2)$, hence $\Gamma (2) \cong {{\mathbb {Z}}}_2 \times {\tilde{\Gamma }}(2)$, and

$$\begin{aligned} {\mathrm{Hom}}( \Gamma (2), {{\mathbb {C}}}^{\times }) \, = \, {{\mathbb {Z}}}_2 \times {\mathrm{Hom}}( {\tilde{\Gamma }}(2), {{\mathbb {C}}}^{\times }) \, = \, {{\mathbb {Z}}}_2 \times {{\mathbb {C}}}^{\times } \times {\mathbb C}^{\times }. \end{aligned}$$

(A.34)

The group $\Gamma _1(2) \subset SL(2,{{\mathbb {Z}}})$ can be defined either as the group of matrices

$$\begin{aligned} \left[ \begin{array}{cc} a &{} b \\ c &{} d \end{array} \right] \end{aligned}$$

(A.29)

such that

• 1. $a \cong 1 \text{ mod } 2$, $d \cong 1 \text{ mod } 2$, $c \cong 0 \text{ mod } 2$, which is the specialization of the definition for $\Gamma _1(N)$ for general N, or equivalently for the case $N=2$,

• 2. $c \cong 0 \text{ mod } 2$.

We can see that the second implies the first, for $N=2$, as follows: if c is even, then in order for the determinant to be 1, neither diagonal entry can be even. The result follows. Technically, the second case is sometimes denoted $\Gamma _0(2)$, hence this means that $\Gamma _1(2) = \Gamma _0(2)$.

Let ${\tilde{\Gamma }}_1(2) \subset PSL(2,{{\mathbb {Z}}})$ denote the image of $\Gamma _1(2)$. The group ${\tilde{\Gamma }}_1(2)$ is also a free product, but of ${{\mathbb {Z}}}_2$ and ${{\mathbb {Z}}}$ instead of two copies of ${{\mathbb {Z}}}$. The generators are the images in $PSL(2,{{\mathbb {Z}}})$ of

$$\begin{aligned} \left[ \begin{array}{cc} 1 &{} -1 \\ 2 &{} -1 \end{array} \right] , \, \, \, \left[ \begin{array}{cc} 1 &{} 1 \\ 0 &{} 1 \end{array} \right] . \end{aligned}$$

(A.35)

The first matrix has order 4 in $SL(2,{{\mathbb {Z}}})$, and its image in $PSL(2,{{\mathbb {Z}}})$ has order 2, whereas the second matrix has infinite order, hence

$$\begin{aligned} {\mathrm{Hom}}( {\tilde{\Gamma }}_1(2), {{\mathbb {C}}}^{\times } )= & {} {{\mathbb {Z}}}_2 \times {{\mathbb {C}}}^{\times }. \end{aligned}$$

In this case, $\Gamma _1(2)$ does not contain a subgroup isomorphic to ${\tilde{\Gamma }}_1(2)$. The matrices (A.35) generate $\Gamma _1(2)$. More formally, $\Gamma _1(2)$ is the free product with amalgamation of ${{\mathbb {Z}}}_4$, generated by the first matrix, and ${\mathbb Z}_2 \times {{\mathbb {Z}}}$, generated by $\pm 1$ times the second matrix, with amalgamation along the common subgroups of order 2. Hence,

$$\begin{aligned} {\mathrm{Hom}}( \Gamma _1(2), {{\mathbb {C}}}^* ) \, = \, {{\mathbb {Z}}}_4 \times {{\mathbb {C}}}^{\times }. \end{aligned}$$

(A.36)

Since $PSL(2,{{\mathbb {Z}}})$ is the free product of ${{\mathbb {Z}}}_2$ and ${{\mathbb {Z}}}_3$, its abelianization is ${{\mathbb {Z}}}_6$ and

$$\begin{aligned} {\mathrm{Hom}}( PSL(2,{{\mathbb {Z}}}), {{\mathbb {C}}}^{\times }) \, = \, {{\mathbb {Z}}}_6. \end{aligned}$$

(A.37)

Similarly, the abelianization of $SL(2,{{\mathbb {Z}}})$ is ${\mathbb Z}_{12}$ and

$$\begin{aligned} {\mathrm{Hom}}( SL(2,{{\mathbb {Z}}}), {{\mathbb {C}}}^{\times }) \, = \, {{\mathbb {Z}}}_{12}. \end{aligned}$$

(A.38)

Let $p: Mp(2,{{\mathbb {Z}}}) \rightarrow SL(2,{{\mathbb {Z}}})$ be projection. Recall $Mp(2,{{\mathbb {Z}}})$ has a unique nontrivial element of order 2, and $p^* -1$ is the set of 2 elements of order 4 which are central in $Mp(2,{{\mathbb {Z}}})$.

As a result, $p^* \Gamma (2)$ is the direct product of ${\mathbb Z}_4$ and a free group of rank 2, hence

$$\begin{aligned} {\mathrm{Hom}}( p^* \Gamma (2), {{\mathbb {C}}}^{\times }) \, = \, {\mathbb Z}_4 \times {{\mathbb {C}}}^{\times } \times {{\mathbb {C}}}^{\times }. \end{aligned}$$

(A.39)

Similarly,

$$\begin{aligned} {\mathrm{Hom}}( p^* \Gamma _1(2), {{\mathbb {C}}}^{\times }) \, = \, {\mathbb Z}_8 \times {{\mathbb {C}}}^{\times }. \end{aligned}$$

(A.40)

As a technical aside, note that the abelianization of a group G is the set of cocharacters of Hom$(G, {{\mathbb {C}}}^{\times })$, not the Hom group itself. For example,

$$\begin{aligned} {\mathrm{Hom}}( \Gamma (2), {{\mathbb {C}}}^{\times }) \, = \, {{\mathbb {Z}}}_2 \times {{\mathbb {C}}}^{\times } \times {{\mathbb {C}}}^{\times }, \end{aligned}$$

(A.41)

but the abelianization of $\Gamma (2)$ is ${{\mathbb {Z}}}_2 \times {{\mathbb {Z}}} \times {{\mathbb {Z}}}$. (In particular, the abelianization of a discrete group cannot contain a ${{\mathbb {C}}}^{\times }$.)

1.5 Summary

In Table 1 we summarize the results of this Appendix, on Picard groups, Hodge line bundles, and flat connections on stacks of the form $[ {{\mathfrak {h}}}/G]$, for ${{\mathfrak {h}}}$ the upper half plane.

Table 1 Listed here are Picard groups, Hodge line bundles, and Hom’s for the examples described in this Appendix

Note that for each of $[ {{\mathfrak {h}}} / SL(2,{{\mathbb {Z}}}) ]$, $[ {{\mathfrak {h}}}/ \Gamma (2) ]$, and $[ {{\mathfrak {h}}} / \Gamma _1(2) ]$, the Hodge line bundle generates the Picard group. As a result, in each case, to construct a square root of the Hodge line bundle, one must replace the original quotient by a quotient by a ${\mathbb Z}_2$ extension. In particular, in $[ {{\mathfrak {h}}} / Mp(2,{\mathbb Z}) ]$, $[ {{\mathfrak {h}}} / p^* \Gamma (2) ]$, $[ {{\mathfrak {h}}} / p^* \Gamma _1(2) ]$, the Hodge line bundle is the square of the generator of the Picard group, so we can identify the Bagger–Witten line bundle with the generator itself.

It is also worth noting that in each of the cases above, the Hodge line bundle only exists over a gerby quotient—the Hodge line bundle does not exist over the effectively-acting quotients ${{\mathfrak {h}}} / PSL(2,{{\mathbb {Z}}})$, ${{\mathfrak {h}}} / {\tilde{\Gamma }}(2)$, or ${{\mathfrak {h}}} / {\tilde{\Gamma }}_1(2)$.