Abstract
We consider a class of Schrödinger operators—referred to as Schrödinger operators over circle maps—that generalize one-frequency quasiperiodic Schrödinger operators, with a base dynamics given by an orientation-preserving homeomorphism of a circle \({\mathbb {T}}^1={\mathbb {R}}/{\mathbb {Z}}\), instead of a circle rotation. In particular, we consider Schrödinger operators over circle diffeomorphisms with a single singular point where the derivative has a jump discontinuity (circle maps with a break) or vanishes (critical circle maps). We show that in a two-parameter region—determined by the geometry of dynamical partitions and \(\alpha \)—the spectrum of Schrödinger operators over every sufficiently smooth such map, is purely singular continuous, for every \(\alpha \)-Hölder-continuous potential V. As a corollary, we obtain that for every sufficiently smooth such map, with an invariant measure \(\mu \) and with rotation number in a set \({\mathcal {S}}\) depending on the class of the considered maps, and \(\mu \)-almost all \(x\in {\mathbb {T}}^1\), the corresponding Schrödinger operator has a purely continuous spectrum, for every Hölder-continuous potential V. For circle maps with a break, this set includes some Diophantine numbers with a Diophantine exponent \(\delta \), for any \(\delta >1\).
Similar content being viewed by others
Data Availability
This article uses no data.
Notes
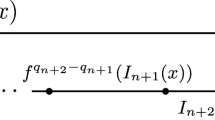
\( {\hat{P}}_n-\widetilde{P}_n=\sum _{i=0}^{n-1}{\hat{A}}_{n-1}\dots {\hat{A}}_{i+1}({\hat{A}}_i-\widetilde{A}_i)\widetilde{A}_{i-1}\dots \widetilde{A}_0\).
References
Avila, A.: Global theory of one-frequency Schrödinger operators. Acta Math. 215, 1–54 (2015)
Avila, A., You, J., Zhou, Q.: Sharp phase transitions for the almost Mathieu operator. Duke Math. J. 166(14), 2697–2718 (2017)
Avron, J., Simon, B.: Singular continuous spectrum for a class of almost periodic Jacobi matrices. Bull. Am. Math. Soc. 6(1), 81–85 (1982)
Cunha, K., Smania, D.: Renormalization for piecewise smooth homeomorphisms on the circle. Ann. Inst. H. Poincaré Anal. Non Linéaire 30(3), 441–462 (2013)
Cunha, K., Smania, D.: Rigidity for piecewise smooth homeomorphisms on the circle. Adv. Math. 250, 193–226 (2014)
Damanik, D.: Schrödinger operators with dynamically defined potentials. Ergod. Theory Dyn. Syst. 37(6), 1681–1764 (2017)
de Faria, E., de Melo, W.: Rigidity of critical circle mappings I. J. Eur. Math. Soc. 1(4), 339–392 (1999)
Furman, A.: On the multiplicative ergodic theorem for uniquely ergodic systems. Ann. Inst. H. Poincaré (B) Probab. Stat. 33(6), 797–815 (1997)
Furstenberg, H.: Strict ergodicity and transformation of the torus. Am. J. Math. 83(4), 573–601 (1961)
Gordon, A.Y.: The point spectrum of the one-dimensional Schrödinger operator. Uspekhi Mat. Nauk 31(4), 257–258 (1976)
Guarino, P., Martens, M., de Melo, W.: Rigidity of critical circle maps. Duke Math. J. 167(11), 2125–2188 (2018)
Herman, M.R.: Sur la conjugasion differentiable des difféomorphismes du cercle a de rotations. Publ. Math. Inst. Hautes Etudes Sci. 49, 5–234 (1979)
Jitomirskaya, S.: Ergodic Schrödinger operators (on one foot). Proc. Sympos. Pure Math. 76(2), 613–647 (2007)
Jitomirskaya, S., Kocić, S.: Spectral theory of Schrödinger operators over circle diffeomorphisms. Int. Math. Res. Not., 2022(13), 9810–9829 (2022)
Jitomirskaya, S., Marx, C.A.: Dynamics and spectral theory of quasi-periodic Schrödinger-type operators. Ergod. Theory Dyn. Syst. 37(8), 2353–2393 (2017)
Khanin, K., Kocić, S.: Renormalization conjecture and rigidity theory for circle diffeomorphisms with breaks. Geom. Funct. Anal. 24(6), 2002–2028 (2014)
Khanin, K., Kocić, S., Mazzeo, E.: \(C^1\)-rigidity of circle diffeomorphisms with breaks for almost all rotation numbers. Ann. Sci. Éc. Norm. Supér. 50, 1163–1203 (2017)
Khanin, K., Teplinsky, A.: Robust rigidity for circle diffeomorphisms with singularities. Invent. Math. 169, 193–218 (2007)
Khanin, K.M., Vul, E.B.: Circle homeomorphisms with weak discontinuities. Adv. Sov. Math. 3, 57–98 (1991)
Kocić, S.: Generic rigidity for circle diffeomorphisms with breaks. Commun. Math. Phys. 344(2), 427–445 (2016)
Kotani, S.: Ljapunov indices determine absolutely continuous spectra of stationary random one-dimensional Schrödinger operators, Stochastic Analysis (Katata/Kyoto, 1982), pp. 225– 247, North-Holland Math. Library, vol. 32. North-Holland, Amsterdam (1984)
Kotani, S.: One-dimensional Random Schrödinger Operators and Herglotz Functions, Probabilistic Methods in Mathematical Physics (Katata/Kyoto, 1985), pp. 219–250. Academic Press, Boston (1987)
Kotani, S.: Generalized Floquet theory for stationary Schrödinger operators in one dimension. Chaos Solitons Fractals 8(11), 1817–1854 (1997)
Last, Y., Simon, B.: Eigenvalues, transfer matrices and absolutely continuous spectrum of one-dimensional Schrödinger operators. Invent. Math. 135(2), 329–367 (1999)
Sinai, Ya.G., Khanin, K.M.: Smoothness of conjugacies of diffeomorphisms of the circle with rotations. Uspekhi Mat. Nauk 44(1), 57–82 (1989)
Swiatek, G.: Rational rotation numbers for maps of the circle. Commun. Math. Phys. 119(1), 109–128 (1988)
Yoccoz, J.-C.: Conjugaison differentiable des difféomorphismes du cercle donc le nombre de rotation vérifie une condition Diophantienne. Ann. Sci. Éc. Norm. Supér. 17, 333–361 (1984)
Yoccoz, J.-C.: Il n’y a pas de contre-example de Denjoy analytique, C. R. Acad. Sc. Paris 298, Série I 7, 141–144 (1984)
Acknowledgements
I am grateful to Svetlana Jitomirskaya for sparking my interest in the spectral theory of Schrödinger operators and for her hospitality during my visit to the University of California Irvine. This material is based upon work supported in part by the National Science Foundation EPSCoR RII Track-4 # 1738834 and the University of Mississippi College of Liberal Arts Summer Research Grant.
Author information
Authors and Affiliations
Corresponding author
Ethics declarations
Conflict of interest
The author states that there is no conflict of interest.
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Proof of Lemma 3.2
Proof of Lemma 3.2
Let \(\zeta ^*\) be a point such that \(f'(\zeta ^*)=1\). Such a point exists, for sufficiently large k, since, by assumption, the first and the last intervals are of the same order, and on the interval \(B_{K'}\) (which is non-empty for sufficiently large k), the function is convex. We will perform an affine orientation-preserving change of variables
that maps \(\zeta ^*\) into 0 and normalizes the second derivative of f there. Under this change of variables f is transformed into \(g=h\circ f\circ h^{-1}\) which satisfies \(g'(0)=1\) and \(g''(0)=2\). Let \(\kappa :=g(0)=\min _y\{g(y)-y\}\). Since f is \(C^{2+\alpha }\)-smooth, so is g, and from (A.1), we have
where \(\mathfrak {C}>0\).
Proof of Lemma 3.2 uses some estimates proved in [18].
Lemma A.1
([18]) Suppose that, for a sequence of real numbers \(\{s_i\}_{i\ge 0}\), there exist \(\mathfrak {C}_1>0\) and \(\alpha \in (0,1)\) such that \(|s_{i+1}-(s_i-s_i^2)|\le \mathfrak {C}_1|s_i|^{2+\alpha }\), for every \(i\ge 0\). Then, there exist constants \(D_1>0\) and \(d_1\in (0,1)\) such that, as long as \(s_0\in (0,d_1]\), the estimate
holds, for every \(i\ge 0\). Moreover, there exists \(D_2>0\) such that
where \(|\delta _i|\le D_2s_0^{\alpha }\), for all \(i\ge 0\), as long as \(s_0\in (0,d_1]\).
Lemma A.2
([18]) Suppose that, for a sequence of real numbers \(\{s_i\}_{i\ge 0}\), there exist \(\mathfrak {C}_2, \mathfrak {C}_3>0\) and \(\kappa ,\alpha \in (0,1)\) such that
-
1.
\(|s_0|\le \mathfrak {C}_2\kappa \),
-
2.
\(|s_{i+1}-(\kappa +s_i+s_i^2)|\le \mathfrak {C}_3|s_i|^{2+\alpha }\), for every \(i\ge 0\).
Fix arbitrary \(\mathfrak {C}_4>0\) and define \(N=\kappa ^{-1/2}\tan ^{-1}(\mathfrak {C}_4\kappa ^{-\frac{\alpha }{2(2+\alpha )}})\). Then, there exist constants \(D_3>0\) and \(d_2\in (0,1)\) such that, as long as \(\kappa \in (0,d_2]\), the following estimate holds for every \(0\le i\le N\),
where \(a_0=\tan ^{-1}(s_0/\sqrt{\kappa })\). Moreover, there exists \(D_4>0\) such that
where \(|\delta _i|\le D_4\kappa ^{\frac{\alpha (\alpha +1)}{2(2+\alpha )}}\), for all \(0\le i<N\), as long as \(\kappa \in (0,d_2]\).
Proof of Lemma 3.2
Let a and b be the left and right end points of I. Let \(t_0=h(a)\) and \(t_i=g^i(t_0)\), i.e., \(t_i=h(f^i(a))\).
Since \(\kappa =g(0)\), there exists a unique number \(i_c\) satisfying \(0<i_c<k\) such that \(t_{i_c}\in [0,\kappa )\). Let \(i_l=i_c-[\kappa ^{-1/2}\tan ^{-1}\kappa ^{-\frac{\alpha }{2(2+\alpha )}}]\) and \(i_r=i_c+[\kappa ^{-1/2}\tan ^{-1}\kappa ^{-\frac{\alpha }{2(2+\alpha )}}]\). Combining \(\tan ^{-1}\frac{1}{x}=\frac{\pi }{2}-\tan ^{-1}x\) with \(\tan ^{-1}x=x+{\mathcal {O}}(x^3)\), \(x\rightarrow 0\), it is easy to derive the following asymptotic formula
To obtain the desired estimates for \(i_l\le i\le i_r\), we can apply Lemma A.2. To obtain the estimates for \(i_l\le i< i_c\), we can apply this lemma to \(s_i=-(t_{i_c-i}-\kappa )\), where \(0\le i\le i_c-i_l\). It immediately follows from this lemma that, for \(i_l\le i< i_c\),
It is not difficult to check that the function \(\chi (\sqrt{\kappa } i)=\frac{\sqrt{\kappa }i}{\cos (\sqrt{\kappa }(i_c-i-1))}\) is monotonically increasing on \(i_l\le i< i_c\). This follows from the fact that the function \(\sqrt{\kappa } i\tan (\sqrt{\kappa } (i_c-i)-1)\) has maximum when \(\sqrt{\kappa } i=\frac{\tan (\sqrt{\kappa } (i_c-i-1))}{1+\tan ^2(\sqrt{\kappa } (i_c-i)-1)}\) and, therefore, \(\chi '(\sqrt{\kappa } i)=\frac{1-\sqrt{\kappa }i\tan (\sqrt{\kappa }(i_c-i-1))}{\cos (\sqrt{\kappa }(i_c-i-1))}\ge (\cos (\sqrt{\kappa }(i_c-i-1)))^{-1}(1+\tan ^2 (\sqrt{\kappa }(i_c-i-1))^{-1}>0\), for \(i_l\le i<i_c\). Since \( i_c=\frac{k}{2}+{\mathcal {O}}(\kappa ^{-\frac{1-\alpha }{2}})= \frac{\pi }{2}\kappa ^{-\frac{1}{2}}+{\mathcal {O}}(\kappa ^{-\frac{1-\alpha }{2}})\) as \(\kappa \rightarrow 0\) (Lemma 3.19 in [17]) and, from asymptotic formula (A.7), \(i_l=\kappa ^{-\frac{1}{2+\alpha }}+{\mathcal {O}}(\kappa ^{-\frac{1-\alpha }{2}})\) and
the function \(\frac{\kappa i^2}{i^2(\cos (\sqrt{\kappa } (i_c-i-1)))^2}\) is bounded and the claim follows for \(i_l\le i< i_c\). Here, we have also used the fact that, since the second derivative of f is bounded both from above and from below by positive constants, the lengths of the intervals \([t_{i-1},t_{i}]\) and \(\Delta _i\) are of the same order. Similarly, we can obtain the desired estimates for \(i_c\le i\le i_r\), by applying Lemma A.2 to \(s_i=t_{i_c+i}\), where \(0\le i\le i_r-i_c\).
For \(0\le i\le i_l\) and \(i_r< i\le k\), we can obtain the desired estimates by applying Lemma A.1. This is a consequence of the convexity and the fact that it follows from (A.5), using the (A.7), that \(t_{i_l}=\kappa ^{\frac{1}{2+\alpha }}+{\mathcal {O}}(\kappa ^{\frac{1}{2+\alpha }+\frac{\alpha (\alpha +1)}{2(2+\alpha )}})\) and, similarly, \(t_{i_r}=\kappa ^{\frac{1}{2+\alpha }}+{\mathcal {O}}(\kappa ^{\frac{1}{2+\alpha }+\frac{\alpha (\alpha +1)}{2(2+\alpha )}})\). We first obtain the estimates for \(0\le i<i_l\). For \(0\le i< i_l-j\), let \(s_i=-t_{i+j}\). For sufficiently large k, and some fixed large j, \(s_0\in (0,d_1]\). Since, for such i’s, \(\kappa < \mathrm{const.} |t_{i+j}|^{2+\alpha }\), it follows from (A.2) that \(s_i\) satisfy the assumptions of Lemma A.1. We can apply this lemma for \(0\le i< i_l-j\). The estimate (A.4) immediately gives us the desired bounds for \(1\le i< i_l\). Similarly, by defining \(s_i=t_{k-j-i}\), for \(0\le i\le i_r-j\), for some large j, we again have \(s_0\in (0,d_1]\), for sufficiently large k. Since \(\kappa < \mathrm{const.} |t_{k-j-i}|^{2+\alpha }\), it again follows from (A.2) that \(s_i\) satisfy the assumptions of Lemma A.1. The estimate (A.4) of Lemma A.1 immediately gives us the desired estimates for \(k-j_r< i\le k\). \(\square \)
Rights and permissions
Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.
About this article
Cite this article
Kocić, S. Singular continuous phase for Schrödinger operators over circle maps. Math. Ann. (2023). https://doi.org/10.1007/s00208-023-02646-2
Received:
Revised:
Accepted:
Published:
DOI: https://doi.org/10.1007/s00208-023-02646-2