Wage inequality and induced innovation in a classical-Marxian growth model

Abstract

The present paper works out a classical-Marxian growth model with an endogenous direction of technical change and a heterogeneous labour force, made up of high-skilled and low-skilled workers. It draws on the Kaleckian mark-up pricing to link wage inequality to the relative unit labour cost at the firm level; on growth cycle models à la Goodwin to formalize the dynamic interaction between labour market and distributive shares of income; on the induced innovation literature to link the bias of technical change to the firm’s choice of the optimal combination of factor-augmenting technologies. We find that, in contrast to the neoclassical literature on skill-biased technical change, the institutional framework that governs the distributional conflict is the ultimate determinant of both wage inequality and the direction of technical change. A decline in low-skilled workers’ bargaining strength or a rise in product market concentration led to both an increase in wage inequality and a bias of technical change favouring high-skilled over low-skilled labour productivity growth. As opposed to the Goodwin model with induced technical change and homogeneous labour force, labour market institutions thus affect steady-state income distribution, capital accumulation and labour productivity growth, and no necessary trade-off arises between labour market regulation and employment. Finally, if the steady-state value of wage inequality exceeds a critical value, an exogenous increase in the mark-up or in the high-skilled workers’ bargaining power allow both capitalists and high-skilled workers to increase their income shares at the expense of the low-skilled workers.

Appendices

Appendix 1

Remind that ${\phi }_{{\widehat{a}}_{H}{\widehat{a}}_{L}}^{{\prime}{\prime}}=0$. Then, substituting Eqs. (17) and (18) into Eqs. (15) and (16), we find:

$${\phi }_{{\widehat{a}}_{H}}{\prime}\left\{{f}^{H}\left[z,\mu \left(e,\gamma \right)\right]\right\}=-\frac{z}{\mu \left(e,\gamma \right)\left(1+z\right)}$$

(28)

$${\phi }_{{\widehat{a}}_{L}}^{\mathrm{^{\prime}}}\left\{{f}^{L}\left[z,\mu \left(e,\gamma \right)\right]\right\}=-\frac{1}{\mu (e,\gamma )(1+z)}$$

(29)

Totally differentiating Eqs. (28) and (29) with respect to $z$ and $\mu$ and rearranging, we have:

$${f}_{z}^{H{\prime}}=-\frac{1}{\mu \left(e,\gamma \right){\left(1+z\right)}^{2}}\frac{1}{{\phi }_{{\widehat{a}}_{H}{\widehat{a}}_{H}}^{{\prime}{\prime}}}>0$$

(30)

$${f}_{z}^{L\mathrm{^{\prime}}}=\frac{1}{\mu \left(e,\gamma \right){\left(1+z\right)}^{2}}\frac{1}{{\phi }_{{\widehat{a}}_{L}{\widehat{a}}_{L}}^{\mathrm{^{\prime}}\mathrm{^{\prime}}}}<0$$

(31)

$${f}_{\mu }^{H{\prime}}=\frac{z}{{\left[\mu \left(e,\gamma \right)\right]}^{2}\left(1+z\right)}\frac{1}{{\phi }_{{\widehat{a}}_{H}{\widehat{a}}_{H}}^{{\prime}{\prime}}}<0$$

(32)

$${f}_{\mu }^{L\mathrm{^{\prime}}}=\frac{1}{{\left[\mu \left(e,\gamma \right)\right]}^{2}\left(1+z\right)}\frac{1}{{\phi }_{{\widehat{a}}_{L}{\widehat{a}}_{L}}^{\mathrm{^{\prime}}\mathrm{^{\prime}}}}<0$$

(33)

It follows that:

$${\phi }_{{\widehat{a}}_{H}}{\prime}=z{\phi }_{{\widehat{a}}_{L}}{\prime}$$

(34)

$${f}_{z}^{H\mathrm{^{\prime}}}=-\rho {f}_{z}^{L\mathrm{^{\prime}}}$$

(35)

$${f}_{\mu }^{H{\prime}}=\rho z{f}_{\mu }^{L{\prime}}$$

(36)

where $\rho \equiv {\phi }_{{\widehat{a}}_{L}{\widehat{a}}_{L}}^{{\prime}{\prime}}/{\phi }_{{\widehat{a}}_{H}{\widehat{a}}_{H}}^{{\prime}{\prime}}$.

Appendix 2

Taking logarithms of Eq. (5), after substituting from Eq. (12), and differentiating with respect to time, we find:

$$\frac{{\dot{\omega }}_{H}}{{\omega }_{H}}=\frac{\dot{z}}{z}-\frac{{\mu }_{e}{\prime}\dot{e}}{1+\mu }-\frac{\dot{z}}{1+z}=\frac{1}{1+z}\frac{\dot{z}}{z}-\frac{{\mu }_{e}{\prime}e}{1+\mu }\frac{\dot{e}}{e}={\omega }_{L}\left[\left(1+\mu \right)\frac{\dot{z}}{z}-{\mu }_{e}{\prime}e(1+z)\frac{\dot{e}}{e}\right]$$

(37)

Taking logarithms of Eq. (6), after substituting from Eq. (12), and differentiating with respect to time, we find:

$$\frac{{\dot{\omega }}_{L}}{{\omega }_{L}}=-\frac{{\mu }_{e}{\prime}\dot{e}}{1+\mu }-\frac{\dot{z}}{1+z}=-\left[\frac{{\mu }_{e}{\prime}e}{1+\mu }\frac{\dot{e}}{e}+\frac{z}{1+z}\frac{\dot{z}}{z}\right]=-{\omega }_{L}\left[z\left(1+\mu \right)\frac{\dot{z}}{z}+{\mu }_{e}{\prime}e(1+z)\frac{\dot{e}}{e}\right]$$

(38)

Thus, $\dot{z}/z=\dot{e}/e=0$ implies ${\dot{\omega }}_{H}/{\omega }_{H}={\dot{\omega }}_{L}/{\omega }_{L}=0$.

Taking logarithms of $\pi =1-{\omega }_{H}-{\omega }_{L}$ and differentiating with respect to time, we find:

$$\frac{\dot{\pi }}{\pi }=-\left(\frac{{\dot{\omega }}_{H}}{\pi }+\frac{{\dot{\omega }}_{L}}{\pi }\right)=-\left(\frac{{\dot{\omega }}_{H}}{{\omega }_{H}}\frac{{\omega }_{H}}{\pi }+\frac{{\dot{\omega }}_{L}}{{\omega }_{L}}\frac{{\omega }_{L}}{\pi }\right)=-\frac{{\omega }_{L}}{\pi }\left(z\frac{{\dot{\omega }}_{H}}{{\omega }_{H}}+\frac{{\dot{\omega }}_{L}}{{\omega }_{L}}\right)$$

(39)

Thus, ${\dot{\omega }}_{H}/{\omega }_{H}={\dot{\omega }}_{L}/{\omega }_{L}=0$ implies $\dot{\pi }/\pi =0$.

Taking logarithms of Eq. (5), after substituting from Eq. (12), and differentiating with respect to time, we find:

$$\begin{array}{l}\frac{\dot{p}}{p}=\frac{{\mu }_{e}{\prime}\dot{e}}{1+\mu }+\frac{{\dot{w}}_{L}}{{w}_{L}}-\frac{{\dot{a}}_{L}}{{a}_{L}}+\frac{\dot{z}}{1+z}=\frac{{\dot{w}}_{L}}{{w}_{L}}-\frac{{\dot{a}}_{L}}{{a}_{L}}+\frac{{\mu }_{e}{\prime}e}{1+\mu }\frac{\dot{e}}{e}+\frac{z}{1+z}\frac{\dot{z}}{z}=\\ =\frac{{\dot{w}}_{L}}{{w}_{L}}-\frac{{\dot{a}}_{L}}{{a}_{L}}+{\omega }_{L}\left[z\left(1+\mu \right)\frac{\dot{z}}{z}+{\mu }_{e}{\prime}e(1+z)\frac{\dot{e}}{e}\right]\end{array}$$

(40)

Thus, $\dot{z}/z=\dot{e}/e=0$ implies $\dot{p}/p={\dot{w}}_{L}/{w}_{L}-{\dot{a}}_{L}/{a}_{L}$.

Using Eq. (20), we have:

$$\begin{array}{l}\frac{\dot{p}}{p}=\frac{{\mu }_{e}{\prime}e}{1+\mu }\frac{\dot{e}}{e}+\frac{z}{1+z}\frac{\dot{z}}{z}+\frac{{\dot{w}}_{H}}{{w}_{H}}-\frac{{\dot{a}}_{H}}{{a}_{H}}-\frac{\dot{z}}{z}=\frac{{\dot{w}}_{H}}{{w}_{H}}-\frac{{\dot{a}}_{H}}{{a}_{H}}+\frac{{\mu }_{e}{\prime}e}{1+\mu }\frac{\dot{e}}{e}-\frac{1}{1+z}\frac{\dot{z}}{z}=\\ =\frac{{\dot{w}}_{H}}{{w}_{H}}-\frac{{\dot{a}}_{H}}{{a}_{H}}-{\omega }_{L}\left[\left(1+\mu \right)\frac{\dot{z}}{z}-{\mu }_{e}{\prime}e(1+z)\frac{\dot{e}}{e}\right]\end{array}$$

(41)

Thus, $\dot{z}/z=\dot{e}/e=0$ implies $\dot{p}/p={\dot{w}}_{H}/{w}_{H}-{\dot{a}}_{H}/{a}_{H}$.

Appendix 3

Differentiating Eqs. (25) and (26) with respect to $z$, we find:

$${\phi }_{{\widehat{a}}_{H}}{\prime}{f}_{z}^{H{\prime}}+{\phi }_{{\widehat{a}}_{L}}{\prime}{f}_{z}^{L{\prime}}+\left({\phi }_{{\widehat{a}}_{H}}{\prime}{f}_{\mu }^{{H}{\prime}}+{\phi }_{{\widehat{a}}_{L}}{\prime}{f}_{\mu }^{{L}{\prime}}\right){\mu }_{e}{\prime}{\left.\frac{de}{dz}\right|}_{AK}=0$$

(42)

$$- \left({f}_{z}^{{H}{\prime}}-{f}_{z}^{{L}{\prime}}\right)+\left[{h}_{e}{\prime}-\left({f}_{\mu }^{{H}{\prime}}-{f}_{\mu }^{{L}{\prime}}\right){\mu }_{e}{\prime}\right]{\left.\frac{de}{dz}\right|}_{Z}=0$$

(43)

After simplifying and rearranging, we have:

$${\left.\frac{de}{dz}\right|}_{AK}=-\frac{\left(1-\rho z\right){f}_{z}^{{L}{\prime}}}{\left(1+\rho {z}^{2}\right){f}_{\mu }^{{L}{\prime}}{\mu }_{e}{\prime}}$$

(44)

$${\left.\frac{de}{dz}\right|}_{Z}=-\frac{\left(1+\rho z\right){f}_{z}^{{L}^{\mathrm{^{\prime}}}}}{{h}_{e}^{\mathrm{^{\prime}}}+\left(1-\rho z\right){f}_{\mu }^{{L}^{\mathrm{^{\prime}}}}{\mu }_{e}^{\mathrm{^{\prime}}}}$$

(45)

Accordingly, ${\left.de/dz\right|}_{AK}>0$ if and only if $z<\overline{z }$, whereas ${\left.de/dz\right|}_{Z}>0$ if and only if ${h}_{e}{\prime}+{\Gamma }_{\mu }{\mu }_{e}{\prime}>0$ (remind that ${\Gamma }_{\mu }\equiv \left(1-\rho z\right){f}_{\mu }^{{L}{\prime}}$).

Differentiating Eqs. (25) and (26) with respect to $\gamma$, we find:

$$\left({\phi }_{{\widehat{a}}_{H}}{\prime}{f}_{\mu }^{{H}{\prime}}+{\phi }_{{\widehat{a}}_{L}}{\prime}{f}_{\mu }^{{L}{\prime}}\right){\mu }_{\gamma }{\prime}+\left({\phi }_{{\widehat{a}}_{H}}{\prime}{f}_{\mu }^{{H}{\prime}}+{\phi }_{{\widehat{a}}_{L}}{\prime}{f}_{\mu }^{{L}{\prime}}\right){\mu }_{e}{\prime}{\left.\frac{\partial e}{\partial \gamma }\right|}_{AK}=0$$

(46)

$$- \left({f}_{\mu }^{{H}{\prime}}-{f}_{\mu }^{{L}{\prime}}\right){\mu }_{\gamma }{\prime}+\left[{h}_{e}{\prime}-\left({f}_{\mu }^{{H}{\prime}}-{f}_{\mu }^{{L}{\prime}}\right){\mu }_{e}{\prime}\right]{\left.\frac{\partial e}{\partial \gamma }\right|}_{Z}=0$$

(47)

After simplifying and rearranging, we have:

$${\left.\frac{\partial e}{\partial \gamma }\right|}_{AK}=-\frac{{\mu }_{\gamma }{\prime}}{{\mu }_{e}{\prime}}>0$$

(48)

$${\left.\frac{\partial e}{\partial \gamma }\right|}_{Z}=-\frac{\left(1-\rho z\right){f}_{\mu }^{{L}{\prime}}{\mu }_{\gamma }{\prime}}{{h}_{e}{\prime}+\left(1-\rho z\right){f}_{\mu }^{{L}{\prime}}{\mu }_{e}{\prime}}$$

(49)

Accordingly, ${\left.\partial e/\partial \gamma \right|}_{Z}>0$ if and only if ${h}_{e}{\prime}+{\Gamma }_{\mu }{\mu }_{e}{\prime}>0$ (remind that ${\Gamma }_{\mu }\equiv \left(1-\rho z\right){f}_{\mu }^{{L}{\prime}}$).

Appendix 4

For the numerical simulation, we specify the functional forms of Eqs. (10), (12), and (13), as follows:

$$\phi =-\frac{1}{2\rho }{\widehat{a}}_{H}^{2}-\frac{1}{2}{\widehat{a}}_{L}^{2}-a{\widehat{a}}_{H}-b{\widehat{a}}_{L}+\tau ,\;\; a>0, b>0, \rho >0, \tau >0$$

(50)

$$\frac{{\dot{w}}_{H}}{{w}_{H}}=\alpha +\frac{\lambda }{1-e}, \;\;\;\alpha <0, \lambda >0$$

(51)

$$\mu =\frac{\gamma }{e},\;\;\; \gamma >0$$

(52)

Equation (50) is a quadratic function for the innovation possibility frontier with ${\phi }_{{\widehat{a}}_{L}{\widehat{a}}_{L}}^{{\prime}{\prime}}=-1$. We assume a non-linear specification for the relation between wage and employment, as in Desai et al. (2006), but we express it as a nominal Phillips curve (Eq. (51)). Equation (53) is a non-linear specification for the relation between mark-up and high-skilled employment rate, such that if $e\to 1$, then $\mu \to \gamma$ and $\pi \to \gamma /(1+\gamma )$, if $e\to 0$, then $\mu \to \infty$ and $\pi \to 1$.

In this case, ${\widehat{a}}_{H}$ and ${\widehat{a}}_{L}$ are given by:

$${\widehat{a}}_{H}=\left[- a+\frac{ez}{\gamma (1+z)}\right]\rho$$

(53)

$${\widehat{a}}_{L}=- b+\frac{e}{\gamma (1+z)}$$

(54)

Thus, the dynamical system becomes:

$$\frac{{\dot{a}}_{K}}{{a}_{K}}=\frac{1}{2}\left({a}^{2}\rho +{b}^{2}+2\tau \right)-\frac{1}{2}\left[\frac{1+\rho {z}^{2}}{{\gamma }^{2}{\left(1+z\right)}^{2}}\right]{e}^{2}$$

(55)

$$\frac{\dot{z}}{z}= \alpha -\beta +a\rho -b+\frac{\lambda \gamma \left(1+z\right)+(1-\rho z)\left(1-e\right)e}{\gamma \left(1+z\right)\left(1-e\right)}$$

(56)

$$\frac{\dot{e}}{e}=\frac{{\dot{a}}_{K}}{{a}_{K}}+\frac{e}{\gamma +e}\left[\frac{\gamma +(\gamma +se)z}{e(1+z)}\right]{a}_{K}-\left[- a+\frac{ez}{\gamma (1+z)}\right]\rho -n$$

(57)

In the baseline scenario (Fig. 1a), we set the parameters as follows:

$$\begin{array}{c}\begin{array}{ccc}\tau =0.058& a=1.185& \begin{array}{ccc}b=1.09& \rho =0.8& s=0.2\end{array}\end{array}\\ \begin{array}{ccc}\alpha =-0.2& \beta =0.04& \begin{array}{ccc}\gamma =0.345& \lambda =0.05& n=0.01\end{array}\end{array}\end{array}$$

(58)

Figures 2a, 3a, 4a display the long-run equilibrium values corresponding to a 1-percentage-point increase in $\alpha$ (i.e. $\alpha =-0.19$), a 1-percentage-point increase in $\beta$ (i.e. $\beta =0.05$), and a 0.5-percentage-points increase in $\gamma$ (i.e. $\gamma =0.35$), respectively.

Figure

Fig. 5

Convergence to the steady state in the baseline scenario. a) Case I: ${z}^{*}<\overline{z }$,b) Case II: ${z}^{*}>\overline{z }$. Note: Time series of wage inequality ($z$), high-skilled employment rate ($e$), and output–capital ratio (${a}_{K}$) in the baseline scenario. Initial values: $z(0)=1.3$, $e(0)=0.7$, ${a}_{K}(0)=0.2$

Fig. 5a shows that the dynamical system is locally stable in the baseline scenario. Small changes in the parameter values do not alter the stability properties of the system.

In the baseline scenario (Fig. 1b), we set the parameters as follows:

$$\begin{array}{l}\begin{array}{ccc}\tau =0.076& a=1.05& \begin{array}{ccc} b=0.71& \rho =0.8& s=0.2\end{array}\end{array}\\ \begin{array}{ccc}\alpha =-0.27& \beta =0.04& \begin{array}{ccc}\gamma =0.46& \lambda =0.05& n=0.005\end{array}\end{array}\end{array}$$

(59)

Figures 2b, 3b, 4b display the long-run equilibrium values corresponding to a 1-percentage-point increase in $\alpha$ (i.e. $\alpha =-0.26$), a 1-percentage-point increase in $\beta$ (i.e. $\beta =0.05$), and a 0.5-percentage-points increase in $\gamma$ (i.e. $\gamma =0.465$), respectively.

Figure 5b shows that the dynamical system is locally stable in the baseline scenario. Small changes in the parameter values do not alter the stability properties of the system.

Appendix 5 - Local stability analysis

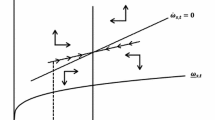
Let us define ${\theta }_{z}\equiv \left(1-\rho z\right){f}_{z}^{{L}{\prime}}$, ${\theta }_{\mu }\equiv \left(1+\rho {z}^{2}\right){f}_{\mu }^{{L}{\prime}}<0$, ${\Gamma }_{z}\equiv \left(1+\rho \right){f}_{z}^{{L}{\prime}}<0$. Remind that ${\Gamma }_{\mu }\equiv \left(1-\rho z\right){f}_{\mu }^{{L}{\prime}}$. ${\theta }_{z}>0$ and ${\Gamma }_{\mu }>0$ if, and only if, $z>\overline{z }$.

We investigate the local stability of the equilibrium linearizing the system of differential Eqs. (22), (23), and (24) around the equilibrium values (${a}_{K}^{*}$, ${z}^{*}$, ${e}^{*}$):

$$\left[\begin{array}{c}{\dot{a}}_{K}\\ \dot{z}\\ \dot{e}\end{array}\right]=\left[\begin{array}{ccc}0& {J}_{12}& {J}_{13}\\ 0& {J}_{22}& {J}_{23}\\ {J}_{31}& {J}_{32}& {J}_{33}\end{array}\right]\left[\begin{array}{c}{a}_{K}-{a}_{K}^{*}\\ z-{z}^{*}\\ e-{e}^{*}\end{array}\right]$$

(60)

where the elements of the Jacobian matrix ${\varvec{J}}$ evaluated at the steady-state values ${a}_{K}^{*}(\alpha ,\beta ,\gamma ,\tau ,s,n)$, ${z}^{*}(\alpha ,\beta ,\gamma ,\tau ,s,n)$, and ${e}^{*}(\alpha ,\beta ,\gamma ,\tau ,s,n)$ are given by:

$${J}_{12}\equiv {\left.\frac{\partial {\dot{a}}_{K}}{\partial z}\right|}_{{a}_{K}={a}_{K}^{*}, z={z}^{*},e={e}^{*}}={\theta }_{z}^{*}{\phi }_{{\widehat{a}}_{L}}^{\mathrm{^{\prime}}}{a}_{K}^{*}$$

(61)

$${J}_{13}\equiv {\left.\frac{\partial {\dot{a}}_{K}}{\partial e}\right|}_{{a}_{K}={a}_{K}^{*}, z={z}^{*},e={e}^{*}}={\theta }_{\mu }^{*}{\phi }_{{\widehat{a}}_{L}}^{\mathrm{^{\prime}}}{\mu }_{e}^{\mathrm{^{\prime}}}{a}_{K}^{*}<0$$

(62)

$${J}_{22}\equiv {\left.\frac{\partial \dot{z}}{\partial z}\right|}_{{a}_{K}={a}_{K}^{*}, z={z}^{*},e={e}^{*}}={\Gamma }_{z}^{*}{z}^{*}<0$$

(63)

$${J}_{23}\equiv {\left.\frac{\partial \dot{z}}{\partial e}\right|}_{{a}_{K}={a}_{K}^{*}, z={z}^{*},e={e}^{*}}=({h}_{e}^{\mathrm{^{\prime}}}+{\Gamma }_{\mu }^{*}{\mu }_{e}^{\mathrm{^{\prime}}}){z}^{*}$$

(64)

$${J}_{31}\equiv {\left.\frac{\partial \dot{e}}{\partial {a}_{K}}\right|}_{{a}_{K}={a}_{K}^{*}, z={z}^{*},e={e}^{*}}={g}_{{a}_{K}}^{\mathrm{^{\prime}}}{e}^{*}>0$$

(65)

$${J}_{32}\equiv {\left.\frac{\partial \dot{e}}{\partial z}\right|}_{{a}_{K}={a}_{K}^{*}, z={z}^{*},e={e}^{*}}=({\theta }_{z}^{*}{\phi }_{{\widehat{a}}_{L}}^{\mathrm{^{\prime}}}+{g}_{z}^{\mathrm{^{\prime}}}-{f}_{z}^{H\mathrm{^{\prime}}}){e}^{*}$$

(66)

$${J}_{33}\equiv {\left.\frac{\partial \dot{e}}{\partial e}\right|}_{{a}_{K}={a}_{K}^{*}, z={z}^{*},e={e}^{*}}=\left({\theta }_{\mu }^{*}{\phi }_{{\widehat{a}}_{L}}^{\mathrm{^{\prime}}}+{g}_{\mu }^{\mathrm{^{\prime}}}-{f}_{\mu }^{{H}^{\mathrm{^{\prime}}}}\right){\mu }_{e}^{\mathrm{^{\prime}}}{e}^{*}<0$$

(67)

Only partial derivatives (62), (63), (65), and (67) are unambiguously signed, whereas the signs of (61), (64) and (66) are crucially dependent on the level of wage inequality, on the effect of the high-skilled employment rate on the growth rate of the high-skilled nominal wage and the rates of high-skilled- and low-skilled-labour-saving innovations, and on the effect of wage inequality on capital accumulation and the rate of high-skilled-labour-saving techniques.

Equation (61) shows that an increase in wage inequality has a stabilizing effect on the dynamics of the output–capital ratio if and only if $z>{z}^{*}$. Indeed, an increase in $z$ has two opposite effects on the rate of change of the output–capital ratio: on the one hand, it stimulates the development of high-skilled-labour-saving techniques, thus exerting downward pressure on the output–capital ratio; on the other hand, it reduces the adoption of low-skilled-labour-saving innovations, thus putting upward pressure on the output–capital ratio. Since the first effect is non-linear and (in absolute value) increasing in $z$ (Eq. 28), the first effect will offset the second one if wage inequality exceeds the critical value $\overline{z }$.

Equation (64) shows that the effect of the high-skilled employment rate on the dynamics of wage inequality is mediated by its impact on the growth rate of the high-skilled workers’ nominal wage and on the rates of adoption of high-skilled- and low-skilled-labour-saving innovations. An increase in $e$ raises the growth rate of the high-skilled workers’ nominal wage and, by reducing the profit share, stimulates both high-skilled- and low-skilled-labour-saving innovations. As the response of high-skilled labour productivity growth to profitability is non-linear and (in absolute value) increasing in $z$ (Eq. 32), the overall effect of an increase in $e$ is crucially dependent on the level of wage inequality: if $z<{z}^{*}$, an increase in $e$ always has a destabilizing effect on the dynamics of wage inequality; if $z>{z}^{*}$, an increase in $e$ has a stabilizing effect if and only if the stimulus to the development of high-skilled-labour-saving innovations offset the impact on the growth rates of high-skilled nominal wage and low-skilled labour productivity.

From Eqs. (62) and (63), we have that the effect of the high-skilled employment rate on the dynamics of the output–capital ratio and the effect of wage inequality on its rate of change act as stabilization factors of the equilibrium. An increase in $e$ lowers the capital share in total costs, putting downward pressure on the output–capital ratio. A rise in $z$ has negative feedback on itself, as it induces the development of high-skilled-labour-saving innovations at the expense of the low-skilled-labour-saving innovation, thus reducing wage inequality.

Equation (66) shows that an increase in wage inequality has a stabilizing effect on the dynamics of the high-skilled employment rate if $z>{z}^{*}$ and high-skilled labour productivity growth is more responsive than capital accumulation to wage inequality.

The characteristic equation of the Jacobian matrix ${\varvec{J}}$ in (60) is given by:

$${\lambda }^{3}+{a}_{1}{\lambda }^{2}+{a}_{2}\lambda +{a}_{3}=0$$

(68)

where $\lambda$ denotes a characteristic root. The coefficients of Eq. (68) are:

$${a}_{1}=-{\text{Tr}}\left({\varvec{J}}\right)=-\left({J}_{22}+{J}_{33}\right)=-\left[{\Gamma }_{z}^{*}{z}^{*}+\left({\theta }_{\mu }^{*}{\phi }_{{\widehat{a}}_{L}}{\prime}+{g}_{\mu }{\prime}-{f}_{\mu }^{{H}{\prime}}\right){\mu }_{e}{\prime}{e}^{*}\right]$$

(69)

$$\begin{array}{l}{a}_{2}=\left|\begin{array}{cc}{J}_{22}& {J}_{23}\\ {J}_{32}& {J}_{33}\end{array}\right|+\left|\begin{array}{cc}0& {J}_{13}\\ {J}_{31}& {J}_{33}\end{array}\right|={\Gamma }_{z}^{*}\left({\theta }_{\mu }^{*}{\phi }_{{\widehat{a}}_{L}}^{\mathrm{^{\prime}}}+{g}_{\mu }^{\mathrm{^{\prime}}}-{f}_{\mu }^{{H}^{\mathrm{^{\prime}}}}\right){\mu }_{e}^{\mathrm{^{\prime}}}{e}^{*}{z}^{*}-{\theta }_{\mu }^{*}{\phi }_{{\widehat{a}}_{L}}^{\mathrm{^{\prime}}}{g}_{{a}_{K}}^{\mathrm{^{\prime}}}{\mu }_{e}^{\mathrm{^{\prime}}}{e}^{*}{a}_{K}^{*}+\\ - {\theta }_{z}^{*}{\phi }_{{\widehat{a}}_{L}}^{\mathrm{^{\prime}}}\left({h}_{e}^{\mathrm{^{\prime}}}+{\Gamma }_{\mu }^{*}{\mu }_{e}^{\mathrm{^{\prime}}}\right){e}^{*}{z}^{*}-({g}_{z}^{\mathrm{^{\prime}}}-{f}_{z}^{{H}^{\mathrm{^{\prime}}}})({h}_{e}^{\mathrm{^{\prime}}}+{\Gamma }_{\mu }^{*}{\mu }_{e}^{\mathrm{^{\prime}}}){e}^{*}{z}^{*}\end{array}$$

(70)

$${a}_{3}=-{\text{Det}}\left({\varvec{J}}\right)={J}_{31}\left({J}_{13}{J}_{22}-{J}_{12}{J}_{23}\right)=-{g}_{{a}_{K}}{\prime}{\phi }_{{\widehat{a}}_{L}}{\prime}\left[{\theta }_{z}^{*}\left({h}_{e}{\prime}+{\Gamma }_{\mu }^{*}{\mu }_{e}{\prime}\right)-{\theta }_{\mu }^{*}{\Gamma }_{z}^{*}{\mu }_{e}{\prime}\right]{e}^{*}{z}^{*}{a}_{K}^{*}$$

(71)

The necessary and sufficient condition for the local stability of the dynamic system is that all characteristic roots are negative or have a negative real part,¹³ which occurs when:

$${a}_{1}>0,\;\;\; {a}_{2}>0,\;\;\; {a}_{3}>0,\;\;\; {a}_{1}{a}_{2}-{a}_{3}>0$$

(72)

Proposition 5

The equilibrium is locally stable if $\left({g}_{z}{\prime}-{f}_{z}^{{H}{\prime}}\right)\left({h}_{e}{\prime}+{\Gamma }_{\mu }^{*}{\mu }_{e}{\prime}\right)<0$ and ${\theta }_{z}^{*}\left({h}_{e}{\prime}+{\Gamma }_{\mu }^{*}{\mu }_{e}{\prime}\right)<0$, and only if ${\theta }_{z}^{*}\left({h}_{e}{\prime}+{\Gamma }_{\mu }^{*}{\mu }_{e}{\prime}\right)>{\theta }_{\mu }^{*}{\Gamma }_{z}^{*}{\mu }_{e}{\prime}$. Then, if ${z}^{*}<\overline{z }$, local stability requires ${\theta }_{z}^{*}\left({h}_{e}{\prime}+{\Gamma }_{\mu }^{*}{\mu }_{e}{\prime}\right)>{\theta }_{\mu }^{*}{\Gamma }_{z}^{*}{\mu }_{e}{\prime}$, whereas ${g}_{z}{\prime}<{f}_{z}^{{H}{\prime}}$ is sufficient for the equilibrium to be locally stable; if ${z}^{*}>\overline{z }$, a sufficient condition for the local stability is ${g}_{z}{\prime}>{f}_{z}^{{H}{\prime}}$ and ${h}_{e}{\prime}+{\Gamma }_{\mu }^{*}{\mu }_{e}{\prime}<0$.

Proof

The condition ${a}_{1}>0$ is always satisfied. The condition ${a}_{3}>0$ is satisfied if and only if ${\theta }_{z}^{*}\left({h}_{e}{\prime}+{\Gamma }_{\mu }^{*}{\mu }_{e}{\prime}\right)>{\theta }_{\mu }^{*}{\Gamma }_{z}^{*}{\mu }_{e}{\prime}$. After rearranging:

$$\begin{array}{l}{a}_{2}=\underset{\gtrless 0}{\underbrace{- {\phi }_{{\widehat{a}}_{L}}^{\mathrm{^{^\prime}}}\left[{\theta }_{z}^{*}\left({h}_{e}^{\mathrm{^{^\prime}}}+{\Gamma }_{\mu }^{*}{\mu }_{e}^{\mathrm{^{\prime}}}\right)-{\theta }_{\mu }^{*}{\Gamma }_{z}^{*}{\mu }_{e}^{\mathrm{^{^\prime}}}\right]{e}^{*}{z}^{*}}}\;\;\underset{>0}{\underbrace{{{{+ {\Gamma }_{z}^{*}\left({g}_{\mu }^{\mathrm{^{^\prime}}}-{f}_{\mu }^{{H}^{\mathrm{^{^\prime}}}}\right){\mu }_{e}^{\mathrm{^{^\prime}}}{e}^{*}{z}^{*}}}} }}\;\;\;\underset{>0}{\underbrace{- {\theta }_{\mu }^{*}{\phi }_{{\widehat{a}}_{L}}^{\mathrm{^{^\prime}}}{g}_{{a}_{K}}^{\mathrm{^{^\prime}}}{\mu }_{e}^{\mathrm{^{^\prime}}}{e}^{*}{a}_{K}^{*}}}+\\\underset{{\gtrless 0}}{\underbrace{- ({g}_{z}^{\mathrm{^{\prime}}}-{f}_{z}^{{H}^{\mathrm{^{^\prime}}}})({h}_{e}^{\mathrm{^{^\prime}}}+{\Gamma }_{\mu }^{*}{\mu }_{e}^{\mathrm{^{^\prime}}}){e}^{*}{z}^{*}}}\end{array}$$

(73)

If ${a}_{3}>0$, $\left({g}_{z}{\prime}-{f}_{z}^{{H}{\prime}}\right)\left({h}_{e}{\prime}+{\Gamma }_{\mu }^{*}{\mu }_{e}{\prime}\right)<0$ is a sufficient condition for ${a}_{2}>0$. After some algebra, we have:

$$\begin{array}{l}{a}_{1}{a}_{2}-{a}_{3}=\underset{>0}{\underbrace{ {{{\phi}_{\widehat{a}_{L}}^{^{\prime}}}{\left[{\Gamma }_{z}^{\ast}{z}^{\ast}+{\left({\theta }_{\mu }^{\ast}{\phi}_{\widehat{a}_{L}}^{^{\prime}}+{g}_{\mu }^{^{\prime}}-{f}_{\mu }^{{H}^{^{\prime}}}\right)}{\mu }_{e}^{^{\prime}}{e}^{\ast}\right]}}}}\;\;\underset{\gtrless 0}{\underbrace{{{\left[{\theta }_{z }^{\ast}{\left({h}_{e}^{^{\prime}}+{\Gamma }_{\mu}^{\ast}{\mu}_{e}^{^{\prime}}\right)-{\theta }_{\mu }^{\ast}{\Gamma }_{z}^{\ast}{\mu}_{e}^{^{\prime}}}\right]}{e}^{\ast}{z}^{\ast}+}}}\\{\underset{< 0}{\underbrace{-{\Gamma }_{z}^{\ast} {\left[{\Gamma }_{z}^{\ast}{z}^{\ast}+{\left({\theta }_{\mu }^{\ast}{\phi}_{\widehat{a}_{L}}^{^{\prime}}+{g}_{\mu }^{^{\prime}}-{f}_{\mu }^{{H}^{^{\prime}}}\right)}{\mu }_{e}^{^{\prime}}{e}^{\ast}\right]}}}}\;\;{\underset{< 0}{\underbrace{{\left({g}_{\mu }^{^{\prime}}-{f}_{\mu }^{{H}^{^{\prime}}}\right)}{\mu }_{e}^{^{\prime}}{e}^{\ast}{z}^{\ast}}}}\;\; {+}\\{\underset{> 0}{\underbrace{+{\theta }_{\mu }^{\ast}{\phi}_{\widehat{a}_{L}}^{^{\prime}}{g}_{a_K}^{{\prime}} {\left({\theta }_{\mu }^{\ast}{\phi}_{\widehat{a}_{L}}^{^{\prime}}+{g}_{\mu }^{^{\prime}}-{f}_{\mu }^{{H}^{^{\prime}}}\right)}{\mu }_{e}^{{\prime}{2}}{e}^{{\ast}{2}}{a}_{K}^{\ast}}+}}\\{\underset{\gtrless 0}{\underbrace{{g}_{a_K}^{{\prime}}{\theta }_{z }^{\ast}{\phi}_{\widehat{a}_{L}}^{^{\prime}} {\left({{{h}_{e}^{{\prime}}+{\Gamma }_{\mu}^{\ast}{\mu}_{e}^{{\prime}}}}\right)}{e}^{\ast}{z}^{\ast} {a}_{K}^{\ast}}}}\; {+}\\\\{\underset{< 0}{\underbrace{+{\left[{\Gamma }_{z}^{\ast}{z}^{\ast}+{\left({\theta }_{\mu }^{\ast}{\phi}_{\widehat{a}_{L}}^{^{\prime}}+{g}_{\mu }^{^{\prime}}-{f}_{\mu }^{{H}^{^{\prime}}}\right)}{\mu }_{e}^{^{\prime}}{e}^{\ast}\right]}}}}\;\;{\underset{\gtrless 0}{\underbrace{\left({g}_{z}^{^{\prime}}-{f}_{z }^{{H}^{^{\prime}}}\right)\;\left({h}_{e}^{^{\prime}}+{\Gamma }_{\mu }^{\ast}{\mu }_{e}^{^{\prime}}\right)}}}{e}^{\ast}{z}^{\ast}\end{array}$$

(74)

If ${a}_{2}>0$ and ${a}_{3}>0$, ${\theta }_{z}^{*}\left({h}_{e}{\prime}+{\Gamma }_{\mu }^{*}{\mu }_{e}{\prime}\right)<0$ is a sufficient condition for ${a}_{1}{a}_{2}-{a}_{3}>0$. We have thus proved the first part of Proposition 1.

If ${z}^{*}<\overline{z }$, then ${\theta }_{z}^{*}<0$ and ${h}_{e}{\prime}+{\Gamma }_{\mu }^{*}{\mu }_{e}{\prime}>0$. Therefore, ${g}_{z}{\prime}<{f}_{z}^{{H}{\prime}}$ is sufficient for the equilibrium to be locally stable. If ${z}^{*}>\overline{z }$, we always have ${\theta }_{z}^{*}\left({h}_{e}{\prime}+{\Gamma }_{\mu }^{*}{\mu }_{e}{\prime}\right)>{\theta }_{\mu }^{*}{\Gamma }_{z}^{*}{\mu }_{e}{\prime}$, since ${\theta }_{z}^{*}>0$ and ${\theta }_{z}^{*}{\Gamma }_{\mu }^{*}<{\theta }_{\mu }^{*}{\Gamma }_{z}^{*}$. Therefore, ${g}_{z}{\prime}>{f}_{z}^{{H}{\prime}}$ and ${h}_{e}{\prime}+{\Gamma }_{\mu }^{*}{\mu }_{e}{\prime}<0$ are a sufficient condition for the local stability. We have thus proved the second part of Proposition 1.

The necessary condition ${\theta }_{z}^{*}\left({h}_{e}{\prime}+{\Gamma }_{\mu }^{*}{\mu }_{e}{\prime}\right)>{\theta }_{\mu }^{*}{\Gamma }_{z}^{*}{\mu }_{e}{\prime}$, or equivalently ${\theta }_{z}^{*}{\phi }_{{\widehat{a}}_{L}}{\prime}\left({h}_{e}{\prime}+{\Gamma }_{\mu }^{*}{\mu }_{e}{\prime}\right)<{\theta }_{\mu }^{*}{\Gamma }_{z}^{*}{\phi }_{{\widehat{a}}_{L}}{\prime}{\mu }_{e}{\prime}$, prevents wage inequality $z$ and employment $e$ from causing an explosive growth of output–capital ratio and wage inequality. Indeed, the effect of employment on the growth of the output–capital ratio (Eq. 62) and the effect of wage inequality on its growth rate (Eq. 63) act as stabilizing forces of the equilibrium, whereas the effect of wage inequality on the growth of the output–capital ratio (Eq. 61) and the effect of employment on the growth of wage inequality (Eq. 64) are not unambiguously signed. The equilibrium will be locally stable only if the effect of the stabilizing forces offset the impact of the ambiguously signed effects – a condition which is always satisfied in the presence of a high level of wage inequality (i.e. if ${z}^{*}>\overline{z }$).

The sufficient condition $\left({g}_{z}{\prime}-{f}_{z}^{{H}{\prime}}\right)\left({h}_{e}{\prime}+{\Gamma }_{\mu }^{*}{\mu }_{e}{\prime}\right)<0$ and ${\theta }_{z}^{*}\left({h}_{e}{\prime}+{\Gamma }_{\mu }^{*}{\mu }_{e}{\prime}\right)<0$ implies that a system is locally stable in the presence of an equilibrium in the balance of power among social classes, in terms of dynamics of high-skilled employment and wage inequality (Eqs. (63), (64), and (66)). Indeed, the system is stable if an imbalance in favour of the high-skilled workers in the dynamics of wage inequality (i.e. ${h}_{e}{\prime}+{\Gamma }_{\mu }^{*}{\mu }_{e}{\prime}>0$) is counteracted by a negative effect of wage inequality on the growth rate of employment (i.e. ${g}_{z}{\prime}-{f}_{z}^{{H}{\prime}}<0$) and a low level of wage inequality (${z}^{*}<\overline{z }$), or alternatively, if an imbalance in favour of the high-skilled workers in the dynamics of employment (i.e. ${g}_{z}{\prime}-{f}_{z}^{{H}{\prime}}>0$) and the level of wage inequality (${z}^{*}>\overline{z }$) is compensated by a negative response of the growth rate of wage inequality to employment (${h}_{e}{\prime}+{\Gamma }_{\mu }^{*}{\mu }_{e}{\prime}<0$).

Appendix 6

Totally differentiating Eqs. (25), (26), and (27) with respect to $\alpha$ yields:

$$\frac{d{z}^{*}}{d\alpha }=\frac{{h}_{\alpha }{\prime}(1+\rho {z}^{2}){f}_{\mu }^{{L}{\prime}}{\mu }_{e}{\prime}}{\sigma }>0$$

(75)

$$\frac{d{e}^{*}}{d\alpha }=-\frac{{h}_{\alpha }^{\mathrm{^{\prime}}}(1-\rho z){f}_{z}^{L\mathrm{^{\prime}}}}{\sigma }$$

(76)

Using Eqs. (75), (76), and $g={\widehat{a}}_{H}-n$, total differentiation of Eqs. (5) and (6), with (12), and (10), (12), (17), and (18) with respect to $\alpha$ yields:

$$\frac{d{\mu }^{*}}{d\alpha }=-\frac{{h}_{\alpha }{\prime}(1-\rho z){f}_{z}^{L{\prime}}{\mu }_{e}{\prime}}{\sigma }$$

(77)

$$\frac{d{g}^{*}}{d\alpha }=\frac{d{\widehat{a}}_{H}^{*}}{d\alpha }=-\frac{{h}_{\alpha }^{\mathrm{^{\prime}}}\rho \left(1+z\right){f}_{z}^{{L}^{\mathrm{^{\prime}}}}{f}_{\mu }^{{L}^{\mathrm{^{\prime}}}}{\mu }_{e}^{\mathrm{^{\prime}}}}{\sigma }>0$$

(78)

$$\frac{d{\widehat{a}}_{L}^{*}}{d\alpha }=\frac{{h}_{\alpha }{\prime}\rho z\left(1+z\right){f}_{z}^{{L}{\prime}}{f}_{\mu }^{{L}{\prime}}{\mu }_{e}{\prime}}{\sigma }<0$$

(79)

$$\frac{d{\widehat{w}}_{H}^{*}}{d\alpha }=-\frac{{h}_{\alpha }^{\mathrm{^{\prime}}}\rho {\left(1+z\right)}^{2}{f}_{z}^{{L}^{\mathrm{^{\prime}}}}{f}_{\mu }^{{L}^{\mathrm{^{\prime}}}}{\mu }_{e}^{\mathrm{^{\prime}}}}{\sigma }>0$$

(80)

$$\frac{d{\omega }_{H}^{*}}{d\alpha }=\frac{{h}_{\alpha }{\prime}\left[1+\rho {z}^{2}+\mu (1+z)\right]{\mu }_{e}{\prime}}{{\mu }^{2}{\left(1+\mu \right)}^{2}{\left(1+z\right)}^{3}{\phi }_{{\widehat{a}}_{L}{\widehat{a}}_{L}}^{{\prime}{\prime}}\sigma }>0$$

(81)

$$\frac{d{\omega }_{L}^{*}}{d\alpha }=-\frac{{h}_{\alpha }^{\mathrm{^{\prime}}}\left[1+\rho {z}^{2}+\rho \mu z\left(1+z\right)\right]{\mu }_{e}^{\mathrm{^{\prime}}}}{{\mu }^{2}{\left(1+\mu \right)}^{2}{\left(1+z\right)}^{3}{\phi }_{{\widehat{a}}_{L}{\widehat{a}}_{L}}^{\mathrm{^{\prime}}\mathrm{^{\prime}}}\sigma }<0$$

(82)

Appendix 7

Totally differentiating Eqs. (25), (26), and (27) with respect to $\beta$ yields:

$$\frac{d{z}^{*}}{d\beta }=-\frac{(1+\rho {z}^{2}){f}_{\mu }^{{L}{\prime}}{\mu }_{e}{\prime}}{\sigma }>0$$

(83)

$$\frac{d{e}^{*}}{d\beta }=\frac{(1-\rho z){f}_{z}^{L\mathrm{^{\prime}}}}{\sigma }$$

(84)

Using Eqs. (75), (76), and $g={\widehat{a}}_{H}-n$, total differentiation of Eqs. (5) and (6), with (12), and (10), (12), (17), and (18) with respect to $\beta$ yields:

$$\frac{d{\mu }^{*}}{d\beta }=\frac{(1-\rho z){f}_{z}^{L{\prime}}{\mu }_{e}{\prime}}{\sigma }$$

(85)

$$\frac{d{g}^{*}}{d\beta }=\frac{d{\widehat{a}}_{H}^{*}}{d\beta }=\frac{\rho \left(1+z\right){f}_{z}^{{L}^{\mathrm{^{\prime}}}}{f}_{\mu }^{{L}^{\mathrm{^{\prime}}}}{\mu }_{e}^{\mathrm{^{\prime}}}}{\sigma }<0$$

(86)

$$\frac{d{\widehat{a}}_{L}^{*}}{d\beta }=-\frac{\rho z\left(1+z\right){f}_{z}^{{L}{\prime}}{f}_{\mu }^{{L}{\prime}}{\mu }_{e}{\prime}}{\sigma }>0$$

(87)

$$\frac{d{\widehat{w}}_{H}^{*}}{d\beta }=\frac{\rho {\left(1+z\right)}^{2}{f}_{z}^{{L}^{\mathrm{^{\prime}}}}{f}_{\mu }^{{L}^{\mathrm{^{\prime}}}}{\mu }_{e}^{\mathrm{^{\prime}}}}{\sigma }<0$$

(88)

$$\frac{d{\omega }_{H}^{*}}{d\beta }=-\frac{\left[1+\rho {z}^{2}+\mu \left(1+z\right)\right]{\mu }_{e}{\prime}}{{\mu }^{2}{\left(1+\mu \right)}^{2}{\left(1+z\right)}^{3}{\phi }_{{\widehat{a}}_{L}{\widehat{a}}_{L}}^{{\prime}{\prime}}\sigma }<0$$

(89)

$$\frac{d{\omega }_{L}^{*}}{d\beta }=\frac{\left[1+\rho {z}^{2}+\rho \mu z\left(1+z\right)\right]{\mu }_{e}^{\mathrm{^{\prime}}}}{{\mu }^{2}{\left(1+\mu \right)}^{2}{\left(1+z\right)}^{3}{\phi }_{{\widehat{a}}_{L}{\widehat{a}}_{L}}^{\mathrm{^{\prime}}\mathrm{^{\prime}}}\sigma }>0$$

(90)

Appendix 8

Totally differentiating Eqs. (25), (26), and (27) with respect to $\gamma$ yields:

$$\frac{d{z}^{*}}{d\gamma }=-\frac{{h}_{e}{\prime}(1+\rho {z}^{2}){f}_{\mu }^{{L}{\prime}}{\mu }_{\gamma }{\prime}}{\sigma }>0$$

(91)

$$\frac{d{e}^{*}}{d\gamma }=\frac{\rho {\left(1+z\right)}^{2}{f}_{z}^{L\mathrm{^{\prime}}}{f}_{\mu }^{{L}^{\mathrm{^{\prime}}}}{\mu }_{\gamma }^{\mathrm{^{\prime}}}}{\sigma }>0$$

(92)

Using Eqs. (91), (92), and $g={\widehat{a}}_{H}-n$, total differentiation of Eqs. (5) and (6), with (12), and (10), (12), (17), and (18) with respect to $\gamma$ yields:

$$\frac{d{\mu }^{*}}{d\gamma }=\frac{{h}_{e}{\prime}(1-\rho z){f}_{z}^{L{\prime}}{\mu }_{\gamma }{\prime}}{\sigma }$$

(93)

$$\frac{d{g}^{*}}{d\gamma }=\frac{d{\widehat{a}}_{H}^{*}}{d\gamma }=\frac{{h}_{e}^{\mathrm{^{\prime}}}\rho \left(1+z\right){f}_{z}^{{L}^{\mathrm{^{\prime}}}}{f}_{\mu }^{{L}^{\mathrm{^{\prime}}}}{\mu }_{\gamma }^{\mathrm{^{\prime}}}}{\sigma }>0$$

(94)

$$\frac{d{\widehat{a}}_{L}^{*}}{d\gamma }=-\frac{{h}_{e}{\prime}\rho z\left(1+z\right){f}_{z}^{{L}{\prime}}{f}_{\mu }^{{L}{\prime}}{\mu }_{\gamma }{\prime}}{\sigma }<0$$

(95)

$$\frac{d{\widehat{w}}_{H}^{*}}{d\gamma }=\frac{{h}_{e}^{\mathrm{^{\prime}}}\rho {\left(1+z\right)}^{2}{f}_{z}^{L\mathrm{^{\prime}}}{f}_{\mu }^{{L}^{\mathrm{^{\prime}}}}{\mu }_{\gamma }^{\mathrm{^{\prime}}}}{\sigma }>0$$

(96)

$$\frac{d{\omega }_{H}^{*}}{d\gamma }=-\frac{{h}_{e}{\prime}\left[1+\rho {z}^{2}+\mu (1+z)\right]{\mu }_{\gamma }{\prime}}{{\mu }^{2}{\left(1+\mu \right)}^{2}{\left(1+z\right)}^{3}{\phi }_{{\widehat{a}}_{L}{\widehat{a}}_{L}}^{{\prime}{\prime}}\sigma }>0$$

(97)

$$\frac{d{\omega }_{L}^{*}}{d\gamma }=\frac{{h}_{e}^{\mathrm{^{\prime}}}\left[1+\rho {z}^{2}+\rho \mu z\left(1+z\right)\right]{\mu }_{\gamma }^{\mathrm{^{\prime}}}}{{\mu }^{2}{\left(1+\mu \right)}^{2}{\left(1+z\right)}^{3}{\phi }_{{\widehat{a}}_{L}{\widehat{a}}_{L}}^{\mathrm{^{\prime}}\mathrm{^{\prime}}}\sigma }<0$$

(98)