Integral formulas for computing a third-order gravitational tensor from volumetric mass density, disturbing gravitational potential, gravity anomaly and gravity disturbance

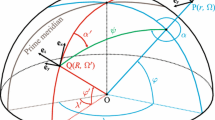
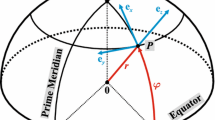
Abstract

A new mathematical model for evaluation of the third-order (disturbing) gravitational tensor is formulated in this article. Firstly, we construct corresponding differential operators for the components of the third-order (disturbing) gravitational tensor in a spherical local north-oriented frame. We show that the differential operators may efficiently be decomposed into an azimuthal and an isotropic part. The differential operators are even more simplified for a certain class of isotropic kernels. Secondly, the differential operators are applied to the well-known integrals of Newton, Abel-Poisson, Pizzetti and Hotine. In this way, 40 new integral formulas are derived. The new integral formulas allow for evaluation of the components of the third-order (disturbing) gravitational tensor from density distribution, disturbing gravitational potential, gravity anomalies and gravity disturbances. Thirdly, we investigate the behaviour of the corresponding integral kernels in the spatial domain. The new mathematical formulas extend the theoretical apparatus of geodesy, i.e. the well-known Meissl scheme, and reveal important properties of the third-order gravitational tensor. They may be exploited in geophysical studies, continuation of gravitational field quantities and analysing the gradiometric-geodynamic boundary value problem.

Appendices

Appendix A: Differential operators for the second-order gravitational gradients

In Sect. 3, we construct the differential operators for the third-order (disturbing) gravitational gradients. For this purpose, we exploit also the differential operators for the second-order gravitational gradients. The six second-order differential operators in terms of angular spherical geocentric coordinates $(r, \Omega )$ read as follows (Reed 1973; Koop 1993):

$$\begin{aligned} {\mathcal {D}}^{xx}&= \frac{1}{r} \left( \frac{\partial }{\partial r} + \frac{1}{r} \frac{\partial ^2}{\partial \varphi ^2} \right) ,\end{aligned}$$

(71)

$$\begin{aligned} {\mathcal {D}}^{xy}&= - \frac{1}{r^2 \cos \varphi } \left( \tan \varphi \frac{\partial }{\partial \lambda } + \frac{\partial ^2}{\partial \varphi \partial \lambda } \right) ,\end{aligned}$$

(72)

$$\begin{aligned} {\mathcal {D}}^{xz}&= - \frac{1}{r} \left( \frac{1}{r} \frac{\partial }{\partial \varphi } - \frac{\partial ^2}{\partial r \partial \varphi } \right) ,\end{aligned}$$

(73)

$$\begin{aligned} {\mathcal {D}}^{yy}&= \frac{1}{r} \left( \frac{\partial }{\partial r} - \frac{\tan \varphi }{r} \frac{\partial }{\partial \varphi } + \frac{1}{r \cos ^2 \varphi } \frac{\partial ^2}{\partial \lambda ^2} \right) ,\end{aligned}$$

(74)

$$\begin{aligned} {\mathcal {D}}^{yz}&= \frac{1}{r \cos \varphi } \left( \frac{1}{r} \frac{\partial }{\partial \lambda } - \frac{\partial ^2}{\partial r \partial \lambda } \right) ,\end{aligned}$$

(75)

$$\begin{aligned} {\mathcal {D}}^{zz}&= \frac{\partial ^2}{\partial r^2}. \end{aligned}$$

(76)

Alternatively, the second-order differential operators of Eqs. (71)–(76) may be expressed in terms of the spherical polar coordinates $(r, \psi , \alpha )$, see, e.g. (Wolf 2007; Šprlák et al. 2014):

$$\begin{aligned}&{\mathcal {D}}^{xx}={\mathcal {D}}_{2}^{1} + \cos 2 \alpha {\mathcal {D}}_{2}^{2}, \quad {\mathcal {D}}^{xy} = - \sin 2 \alpha {\mathcal {D}}_{2}^{2},\nonumber \\&{\mathcal {D}}^{xz}=\cos \alpha {\mathcal {D}}_{2}^{3}, \quad {\mathcal {D}}^{yy} = {\mathcal {D}}_{2}^{1} - \cos 2 \alpha {\mathcal {D}}_{2}^{2},\nonumber \\&{\mathcal {D}}^{yz}=- \sin \alpha {\mathcal {D}}_{2}^{3}, \quad {\mathcal {D}}^{zz} = {\mathcal {D}}_{2}^{4}, \end{aligned}$$

(77)

where

$$\begin{aligned} {\mathcal {D}}_{2}^{1}&= \frac{1}{r} \left[ \frac{\partial }{\partial r} + \frac{1}{2 r} \left( \frac{\partial ^2}{\partial \psi ^2} + \cot \psi \frac{\partial }{\partial \psi } \right) \right] , \end{aligned}$$

(78)

$$\begin{aligned} {\mathcal {D}}_{2}^{2}&= \frac{1}{2 r^2} \left( \frac{\partial ^2}{\partial \psi ^2} - \cot \psi \frac{\partial }{\partial \psi } \right) ,\end{aligned}$$

(79)

$$\begin{aligned} {\mathcal {D}}_{2}^{3}&= \frac{1}{r} \left( \frac{1}{r} \frac{\partial }{\partial \psi } - \frac{\partial ^2}{\partial r \, \partial \psi } \right) ,\end{aligned}$$

(80)

$$\begin{aligned} {\mathcal {D}}_{2}^{4}&= \frac{\partial ^2}{\partial r^2}. \end{aligned}$$

(81)

Note that the subscript in Eqs. (78)–(81) refers to the order of the gravitational gradients.

Appendix B: Explicit decomposition of degree-dependent terms

To arrive at the new isotropic kernels ${\mathcal {K}}^{i*, j}$; $i \in \{0, 1, 2, 3\}$; $j \in \{{\mathcal {N}}, {\mathcal {P}}, {\mathcal {S}}, {\mathcal {H}}\}$ in the closed form, see Sect. 4, an explicit decomposition of some degree-dependent terms is required. Such a decomposition is completely defined by the following equations:

$$\begin{aligned}&h_n^{{\mathcal {N}}}\ (n + 1) (n + 2) (n + 3) = 6 + 11 n + 6 n^2 + n^3,\end{aligned}$$

(82)

$$\begin{aligned}&h_n^{{\mathcal {N}}}\ (n + 2) (n + 3) = 6 + 5 n + n^2,\end{aligned}$$

(83)

$$\begin{aligned}&h_n^{{\mathcal {N}}}\ (n + 3) = 3 + n,\end{aligned}$$

(84)

$$\begin{aligned}&h_n^{{\mathcal {N}}} \!=\! 1,\end{aligned}$$

(85)

$$\begin{aligned}&h_n^{{\mathcal {P}}}\ (n \!+\! 1) (n \!+\! 2) (n \!+\! 3) \!=\! 6 \!+\! 23 n \!+\! 28 n^2 \!+\! 13 n^3 \!+\! 2 n^4,\end{aligned}$$

(86)

$$\begin{aligned}&h_n^{{\mathcal {P}}}\ (n + 2) (n + 3) = 6 + 17 n + 11 n^2 + 2 n^3,\end{aligned}$$

(87)

$$\begin{aligned}&h_n^{{\mathcal {P}}}\ (n + 3) = 3 + 7 n + 2 n^2,\end{aligned}$$

(88)

$$\begin{aligned}&h_n^{{\mathcal {P}}} = 1 + 2 n,\end{aligned}$$

(89)

$$\begin{aligned}&h_n^{{\mathcal {S}}}\ (n + 1) (n + 2) (n + 3) \nonumber \\&= \frac{72}{n - 1} + 66 + 43 n + 15 n^2 + 2 n^3,\end{aligned}$$

(90)

$$\begin{aligned}&h_n^{{\mathcal {S}}}\ (n + 2) (n + 3) = \frac{36}{n - 1} + 30 + 13 n + 2 n^2,\end{aligned}$$

(91)

$$\begin{aligned}&h_n^{{\mathcal {S}}}\ (n + 3) = \frac{12}{n - 1} + 9 + 2 n,\end{aligned}$$

(92)

$$\begin{aligned}&h_n^{{\mathcal {S}}} = \frac{3}{n - 1} + 2,\end{aligned}$$

(93)

$$\begin{aligned}&h_n^{{\mathcal {H}}}\ (n + 1) (n + 2) (n + 3) = 6 + 17 n + 11 n^2 + 2 n^3,\end{aligned}$$

(94)

$$\begin{aligned}&h_n^{{\mathcal {H}}}\ (n + 2) (n + 3) = - \frac{2}{n + 1} + 8 + 9 n + 2 n^2,\end{aligned}$$

(95)

$$\begin{aligned}&h_n^{{\mathcal {H}}}\ (n + 3) = - \frac{2}{n + 1} + 5 + 2 n,\end{aligned}$$

(96)

$$\begin{aligned}&h_n^{{\mathcal {H}}} = - \frac{1}{n + 1} + 2. \end{aligned}$$

(97)

Note that the symbols $h_n^{j}$; $j \in \{{\mathcal {N}}, {\mathcal {P}}, {\mathcal {S}}, {\mathcal {H}}\}$ stand for the eigenvalues of the Newton, Abel-Poisson, Pizzetti and Hotine kernels. These are defined by Eqs. (39)–(42).

Appendix C: Sums of infinite series

To derive the new isotropic kernels ${\mathcal {K}}^{i*, j}$; $i \in \{0, 1, 2, 3\}$; $j \in \{{\mathcal {N}}, {\mathcal {P}}, {\mathcal {S}}, {\mathcal {H}}\}$ in closed form, see Sect. 4, we also need closed form expressions for some infinite series. Complete set of these summation rules reads as follows:

$$\begin{aligned}&S_{1}(t,u) \!=\! \sum \limits _{n=0}^{\infty }t^{n+4}\frac{1}{n + 1} P_{n,0}(u)\!=\!t^3 \ln \left( \frac{g + t \!-\! u}{1 \!-\! u}\right) ,\end{aligned}$$

(98)

$$\begin{aligned}&S_{2}(t,u) = \sum \limits _{n=0}^{\infty }t^{n+4}P_{n,0}(u)= \frac{t^4}{g},\end{aligned}$$

(99)

$$\begin{aligned}&S_{3}(t,u) = \sum \limits _{n=0}^{\infty }t^{n+4}nP_{n,0}(u) =\frac{t^5 (u - t)}{g^3},\end{aligned}$$

(100)

$$\begin{aligned}&S_{4}(t,u) = \sum \limits _{n=0}^{\infty }t^{n+4}n^2P_{n,0}(u)\nonumber \\&\quad = \frac{t^5}{g^3} \left[ u - 2 t + \frac{3 t (t - u)^2}{g^2}\right] ,\end{aligned}$$

(101)

$$\begin{aligned}&S_{5}(t,u) =\sum \limits _{n=0}^{\infty }t^{n+4}n^3 P_{n,0}(u)=\frac{t^5}{g^3} \Bigg [u - 4 t \nonumber \\&\quad + \frac{9 t (t - u) (2 t - u)}{g^2} - \frac{15 t^2 (t - u)^3}{g^4}\Bigg ],\end{aligned}$$

(102)

$$\begin{aligned}&S_{6}(t,u) = \sum \limits _{n=0}^{\infty }t^{n+4}n^4P_{n,0}(u)\nonumber \\&\quad = \frac{t^5}{g^3} \left[ u - 8 t + \frac{12 t (t - u) (7 t - u) + 9 t u^2}{g^2} \right. \nonumber \\&\qquad \left. - \frac{90 t^2 (t - u)^2 (2 t - u)}{g^4} + \frac{105 t^3 (t - u)^4}{g^6}\right] ,\end{aligned}$$

(103)

$$\begin{aligned}&S_{7}(t,u) = \sum \limits _{n=1}^{\infty }t^{n+4}\frac{1}{n + 1} P_{n,1}(u) \nonumber \\&\quad = t^3 \sqrt{1 - u^2} \left[ \frac{1}{1 - u} - \frac{g + t}{g (g + t - u)}\right] ,\end{aligned}$$

(104)

$$\begin{aligned}&S_{8}(t,u) = \sum \limits _{n=1}^{\infty }t^{n+4}P_{n,1}(u)= \frac{t^5 \sqrt{1 - u^2}}{g^3},\end{aligned}$$

(105)

$$\begin{aligned}&S_{9}(t,u) = \sum \limits _{n=1}^{\infty }t^{n+4}nP_{n,1}(u)\nonumber \\&\quad =\frac{t^5 \sqrt{1 - u^2}}{g^3} \left[ 1 + \frac{3 t (u - t)}{g^2}\right] ,\end{aligned}$$

(106)

$$\begin{aligned}&S_{10}(t,u) = \sum \limits _{n=1}^{\infty }t^{n+4}n^2 P_{n,1}(u)\nonumber \\&= \frac{t^5 \sqrt{1 - u^2}}{g^3} \left[ 1 + \frac{3 t (3 u - 4 t)}{g^2} + \frac{15 t^2 (t - u)^2}{g^4}\right] ,\end{aligned}$$

(107)

$$\begin{aligned}&S_{11}(t,u) = \sum \limits _{n=1}^{\infty }t^{n+4}n^3 P_{n,1}(u)\nonumber \\&\quad = \frac{t^5 \sqrt{1 - u^2}}{g^3} \left[ 1 + \frac{3 t (7 u - 13 t)}{g^2} \right. \nonumber \\&\quad \quad \left. + \frac{45 t^2 (t - u) (3 t - 2 u)}{g^4} - \frac{105 t^3 (t - u)^3}{g^6}\right] , \end{aligned}$$

(108)

$$\begin{aligned} S_{12}(t,u)&= \sum \limits _{n=2}^{\infty }t^{n+4}\frac{1}{n + 1}P_{n,2}(u)\nonumber \\&= t^3 (1 - u^2) \left[ \frac{1}{(1 - u)^2} - \frac{t^2}{g^3 (g + t - u)} \right. \nonumber \\&\left. - \frac{(g + t)^2}{g^2 (g + t - u)^2}\right] ,\end{aligned}$$

(109)

$$\begin{aligned} S_{13}(t,u)&= \sum \limits _{n=2}^{\infty }t^{n+4}P_{n,2}(u)= \frac{3 t^6 (1 - u^2)}{g^5},\end{aligned}$$

(110)

$$\begin{aligned} S_{14}(t,u)&= \sum \limits _{n=2}^{\infty }t^{n+4}nP_{n,2}(u)\nonumber \\&= \frac{3 t^6 (1 - u^2)}{g^5} \left[ 2 + \frac{5 t (u - t)}{g^2}\right] ,\end{aligned}$$

(111)

$$\begin{aligned} S_{15}(t,u)&= \sum \limits _{n=2}^{\infty }t^{n+4}n^2 P_{n,2}(u)=\frac{3 t^6 (1 - u^2)}{g^5} \left[ 4 \right. \nonumber \\&\left. + \frac{5 t (5 u - 6 t)}{g^2} + \frac{35 t^2 (t - u)^2}{g^4}\right] ,\end{aligned}$$

(112)

$$\begin{aligned} S_{16}(t,u)&= \sum \limits _{n=3}^{\infty }t^{n+4}\frac{1}{n + 1}P_{n,3}(u)\nonumber \\&= t^3 \sqrt{(1 - u^2)^{3}} \left[ \frac{2}{(1 - u)^3} - \frac{3 t^3}{g^5 (g + t - u)} \right. \nonumber \\&\left. - \frac{3 t^2 (g + t)}{g^4 (g + t - u)^2} - \frac{2 (g + t)^3}{g^3 (g + t - u)^3}\right] ,\end{aligned}$$

(113)

$$\begin{aligned} S_{17}(t,u)&= \sum \limits _{n=3}^{\infty }t^{n+4}P_{n,3}(u)= \frac{15 t^7 \sqrt{(1 - u^2)^{3}}}{g^7},\end{aligned}$$

(114)

$$\begin{aligned} S_{18}(t,u)&= \sum \limits _{n=3}^{\infty }t^{n+4}nP_{n,3}(u)\nonumber \\&= \frac{15 t^7 \sqrt{(1 - u^2)^{3}}}{g^7} \left[ 3 + \frac{7 t (u - t)}{g^2}\right] ,\end{aligned}$$

(115)

$$\begin{aligned} S_{19}(t,u)&= \sum \limits _{n=2}^{\infty }t^{n+4}\frac{1}{n - 1}P_{n,0}(u)\nonumber \\&= t^4 \left[ 1 - g - t u - t u \ln \left( \frac{1 + g - t u}{2}\right) \right] ,\end{aligned}$$

(116)

$$\begin{aligned} S_{20}(t,u)&= \sum \limits _{n=2}^{\infty }t^{n+4}P_{n,0}(u)= S_{2}(t,u) - t^4 (1 + t u),\end{aligned}$$

(117)

$$\begin{aligned} S_{21}(t,u)&= \sum \limits _{n=2}^{\infty }t^{n+4}nP_{n,0}(u) = S_{3}(t,u) - t^5 u,\end{aligned}$$

(118)

$$\begin{aligned} S_{22}(t,u)&= \sum \limits _{n=2}^{\infty }t^{n+4}n^2 P_{n,0}(u)= S_{4}(t,u) - t^5 u,\end{aligned}$$

(119)

$$\begin{aligned} S_{23}(t,u)&= \sum \limits _{n=2}^{\infty }t^{n+4}n^3 P_{n,0}(u)= S_{5}(t,u) - t^5 u, \end{aligned}$$

(120)

$$\begin{aligned} S_{24}(t,u)&= \sum \limits _{n=2}^{\infty }t^{n+4}\frac{1}{n - 1}P_{n,1}(u)\nonumber \\&= t^5 \sqrt{1 - u^2} \left[ \frac{(g + 1)^2}{g (1 + g - t u)} - 2 \right. \nonumber \\&\left. - \ln \left( \frac{1 + g - t u}{2}\right) \right] ,\end{aligned}$$

(121)

$$\begin{aligned} S_{25}(t,u)&= \sum \limits _{n=2}^{\infty }t^{n+4}P_{n,1}(u)= S_{8}(t,u) - t^5 \sqrt{1 - u^2},\end{aligned}$$

(122)

$$\begin{aligned} S_{26}(t,u)&= \sum \limits _{n=2}^{\infty }t^{n+4}nP_{n,1}(u) = S_{9}(t,u) - t^5 \sqrt{1 - u^2},\nonumber \\\end{aligned}$$

(123)

$$\begin{aligned} S_{27}(t,u)&= \sum \limits _{n=2}^{\infty }t^{n+4}n^2 P_{n,1}(u)= S_{10}(t,u) - t^5 \sqrt{1 - u^2},\nonumber \\\end{aligned}$$

(124)

$$\begin{aligned} S_{28}(t,u)&= \sum \limits _{n=2}^{\infty }t^{n+4}\frac{1}{n - 1}P_{n,2}(u)\nonumber \\&= \frac{t^6 (1 - u^2)}{1 + g - t u} \left[ 1 + \frac{1}{g^3} + \frac{(g + 1)^3}{g^2 (1 + g - t u)}\right] ,\nonumber \\\end{aligned}$$

(125)

$$\begin{aligned} S_{29}(t,u)&= \sum \limits _{n=3}^{\infty }t^{n+4}\frac{1}{n - 1}P_{n,3}(u)\nonumber \\&= \frac{t^7 \sqrt{(1 - u^2)^{3}}}{1 + g - t u} \left[ \frac{3}{g^5} + \frac{1}{1 + g - t u} \right. \nonumber \\&\left. + \frac{4 g + 3}{g^4 (1 + g - t u)} + \frac{2 (g + 1)^4}{g^3 (1 + g - t u)^2}\right] . \end{aligned}$$

(126)

Note that some of the summation rules may be found, e.g. in (Pick et al. 1973; Moritz 1980; Martinec 2003; Šprlák and Novák 2014).