On the foundation of monopoly in bilateral exchange

Abstract

We address the problem of monopoly in general equilibrium in a mixed version of a monopolistic two-commodity exchange economy where the monopolist, represented as an atom, is endowed with one commodity and “small traders,” represented by an atomless part, are endowed only with the other. First we provide an economic theoretical foundation of the monopoly solution in this bilateral framework through a formalization of an explicit trading process inspired by Pareto (Cours d’économie politique. F. Rouge Editeur, Lausanne, 1896) for an exchange economy with a finite number of commodities, and we give the conditions under which our monopoly solution has the geometric characterization proposed by Schydlowsky and Siamwalla (Q J Econ 80:147–153, 1966). Then, we provide a game theoretical foundation of our monopoly solution through a two-stage reformulation of our model. This allows us to prove that the set of the allocations corresponding to a monopoly equilibrium and the set of the allocations corresponding to a subgame perfect equilibrium of the two-stage game coincide. Finally, we compare our model of monopoly with a bilateral exchange version of a pioneering model proposed by Forchheimer (Jahrbuch für Gesetzgebung, Verwaltung und Volkswirschafts im Deutschen Reich 32:1–12, 1908), known as a model of “partial monopoly” since there a monopolist shares a market with a“competitive fringe.” Journal of Economic Literature Classification Numbers: D42, D51.

1 Introduction

To the best of our knowledge, Vilfredo Pareto was the first who gave a formalized treatment of the problem of monopoly for a general pure exchange economy with any finite number of commodities, in the first volume of his Cours d’économie politique, published in 1896, pp. 62–68 (henceforth just Pareto (1896)). His monopoly quantity-setting solution rests on the assumption that the monopolist gets no utility from the only commodity he is endowed with and only cares about the revenue he can obtain by selling it.

Seventy years later, Schydlowsky and Siamwalla (1966) proposed a formulation of the problem of monopoly, without any mention to the previous work by Pareto (1896). In the context of a pure exchange economy, they considered a bilateral framework where one commodity is held by one trader behaving as a monopolist while the other is held by a “competitors’ community.” In contrast to Pareto’s analysis, the monopolist desires both commodities. The authors provided a geometrical representation of the monopoly solution as the point of tangency between the monopolist’s indifference curve and the offer curve of the competitors’ community.

In this paper, we consider the mixed version of a monopolistic two-commodity exchange economy introduced by Shitovitz (1973) in his Example 1, in which one commodity is held only by the monopolist, represented as an atom, and the other is held only by small traders, represented by an atomless part.

In our setup, the monopolist acts strategically, making a bid of the commodity he holds in exchange for the other commodity, while the atomless part behaves à la Walras. Given the monopolist’s bid, prices adjust to equate the monopolist’s bid to the aggregate net demand of the atomless part. Each trader belonging to the atomless part then obtains his Walrasian demand whereas monopolist’s final holding is determined as the difference between his endowment and his bid, for the commodity he holds, and as the value of his bid in terms of relative prices, for the other commodity. We define a monopoly equilibrium as a strategy played by the monopolist, corresponding to a positive bid of the commodity he holds, which guarantees him to obtain, via the trading process described above, a most preferred final holding among those he can achieve through his bids.

First of all, the analytical framework proposed in this paper to define and study monopoly equilibrium in bilateral exchange permits us to provide an economic theoretical foundation of the monopoly solution previously characterized in geometrical terms by Schydlowsky and Siamwalla (1966). Indeed, we show that, if our framework is simplified under the assumption that the aggregate demand of the atomless part for the commodity held by the monopolist is invertible and differentiable, a monopoly equilibrium has the geometric characterization proposed by Schydlowsky and Siamwalla (1966). This result rests on a notion which has a well-known counterpart in partial equilibrium analysis and was also used by Pareto (1896) to formulate his solution to the monopoly problem in exchange economies: the marginal revenue of the monopolist. This makes us also able to establish a relationship between our model and the standard models of monopoly in partial equilibrium.

Then, we adapt to our monopoly bilateral exchange context the version of the Shapley window model used by Busetto et al. (2020), and we assume that the atomless part behaves à la Cournot making bids of the commodity it holds. We show that there is no Cournot-Nash equilibrium in the market game generated by the strategic interaction between the monopolist and the atomless part through the Shapley window trading process, thereby confirming an analogous negative result obtained by Okuno et al. (1980, p. 24) for the monopolistic version of their bilateral strategic market game. Moreover, we provide an example exhibiting a bilateral exchange economy which admits a monopoly equilibrium but no Cournot-Nash equilibrium. Our example shows that it is not possible to provide a game theoretical foundation of our monopoly solution in terms of an equivalence between the set of the allocations corresponding to a monopoly equilibrium and the set of the allocations corresponding to a Cournot-Nash equilibrium, in a one-stage setting.

Sadanand (1988, p. 174) started from the negative result about the existence of a Cournot-Nash equilibrium in a one-shot monopolistic bilateral strategic market game obtained by Okuno et al. (1980), which led him to introduce a monopoly price-setting solution in a two-stage version of the strategic market game analyzed by those authors.

Following Sadanand (1988), we provide a sequential reformulation of the mixed version of the Shapley window model in terms of a two-stage game with observed actions where the quantity-setting monopolist moves first and the atomless part moves in the second stage, after observing the move of the monopolist in the first stage. This two-stage reformulation of our model allows us to provide a game theoretical foundation of the quantity-setting monopoly solution: we prove that the set of the allocations corresponding to a monopoly equilibrium and the set of those corresponding to a subgame perfect equilibrium of the two-stage game coincide.

Finally, we compare our model of monopoly with a bilateral exchange version of a pioneering model proposed by Forchheimer (1908): it is known in the literature as a model of “partial monopoly” since it assumes that a monopolist shares a market with a competitive fringe. We show that the two models, and the corresponding equilibria, differ and this is essentially due to the role played, in the partial monopoly one, by the competitive fringe in activating the market even when the monopolist does not supply anything of the commodity he holds. To capture this feature of the partial monopoly model, we formulate a notion of “fully active” subgame perfect equilibrium and use it to prove an equivalence between this concept and the quantity setting partial monopoly solution, this way providing it with a game theoretical foundation. This result neither implies nor is implied by the equivalence theorem we proved for the monopoly model. More precisely, we point out that the key difference between our monopoly model and the partial monopoly model is that in the former scenario, in the absence of a competitive fringe, if the monopolist chooses not to be active, the market cannot be active either. With a competitive fringe, the market can be active even if the monopolist is not. This entails that the partial monopoly model is a special case of the Cournot-Walras equilibrium model proposed by Busetto et al. (2008). Instead, unlike Busetto et al. (2008), the equivalence result concerning our monopoly model has to explicitly consider the case where, because the monopolist chooses not to be active, the market is not active either.

The paper is organized as follows. In Sect. 2, we introduce the mathematical model and we define the notion of a monopoly equilibrium. In Sect. 3, we show that our model provides an economic theoretical foundation of monopoly. In Sect. 4, we provide a game theoretical foundation of the monopoly solution in a two-stage framework. In Sect. 5, we provide an economic theoretical foundation of partial monopoly à la Forchheimer. In Sect. 6, we provide a game theoretical foundation of partial monopoly à la Forchheimer. In Sect. 7, we draw some conclusions and we suggest some further lines of research. The proofs of all the propositions are reported in the appendix.

2 Mathematical model

We consider a pure exchange economy with large traders, represented as atoms, and small traders, represented by an atomless part. The space of traders is denoted by the measure space $(T,\mathcal{T},\mu )$, where T is the set of traders, ${{{\mathcal {T}}}}$ is the $\sigma$-algebra of all $\mu$-measurable subsets of T, and $\mu$ is a real valued, non-negative, countably additive measure defined on ${{{\mathcal {T}}}}$. We assume that $(T,{{{\mathcal {T}}}},\mu )$ is finite, i.e., $\mu (T)<\infty$. Let $T_0$ denote the atomless part of T. We assume that $\mu (T_0)>0$.¹ Moreover, we assume that $T \setminus T_0=\{m\}$, i.e., the measure space $(T,{{{\mathcal {T}}}},\mu )$ contains only one atom, the “monopolist.” A null set of traders is a set of measure 0. Null sets of traders are systematically ignored throughout the paper. Thus, a statement asserted for “each” trader in a certain set is to be understood to hold for all such traders except possibly for a null set of traders. A coalition is a nonnull element of ${{{\mathcal {T}}}}$. The word “integrable” is to be understood in the sense of Lebesgue.

In the exchange economy, there are two different commodities. A commodity bundle is a point in $R^2_+$. An assignment (of commodity bundles to traders) is an integrable function $\textbf{x}$: $T \rightarrow R^2_+$. There is a fixed initial assignment $\textbf{w}$, satisfying the following assumption.

Assumption 1

$\textbf{w}^i(m)>0$, $\textbf{w}^j(m)=0$ and $\textbf{w}^i(t)=0$, $\textbf{w}^j(t)>0$, for each $t \in T_0$, $i=1 \text { or } 2$, $j=1 \text { or } 2$, $i \ne j$.

An allocation is an assignment $\textbf{x}$ such that $\int _T\textbf{x}(t)\,d\mu =\int _T\textbf{w}(t)\,d\mu$. The preferences of each trader $t \in T$ are described by a utility function $u_t:R^2_+ \rightarrow R$, satisfying the following assumptions.

Assumption 2

$u_t:R^2_+ \rightarrow R$ is continuous, strongly monotone, and strictly quasi-concave, for each $t \in T$.

Let ${{{\mathcal {B}}}}$ denote the Borel $\sigma$-algebra of $R^2_+$. Moreover, let ${{{\mathcal {T}}}} \bigotimes {{{\mathcal {B}}}}$ denote the $\sigma$-algebra generated by the sets $D\times F$ such that $D \in {{{\mathcal {T}}}}$ and $F \in {{{\mathcal {B}}}}$.

Assumption 3

$u: T \times R^2_+ \rightarrow R$, given by $u(t,x)=u_t(x)$, for each $t \in T$ and for each $x \in R^2_+$, is ${{{\mathcal {T}}}} \bigotimes {{{\mathcal {B}}}}$-measurable.

A price vector is a nonnull vector $p \in R^2_+$. Let $\textbf{X}^0: T_0 \times R^2_{++} \rightarrow \mathcal{P}(R^2_+)$ be a correspondence such that, for each $t \in T_0$ and for each $p \in R^2_{++}$, $\textbf{X}^0(t,p)=\text {argmax}\{u(x):\, x \in R^2_+ \text { and } px \le p\textbf{w}(t)\}$. For each $p \in R^2_{++}$, let $\int _{T_0}\textbf{X}^0(t,p)\,d\mu =\{\int _{T_0}{} \textbf{x}(t,p)\,d\mu :\,\textbf{x}(\cdot ,p)$ is integrable and $\textbf{x}(t,p) \in \textbf{X}^0(t,p), \text { for each } t \in T_0\}$. Since the correspondence $\textbf{X}^0(t,\cdot )$ is nonempty and single-valued, by Assumption 2, it is possible to define the Walrasian demand of traders in the atomless part as the function $\textbf{x}^0: T_0 \times R^2_{++} \rightarrow R^2_+$ such that $\textbf{X}^0(t,p)=\{\textbf{x}^0(t,p)\}$, for each $t \in T_0$ and for each $p \in R^2_{++}$.

We can now state and show the following proposition.

Proposition 1

Under Assumptions 1, 2, and 3, the function $\textbf{x}^0(\cdot ,p)$ is integrable and $\int _{T_0}{} \textbf{X}^0(t,p)\,d\mu =\int _{T_0}{} \textbf{x}^0(t,p)\,d\ \mu$ for, each $p \in R^2_{++}$.

Let $\textbf{E}(m)=\{(e_{ij}) \in R^4_+{:} \sum _{j=1}^2e_{ij} \le \textbf{w}^i(m),\,i=1,2\}$ denote the strategy set of atom m. We denote by $e \in \textbf{E}(m)$ a strategy of atom m, where $e_{ij}$, $i,j=1,2$, represents the amount of commodity i that atom m offers in exchange for commodity j. Moreover, we denote by E the matrix corresponding to a strategy $e \in \textbf{E}(m)$.

We then provide the following definition.

Definition 1

Given a strategy $e \in \textbf{E}(m)$, a price vector p is said to be market clearing if

$$\begin{aligned} p \in R^2_{++},\,\int _{T_0}\textbf{x}^{0j}(t,p)\,d\mu +\sum _{i=1}^2e_{ij}\mu (m)\frac{p^i}{p^j}=\int _{T_0}\textbf{w}^j(t)\,d\mu +\sum _{i=1}^2e_{ji}\mu (m), \end{aligned}$$

(1)

$j=1,2$.

The following proposition shows that market clearing price vectors can be normalized.

Proposition 2

Under Assumptions 1, 2, and 3, if p is a market clearing price vector, then $\alpha p$, with $\alpha >0$, is also a market clearing price vector.

Henceforth, we say that a price vector p is normalized if $p \in \Delta$ where $\Delta =\{p \in R^2_+{:}\sum _{i=1}^2p^i=1\}$. Moreover, we denote by $\partial \Delta$ the boundary of the unit simplex $\Delta$.

The next proposition shows that the two equations in (1) are not independent.

Proposition 3

Under Assumptions 1, 2, and 3, given a strategy $e \in \textbf{E}(m)$, a price vector $p \in \Delta \setminus \partial \Delta$ is market clearing for $j=1$ if and only if it is market clearing for $j=2$.

The next proposition is based on Property (iv) of the aggregate demand of an atomless set of traders established by Debreu (1982, p. 728).

Proposition 4

Under Assumptions 1, 2, and 3, let $\{p^n\}$ be a sequence of normalized price vectors such that $p^n \in \Delta {\setminus } \partial \Delta$, for each $n=1,2,\ldots$, and which converges to a normalized price vector ${{\bar{p}}}$. If ${{\bar{p}}}^i=0$ and $\textbf{w}^i(m)>0$, then the sequence $\{\int _{T_0}\textbf{x}^{0i}(t,p^n)\,d\mu \}$ diverges to $+\infty$.

The following proposition provides a necessary and sufficient condition for the existence of a market clearing price vector. In order to state and prove it, we provide the following preliminary definitions.

Definition 2

A square matrix G is said to be triangular if $g_{ij}=0$ whenever $i>j$ or $g_{ij}=0$ whenever $i<j$.

Definition 3

We say that commodities i, j stand in relation Q if $\textbf{w}^i(t)>0$, for each $t \in T_0$, and there is a nonnull subset $T^i$ of $T_0$ such that $u_t(\cdot )$ is differentiable, additively separable, i.e., $u_t(x)=v^i_t(x^i)+v^j_t(x^j)$, for each $x \in R^2_+$, and $\frac{dv^j_t(0)}{dx^j}=+\infty$, for each $t \in T^i$.²

Moreover, we introduce the following assumption.

Assumption 4

Commodities i, j stand in relation Q.

Proposition 5

Under Assumptions 1, 2, 3, and 4, given a strategy $e \in \textbf{E}(m)$, there exists a market clearing price vector $p \in \Delta {\setminus } \partial \Delta$ if and only if the matrix E is triangular.

We denote by $\pi (\cdot )$ a correspondence which associates, with each strategy $e \in \textbf{E}(m)$, the set of price vectors p satisfying (1), if E is triangular, and is equal to $\{0\}$, otherwise. A price selection $p(\cdot )$ is a function which associates, with each strategy selection $e \in \textbf{E}(m)$, a price vector $p \in \pi (e)$.

Given a strategy $e \in \textbf{E}(m)$ and a price vector p, consider the assignment determined as follows:

$$\begin{aligned} \textbf{x}^j(m,e,p) & = \textbf{w}^j(m)- \sum _{i=1}^2{e}_{ji}+\sum _{i=1}^2{e}_{ij}\frac{p^i}{p^j}, \text { if}\, p \in \Delta \setminus \partial \Delta ,\\ \textbf{x}^j(m,e,p) & = \textbf{w}^j(m), \text { otherwise}, \end{aligned}$$

$j=1,2$,

$$\begin{aligned} \textbf{x}^j(t,p) = \textbf{x}^{0j}(t,p), \text { if }p \in \Delta \setminus \partial \Delta ,\\ \textbf{x}^j(t,p) = \textbf{w}^j(t), \text { otherwise}, \end{aligned}$$

$j=1,2$, for each $t \in T_0$.

Given a strategy $e \in \textbf{E}(m)$ and a price selection $p(\cdot )$, traders’ final holdings are determined according to this rule and consequently expressed by the assignment

$$\begin{aligned} \textbf{x}(m) &= \textbf{x}(m,e,p(e)), \\ \textbf{x}(t) &= \textbf{x}(t,p(e)), \end{aligned}$$

for each $t \in T_0$.

The next proposition shows that traders’ final holdings constitute an allocation.

Proposition 6

Under Assumptions 1, 2, 3, and 4, given a strategy $e \in \textbf{E}(m)$ and a price selection $p(\cdot )$, the assignment $\textbf{x}(m)=\textbf{x}(m,e,p(e))$, $\textbf{x}(t)=\textbf{x}(t,p(e))$, for each $t \in T_0$, is an allocation.

We can now provide the definition of a monopoly equilibrium.

Definition 4

A strategy ${{\tilde{e}}} \in \textbf{E}(m)$ such that ${{\tilde{E}}}$ is triangular is a monopoly equilibrium, with respect to a price selection $p(\cdot )$, if

$$\begin{aligned} u_m(\textbf{x}(m,{{\tilde{e}}},p({{\tilde{e}}})) \ge u_m(\textbf{x}(m,e,p(e)), \end{aligned}$$

for each $e \in \textbf{E}(m)$.

A monopoly allocation is an allocation $\tilde{\textbf{x}}$ such that $\tilde{\textbf{x}}(m)=\textbf{x}(m,{{\tilde{e}}},p({{\tilde{e}}}))$ and $\tilde{\textbf{x}}(t)=\textbf{x}^0(t,p({{\tilde{e}}}))$, for each $t \in T_0$, where ${{\tilde{e}}}$ is a monopoly equilibrium, with respect to a price selection $p(\cdot )$.

3 An economic theoretical foundation of monopoly

The formalization of the monopoly problem in bilateral exchange proposed in the previous section can be simplified by introducing the assumption that the aggregate demand of the atomless part for the commodity held by the monopolist is invertible and differentiable. We show that, under these restrictions, our monopoly equilibrium has the geometric representation proposed by Schydlowsky and Siamwalla (1966) and can consequently be interpreted as its economic theoretical foundation. Moreover, since this result is obtained on the basis of concepts which have a counterpart in the traditional partial equilibrium analysis of monopoly, it permits us to establish a relationship between that analysis and our more general approach.

We start by proving the following proposition which states a necessary and sufficient condition for the atomless part’s aggregate demand to be invertible.

Proposition 7

Under Assumptions 1, 2, 3, and 4, let $\textbf{w}^i(m)>0$. Then, the function $\int _{T_0}{} \textbf{x}^{0i}(t,\cdot )\,d\mu$ is invertible if and only if, for each $x \in R_{++}$, there is a unique $p \in \Delta {\setminus } \partial \Delta$ such that $x=\int _{T_0}\textbf{x}^{0i}(t,p)\,d\mu$.

Let $p^{0i}(\cdot )$ denote the inverse of the function $\int _{T_0}{} \textbf{x}^{0i}(t,\cdot )\,d\mu$.

The following proposition shows that, when the aggregate demand of the atomless part for the commodity held by the monopolist is invertible, there exists a unique price selection.

Proposition 8

Under Assumptions 1, 2, 3, and 4, if $\textbf{w}^i(m)>0$ and the function $\int _{T_0}\textbf{x}^{0i}(t,\cdot )\,d\mu$ is invertible, then there exists a unique price selection $\mathring{p}(\cdot )$.

By analogy with partial equilibrium analysis, $\mathring{p}(\cdot )$ can be called the inverse demand function of the monopolist. When the aggregate demand of the atomless part for the commodity held by the monopolist is invertible, the monopoly equilibrium in Definition 4 can be reformulated with respect to monopolist’s inverse demand function $\mathring{p}(\cdot )$. Moreover, under this assumption, it can be expressed in terms of the notion of offer curve of the atomelss part, defined as the set $\{x \in R^2_+{:}x=\int _{T_0}\textbf{x}^0(t,p)\,d\mu \text { for some } p \in \Delta {\setminus } \partial \Delta \}$.

In order to provide the characterization of the monopoly equilibrium proposed by Schydlowsky and Siamwalla (1966), we need to introduce also the following assumption.

Assumption 5

$u_m:R^2_+ \rightarrow R$ is differentiable.

We have to show that, under the assumption that the aggregate demand of the atomless part for the commodity held by the monopolist is both invertible and differentiable, the monopoly equilibrium introduced in Definition 4 has the geometric characterization previously proposed by Schydlowsky and Siamwalla (1966): at a strictly positive monopoly allocation, the monopolist’s indifference curve is tangent to the atomless part’s offer curve.³

The following proposition shows that the function $h(\cdot )$, defined on $R_{++}$ and such that

$$\begin{aligned} p^ix^i+p^jx^j=p^i\int _{T_0}{} \textbf{w}^i(t)\,d\mu +p^j\int _{T_0}{} \textbf{w}^j(t)\,d\mu , \end{aligned}$$

where $p=p^{0i}(x^i)$ and $x^j=h(x^i)$, represents the offer curve of the atomless part in the sense that its graph coincides with the atomless part’s offer curve.

Proposition 9

Under Assumptions 1, 2, 3, and 4, if $\textbf{w}^i(m)>0$ and the function $\int _{T_0}\textbf{x}^{0i}(t,\cdot )\,d\mu$ is invertible, then the graph of the function $h(\cdot )$, the set $\{x \in R^2_+{:}x^j=h(x^i)\}$, coincides with the set $\{x \in R^2_+{:}x=\int _{T_0}\textbf{x}^0(t,p)\,d\mu \text { for some } p \in \Delta {\setminus } \partial \Delta \}$, the offer curve of the atomless part.

Borrowing from Pareto (1896), we now introduce in our general framework a notion which has a counterpart in partial equilibrium analysis: the marginal revenue of the monopolist.

In the rest of this section, with a slight abuse of notation, given a price vector $(p^i,p^j) \in \Delta \setminus \partial \Delta$, we denote by p the scalar $p=\frac{p^i}{p^j}$, whenever $\textbf{w}^i(m)>0$.

Suppose that $\textbf{w}^i(m)>0$, that the function $\int _{T_0}\textbf{x}^{0i}(t,\cdot )\,d\mu$ is invertible, and that the function $p^{0i}(\cdot )$ is differentiable. Then, $\mathring{p}(\cdot )$, the inverse demand function of the monopolist, is differentiable and we have that $\frac{d\mathring{p}(e)}{de_{ij}}=\frac{dp^{0i}(e_{ij}\mu (m))}{dx^i}\mu (m)$, at each $e \in \textbf{E}(m)$ such that E is triangular, by Proposition 7. In this context, the revenue of the monopolist can be defined as $\mathring{p}(e)e_{ij}$ and his marginal revenue as $\frac{d\mathring{p}(e)}{de_{ij}}e_{ij}+\mathring{p}(e)$, for each $e \in \textbf{E}(m)$ such that E is triangular.

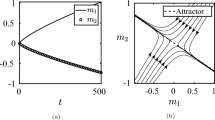
Then, the next proposition establishes that, at an interior monopoly solution, the slope of the monopolist’s indifference curve and the slope of the atomless part’s offer curve are both equal to the opposite of the monopolist’s marginal revenue. Therefore, the tangency characterization of a monopoly equilibrium is demonstrated. This provides a formal foundation of the geometric characterization of the monopoly equilibrium proposed by Schydlowsky and Siamwalla (1966). At the same time, since the tangency property is expressed in terms of the concept of marginal revenue of the monopolist, it casts a bridge between our monopoly equilibrium and the notions proposed within the standard analyses of monopoly in a partial equilibrium context.

Proposition 10

Under Assumptions 1, 2, 3, 4, and 5, if $\textbf{w}^i(m)>0$, the function $\int _{T_0}\textbf{x}^{0i}(t,\cdot )\,d\mu$ is invertible, the function $p^{0i}(\cdot )$ is differentiable, and ${{\tilde{e}}} \in \textbf{E}(m)$ is a monopoly equilibrium such that ${{\tilde{e}}}<\textbf{w}^i(m)$, then

$$\begin{aligned} -\frac{\frac{\partial u_m(\tilde{\textbf{x}}(m))}{\partial x^i}}{\frac{\partial u_m(\tilde{\textbf{x}}(m))}{\partial x^j}}=-\left( \frac{d\mathring{p}({{\tilde{e}}})}{de_{ij}}{{\tilde{e}}}_{ij}+\mathring{p}({{\tilde{e}}})\right) =\frac{dh(\int _{T_0}\tilde{\textbf{x}}^i(t)\,d\mu )}{dx^i}, \end{aligned}$$

where $\tilde{\textbf{x}}$ is the monopoly allocation corresponding to ${{\tilde{e}}}$.

Finally, we provide an example that illustrates the geometric characterization of a monopoly equilibrium proposed by Schydlowsky and Siamwalla (1966).

Example 1

Consider the following specification of an exchange economy satisfying Assumptions 1, 2, 3, and 4. $T_0=[0,1]$, $T \setminus T_0=\{m\}$, $\mu (m)=1$, $\textbf{w}(m)=(1,0)$, $u_m(x)=\frac{1}{2}x^1+\sqrt{x^2}$, $T_0$ is taken with Lebesgue measure, $\textbf{w}(t)=(0,1)$, $u_t(x)=\sqrt{x^1}+x^2$, for each $t \in T_0$. Then, at the unique monopoly equilibrium ${{\tilde{e}}} \in \textbf{E}(m)$, the slope of the indifference curve of the monopolist is equal to the opposite of his marginal revenue, which, in turn, is equal to the slope of the function which represents the offer curve of the atomless part.

Proof

The unique monopoly equilibrium is the strategy ${{\tilde{e}}} \in \textbf{E}(m)$ such that ${{\tilde{e}}}_{12}=\frac{1}{4}$, $\mathring{p}({{\tilde{e}}})=1$, $\tilde{\textbf{x}}(m)=(\frac{3}{4},\frac{1}{4})$, and $\tilde{\textbf{x}}(t)=(\frac{1}{4},\frac{3}{4})$, for each $t \in T_0$. Moreover, we have that $x^2=h(x^1)=-\frac{\sqrt{x}^1}{2}+1$ and

$$\begin{aligned} -\frac{\frac{\partial u_m(\tilde{\textbf{x}}(m))}{\partial x^i}}{\frac{\partial u_m(\tilde{\textbf{x}}(m))}{\partial x^j}}=-\left( \frac{d\mathring{p}({{\tilde{e}}})}{de_{ij}}{{\tilde{e}}}_{ij}+\mathring{p}({{\tilde{e}}})\right) =-\frac{1}{2}=\frac{dh(\int _{T_0}\tilde{\textbf{x}}^i(t)\,d\mu )}{dx^i}. \end{aligned}$$

$\square$

4 A game theoretical foundation of monopoly

We now provide the definition of a Cournot-Nash equilibrium in the bilateral exchange model introduced in Sect. 2, adapting to this framework the version of the Shapley window model used by Busetto et al. (2020).

A strategy correspondence is a correspondence $\textbf{B}:T \rightarrow {{{\mathcal {P}}}}(R^4_+)$ such that, for each $t \in T$, $\textbf{B}(t)=\{(b_{ij}) \in R^4_+{:} \sum _{j=1}^2b_{ij} \le \textbf{w}^i(t),\,i=1,2\}$. We denote by $b(t) \in \textbf{B}(t)$ a strategy of trader t, where $b_{ij}(t)$, $i,j=1,2$, represents the amount of commodity i that trader t offers in exchange for commodity j. A strategy selection is an integrable function $\textbf{b}:T \rightarrow R^4_+$, such that, for each $t \in T$, $\textbf{b}(t) \in \textbf{B}(t)$. Given a strategy selection $\textbf{b}$, we denote by $\bar{\textbf{B}}$ the matrix such that $\bar{\textbf{b}}_{ij}=\left(\int _T \textbf{b}_{ij}(t)\,d\mu \right)$, $i,j=1,2$. Moreover, we denote by $\textbf{b} {\setminus } b(t)$ the strategy selection obtained from $\textbf{b}$ by replacing $\textbf{b}(t)$ with $b(t) \in \textbf{B}(t)$.

We need to provide now the following two definitions (see Sahi and Yao 1989).

Definition 5

A nonnegative square matrix G is said to be irreducible if, for every pair (i, j), with $i \ne j$, there is a positive integer k such that $g^{(k)}_{ij}>0$, where $g^{(k)}_{ij}$ denotes the ij-th entry of the k-th power $G^k$ of G.

Definition 6

Given a strategy selection $\textbf{b}$, a price vector p is said to be market clearing if

$$\begin{aligned} p \in R^2_{++},\,\sum _{i=1}^2p^i\bar{\textbf{b}}_{ij} = p^j\left(\sum _{i=1}^2\bar{\textbf{b}}_{ji}\right),\, j=1,2. \end{aligned}$$

(2)

By Lemma 1 in Sahi and Yao (1989), there is a unique, up to a scalar multiple, price vector p satisfying (2) if and only if $\bar{\textbf{B}}$ is irreducible. Then, we denote by $p(\textbf{b})$ a function which associates with each strategy selection $\textbf{b}$ the unique, up to a scalar multiple, price vector p satisfying (2), if $\bar{\textbf{B}}$ is irreducible, and is equal to 0, otherwise. For each strategy selection $\textbf{b}$ such that $p(\textbf{b}) \gg 0$, we assume that the price vector $p(\textbf{b})$ is normalized.

Given a strategy selection $\textbf{b}$ and a price vector p, consider the assignment determined as follows:

$$\begin{aligned} \textbf{x}^j(t,\textbf{b}(t),p) &= \textbf{w}^j(t)- \sum _{i=1}^2\textbf{b}_{ji}(t)+\sum _{i=1}^2\textbf{b}_{ij}(t)\frac{p^i}{p^j}, \text { if }p \in \Delta \setminus \partial \Delta ,\\ \textbf{x}^j(t,\textbf{b}(t),p) &= \textbf{w}^j(t), \text { otherwise}, \end{aligned}$$

$j=1,2$, for each $t \in T$.

Given a strategy selection $\textbf{b}$ and the function $p(\textbf{b})$, traders’ final holdings are determined according to this rule and consequently expressed by the assignment

$$\begin{aligned} \textbf{x}(t)=\textbf{x}(t,\textbf{b}(t),p(\textbf{b})), \end{aligned}$$

for each $t \in T$. It is straightforward to show that this assignment is an allocation.

We are now able to define a notion of Cournot-Nash equilibrium for this reformulation of the Shapley window model.

Definition 7

A strategy selection $\hat{\textbf{b}}$ such that $\bar{\hat{\textbf{B}}}$ is irreducible is a Cournot-Nash equilibrium if

$$\begin{aligned} u_t(\textbf{x}(t,\hat{\textbf{b}}(t),p(\hat{\textbf{b}}))) \ge u_t(\textbf{x}(t,b(t),p(\hat{\textbf{b}} \setminus b(t)))), \end{aligned}$$

for each $b(t) \in \textbf{B}(t)$ and for each $t \in T$.

We notice that, as this definition of a Cournot-Nash equilibrium explicitly refers to irreducible matrices, it applies only to active equilibria according to Definition 4 in Sahi and Yao (1989, p. 330). A Cournot-Nash allocation is an allocation $\hat{\textbf{x}}$ such that $\hat{\textbf{x}}(t)=\textbf{x}(t,\hat{\textbf{b}}(t),p(\hat{\textbf{b}}))$, for each $t \in T$, where $\hat{\textbf{b}}$ is a Cournot-Nash equilibrium.

The next proposition provides, for our framework, the same negative result about the existence of a Cournot-Nash equilibrium obtained by Okuno et al. (1980, p. 24) and by Sadanand (1988, p. 174).

Proposition 11

Under Assumptions 1, 2, 3, and 4, there exists no Cournot-Nash equilibrium.

Proposition 11 has the relevant consequence that the set of monopoly allocations cannot coincide with the set of Cournot-Nash allocations in a one-stage setting, as confirmed by the following example.

Example 2

Consider the exchange economy specified in Example 1. Then, there is a unique monopoly allocation and no Cournot-Nash allocation.

Proof

From Example 1, we have that the unique monopoly equilibrium is the strategy ${{\tilde{e}}} \in \textbf{E}(m)$ such that ${{\tilde{e}}}_{12}=\frac{1}{4}$ and the unique monopoly allocation is $\tilde{\textbf{x}}(m)=(\frac{3}{4},\frac{1}{4})$ and $\tilde{\textbf{x}}(t)=(\frac{1}{4},\frac{3}{4})$, for each $t \in T_0$. However, there is no Cournot-Nash allocation, by Proposition 12. $\square$

The example shows the nonequivalence between the set of monopoly allocations and Cournot-Nash allocations in a one-stage game. The analogous negative result reached by Okuno et al. (1980) led these authors to conclude: “[...] We are unable to model pure monopoly without a competitive fringe in a useful way in this setup” (see Footnote 1, p. 24).

In his pathbreaking analysis of monopoly in mixed exchange economies, Sadanand (1988) already recognized the two stage-flavor of monopoly equilibrium. Taking inspiration from his work, we now introduce a two-stage game where the monopolist moves first and the atomless part moves in the second stage, after observing the move of the monopolist in the first stage. Therefore, we provide a sequential reformulation of the mixed version of the Shapley window model introduced in the previous section, in terms of a two-stage game with observed actions, following Fudenberg and Tirole (1991, p. 70).

The game is played in two stages, labelled as 0 and 1. An action correspondence in stage 0 is a correspondence $\textbf{A}^0:T \rightarrow {{{\mathcal {P}}}}(R^4_+)$ such that $\textbf{A}^0(m)=\{(a_{ij}) \in R^4_+{:} \sum _{j=1}^2a_{ij} \le \textbf{w}^i(m),\,i=1,2\}$ and $\textbf{A}^0(t)$ is the singleton {“do nothing”}, for each $t \in T_0$. An action correspondence in stage 1 is a correspondence $\textbf{A}^1:T \rightarrow {{{\mathcal {P}}}}(R^4_+)$ such that $\textbf{A}^1(m)$ is the singleton {“do nothing”} and $\textbf{A}^1(t)=\{(a_{ij}) \in R^4_+{:} \sum _{j=1}^2a_{ij} \le \textbf{w}^i(t),\,i=1,2\}$, for each $t \in T_0$. We denote by $a^0(t) \in \textbf{A}^0(t)$ an action of trader t in stage 0, where $a^0_{ij}(m)$, $i,j=1,2$, represents the amount of commodity i that atom m offers in exchange for commodity j. An action selection in stage 0 is a function $\textbf{a}^0:T \rightarrow R^4_+$, such that $\textbf{a}^0(t) \in \textbf{A}^0(t)$, for each $t \in T$. We denote by $a^1(t) \in \textbf{A}^1(t)$ an action of trader t in stage 1, where $a^1_{ij}(t)$, $i,j=1,2$, represents the amount of commodity i that a trader $t \in T_0$ offers in exchange for commodity j. An action selection in stage 1 is a function $\textbf{a}^1:T \rightarrow R^4_+$, whose restriction on $T_0$ is integrable, such that $\textbf{a}^1(t) \in \textbf{A}^1(t)$, for each $t \in T$. Let $S^0$ and $S^1$ denote the sets of all action selections in stage 0 and in stage 1, respectively. Any action selection at the end of a stage determines a history at the beginning of the next stage.

We denote by $\textbf{h}^0=\emptyset$ the history at the beginning of stage 0 and by $\textbf{h}^1$ a history at the beginning of stage 1 where $\textbf{h}^1=\textbf{a}^0$, for some $\textbf{a}^0 \in S^0$. Let $H^0$ and $H^1$ denote the sets of all stage 0 and stage 1 histories, respectively, where $H^0=\emptyset$ and $H^1=S^0$. Let $H^2=S^0 \times S^1$ denote the set of all terminal histories. Given a terminal history $\textbf{h}^2 = (\textbf{a}^0,\textbf{a}^1)$, we denote by $\bar{\textbf{A}}$ the matrix such that $\bar{\textbf{a}}_{ij} = \textbf{a}_{ij}^0(m)+\int _{T_0}{} \textbf{a}^1_{ij}(t)\,d\mu$, $i,j=1,2$.

We need now to introduce the following definition (see Sahi and Yao 1989).

Definition 8

Given a terminal history $\textbf{h}^2=(\textbf{a}^0,\textbf{a}^1$), a price vector p is said to be market clearing if

$$\begin{aligned} p \in R^2_{++},\,\sum _{i=1}^2p^i\bar{\textbf{a}}_{ij}=p^j(\sum _{i=1}^2\bar{\textbf{a}}_{ji}),\, j=1,2. \end{aligned}$$

(3)

By Lemma 1 in Sahi and Yao (1989), there is a unique, up to a scalar multiple, price vector p satisfying (3) if and only if $\bar{\textbf{A}}$ is irreducible. Then, we denote by $p(\textbf{h}^2)$ a function which associates with each final history $\textbf{h}^2=(\textbf{a}^0,\textbf{a}^1)$ the unique, up to a scalar multiple, price vector p satisfying (3), if $\bar{\textbf{A}}$ is irreducible, and is equal to 0, otherwise. For each final history $\textbf{h}^2=(\textbf{a}^0,\textbf{a}^1)$ such that $p(\textbf{h}^2) \gg 0$, we assume that the price vector $p(\textbf{h}^2)$ is normalized.

Given a terminal history $\textbf{h}^2=(\textbf{a}^0,\textbf{a}^1)$ and a price vector p, consider the assignment determined as follows:

$$\begin{aligned} \textbf{x}^j(m,\textbf{h}^2(m),p)= & {} \textbf{w}^j(m)- \sum _{i=1}^2\textbf{a}^0_{ji}(m)+\sum _{i=1}^2\textbf{a}^0_{ij}(m)\frac{p^i}{p^j}, \text { if }p \in \Delta \setminus \partial \Delta ,\\ \textbf{x}^j(m,\textbf{h}^2(m),p)= & {} \textbf{w}^j(m), \text { otherwise}, \end{aligned}$$

$j=1,2$,

$$\begin{aligned} \textbf{x}^j(t,\textbf{h}^2(t),p) &= \textbf{w}^j(t)- \sum _{i=1}^2\textbf{a}^1_{ji}(t)+\sum _{i=1}^2\textbf{a}^1_{ij}(t)\frac{p^i}{p^j}, \text { if }p \in \Delta \setminus \partial \Delta ,\\ \textbf{x}^j(t,\textbf{b}(t),p) &= \textbf{w}^j(t), \text { otherwise}, \end{aligned}$$

$j=1,2$, for each $t \in T_0$.

Given a terminal history $\textbf{h}^2=(\textbf{a}^0,\textbf{a}^1$) and the function $p(\textbf{h}^2)$, traders’ final holdings are determined according to this rule and consequently expressed by the assignment

$$\begin{aligned} \textbf{x}(t)=\textbf{x}(t,\textbf{h}^2(t),p(\textbf{h}^2)), \end{aligned}$$

for each $t \in T$. It is straightforward to show that this assignment is an allocation.

We denote by s(t) a strategy of trader t, where s(t) denotes the sequence of functions $\{s^0(t,\cdot ),s^1(t,\cdot )\}$ such that $s^0(t,\cdot ): H^0 \rightarrow \textbf{A}^0(t)$ and $s^1(t,\cdot ): H^1 \rightarrow \textbf{A}^1(t)$. A strategy profile $\textbf{s}$ is a map which associates with each $t \in T$ a sequence of functions $\{\textbf{s}^0,\textbf{s}^1\}$ such that $\textbf{s}^0(t,\cdot ): H^0 \rightarrow \textbf{A}^0(t)$, $\textbf{s}^1(t,\cdot ): H^1 \rightarrow \textbf{A}^1(t)$, $\textbf{s}^0(\cdot ,\textbf{h}^0) \in S^0$, and $\textbf{s}^1(\cdot ,\textbf{h}^1) \in S^1$, for each $\textbf{h}^1 \in H^1$. Given a strategy profile $\textbf{s}$, the functions $\textbf{s}^0(\cdot ,\textbf{h}^0)$ and $\textbf{s}^1(\cdot ,\textbf{h}^1)$, for each $\textbf{h}^1 \in H^1$, are called strategy selections. We denote by $\textbf{s} {\setminus } s(t)=\{\textbf{s}^0 {\setminus } s(t,\cdot ),\textbf{s}^1 {\setminus } s^1(t,\cdot )\}$ the strategy profile obtained from $\textbf{s}^0$ and $\textbf{s}^1$ by replacing, respectively, $\textbf{s}^0(t,\cdot )$ with $s^0(t,\cdot )$ and $\textbf{s}^1(t,\cdot )$ with $s^1(t,\cdot )$. Finally, we denote by $\textbf{h}^2(\textbf{s})$ the function which associates with each strategy profile $\textbf{s}$ the terminal history which corresponds to the action selections $\{\textbf{a}^0(\textbf{s}),\textbf{a}^1(\textbf{s})\}$ such that $\textbf{a}^0(\textbf{s})=\textbf{s}^0(\cdot ,\textbf{h}^0)$ and $\textbf{a}^1(\textbf{s})=\textbf{s}^1(\cdot ,\textbf{h}^1)$, with $\mathbf{h^1}=\textbf{s}^0(\cdot ,\textbf{h}^0)$, and by $\bar{\textbf{A}}(\textbf{s})$ the corresponding aggregate matrix.

We now proceed to consider the subgame represented by the stage 1 of the game outlined above, given the history $\textbf{h}^1 \in H^1$. Given a strategy s(t) of trader t and a history $\textbf{h}^1 \in H^1$, we denote by $s|\textbf{h}^1(t)$ the action such that $s|\textbf{h}^1(t)=s^1(t,\textbf{h}^1)$. Given a strategy profile $\textbf{s}$ and a history $\textbf{h}^1 \in H^1$, we denote by $\textbf{s}|\textbf{h}^1$ the strategy selection such that $\textbf{s}|\textbf{h}^1(t)=\textbf{s}^1(t,\textbf{h}^1)$, for each $t \in T$. Given a history $\textbf{h}^1 \in H^1$, we denote by $\textbf{s}|\textbf{h}^1 \setminus s|\textbf{h}^1(t)$ the strategy selection obtained from $\textbf{s}|\textbf{h}^1$ by replacing $\textbf{s}|\textbf{h}^1(t)$ with $s|\textbf{h}^1(t)$. Finally, we denote by $\textbf{h}^2(\textbf{s}|\textbf{h}^1)$ the function which associates with each strategy selection $\textbf{s}|\textbf{h}^1$ the terminal history which corresponds to the action selections $\{\textbf{a}^0(\textbf{s}|\textbf{h}^1),\textbf{a}^1(\textbf{s}|\textbf{h}^1)\}$ such that $\textbf{a}^0(\textbf{s}|\textbf{h}^1)=\textbf{h}^1$ and $\textbf{a}^1(\textbf{s}|\textbf{h}^1)=\textbf{s}|\textbf{h}^1$, and by $\bar{\textbf{A}}(\textbf{s}|\textbf{h}^1)$ the corresponding aggregate matrix.

We are now able to define the notion of subgame perfect equilibrium for the two-stage game described above.

Definition 9

A strategy profile $\textbf{s}^{*}$ such that $\bar{\textbf{A}}(\textbf{s}^{*})$ is irreducible is a subgame perfect equilibrium if

$$\begin{aligned} u_t(\textbf{x}(t,\textbf{h}^2(\textbf{s}^{*})(t),p(\textbf{h}^2(\textbf{s}^{*})))) \ge u_t(\textbf{x}(t,\textbf{h}^2(\textbf{s}^{*} \setminus s(t))(t),p(\textbf{h}^2(\textbf{s}^{*} \setminus s(t))))), \end{aligned}$$

for each s(t) and for each $t \in T$, $\bar{\textbf{A}}(\textbf{s}^{*}|\textbf{h}^1))$ is irreducible, for each $\textbf{h}^1 \in H^1$ such that $\textbf{h}^1(m)>0$, and

$$\begin{aligned}{} & {} u_t(\textbf{x}(t,\textbf{h}^2(\textbf{s}^{*}|\textbf{h}^1)(t),p(\textbf{h}^2(\textbf{s}^{*}|\textbf{h}^1))))\\{} & {} \ge u_t(\textbf{x}(t,\textbf{h}^2(\textbf{s}^{*}|\textbf{h}^1 \setminus s|\textbf{h}^1)(t))(t),p(\textbf{h}^2(\textbf{s}^{*}|\textbf{h}^1 \setminus s|\textbf{h}^1)(t))))), \end{aligned}$$

for each $\textbf{h}^1 \in H^1$, for each $s|\textbf{h}^1(t)$, and for each $t \in T$.

A subgame perfect allocation is an allocation $\textbf{x}^{*}$ such that $\textbf{x}(t,\textbf{h}^2(\textbf{s}^{*})(t),$ $p(\textbf{h}^2(\textbf{s}^{*})))$, for each $t \in T$, where $\textbf{s}^{*}$ is a subgame perfect equilibrium.

The following proposition shows the equivalence between the set of monopoly allocations and the set of subgame perfect allocations for our two-stage game.

Proposition 12

Under Assumptions 1, 2, 3, and 4, the set of monopoly allocations coincides with the set of subgame perfect allocations.

We support this result by means of the following example.

Example 3

Consider the exchange economy specified in Example 1. Then, there is a unique monopoly allocation which is also the unique subgame perfect allocation.

Proof

From Example 1, we have that the unique monopoly equilibrium is the strategy ${{\tilde{e}}} \in \textbf{E}(m)$ such that ${{\tilde{e}}}_{12}=\frac{1}{4}$ and the unique monopoly allocation is $\tilde{\textbf{x}}(m)=(\frac{3}{4},\frac{1}{4})$ and $\tilde{\textbf{x}}(t)=(\frac{1}{4},\frac{3}{4})$, for each $t \in T_0$. Moreover, the strategy profile $\tilde{\textbf{s}}$ such that $\textbf{a}^0_{12}(\tilde{\textbf{s}})(m)=\frac{1}{4}$ and $\textbf{a}^1_{21}(\tilde{\textbf{s}})(t)=\frac{1}{4}$, for each $t \in T_0$, is the unique subgame perfect equilibrium and $\tilde{\textbf{x}}(t)=\textbf{x}(t,\textbf{h}^2(\tilde{\textbf{s}})(t),p(\textbf{h}^2(\tilde{\textbf{s}})))$, for each $t \in T$. Hence, the unique monopoly allocation is also the unique subgame perfect allocation. $\square$

The result in Example 3 is explained by the fact that, in a two-stage game, the monopolist is able to modify the supply of the commodity held by the atomless part and to reach the monopoly equilibrium. In contrast, as shown by Example 2, in a one-stage game, the supply is fixed, leading the monopolist to offer an infinitesimal quantity of the commodity he holds.

5 An economic theoretical foundation of partial monopoly à la Forchheimer

In this section, we propose a bilateral exchange version of the pioneering model of partial monopoly proposed by Forchheimer (1908), where a monopolist shares a market with a competitive fringe (for a detailed analysis of Forchheimer’s work, see also Reid (1979)), and compare it with the monopoly model developed in the previous sections. We show that the two models, and the corresponding equilibria, are different. Rather, we argument that Forchheimer’s partial monopoly model can be interpreted as a special case of the Cournot-Walras framework proposed by Busetto et al. (2008). Borrowing from the work of these authors, we formulate the concept of fully active subgame perfect equilibrium, which captures the main feature of Forchheimer’s approach, and use it to provide a game theoretical foundation to the partial monopoly solution.

In order to translate the model proposed by Forchheimer (1908) into the bilateral exchange framework, Assumption 1 in Sect. 2 has to be reformulated as follows.

Assumption 1

$\text{w}^i(m)>0$, $\text{w}^j(m)=0$ and $\text{w}^i(t)=0$, $i=1 \text { or } 2$, $j=1 \text { or } 2$, $i \ne j$. Moreover, there is a coalition $S \subset T_0$ such that $\text{w}^1(t)>0$, $\text{w}^2(t)=0$, for each $t \in S$, $\text{w}^1(t)=0$, $\text{w}^2(t)>0$, for each $t \in T_0 {\setminus } S$.

Assumption $1^{\prime }$ introduces a competitive fringe in bilateral exchange by requiring that the monopolist shares the endowment of the commodity he holds with a fraction of the atomless part, and is a special case of Assumption 1 in Busetto et al. (2018).

These authors, in their Assumption 4, imposed that the set of commodities is strongly connected in terms of a relation based on traders’ characteristics, called relation C. This relation can be adapted to the bilateral framework as follows.

Definition 10

We say that commodities i, j stand in relation C if there is a coalition $T^i$ such that $T^i \subset \{t \in T_0:\textbf{w}^i(t)>0,\,\textbf{w}^j(t)=0\}$, $u_t(\cdot )$ is differentiable, additively separable, i.e., $u_t(x)=v^i_t(x^i)+v^j_t(x^j)$, for each $x \in R^2_+$, and $\frac{dv^j_t(0)}{dx^j}=+\infty$, for each $t \in T^i$.

Assumption 4 in Busetto et al. (2018) can then be restated in the present context as follows.

Assumption 4

Commodities 1 and 2 and commodities 2 and 1 stand in relation C.

We proceed now through the steps which lead to the definition of a partial monopoly equilibrium à la Forchheimer.

It is straightforward to verify that Definition 1 and Propositions 1, 2, 3, and 4 also hold, mutatis mutandis, under Assumptions $1^{\prime }$, 2, and 3. In particular, we have that, given a strategy $e \in \textbf{E}(m)$, if $\textbf{w}^i(m)>0$ and $e_{ij}=0$, $i \ne j$, then, the pair $(p,\textbf{x})$, consisting of a price vector p satisfying (1) and an allocation $\textbf{x}$ such that $\textbf{x}(t)=\textbf{x}^0(t,p)$, for each $t \in T_0$, is a Walras equilibrium of the exchange economy determined by the atomless part under Assumption $1^{\prime }$.

The following proposition replaces Proposition 5 in Sect. 2 within the partial monopoly equilibrium framework.

Proposition 13

Under Assumptions $1^{\prime }$, 2, 3, and $4^{\prime }$, given a strategy $e \in \textbf{E}(m)$, there exists a market clearing price vector $p \in \Delta {\setminus } \partial \Delta$.

We denote by $\phi (\cdot )$ a correspondence which associates, with each strategy $e \in \textbf{E}(m)$, the set of price vectors $p \in \Delta {\setminus } \partial \Delta$ satisfying (1). A price selection $p(\cdot )$ is a function which associates, with each strategy selection $e \in \textbf{E}(m)$, a price vector $p \in \phi (e)$.

The main difference between correspondence $\pi$ and correspondence $\phi$ is that, in the former, prices are strictly positive if and only the monopolist supplies a positive amount of the commodity he holds whereas, in the latter, prices are strictly positive even when the monopolist supplies a null amount of his endowment. This is due to the fact that, when the monopolist is inactive, under Assumption $1^{\prime }$, the atomless part constitutes a self-contained exchange economy which, under the further Assumptions 2 and 3, admits a Walras equilibrium with strictly positive prices. Given a strategy $e \in \textbf{E}(m)$ and a price selection $p(\cdot )$, traders’ final holdings are determined according to the usual rule, described in Sect. 2, which applies in this case only for strictly positive prices, and they constitute an allocation, by the same argument used in the second part of the proof of Proposition 6.

We can now provide the definition of a partial monopoly equilibrium.

Definition 11

A strategy ${\check{e}} \in \textbf{E}(m)$ is a partial monopoly equilibrium, with respect to a price selection $p(\cdot )$, if

$$\begin{aligned} u_m(\textbf{x}(m,{\check{e}},p({\check{e}})) \ge u_m(\textbf{x}(m,e,p(e)), \end{aligned}$$

for each $e \in \textbf{E}(m)$.

A partial monopoly allocation is an allocation $\check{\textbf{x}}$ such that $\check{\textbf{x}}(m)=\textbf{x}(m,{\check{e}},p({\check{e}}))$ and $\check{\textbf{x}}(t)=\textbf{x}^0(t,p({\check{e}}))$, for each $t \in T_0$, where ${\check{e}}$ is a monopoly equilibrium, with respect to a price selection $p(\cdot )$.

The strategic market game associated with of a monopoly structure à la Forchheimer determined under Assumptions $1^{\prime }$, 2, 3, $4^{\prime }$ is a special case of the version of the Shapley window model analysed by Busetto et al. (2018). The following proposition shows the existence of a Cournot-Nash equilibrium for this strategic market game.

Proposition 14

Under Assumptions $1^{\prime }$, 2, 3, and $4^{\prime }$, there exists a Cournot-Nash equilibrium.

The main difference between the strategic market game associated with the model of monopoly developed in the previous sections in this paper and that associated with the bilateral exchange version of the partial monopoly à la Forchheimer sketched here, in a one-stage setting, consists in the fact that, in the former, a Cournot-Nash equilibrium does not exist, by Proposition 11, whereas, in the latter, it does exist, by Theorem 2 in Busetto et al. (2018). The crucial reason explaining this difference is the role the competitive fringe plays in making the market active, which prevents the atomless part it faces to supply a fixed amount of its endowment.

Busetto et al. (2018) proposed a respecification à la Cournot-Walras of the mixed version of the Shapley window model for an exchange economy with a finite number of commodities. In a one-stage setting, they obtained the negative result that the set of the Cournot-Walras equilibrium allocations of this respecification does not coincide with the set of the Cournot-Nash allocations of the mixed version of the original Shapley model. Indeed, the bilateral exchange version of the partial monopoly model introduced by Forchheimer (1908) can be viewed as a two-commodity monopoly version of the Cournot-Walras framework proposed by Busetto et al. (2008), where one commodity is held by the monopolist and a fringe of the atomless part whereas the other commodity is only held by the atomless part.

The following example, close to Example 2 in Busetto et al. (2018), confirms, with regards to the partial monopoly model, the negative result obtained, in a one-stage setting, by those authors within their more general framework.

Example 4

Consider the following specification of an exchange economy satisfying Assumptions $1^{\prime }$, 2, 3, and $4^{\prime }$. $T_0=[0,1]$, $T \setminus T_0=\{m\}$, $\mu (m)=1$, $\textbf{w}(m)=(1,0)$, $u_m(x)=ln x^1+ln x^2$, $T_0$ is taken with Lebesgue measure, $\textbf{w}(t)=(1,0)$, $u_t(x)=ln {x^{1}}+ln {x^{2}}$, for each $t \in [0,\frac{1}{2}]$, $\textbf{w}(t)=(0,1)$, $u_t(x)=ln {x^{1}}+{l} {nx^{2}}$, for each $t \in [\frac{1}{2},1]$. Then, there is a unique partial monopoly allocation which does not coincide with the unique Cournot-Nash allocation.

Proof

The unique partial monopoly equilibrium is the strategy ${\check{e}} \in \textbf{E}(m)$ such that ${\check{e}}=\frac{-1+\sqrt{5}}{4}$ and the unique partial monopoly allocation is $\check{\textbf{x}}(m)=(\frac{5-\sqrt{5}}{4},\frac{-1+\sqrt{5}}{4\sqrt{5}}$), $\check{\textbf{x}}(t)=(\frac{1}{2},\frac{1}{2\sqrt{5}})$, for each $t \in [0,\frac{1}{2}]$, and $\check{\textbf{x}}(t)=(\frac{\sqrt{5}}{2},\frac{1}{2})$, for each $t \in [\frac{1}{2},1]$. The unique Cournot-Nash equilibrium is the strategy selection $\hat{\textbf{b}}$ such that $\hat{\textbf{b}}_{12}(m)=\frac{1}{2}$, $\hat{\textbf{b}}_{12}(t)=\frac{1}{2}$, for each $t \in [0,\frac{1}{2}]$, $\hat{\textbf{b}}_{21}(t)=\frac{1}{2}$, for each $t \in [\frac{1}{2},1]$, and the unique Cournot-Nash allocation is $\hat{\textbf{x}}(m)=(\frac{1}{2},\frac{1}{6})$, $\hat{\textbf{x}}(t)=(\frac{1}{2},\frac{1}{6})$, for each $t \in [0,\frac{1}{2}]$, and $\hat{\textbf{x}}(t)=(\frac{3}{2},\frac{1}{2})$, for each $t \in [\frac{1}{2},1]$. Hence, there is a unique partial monopoly allocation which does not coincide with the unique Cournot-Nash allocation. $\square$

6 An game theoretical foundation of partial monopoly à la Forchheimer

Their negative result in a one-stage setting led Busetto et al. (2008) to propose a further reformulation of the Shapley model as a two-stage game. By using it, they could give a foundation of their Cournot-Walras equilibrium concept: they showed that the set of the Cournot-Walras equilibrium allocations coincides with the set of the Markov perfect equilibrium allocations of the two-stage reformulation of the Shapley model.

Our negative result in a one-stage setting, provided by Example 4, leads us to propose a two-stage foundation of the partial monopoly model which is the analogous, in the new context, of that proposed by Busetto et al. (2008). We replace their notion of Markov perfect equilibrium with that of subgame perfect equilibrium since we have only one trader, the monopolist, moving in stage 0. It is immediate to verify that the two-stage game suitable to found the partial monopoly equilibrium can be obtained by adapting the two-stage reformulation of our monopoly model, developed in Sect. 4, consistently with the fundamental assumptions which characterize Forchheimer’s approach. This way, also the associated subgame perfect equilibrium can be defined. In order to distinguish this subgame prefect equilibrium from the previous one, we call it fully active, since the equilibrium strategy profile determines, through aggregation, irreducible matrices for all subgames.

Definition 12

A strategy profile $\textbf{s}^{**}$ such that $\bar{\textbf{A}}(\textbf{s}^{**}|\textbf{h}^1)$ is irreducible, for each $\textbf{h}^1 \in H^1$, is a fully active subgame perfect equilibrium if

$$\begin{aligned} u_t(\textbf{x}(t,\textbf{h}^2(\textbf{s}^{**})(t),p(\textbf{h}^2(\textbf{s}^{**})))) \ge u_t(\textbf{x}(t,\textbf{h}^2(\textbf{s}^{**} \setminus s(t))(t),p(\textbf{h}^2(\textbf{s}^{**} \setminus s(t))))), \end{aligned}$$

for each s(t) and for each $t \in T$, and

$$\begin{aligned}{} & {} u_t(\textbf{x}(t,\textbf{h}^2(\textbf{s}^{**}|\textbf{h}^1)(t),p(\textbf{h}^2(\textbf{s}^{**}|\textbf{h}^1))))\\{} & {} \ge u_t(\textbf{x}(t,\textbf{h}^2(\textbf{s}^{**}|\textbf{h}^1 \setminus s|\textbf{h}^1)(t))(t),p(\textbf{h}^2(\textbf{s}^{**}|\textbf{h}^1 \setminus s|\textbf{h}^1)(t))))), \end{aligned}$$

for each $\textbf{h}^1 \in H^1$, for each $s|\textbf{h}^1(t)$, and for each $t \in T$.

A fully active subgame perfect allocation is an allocation $\textbf{x}^{**}$ such that $\textbf{x}(t,\textbf{h}^2(\textbf{s}^{**})(t),p(\textbf{h}^2(\textbf{s}^{**})))$, for each $t \in T$, where $\textbf{s}^{**}$ is a fully active subgame perfect equilibrium.

The following proposition shows the equivalence between the set of partial monopoly allocations and the set of fully active subgame perfect allocations for our two-stage game.

Proposition 15

Under Assumptions $1^{\prime }$, 2, 3, and $4^{\prime }$, the set of partial monopoly allocations coincides with the set of fully active subgame perfect allocations.

The main difference between the two-stage strategic market games associated, respectively, with the model of monopoly introduced in Sect. 2 and the bilateral exchange version of the partial monopoly à la Forchheimer, sketched in Sect. 5, consists in the fact that, in the former, exchange takes place in the second stage if and only if the monopolist supplies a positive amount of the commodity he holds whereas, in the latter, the competitive fringe prevents the market to be inactive even when the monopolist supplies a null amount of the commodity he holds. This happens—as we have already observed—because, in the partial monopoly model, when the monopolist is inactive, the atomless part constitutes a self-contained exchange economy which admits a Walras equilibrium. This equilibrium, in turn, coincides, under Assumption $4^{\prime }$, with an active Cournot-Nash equilibrium, by Theorem 2 in Codognato and Ghosal (2000). This fundamental difference is embodied in the definitions of subgame perfect equilibrium and fully active subgame perfect equilibrium we have used to provide a foundation of, respectively, the notion of monopoly equilibrium and that of partial monopoly equilibrium, and in the proof of the two propositions—respectively, Propositions 12 and 15— which state this foundation. In particular, in the proof of Proposition 12, the case where the monopolist supplies a null amount of the commodity he holds must be explicitly considered as, in this case, prices are null, exchange does not take place, and the strategy profiles determine, through aggregation, matrices which are not irreducible. In contrast, in the proof of Proposition 15, this case does not need to be considered.

The comparison developed above between the monopoly and the partial monopoly solutions and their game theoretical counterparts permits us to highlight, in general terms, the role of the competitive fringe in activating the market. Example 4 supports our analysis exhibiting a case where a partial monopoly equilibrium exists when a commodity is held both by the monopolist and a fraction of the atomless part but, as it is straightforward to verify, a monopoly equilibrium does not exist anymore when the competitive fringe evaporates. We leave for further research a more detailed investigation on the relationship between monopoly and partial monopoly in the case where the weight of the competitive fringe becomes infinitesimal.

7 Conclusion

In this paper, we have provided both a general economic foundation and a game theoretical foundation of the quantity-setting monopoly solution in bilateral exchange which, to the best of our knowledge, were gaps in the literature on monopoly in general equilibrium.

We leave for future research addressing the problem of a price-setting monopolist, in the same bilateral framework as used in this paper. This goal could lead to a game theoretical foundation of a monopoly solution of this type in a two-stage setup, as suggested by Sadanand (1988).

Shitovitz (1973), in his Example 1, showed that, in the mixed version of a monopolistic two-commodity exchange economy, the set of allocations in the core does not coincide with the set of Walrasian allocations. This example raised the question whether the core solution to monopolistic market games is “advantageous” or “disadvantageous” for the monopolist (see Aumann 1973; Drèze et al. 1977; Greenberg and Shitovitz 1977, among others). The same issue could be analysed using our monopoly equilibrium solution.

Appendix

Proof of Proposition 1

Let $p \in R^l_{++}$. Then, the graph of the correspondence $\textbf{X}(\cdot ,p)$, $\{(t,x){:}x \in \textbf{X}(\cdot ,p)\}$, is a subset of ${{{\mathcal {T}}}} \bigotimes {{{\mathcal {B}}}}$, by the same argument as that used by Busetto et al. (2011) (see the proof of their Proposition). But then, by the measurable choice theorem in Aumann (1969), there exists a measurable function $\bar{\textbf{x}}(\cdot ,p)$ such that, $\bar{\textbf{x}}(t,p) \in \textbf{X}(t,p)$, for each $t \in T_0$, which is also integrable as $\bar{\textbf{x}}^j(t,p) \le \frac{\sum _{i=1}^lp^i\textbf{w}^i(t)}{p^j},\,j=1,2$, for each $t \in T_0$. We must have that $\textbf{x}^0(\cdot ,p)=\bar{\textbf{x}}(\cdot ,p)$ as $\textbf{X}^0(t,p)=\{\textbf{x}^0(t,p)\}$, for each $t \in T_0$. Hence, the function $\textbf{x}^0(\cdot ,p)$ is integrable and $\int _{T_0}{} \textbf{X}^0(t,p)\,d\mu =\int _{T_0}{} \textbf{x}^0(t,p)\,d\mu$, for each $p \in R^2_{++}$. $\square$

Proof of Proposition 2

It straightforwardly follows from homogeneity of degree zero of the function $\textbf{x}^0(t,\cdot )$, for each $t \in T_0$, and from (1). $\square$

Proof of Proposition 3

Let a strategy $e \in \textbf{E}(m)$ be given. Suppose, without loss of generality, that $\textbf{w}^1(m)>0$. Let $p \in \Delta {\setminus } \partial \Delta$ be a price vector. Suppose that p is market clearing for $j=1$. Then, (1) reduces to

$$\begin{aligned} \int _{T_0}{} \textbf{x}^{01}(t,p)\,d\mu =e_{12}\mu (m). \end{aligned}$$

We have that

$$\begin{aligned} p^1\int _{T_0}{} \textbf{x}^{01}(t,p)\,d\mu +p^2\int _{T_0}\textbf{x}^{02}\,d\mu (t,p)=p^2\int _{T_0}{} \textbf{w}^2(t)\,d\mu , \end{aligned}$$

as $p^1\textbf{x}^{01}(t,p)+p^2\textbf{x}^{02}(t,p)=p^2\textbf{w}^2(t)$, by Assumption 2, for each $t \in T_0$. Then, we have that

$$\begin{aligned} \int _{T_0}\textbf{x}^{02}\,d\mu (t,p)+e_{12}\mu (m)\frac{p^1}{p^2}=\int _{T_0}\textbf{w}^2(t)\,d\mu . \end{aligned}$$

Therefore, p is market clearing for $j=2$. Suppose now that (1) is satisfied for $j=2$. Then, (1) reduces to

$$\begin{aligned} \int _{T_0}{} \textbf{x}^{02}\,d\mu (t,p)+e_{12}\mu (m)\frac{p^1}{p^2}=\int _{T_0}{} \textbf{w}^2(t)\,d\mu . \end{aligned}$$

But then, we have that

$$\begin{aligned} p^2\int _{T_0}\textbf{x}^{02}\,d\mu (t,p)+p^1e_{12}\mu (m)=p^2\int _{T_0}{} \textbf{w}^2(t)\,d\mu . \end{aligned}$$

On the other hand, we know from the previous argument that

$$\begin{aligned} p^1\int _{T_0}{} \textbf{x}^{01}(t,p)\,d\mu +p^2\int _{T_0}\textbf{x}^{02}\,d\mu (t,p)=p^2\int _{T_0}{} \textbf{w}^2(t)\,d\mu . \end{aligned}$$

Then, we obtain that

$$\begin{aligned} \int _{T_0}{} \textbf{x}^{01}(t,p)=e_{12}\mu (m). \end{aligned}$$

Therefore, p is market clearing for $j=1$. Hence, $p \in \Delta {\setminus } \partial \Delta$ is market clearing for $j=1$ if and only if it is market clearing for $j=2$. $\square$

Proof of Proposition 4

According to Debreu (1982), we let $|x|=\sum _{i=1}^2|x^i|$, for each $x \in R^2_+$, and $d[0,V]=\inf _{x \in V}|x|$, for each $V \subset R^2_+$. Let $\{p^n\}$ be a sequence of normalized price vectors such that $p^n \in \Delta \setminus \partial \Delta$, for each $n=1,2,\ldots$, which converges to a normalized price vector ${{\bar{p}}}$. Suppose, without loss of generality, that ${{\bar{p}}}^1=0$ and $\textbf{w}^1(m)>0$. Then, we have that ${{\bar{p}}}^2=1$. But then, the sequence $\{d[0,\textbf{X}^0(t,p^n)]\}$ diverges to $+\infty$ as ${{\bar{p}}}^2\textbf{w}^2(t)>0$, for each $t \in T_0$, by Lemma 4 in Debreu (1982, p. 721). Therefore, the sequence $\{d[0,\int _{T_0}\textbf{X}^0(t,p^n)\,d\mu ]\}$ diverges to $+\infty$, by the argument used in the proof of Property (iv) in Debreu (1982, p. 728). This implies that the sequence $\sum _{i=1}^2\{\int _{T_0}\textbf{x}^{0i}(t,p^n)\,d\mu \}$ diverges to $+\infty$ as $\int _{T_0}\textbf{X}^0(t,p)\,d\mu =\int _{T_0}{} \textbf{x}^0(t,p)\,d\mu$, for each $p \in \Delta {\setminus } \partial \Delta$, by Proposition 1. Suppose that the sequence $\{\int _{T_0}{} \textbf{x}^{02}(t,p^n)\,d\mu \}$ diverges to $+\infty$. Then, there exists an $n_0$ such that $\int _{T_0}\textbf{x}^{02}(t,p^n)\,d\mu >\int _{T_0}{} \textbf{w}^2(t)\,d\mu$, for each $n \ge n_0$. But we have that $\textbf{x}^{02}(t,p) \le \textbf{w}^2(t)$, for each $t \in T_0$ and for each $p \in \Delta {\setminus } \partial \Delta$, a contradiction. Then, the sequence $\{\int _{T_0}\textbf{x}^{01}(t,p^n)\,d\mu \}$ diverges to $+\infty$. Hence, the sequence $\{\int _{T_0}{} \textbf{x}^{0i}(t,p^n)\,d\mu \}$ diverges to $+\infty$ whenever ${{\bar{p}}}^i=0$ and $\textbf{w}^i(m)>0$. $\square$

Proof of Proposition 5

Suppose, without loss of generality, that $\textbf{w}^1(m)>0$ and let $e \in \textbf{E}(m)$ be a strategy. Suppose that there exists a market clearing price vector $p \in \Delta {\setminus } \partial \Delta$ and that the matrix E is not triangular. Then, it must be that $e_{12}=0$. But then, we have that $\int _{T^2}\textbf{x}^{01}(t,p)\,d\mu =0$ as $\mu (T^2)>0$, by (1). Consider a trader $\tau \in T^2$. We have that $\frac{\partial u_{\tau }(\textbf{x}^0(\tau ,p))}{\partial x^1}=+\infty$ as 2 and 1 stand in the relation Q, by Assumption 4, and $\frac{\partial u_{\tau }(\textbf{x}^0(\tau ,p))}{\partial x^1} \le \lambda p^1$, by the necessary conditions of the Kuhn-Tucker theorem. Moreover, it must be that $\textbf{x}^{02}(\tau ,p)=\textbf{w}^2(\tau )>0$ as $u_{\tau }(\cdot )$ is strongly monotone, by Assumption 2, and $p\textbf{w}(\tau )>0$. Then, $\frac{\partial u_{\tau }(\textbf{x}^0(\tau ,p))}{\partial x^2}=\lambda p^2$, by the necessary conditions of the Kuhn–Tucker theorem. But then, it must be that $\frac{\partial u_{\tau }(\hat{\textbf{x}}(\tau ))}{\partial x^2}=+\infty$ as $\lambda =+\infty$, contradicting the assumption that $u_{\tau }(\cdot )$ is continuously differentiable. Therefore, the matrix E must be triangular. Suppose now that E is triangular. Then, it must be that $e_{12}>0$. Let $\{p^n\}$ be a sequence of normalized price vectors such that $p^n \in \Delta \setminus \partial \Delta$, for each $n=1,2,\ldots$, which converges to a normalized price vector ${{\bar{p}}}$ such that ${{\bar{p}}}^1=0$. Then, the sequence $\{\int _{T_0}{} \textbf{x}^{01}(t,p^n)\,d\mu \}$ diverges to $+\infty$, by Proposition 4. But then, there exists an $n_0$ such that $\int _{T_0}{} \textbf{x}^{01}(t,p^n)\,d\mu >e_{12}\mu (m)$, for each $n \ge n_0$. Therefore, we have that $\int _{T_0}\textbf{x}^{01}(t,p^{n_0})\,d\mu >e_{12}\mu (m)$. Let $q \in \Delta {\setminus } \partial \Delta$ be a price vector such that $\frac{q^2\int _{T_0}{} \textbf{w}^2(t)\,d\mu }{q^1}=e_{12}\mu (m)$. Consider first the case where $\int _{T_0}\textbf{x}^{01}(t,q)\,d\mu =e_{12}\mu (m)$. Then, q is market clearing as it is market clearing for $j=1$, by Proposition 3. Consider now the case where $\int _{T_0}{} \textbf{x}^{01}(t,q)\,d\mu \ne e_{12}\mu (m)$. Then, it must be that $\int _{T_0}\textbf{x}^{01}(t,q)\,d\mu <e_{12}\mu (m)$ as $\textbf{x}^{01}(t,q) \le \frac{q^2\textbf{w}^2(t)}{q^1}$, for each $t \in T_0$. But then, we have that $\int _{T_0}\textbf{x}^{01}(t,q)\,d\mu<e_{12}\mu (m)<\int _{T_0}\textbf{x}^{01}(t,p^{n_0})\,d\mu$. Let $O \subset \Delta {\setminus } \partial \Delta$ be a compact and convex set which contains $p^{n_0}$ and q. Then, the correspondence $\int _{T_0}{} \textbf{X}^0(t,\cdot )\,d\mu$ is upper hemicontinuous on O, by the argument used in the proof of Property (ii) in Debreu (1982, p. 727). But then, the function $\{\int _{T_0}{} \textbf{x}^{01}(t,\cdot )\,d\mu \}$ is continuous on O as $\int _{T_0}{} \textbf{X}^0(t,p)\,d\mu =\int _{T_0}{} \textbf{x}^0(t,p)\,d\mu$, for each $p \in \Delta \setminus \partial \Delta$, by Proposition 1. Therefore, there is a price vector $\dot{p} \in \Delta {\setminus } \partial \Delta$ such that $\int _{T_0}{} \textbf{x}^{01}(t,\dot{p})\,d\mu =e_{12}\mu (m)$, by the intermediate value theorem. Then, $\dot{p}$ is market clearing as it is market clearing for $j=1$, by Proposition 3. Hence, given a strategy $e \in \textbf{E}(m)$, there exists a market clearing price vector $p \in \Delta {\setminus } \partial \Delta$ if and only if the matrix E is triangular. $\square$

Proof of Proposition 6

Let a price selection $p(\cdot )$ and a strategy $e \in \textbf{E}(m)$ be given. Suppose that E is not triangular. Then, we have that $\textbf{x}(m)=\textbf{x}(m,e,p(e))=\textbf{w}(m)$ and $\textbf{x}(t)=\textbf{x}(t,p(e))=\textbf{w}(t)$, for each $t \in T_0$ as $p(e)=0$. Suppose that E is triangular. Then, we have that

$$\begin{aligned}{} & {} \int _T\textbf{x}^j(t)\,d\mu =(\textbf{w}^j(m)-\sum _{i=1}^2{e}_{ji}+\sum _{i=1}^2{e}_{ij}\frac{p^i}{p^j})\mu (m)+\int _{T_0}\textbf{x}^{0j}(t,p)\,d\mu \\ {}{} & {} =\int _T\textbf{w}^j(t)\,d\mu , \end{aligned}$$

$j=1,2$, as p(e) is market clearing. Hence, given a price selection $p(\cdot )$ and a strategy $e \in \textbf{E}(m)$, the assignment $\textbf{x}(m)=\textbf{x}(m,e,p(e))$, $\textbf{x}(t)=\textbf{x}(t,p(e))$, for each $t \in T_0$, is an allocation. $\square$

Proof of Proposition 7

Let $\textbf{w}^i(m)>0$. Suppose that $\int _{T_0}\textbf{x}^{0i}(t,p)\,d\mu =0$, for some $p \in \Delta {\setminus } \partial \Delta$. Then, we have that $\int _{T^i}{} \textbf{x}^{0i}(t,p)\,d\mu =0$ as $\mu (T^i)>0$ and the necessary Kuhn-Tucker conditions lead, mutatis mutandis, to the same contradiction as in the proof of Proposition 5. But then, we have that $\int _{T_0}\textbf{x}^{0i}(t,p)\,d\mu >0$, for each $p \in \Delta {\setminus } \partial \Delta$. Therefore, the function $\int _{T_0}\textbf{x}^{0i}(t,\cdot )\,d\mu$ is restricted to the codomain $R_{++}$. For each $x \in R_{++}$, there exists at least one $p \in \Delta {\setminus } \partial \Delta$ such that $x=\int _{T_0}\textbf{x}^{0i}(t,p)\,d\mu$, by the same argument used in the proof of Proposition 5. Then, the function $\int _{T_0}\textbf{x}^{0i}(t,\cdot )\,d\mu$ is onto as its range coincides with its codomain. Therefore, the function $\int _{T_0}\textbf{x}^{0i}(t,\cdot )\,d\mu$ is invertible if and only if it is one-to-one. Hence, the function $\int _{T_0}\textbf{x}^{0i}(t,\cdot )\,d\mu$ is invertible if and only, for each $x \in R_{++}$, there is a unique $p \in \Delta \setminus \partial \Delta$ such that $x=\int _{T_0}{} \textbf{x}^{0i}(t,p)\,d\mu$. $\square$

Proof of Proposition 8

Suppose that $\textbf{w}^i(m)>0$ and that the function $\int _{T_0}\textbf{x}^{0i}(t,\cdot )\,d\mu$ is invertible. Let $\mathring{p}(e)$ be a function which associates, with each strategy $e \in \textbf{E}(m)$, the price vector $p=p^{0i}(e_{ij}\mu (m))$, if E is triangular, and is equal to $\{0\}$, otherwise. Then, $\mathring{p}(\cdot )$ is the unique price selection as $\pi (e)=\{\mathring{p}(e)\}$, for each $e \in \textbf{E}(m)$. $\square$

Proof of Proposition 9

Suppose that $\textbf{w}^i(m)>0$ and that the function $\int _{T_0}\textbf{x}^{0i}(t,\cdot )\,d\mu$ is invertible. Suppose that ${{\bar{x}}} \in \{x \in R^2_+{:} x^j=h(x^i)\}$. Then, there is a unique price vector ${{\bar{p}}}=p^{0i}({{\bar{x}}}^i)$ such that ${{\bar{x}}}^i=\int _{T_0}{} \textbf{x}^{0i}(t,{{\bar{p}}})\,d\mu$, as the function $\int _{T_0}{} \textbf{x}^{0i}(t,\cdot )\,d\mu$ is invertible. We have that

$$\begin{aligned} {{\bar{p}}}^i\int _{T_0}\textbf{x}^{0i}(t,{{\bar{p}}})\,d\mu +{{\bar{p}}}^j\int _{T_0}\textbf{x}^{0j}(t,{{\bar{p}}})\,d\mu =p^i\int _{T_0}\textbf{w}^i(t)\,d\mu +p^j\int _{T_0}{} \textbf{w}^j(t)\,d\mu , \end{aligned}$$

by Walras’ law. Then, it must be that ${{\bar{x}}}^j=\int _{T_0}\textbf{x}^{0j}(t,{{\bar{p}}})\,d\mu$, where ${{\bar{x}}}^j=h({{\bar{x}}}^i)$. But then, ${{\bar{x}}} \in \{x \in R^2{:}x=\int _{T_0}{} \textbf{x}^0(t,p)\,d\mu \text { for some } p \in \Delta {\setminus } \partial \Delta \}$. Therefore, $\{x \in R^2_+{:} x^j=h(x^i)\} \subset \{x \in R^2_+{:}x=\int _{T_0}{\mathbf{x}}^0(t,p)\,d\mu$ for some $p\in \Delta {\setminus } \partial \Delta \}$. Suppose now that ${{\bar{x}}} \in \{x \in R^2{:}x=\int _{T_0}{} \textbf{x}^0(t,p)\,d\mu \text { for some } p \in \Delta {\setminus } \partial \Delta \}$. Let ${{\bar{p}}}$ be such that ${{\bar{x}}}=\int _{T_0}{} \textbf{x}^0(t,{{\bar{p}}})\,d\mu$. Then, we have that ${{\bar{p}}}=p^{0i}({{\bar{x}}}^i)$ as the function $\int _{T_0}{} \textbf{x}^{0i}(t,\cdot )\,d\mu$ is invertible. We have that

$$\begin{aligned} {{\bar{p}}}^i{{\bar{x}}}^i+{{\bar{p}}}^j{{\bar{x}}}^j={{\bar{p}}}^i\int _{T_0}\textbf{w}^i(t)+p^j\int _{T_0}{} \textbf{w}^j(t), \end{aligned}$$

by Walras’ law. Then, we have that ${{\bar{x}}}^j=h({{\bar{x}}}^i)$. But then, ${{\bar{x}}} \in \{x \in R^2_+{:}x^j=h(x^i)\}$. Therefore, $\{x \in R^2_+{:}x=\int _{T_0}{} \textbf{x}^0(t,p)\,d\mu \text { for some }p \in \Delta {\setminus } \partial \Delta \} \subset \{x \in R^2_+{:}x^j=h(x^i)\}$. Hence, the graph of the function $h(\cdot )$, the set $\{x \in R^2_+{:}x^j=h(x^i)\}$, coincides with the set $\{x \in R^2_+{:}x=\int _{T_0}{} \textbf{x}^0(t,p)\,d\mu \text { for some } p \in \Delta {\setminus } \partial \Delta \}$, the offer curve of the atomless part. $\square$

Proof of Proposition 10

Suppose that $\textbf{w}^i(m)>0$, that the function $\int _{T_0}\textbf{x}^{0i}(t,\cdot )\,d\mu$ is invertible and that the function $p^{0i}(\cdot )$ is differentiable. Let ${{\tilde{e}}} \in \textbf{E}(m)$ be a monopoly equilibrium such that ${{\tilde{e}}}<\textbf{w}^i(m)$ and let $\tilde{\textbf{x}}$ be the corresponding monopoly allocation. Then, $\mathring{p}(\cdot )$, the inverse demand function of the monopolist, is differentiable and the necessary Kuhn-Tucker conditions imply that

$$\begin{aligned} -\frac{\partial u_m(\tilde{\textbf{x}}(m))}{\partial x^i}+\frac{\partial u_m(\tilde{\textbf{x}}(m))}{\partial x^j}\left( \frac{d\mathring{p}({{\tilde{e}}})}{de_{ij}}{{\tilde{e}}}_{ij}+\mathring{p}({{\tilde{e}}})\right) =0. \end{aligned}$$

Then, we have that

$$\begin{aligned} -\frac{\frac{\partial u_m(\tilde{\textbf{x}}(m))}{\partial x^i}}{\frac{\partial u_m(\tilde{\textbf{x}}(m))}{\partial x^j}}=-\left( \frac{d\mathring{p}({{\tilde{e}}})}{de_{ij}}{{\tilde{e}}}_{ij}+\mathring{p}({{\tilde{e}}})\right) . \end{aligned}$$

Moreover, we have that

$$\begin{aligned} h(x^i)=-p^{0i}(x^i)x^i+\int _{T_0}{} \textbf{w}^j(t)\,d\mu . \end{aligned}$$

Differentiating the function $h(\cdot )$, we obtain

$$\begin{aligned} \frac{dh(x^i)}{x^i}=-\left( \frac{dp^{0i}(x^i)}{dx^i}x^i+p^{0i}(x^i)\right) . \end{aligned}$$

At the monopoly allocation $\tilde{\textbf{x}}$, we have that

$$\begin{aligned} \frac{d\mathring{p}({{\tilde{e}}})}{de_{ij}}{{\tilde{e}}}_{ij}+\mathring{p}({{\tilde{e}}})=\frac{dp^{0i}(\int _{T_0}\tilde{\textbf{x}}^i(t)\,d\mu )}{dx^i}\int _{T_0}\tilde{\textbf{x}}^i(t)\,d\mu +p^{0i}(\int _{T_0}\tilde{\textbf{x}}^i(t)\,d\mu ), \end{aligned}$$

as $\frac{d\mathring{p}({{\tilde{e}}})}{de_{ij}}=\frac{dp^{0i}({{\tilde{e}}}_{ij}\mu (m))}{dx^i}\mu (m)$ and ${{\tilde{e}}}_{12}\mu (m)=\int _{T_0}{} \textbf{x}^{0i}(t, p({{\tilde{e}}}))\,d\mu$. Hence, we have that

$$\begin{aligned} -\frac{\frac{\partial u_m(\tilde{\textbf{x}}(m))}{\partial x^i}}{\frac{\partial u_m(\tilde{\textbf{x}}(m))}{\partial x^j}}=-\left( \frac{d\mathring{p}({{\tilde{e}}})}{de_{ij}}{{\tilde{e}}}_{ij}+\mathring{p}({{\tilde{e}}})\right) =\frac{dh(\int _{T_0}\tilde{\textbf{x}}^i(t)\,d\mu )}{dx^i}. \end{aligned}$$

$\square$

Proof of Proposition 11

Suppose, without loss of generality, that $\textbf{w}^1(m)>0$ and that $\hat{\textbf{b}}$ is a Cournot-Nash equilibrium. Then, we have that $\textbf{x}(m,\hat{\textbf{b}}(m),$ $p(\hat{\textbf{b}}))=(\textbf{w}^1(m)-\hat{\textbf{b}}_{12}(m),\bar{\hat{\textbf{b}}}_{21})$. Let $b^{\prime }(m)$ be a strategy such that $0<b^{\prime }_{12}(m)<{{{\hat{\textbf{b}}}}}_{12}(m)$. Then, we have that

$$\begin{aligned} u_m(\textbf{x}(m,{{{\hat{\textbf{b}}}}} \setminus b^{\prime }(m),p({ {{\hat{\textbf{b}}}}} \setminus b^{\prime }(m))))>u_m(\textbf{x}(m,{ {{\hat{\textbf{b}}}}}(m),p({{{\hat{\textbf{b}}}}}))), \end{aligned}$$

as $\textbf{x}(m,{{{\hat{\textbf{b}}}}} {\setminus } b^{\prime }(m),p({ {{\hat{\textbf{b}}}}} {\setminus } b^{\prime }(m)))=(\textbf{w}^i(m)-b^{\prime }_{12}(m)),\bar{\hat{\textbf{b}}}_{21})$ and $u_m(\cdot )$ is strongly monotone, by Assumption 2, a contradiction. Hence, there is no Cournot-Nash equilibrium. $\square$

Proof of Proposition 12

Suppose, without loss of generality, that $\textbf{w}^1(m)>0$. Let $\tilde{\textbf{x}}$ be a monopoly allocation. Then, we have that $\tilde{\textbf{x}}(m)=\textbf{x}(m,{{\tilde{e}}},p({{\tilde{e}}}))$ and $\tilde{\textbf{x}}(t)=\textbf{x}^0(t,p({{\tilde{e}}}))$, for each $t \in T_0$, where ${{\tilde{e}}}$ is a monopoly equilibrium, with respect to a price selection $p(\cdot )$. Consider, first, stage 1 of the game. Let $e \in \textbf{E}(m)$ be a strategy selection and let $\textbf{h}^1$ be a history at the beginning of stage 1 of the game such that $\textbf{h}^1(m)=e$. Suppose that E is triangular. Then, we have that $p(e) \gg 0$ and $p(e)\textbf{x}^0(t,p(e))=p(e)\textbf{w}^2(t)$, for each $t \in T_0$, by Assumption 2. But then, there exist $\lambda ^j(t) \ge 0$, $j=1,2$, $\sum _{j=1}^2\lambda ^j(t)=1$, such that

$$\begin{aligned} \textbf{x}^0(t,p(e))=\lambda ^j(t)\frac{p^2(e)\textbf{w}^2(t)}{p^j(e)}, \end{aligned}$$

$j=1,2$, for each $t \in T_0$, by Lemma 5 in Codognato and Ghosal (2000). Let ${\lambda }: T_0 \rightarrow R^2_+$ be a function such that ${\lambda }^j(t)={\lambda} ^j(t)$, $j=1,2$, for each $t \in T_0$. It is straightforward to show that the function $\textbf{w}^i(t){\lambda }^j(t)$, $i,j=1,2$, for each $t \in T_0$, is integrable on $T_0$. Let $\tilde{\textbf{s}}|\textbf{h}^1$ denote a strategy selection of the subgame represented by the stage 1 of the game such that $\textbf{a}^1(\tilde{\textbf{s}}|\textbf{h}^1)(m)=${“do nothing”} and $\textbf{a}^1_{ij}(\tilde{\textbf{s}}|\textbf{h}^1)(t)=\textbf{w}^i(t){\lambda }^j(t)$, $i,j=1,2$, for each $t \in T_0$. It is immediate to verify that $(\tilde{\textbf{s}}|\textbf{h}^1)(t) \in \textbf{A}^1(t)$, for each $t \in T$. Consider the matrix $\bar{\textbf{A}}(\tilde{\textbf{s}}|\textbf{h}^1)$. We have that

$$\begin{aligned} \bar{\textbf{a}}_{12}(\tilde{\textbf{s}}|\textbf{h}^1)=\textbf{a}^0_{12}(\tilde{\textbf{s}}|\textbf{h}^1)(m)\mu (m)+\int _{t \in T_0}{} \textbf{a}^1_{12}(\tilde{\textbf{s}}|\textbf{h}^1)(t)\,d\mu =e_{12}\mu (m)>0. \end{aligned}$$

By the same argument used in the proof of Proposition 5, Assumption 4 implies that $\textbf{x}^{01}(t,p(e))>0$, for each $t \in T^2$. Then, we have that ${\lambda }^1(t)>0$, for each $t \in T^2$. But then, we have that

$$\begin{aligned}{} & {} \bar{\textbf{a}}_{21}(\tilde{\textbf{s}}|\textbf{h}^1)=\textbf{a}^0_{21}(\tilde{\textbf{s}}|\textbf{h}^1)(m)\mu (m)+\int _{t \in T_0}{} \textbf{a}^1_{21}(\tilde{\textbf{s}}|\textbf{h}^1)(t)\,d\mu \\ {}{} & {} =\int _{t \in T_0}\textbf{w}^2(t){\lambda }^1(t)\,d\mu >0. \end{aligned}$$

Therefore, the matrix $\bar{\textbf{A}}(\tilde{\textbf{s}}|\textbf{h}^1)$ is irreducible. Then, from (1), we obtain that

$$\begin{aligned}{} \int _{T_0}\textbf{x}^1(t,p(e))\,d\mu =\int _{T_0}{\lambda }^1(t)\frac{p^2(e)\textbf{w}^2(t)}{p^1(e)}\,d\mu \\ {}{} =\int _{T_0}{} \textbf{a}^1_{21}(\tilde{\textbf{s}}|\textbf{h}^1)(t)\frac{p^2(e)}{p^1(e)}\,d\mu =e_{12}\mu (m). \end{aligned}$$

But then, it must be that $p(e)=p(\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1))$ as p(e) satisfies (3) and the matrix $\bar{\textbf{A}}(\tilde{\textbf{s}}|\textbf{h}^1)$ is irreducible. Therefore, it is straightforward to verify that

$$\begin{aligned} \textbf{x}(m,\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1)(m),p(\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1)))=\textbf{x}(m,e,p(e)) \end{aligned}$$

and

$$\begin{aligned} \textbf{x}(t,\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1)(t),p(\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1)))=\textbf{x}(t,p(e)), \end{aligned}$$

for each $t \in T_0$. It remains now to show that no trader $t \in T$, in stage 1 of the game, has an advantageous deviation from $\tilde{\textbf{s}}|\textbf{h}^1$. This is trivially true for m. Suppose that there exist a trader $\tau \in T_0$ and a strategy $s(\tau )$ such that

$$\begin{aligned}{} & {} u_{\tau }(\textbf{x}(\tau ,\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1 \setminus s|\textbf{h}^1(\tau ))(\tau ),p(\textbf{h}^2(\textbf{s}^{*}|\textbf{h}^1 \setminus s|\textbf{h}^1(\tau )))))\\ {}{} & {} > u_{\tau }(\textbf{x}(\tau ,\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1)(\tau ),p(\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1)))). \end{aligned}$$

It is straightforward to verify that Definition 8 implies that $p(\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1 {\setminus } s|\textbf{h}^1)(\tau )))=p(\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1))$. Then, we have that

$$\begin{aligned}{} & {} u_{\tau }(\textbf{x}(\tau ,\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1 \setminus s|\textbf{h}^1(\tau ))(\tau ),p(\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1))))\\{} & {} > u_{\tau }(\textbf{x}(\tau ,\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1)(\tau ),p(\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1))))=u_{\tau }(\textbf{x}(\tau ,p(e))). \end{aligned}$$

It is also immediate to verify that

$$\begin{aligned} p(\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1))\textbf{x}(\tau ,\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1 \setminus s|\textbf{h}^1(\tau ))(\tau ),p(\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1)))=p(\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1))\textbf{w}(\tau ). \end{aligned}$$

Then, we have that

$$\begin{aligned} u_{\tau }(\textbf{x}(\tau ,p(e)))>u_{\tau }(\textbf{x}(\tau ,\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1 \setminus s|\textbf{h}^1(\tau ))(\tau ),p(\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1)))), \end{aligned}$$

a contradiction. Therefore, it must be that

$$\begin{aligned}{} & {} u_t(\textbf{x}(t,\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1)(t),p(\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1))))\\ {}{} & {} \ge u_t(\textbf{x}(t,\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1 \setminus s|\textbf{h}^1(t))(t),p(\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1 \setminus s|\textbf{h}^1(t))))), \end{aligned}$$

for each $t \in T_0$.

Suppose that E is not triangular. Then, we have that $p(e)=0$. Let $\tilde{\textbf{s}}|\textbf{h}^1$ denote a strategy selection of the subgame represented by the stage 1 of the game such that $\textbf{a}^1(\tilde{\textbf{s}}|\textbf{h}^1)(m)= {\text {``do nothing''}}$ and $\textbf{a}^1_{ij}(\tilde{\textbf{s}}|\textbf{h}^1)(t)=0$, $i,j=1,2$, for each $t \in T_0$. It is immediate to verify that $(\tilde{\textbf{s}}|\textbf{h}^1)(t) \in \textbf{A}^1(t)$, for each $t \in T$ and that the matrix $\bar{\textbf{A}}(\tilde{\textbf{s}}|\textbf{h}^1)$ is not irreducible. Then, it must be that $p(e)=p(\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1)$. Therefore, we have that

$$\begin{aligned} \textbf{x}(m,\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1)(m),p(\tilde{\textbf{s}}|\textbf{h}^1))=\textbf{w}^1(m)=\textbf{x}(m,e,p(e)) \end{aligned}$$

and

$$\begin{aligned} \textbf{x}(t,\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1)(t),p((\tilde{\textbf{s}}|\textbf{h}^1)))=\textbf{w}^2(t)=\textbf{x}(t,p(e)), \end{aligned}$$

for each $t \in T_0$. It remains now to show that no trader $t \in T$, in stage 1 of the game, has an advantageous deviation from $\tilde{\textbf{s}}|\textbf{h}^1$. This is trivially true for m. Suppose that there exist a trader $\tau \in T_0$ and an strategy $s(\tau )$ such that

$$\begin{aligned}{} & {} u_{\tau }(\textbf{x}(\tau ,\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1 \setminus s|\textbf{h}^1(\tau ))(\tau ),p(\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1 \setminus s|\textbf{h}^1(\tau )))))\\ {}{} & {} > u_{\tau }(\textbf{x}(\tau ,\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1)(\tau ),p(\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1)))). \end{aligned}$$

Then, we have that

$$\begin{aligned}{} & {} \textbf{w}^2(\tau )=u_{\tau }(\textbf{x}(\tau ,\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1 \setminus s|\textbf{h}^1(\tau ))(\tau ),p(\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1))))\\{} & {} > u_{\tau }(\textbf{x}(\tau ,\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1)(\tau ),p(\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1))))=\textbf{w}^2(\tau ), \end{aligned}$$

as $p(\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1 {\setminus } s|\textbf{h}^1(\tau )))=p(\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1))=0$, a contradiction. Therefore, we conclude that $\bar{\textbf{A}}(\tilde{\textbf{s}}|\textbf{h}^1)$ is irreducible, for each $\textbf{h}^1 \in H^1$ such that $\textbf{h}^1(m)>0$, and

$$\begin{aligned}{} & {} u_t(\textbf{x}(t,\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1)(t),p(\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1))))\\ {}{} & {} \ge u_t(\textbf{x}(t,\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1 \setminus s|\textbf{h}^1(t))(t),p(\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1 \setminus s|\textbf{h}^1(t))))), \end{aligned}$$

for each $\textbf{h}^1 \in H^1$, for each $s|\textbf{h}^1(t)$, and for each $t \in T$. Consider now stages 0 and 1 of the game. Let $\tilde{\textbf{s}}$ be a strategy profile such that $\tilde{\textbf{s}}(m,\textbf{h}^0)={{\tilde{e}}}$ and $\tilde{\textbf{s}}(t,\textbf{h}^0)={\text {``do nothing''}}$, for each $t \in T_0$, and $\tilde{\textbf{s}}(t,\textbf{h}^1)=(\tilde{\textbf{s}}|\textbf{h}^1)(t)$, for each $\textbf{h}^1 \in H^1$, and for each $t \in T$. Let $\tilde{\textbf{h}}^1$ be such that $\tilde{\textbf{h}}^1(m)={{\tilde{e}}}$. We have that $\textbf{h}^2(\tilde{\textbf{s}})=\textbf{h}^2(\tilde{\textbf{s}}|\tilde{\textbf{h}}^1)$ as $\textbf{a}^0(\tilde{\textbf{s}})=\tilde{\textbf{s}}^0(\cdot ,\textbf{h}^0)=\tilde{\textbf{h}}^1=\textbf{a}^0(\tilde{\textbf{s}}|\tilde{\textbf{h}}^1)$ and $\textbf{a}^1(\tilde{\textbf{s}})=\tilde{\textbf{s}}^1(\cdot ,\tilde{\textbf{h}}^1)=\tilde{\textbf{s}}|\tilde{\textbf{h}}^1=\textbf{a}^1(\tilde{\textbf{s}}|\tilde{\textbf{h}}^1)$. Then, it must be that $p({{\tilde{e}}})=p(\textbf{h}^2(\tilde{\textbf{s}}|\tilde{\textbf{h}}^1))=p(\textbf{h}^2(\tilde{\textbf{s}}))$. But then, it is straightforward to verify that

$$\begin{aligned} \textbf{x}(m,\textbf{h}^2(\tilde{\textbf{s}})(m),p(\textbf{h}^2(\tilde{\textbf{s}})))=\textbf{x}(m,{{\tilde{e}}},p({{\tilde{e}}})) \end{aligned}$$

and

$$\begin{aligned} \textbf{x}(t,\textbf{h}^2(\tilde{\textbf{s}}(t)),p(\textbf{h}^2(\tilde{\textbf{s}})))=\textbf{x}(t,p({{\tilde{e}}})), \end{aligned}$$

for each $t \in T_0$. Suppose that there exists a strategy s(m) of the monopolist such that

$$\begin{aligned} u_m(\textbf{x}(m,\textbf{h}^2(\tilde{\textbf{s}} \setminus s(m))(m),p(\textbf{h}^2(\tilde{\textbf{s}} \setminus s(m)))))>u_m(\textbf{x}(m,\textbf{h}^2(\tilde{\textbf{s}})(m),p(\textbf{h}^2(\tilde{\textbf{s}})))). \end{aligned}$$

Let $e=\tilde{\textbf{s}}^0 \setminus s(m,\textbf{h}^0)(m)$. Then, we have that $p(e)=p(\textbf{h}^2(\tilde{\textbf{s}}\setminus s(m)))$ by the same argument used before. But then, we have that

$$\begin{aligned} \textbf{x}(m,\textbf{h}^2(\tilde{\textbf{s}}\setminus s(m))(m),p(\textbf{h}^2(\tilde{\textbf{s}}\setminus s(m))))=\textbf{x}(m,e,p(e)). \end{aligned}$$

Therefore, it must be that

$$\begin{aligned}{} & {} u_m\textbf{x}(m,e,p(e))=u_m(\textbf{x}(m,\textbf{h}^2(\tilde{\textbf{s}} \setminus s(m))(m),p(\textbf{h}^2(\tilde{\textbf{s}} \setminus s(m)))))\\ {}{} & {} >u_m(\textbf{x}(m,\textbf{h}^2(\tilde{\textbf{s}})(m),p(\textbf{h}^2(\tilde{\textbf{s}}))))=\textbf{x}(m,{{\tilde{e}}},p({{\tilde{e}}})), \end{aligned}$$

a contradiction. Suppose that there exist a trader $\tau \in T_0$ and a strategy $s(\tau )$ such that

$$\begin{aligned}{} & {} u_{\tau }(\textbf{x}(\tau ,\textbf{h}^2(\tilde{\textbf{s}} \setminus s(\tau ))(\tau ),p(\textbf{h}^2(\tilde{\textbf{s}}\setminus s(\tau )))))\\{} & {} > u_{\tau }(\textbf{x}(\tau ,\textbf{h}^2(\tilde{\textbf{s}}(\tau ),p(\textbf{h}^2(\tilde{\textbf{s}})))). \end{aligned}$$

It is straightforward to verify that Definition 8 implies that $p(\textbf{h}^2(\tilde{\textbf{s}} {\setminus } s(\tau )))=p(\textbf{h}^2(\tilde{\textbf{s}}))$. Then, we have that

$$\begin{aligned}{} & {} u_{\tau }(\textbf{x}(\tau ,\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1 \setminus s|\tilde{\textbf{h}}^1(\tau ))(\tau ),p(\textbf{h}^2(\tilde{\textbf{s}}|\tilde{\textbf{h}}^1 \setminus s|\tilde{\textbf{h}}^1(\tau )))))\\ {}{} & {} =u_{\tau }(\textbf{x}(\tau ,\textbf{h}^2(\tilde{\textbf{s}} \setminus s(\tau ))(\tau ),p(\textbf{h}^2(\tilde{\textbf{s}}\setminus s(\tau )))))\\ {}{} & {} > u_{\tau }(\textbf{x}(\tau ,\textbf{h}^2(\tilde{\textbf{s}}(\tau ),p(\textbf{h}^2(\tilde{\textbf{s}}))))\\ {}{} & {} =u_{\tau }(\textbf{x}(\tau ,\textbf{h}^2(\tilde{\textbf{s}}|\tilde{\textbf{h}}^1)(\tau ),p(\textbf{h}^2(\tilde{\textbf{s}}|\tilde{\textbf{h}}^1)))), \end{aligned}$$

a contradiction. Thus the set of monopoly allocations is a subset of the set of subgame perfect allocations. Let $\textbf{x}^{*}$ be a subgame perfect allocation. Then, we have that $\textbf{x}^{*}=\textbf{x}(t,\textbf{h}^2(\textbf{s}^{*})(t),p(\textbf{h}^2(\textbf{s}^{*})))$, for each $t \in T$, where $\textbf{s}^{*}$ is a subgame perfect equilibrium. Let p(e) be a function which associates, with each strategy selection $e \in \textbf{E}(m)$, the price vector $p(\textbf{h}^2(\textbf{s}^{*}|\textbf{h}^1))$ corresponding to the history $\textbf{h}^1$ such that $\textbf{h}^1(m)=e$. Let $e \in \textbf{E}(m)$ be a strategy selection. Suppose that E is triangular. Then, it must that $p(e)=p(\textbf{h}^2(\textbf{s}^{*}|\textbf{h}^1)) \gg 0$ as the matrix $\bar{\textbf{A}}(\textbf{s}^{*}|\textbf{h}^1))$ is irreducible. Suppose that E is not triangular. Then, it must be that $p(e)=p(\textbf{h}^2(\textbf{s}^{*}|\textbf{h}^1))=0$ as the matrix $\bar{\textbf{A}}(\textbf{s}^{*}|\textbf{h}^1))$ is not irreducible. It is straightforward to verify that

$$\begin{aligned} \textbf{x}(m,e,p(e))=\textbf{x}(m,\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1)(m),p(\textbf{h}^2(\textbf{s}^{*}|\textbf{h}^1))), \end{aligned}$$

for each strategy selection $e \in \textbf{E}(m)$ and for each history $\textbf{h}^1$ such that $\textbf{h}^1(m)=e$. It is also straightforward to show that

$$\begin{aligned} u_t(\textbf{x}(t,\textbf{h}^2(\textbf{s}^{*}|\textbf{h}^1)(t),p(\textbf{h}^2(\textbf{s}^{*}|\textbf{h}^1))))>u_t(y), \end{aligned}$$

for all $y \in \{x \in R^2_+{:}p(\textbf{h}^2(\textbf{s}^{*}|\textbf{h}^1))x=p(\textbf{h}^2(\textbf{s}^{*}|\textbf{h}^1))\textbf{w}^2(t)\}$, for each $\textbf{h}^1 \in H^1$ such that $\textbf{h}^1(m)>0$ and for each $t \in T_0$, by the same argument used by Codognato and Ghosal (2000) in the proof of their Theorem 2, p. 49. Then, we have that

$$\begin{aligned} \textbf{x}(t,p(e))=\textbf{x}(t,\textbf{h}^2(\textbf{s}^{*}|\textbf{h}^1)(t),p(\textbf{h}^2(\textbf{s}^{*}|\textbf{h}^1))), \end{aligned}$$

for each strategy $e \in \textbf{E}(m)$, for each history $\textbf{h}^1$ such that $\textbf{h}^1(m)=e$, and for each $t \in T_0$. Let $e \in \textbf{E}(m)$ be a strategy selection such that E is triangular and let $\textbf{h}^1$ be a history such that $\textbf{h}^1(m)=e$. Then, we have that

$$\begin{aligned}{} & {} \int _{T_0}{} \textbf{x}^1(t,p(e))\,d\mu +\textbf{x}^1(m,e,p(e))=\int _{T_0}\textbf{x}^1(t,p(e))\,d\mu +e_{12}\\ {}{} & {} =\int _{T_0}{} \textbf{x}^1(t,\textbf{h}^2(\textbf{s}^{*}|\textbf{h}^1)(t),p(\textbf{h}^2(\textbf{s}^{*}|\textbf{h}^1)))\,d\mu \\ {}{} & {} + \textbf{x}^1(m,\textbf{h}^2(\tilde{\textbf{s}}|\textbf{h}^1)(m),p(\textbf{h}^2(\textbf{s}^{*}|\textbf{h}^1)))=\textbf{w}^1(m) \end{aligned}$$

as the assignment $\textbf{x}(t,\textbf{h}^2(\textbf{s}^{*}|\textbf{h}^1)(t),p(\textbf{h}^2(\textbf{s}^{*}|\textbf{h}^1)))$, for each $t \in T$, is an allocation. But then, p(e) satisfies (1) by Proposition  3. Therefore, p(e) is a price selection. Let $e^{*}$ be a strategy selection such that $e^{*}=\textbf{s}^{*}(m,\textbf{h}^0)$ and let ${\textbf{h}^1}^{*}$ be such that ${\textbf{h}^1}^{*}(m)=e^{*}$. We have that $\textbf{h}^2(\textbf{s}^{*})=\textbf{h}^2(\textbf{s}^{*}|{\textbf{h}^1}^{*})$ as $\textbf{a}^0(\textbf{s}^{*})={\textbf{s}^0}^{*}(\cdot ,\textbf{h}^0)={\textbf{h}^1}^{*}=\textbf{a}^0(\textbf{s}^{*}|{\textbf{h}^1}^{*})$ and $\textbf{a}^1(\textbf{s}^{*})={\textbf{s}^1}^{*}(\cdot ,{\textbf{h}^1}^{*})=\textbf{s}^{*}|{\textbf{h}^1}^{*}=\textbf{a}^1(\textbf{s}^{*}|{\textbf{h}^1}^{*})$. Then, it must be that $e^{*}>0$ as $\bar{\textbf{A}}(\textbf{s}^{*})$ is irreducible and $p(e^{*})=p(\textbf{h}^2(\textbf{s}^{*}|{\textbf{h}^1}^{*})=p(\textbf{h}^2(\textbf{s}^{*}))$. But then, it is straightforward to verify that

$$\begin{aligned} \textbf{x}(m,e^{*},p(e^{*}))=\textbf{x}(m,\textbf{h}^2(\textbf{s}^{*})(m),p(\textbf{h}^2(\textbf{s}^{*}))). \end{aligned}$$

Suppose that there exists a strategy $e \in \textbf{E}(m)$ such that

$$\begin{aligned} u_m(\textbf{x}(m,e,p(e)))>u_m(\textbf{x}(m,e^{*},p(e^{*}))). \end{aligned}$$

Let s(m) be a strategy of the monopolist such that ${\textbf{s}^0}^{*} {\setminus } s(m,\textbf{h}^0)(m)=e$. Then, we have that $p(e)=p(\textbf{h}^2(\textbf{s}^{*}\setminus s(m)))$ by the same argument used before. But then, we have that

$$\begin{aligned} \textbf{x}(m,e,p(e))=\textbf{x}(m,\textbf{h}^2(\textbf{s}^{*}\setminus s(m))(m),p(\textbf{h}^2(\textbf{s}^{*}\setminus s(m)))). \end{aligned}$$

Therefore, it must be that

$$\begin{aligned}{} & {} u_m(\textbf{x}(m,\textbf{h}^2(\textbf{s}^{*} \setminus s(m))(m),p(\textbf{h}^2(\textbf{s}^{*} \setminus s(m)))))=u_m\textbf{x}(m,e,p(e))\\ {}{} & {} >\textbf{x}(m,e^{*},p(e^{*}))=u_m(\textbf{x}(m,\textbf{h}^2(\textbf{s}^{*})(m),p(\textbf{h}^2(\textbf{s}^{*})))), \end{aligned}$$

a contradiction. Thus the set of subgame perfect allocations is a subset of the set of monopoly allocations. Hence, the set of monopoly allocations coincides with the set of subgame perfect allocations. $\square$

Proof of Proposition 13

Suppose, without loss of generality, that $\textbf{w}^1(m)>0$ and let $e \in \textbf{E}(m)$ be a strategy. Suppose that $e_{12}>0$. Let $\{p^n\}$ be a sequence of normalized price vectors such that $p^n \in \Delta {\setminus } \partial \Delta$, for each $n=1,2,\ldots$, which converges to a normalized price vector ${{\bar{p}}}$ such that ${{\bar{p}}}^1=0$. Then, the sequence $\{\int _{T_0}\textbf{x}^{01}(t,p^n)\,d\mu \}$ diverges to $+\infty$, by Proposition 4. But then, there exists an $n_0$ such that $\int _{T_0}\textbf{x}^{01}(t,p^n)\,d\mu >\int _S\textbf{w}^1(t)\,d\mu +e_{12}\mu (m)$, for each $n \ge n_0$. Therefore, we have that $\int _{T_0}\textbf{x}^{01}(t,p^{n_0})\,d\mu >\int _S\textbf{w}^1(t)\,d\mu +e_{12}\mu (m)$. Let $q \in \Delta \setminus \partial \Delta$ be a price vector such that $\frac{q^2\int _{T_0 {\setminus } S}\textbf{w}^2(t)\,d\mu }{q^1}=e_{12}\mu (m)$. Consider first the case where $\int _{T_0}{} \textbf{x}^{01}(t,q)\,d\mu =\int _S\textbf{w}^1(t)\,d\mu +e_{12}\mu (m)$. Then, q is market clearing as it is market clearing for $j=1$, by Proposition 3. Consider now the case where $\int _{T_0}{} \textbf{x}^{01}(t,q)\,d\mu \ne \int _S\textbf{w}^1(t)\,d\mu +e_{12}\mu (m)$. Then, it must be that $\int _{T_0}\textbf{x}^{01}(t,q)\,d\mu <\int _S\textbf{w}^1(t)\,d\mu +e_{12}\mu (m)$ as $\textbf{x}^{01}(t,q) \le \textbf{w}^1(t)$, for each $t \in S$, and $\textbf{x}^{01}(t,q) \le \frac{q^2\textbf{w}^2(t)}{q^1}$, for each $t \in {T_0 {\setminus } S}$. But then, we have that $\int _{T_0}\textbf{x}^{01}(t,q)\,d\mu<\int _S\textbf{w}^1(t)+e_{12}\mu (m)<\int _{T_0}\textbf{x}^{01}(t,p^{n_0})\,d\mu$. Therefore, by the argument used in the proof of Proposition 5, there exists a market clearing price vector $p \in \Delta \setminus \partial \Delta$. Suppose that $e_{12}=0$. Then, there exists a Walras equilibrium $(p,\textbf{x})$ of the exchange economy determined by the atomless part, by Theorem 9 in Debreu (1982). Therefore, there exists a market clearing price vector $p \in \Delta \setminus \partial \Delta$. $\square$

Proof of Proposition 14

It is a consequence of Theorem 2 in Busetto et al. (2018) as Assumptions $1^{\prime }$ and $4^{\prime }$ are special cases of their Assumptions 1 and 4 and 2 and 3 are the same. $\square$

Proof of Proposition 15

It follows from the proof of the Theorem in Busetto et al. (2008), replacing their Assumption 5 with Assumption $4^{\prime }$, and replacing the notion of Pseudo-Markov perfect equilibrium with that of fully active subgame perfect equilibrium. $\square$