1 Introduction

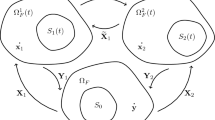
In this paper, we derive a priori estimates for solutions to an inviscid flow-structure interaction model. The system consists of the incompressible Euler equations defined in a channel with a free boundary at the top of the channel. The free boundary is elastic and deforms according to a scalar second-order linear equation satisfied by the transversal displacement. For simplicity, we consider the wave equation as an approximation for the dynamics of elasticity.

We consider the problem with periodic boundary conditions in the horizontal directions, and we reformulate the problem on a fixed domain using the Arbitrary Lagrangian Eulerian (ALE) variable, which is a change of coordinate involving the harmonic extension of the interface graph function. The interaction between the flow and the structure is captured mathematically through the pressure acting as a forcing term in the wave equation, while the normal velocity of the flow is matched with the normal component of the velocity of the interface through the kinematic condition.

The a priori estimates combine the energy estimate for the elastic displacement with the vorticity and pressure estimates. A characteristic feature of the system is that no stability condition is required for the local-in-time existence of solutions. The Rayleigh–Taylor stability condition is well-known to be necessary for problems involving the free boundary Euler equation with constant pressure at the free interface. However, in the setting we consider, the elasticity of the interface acts as a stabilizing and a regularizing agent, even though the wave equation does not incorporate any smoothing effects. Compared to the case of the plate equation considered in [21], the system considered here features an additional stabilization mechanism. Namely, the pressure term is more regular, and separate tangential estimates on the Euler equation that exploit the cancellation of the boundary term with energy estimates on the structural variable are unnecessary. Instead, the pressure forcing term in the wave equation can be estimated directly using the regularity property of the Robin-to-Dirichlet map, with the pressure term as the solution to a boundary value elliptic problem with Robin boundary data. The normal component of the fluid velocity can then be controlled directly using energy estimates on the wave equation. The full regularity of the fluid velocity can then be recovered using div-curl type estimates. We emphasize that the regularity of solutions considered in this paper are at the sharp level (\(H^{2.5+\delta }\) where \(\delta >0\) is arbitrarily small) expected for the Euler equation, leading to diffeomorphic flow maps of the changing domain.

Physically speaking, it is notable that second-order hyperbolic dynamics used to model the interface are also used to model the torsion or twist motions of an airfoil subject to airflow, though the model involved is slightly different since it involves the torsion angle as a variable instead of elastic deformation. Moreover, the incompressibility condition imposed on the flow is appropriate for flows at low Mach numbers. In our model, we choose the simple configuration where the flow happens along a horizontal channel with periodic boundary conditions, with the moving elastic interface at the top and the fixed wall at the bottom. However, this type of simplification is not essential and can be extended to the case of a general domain using flattening of the boundary and a partition of unity ([14]).

Inviscid flow-structure systems have been treated on fixed domains in many references; see [11, 22, 34]. These works consider linearized potential flow models coupled with nonlinear structural dynamics. The linearized potential flow equation is derived from the Euler equation under the irrotationality assumption. On the other hand, both compressible and incompressible viscous linearized flow models have also been considered in the literature; see [1, 2, 4, 6, 8,9,10, 12, 15, 18,19,20, 33] and [27, 32] for related references.

The well-posedness of free boundary models involving viscous fluid flow interacting with a lower-dimensional structure has also been studied extensively. The first instance of such treatments is due to Desjardins et al.  [7, 13] who obtained weak solutions to a system involving the incompressible Navier–Stokes equations coupled with a lower-dimensional interface modeled by a strongly damped linear plate equation. Models involving second-order equations for the lower-dimensional interface have been studied by Lequeurre [25, 26]. More recent works on the interaction between viscous flow and linear plate structural dynamics with and without damping have been considered in [3, 16, 17]. Other intricate systems involving the interaction between viscous flow and a linear or nonlinear Koiter shell have also been studied in [23, 24, 28,29,30,31, 35,36,37].

Our main result in this paper is the derivation of a priori bounds for the existence of local-in-time smooth solutions to the system, as well as uniqueness of solutions. We note that solutions to the system can be constructed using the scheme introduced in [21], whereby we solve the Euler system with variable coefficients and non-homogeneous boundary and divergence data. In [21], the inviscid flow interacts with a fourth-order plate equation, while here, the elastic body evolves according to a second-order wave equation. We present the a priori estimates and the uniqueness; the construction is omitted as it can be obtained by following [21].

The paper is organized as follows. In Sect. 2, we introduce the model and state the main results, Theorems 2.1 on existence and 2.3 on uniqueness. The a priori bounds for the existence are provided in Sect. 3. They couple the energy estimates for the wave equation with the tangential estimates for the Euler equations. An important feature of the estimates is that they are performed at a lower regularity level for the velocity. The velocity is then reconstructed using the pressure bounds and the estimates for the ALE vorticity. Finally, the proof of uniqueness is provided at the end of the section.

2 The model and the main results

Our goal is to address a flow-structure problem in which an incompressible fluid, modeled by the Euler equations

$$\begin{aligned} \begin{aligned}&u_t + u\cdot \nabla u + \nabla p = 0 \\ {}&\nabla \cdot u=0 \end{aligned} \end{aligned}$$
(2.1)

in an open bounded domain

$$\begin{aligned} \Omega (t) = \bigl \{ (x_1,x_2,x_3) \in {\mathbb {T}}^2\times {\mathbb {R}} : 0\le x_3 \le 1+w(x_1,x_2) \bigr \} , \end{aligned}$$
(2.2)

interacts with a membrane on a top boundary, which evolves according to the wave equation

$$\begin{aligned} w_{tt} - \Delta _2 w = p \text { on }{\Gamma _1\times [0,T]} , \end{aligned}$$
(2.3)

where \(\Gamma _j={{\mathbb {T}}}^2 \times \{j\}\), for \(j=0,1\) and p is evaluated at \((x_1,x_2,1+w(x_1,x_2))\). For simplicity (the general situation can be addressed using straightening of the boundary and a partition of unity, as in [14]), we assume the periodic boundary conditions on the side, i.e., the initial domain is represented by

$$\begin{aligned} \Omega (0)=\Omega ={{\mathbb {T}}}^2\times [0,1] , \end{aligned}$$

while the upper boundary of the domain \(\Omega (t)\) evolves according to

$$\begin{aligned} w_t + u_1 \partial _{1} w + u_2 \partial _{2} w = u_3 . \end{aligned}$$
(2.4)

The initial condition for the displacement w reads

$$\begin{aligned} (w,w_t)|_{t=0}=(0,w_1) , \end{aligned}$$
(2.5)

noting that the general nonzero w(0) can be considered using identical approach. On the bottom of the domain, we impose the impermeability condition for the velocity

$$\begin{aligned} u\cdot N = 0 \text { on }{\Gamma _0} , \end{aligned}$$

where N denotes the outward unit normal.

The same analysis and theorems also apply if we have a second-order nonlinear elastic equation (2.3) replaced by the equation of the form

$$\begin{aligned} w_{tt} - \textrm{div}(\sigma (w)) = p \text { on } {\Gamma _1\times [0,T]} , \end{aligned}$$

where \(\sigma (w)\) is a sufficiently smooth nonlinear tensor satisfying the coercivity condition

$$\begin{aligned} \int _{\Gamma _{1}} \sigma (w) \cdot \nabla _2 w \, dx \ge \kappa \Vert \nabla _2 w \Vert _{L^{2}(\Gamma _{1})}^{2} , \end{aligned}$$
(2.6)

for some \(\kappa >0\), where \(\nabla _2=(\partial _{1},\partial _{2})\).

2.1 ALE change of variable

It is essential to switch to the ALE variables as follows. Let \(\psi :\Omega \rightarrow {{\mathbb {R}}}\) be the harmonic extension of \(1+w\) to the domain \(\Omega =\Omega (0)\), which means that \(\psi \) is a solution of

$$\begin{aligned} \begin{aligned}&\Delta \psi = 0 \text { on } \Omega , \end{aligned} \end{aligned}$$

with the boundary conditions

$$\begin{aligned} \begin{aligned}&\psi (x_1,x_2,1,t)=1+w(x_1,x_2,t) \text { on } \Gamma _1 \\ {}&\psi (x_1,x_2,0,t)=0 \text { on } \Gamma _0 . \end{aligned} \end{aligned}$$

With \(\psi \) as above, we define the ALE variable \(\eta :\Omega \times [0,T]\rightarrow \Omega (t) \) with

$$\begin{aligned} \eta (x_1,x_2,x_3,t)=(x_1,x_2,\psi (x_1,x_2,x_3,t)) \mathrm{,\qquad {}} (x_1,x_2,x_3)\in \Omega . \end{aligned}$$

The inverse of the derivative of \(\nabla \eta \) is computed as

$$\begin{aligned} a = (\nabla \eta )^{-1} = \frac{1}{J} \begin{pmatrix} \partial _{3}\psi &{} \quad 0 &{} \quad 0 \\ 0 &{} \quad \partial _{3}\psi &{} \quad 0 \\ -\partial _{1}\psi &{} \quad -\partial _{2}\psi &{} \quad 1 \end{pmatrix} , \end{aligned}$$
(2.7)

where

$$\begin{aligned} J=\partial _{3}\psi \end{aligned}$$

is the Jacobian. Note that we have the Piola identity

$$\begin{aligned} \partial _{i}(J a_{ij})=0 \mathrm{,\qquad {}} j=1,2,3 , \end{aligned}$$
(2.8)

where, unless indicated otherwise (usually with a summation symbol), we use the summation convention on repeated indices. With

$$\begin{aligned} \begin{aligned}&v(x,t) = u(\eta (x,t),t) \\ {}&q(x,t) = p(\eta (x,t),t) \end{aligned} \end{aligned}$$

denoting the ALE velocity and pressure, the Euler equations (2.1) take the form

$$\begin{aligned} \begin{aligned}&\partial _{t} v_i + v_1 a_{j1} \partial _{j}v_i + v_2 a_{j2} \partial _{j}v_i + J^{-1}(v_3-\psi _t) \partial _{3} v_i + a_{ki}\partial _{k}q =0 , \\\quad&a_{ki} \partial _{k}v_i=0 \end{aligned} \end{aligned}$$
(2.9)

in \(\Omega \), with the initial condition

$$\begin{aligned} v|_{t=0} = v_0 \end{aligned}$$
(2.10)

and the boundary condition

$$\begin{aligned} v_3=0 \text { on } \Gamma _0 . \end{aligned}$$
(2.11)

Note that, using the form of the third column of (2.7), the first equation in (2.9) may also be expressed as

$$\begin{aligned} \partial _{t} v_i + v_k a_{jk} \partial _{j}v_i - J^{-1} \psi _t \partial _{3} v_i + a_{ki}\partial _{k}q =0 \text { in }\Omega \mathrm{,\qquad {}} i=1,2,3 . \end{aligned}$$
(2.12)

The kinematic condition (2.4) takes the form

$$\begin{aligned} Ja_{3i}v_i = w_t \text { on }\Gamma _1 , \end{aligned}$$
(2.13)

while (2.3) reads

$$\begin{aligned} w_{tt} -\Delta _2 w = q , \end{aligned}$$
(2.14)

with the pressure normalized by

$$\begin{aligned} \int _{\Gamma _1} q = 0 . \end{aligned}$$
(2.15)

The next statement on a priori bound for the local existence is our main result.

Theorem 2.1

Assume that (v, q, w) is a \(C^{\infty }\) solution on a time interval \([0,T_0]\), where \(T_0>0\), such that

$$\begin{aligned} \begin{aligned} \Vert v_0\Vert _{H^{r}}, \Vert w_1\Vert _{H^{r-0.5}(\Gamma _1)} \le M_0 , \end{aligned} \end{aligned}$$
(2.16)

where \(M_0\ge 1\) and \(r>2.5\). Then v, w, and q satisfy

$$\begin{aligned} \begin{aligned}&\Vert v\Vert _{H^{r}}, \Vert v_t\Vert _{H^{r-1}}, \Vert w\Vert _{H^{r+0.5}(\Gamma _1)} \le M \mathrm{,\qquad {}} t\in [0,T] , \end{aligned} \end{aligned}$$
(2.17)

with \(M=C M_0^{r+1}\) and

$$\begin{aligned} \begin{aligned}&\Vert w_t\Vert _{H^{r-0.5}(\Gamma _1)}, \Vert w_{tt}\Vert _{H^{r-1.5}(\Gamma _1)}, \Vert q\Vert _{H^{r}} \le K \mathrm{,\qquad {}} t\in [0,T] , \end{aligned} \end{aligned}$$
(2.18)

where K is a constant depending on \(M_0\) and \(T\in (0,T_0]\) is a sufficiently small time depending on \(M_0\).

Here and in the sequel \(C\ge 2\) denotes a sufficiently large generic constant. Also, if the domain of the Sobolev space is not specified, as in (2.17), it is understood to be \(\Omega \).

Remark 2.2

Here we point out differences in the membrane case treated here compared to the plate setting from [21]. While in both cases we achieve the sharp level of regularity for the Euler equations (\(H^{2.5+\delta }\), where \(\delta >0\) is arbitrary), the regularity for the membrane displacement w and its velocity \(w_t\) is lower here. However, the different form of the critical pressure quantity (3.16) below, which drives the energy for the wave part, is directly controllable. This allows for a simplification of the approach since the tangential estimates for the velocity are not needed. In addition, the fact that the pressure term is controllable allows for a simpler construction; in particular, the smoothing term in the wave equation is no longer needed. Due to this and the new treatment of the pressure, the uniqueness and construction also hold here for the data in \(H^{2.5+\delta }\). In addition to the simplification induced by the higher regularity of the pressure energy term, we also simplify the vorticity estimate, which no longer depends on the Sobolev extension of the Jacobian. \(\square \)

Throughout the paper, we keep \(r>2.5\) fixed, allowing all the constants to depend on this parameter without mention. Next, we assert the uniqueness of solutions.

Theorem 2.3

Let \(r>2.5\). Assume that two solutions (v, w) and \((\tilde{v}, \tilde{w})\) satisfy the regularity (2.17)–(2.18) for some \(T>0\) and

$$\begin{aligned} (v(0),w_t(0))=(\tilde{v}(0),\tilde{w}_t(0)) . \end{aligned}$$

Then \((v,w)=(\tilde{v}, \tilde{w})\) on [0, T].

Both theorems are proven in the next section. Finally, we state the existence theorem for solutions in the class asserted in the a priori estimates.

Theorem 2.4

Assume that the pair

$$\begin{aligned} (v_{0}, w_{1})\in H^{r} \times H^{r-0.5}(\Gamma _{1}) , \end{aligned}$$
(2.19)

where \(r>2.5\), satisfies the compatibility conditions

$$\begin{aligned} v_{0}\cdot N |_{\Gamma _{1}} =w_1 \end{aligned}$$
(2.20)

and

$$\begin{aligned} v_{0}\cdot N |_{\Gamma _{0}}=0 \end{aligned}$$
(2.21)

with

$$\begin{aligned} \textrm{div}v_{0}=0 \text { in }\Omega \end{aligned}$$
(2.22)

and

$$\begin{aligned} \int _{\Gamma _1} w_1 = 0 . \end{aligned}$$
(2.23)

Then there exists a solution \((v,q,w,w_{t} )\) to the Euler-plate system (2.9)–(2.15) with the initial data \((v_0,w_1)\) on a time interval [0, T] such that

$$\begin{aligned} \begin{aligned}&v \in L^{\infty }([0,T];H^{r}(\Omega )) \cap C([0,T];H^{r-\delta }(\Omega )) , \\ {}&v_{t} \in L^{\infty }([0,T];H^{r-1}(\Omega )) , \\ {}&q \in L^{\infty }([0,T];H^{r}(\Omega )) , \\ {}&w \in L^{\infty }([0,T];H^{r+0.5}(\Gamma _{1})) , \\ {}&w_{t} \in L^{\infty }([0,T];H^{r-0.5}(\Gamma _{1})) , \end{aligned} \end{aligned}$$
(2.24)

for every \(\delta >0\) and with some time \(T>0\) depending on \(\Vert v_0\Vert _{H^{r}}\) and \(\Vert w_1\Vert _{H^{r-0.5}(\Gamma _1)}\).

The proof of this existence theorem follows the parallel statement in [21]. Note however that the regularity properties of v, q, and w in Theorem 2.1 allow us to conclude the boundedness of the critical term \(\int _{0}^{t} \int _{\Gamma _1} q \Lambda ^{2(r-0.5)} w_{t}\) in (3.15) below, which may be rewritten as \( \int _{0}^{t} \int _{\Gamma _1} \Lambda ^{r-0.5} q \Lambda ^{r-0.5} w_{t}\), and thus the regularization step with the dissipative term involving \(\nu \) in [21] is not necessary, leading to a great simplification of the construction. To avoid repetition, we omit further details.

3 Proof of the a priori bounds

Here, we provide a priori estimates for the system, thus proving Theorem 2.1. With \(C_0\) and K to be determined, we have (2.17) on some interval [0, T], and we intend to provide a lower bound on T.

We start with the bounds on the inverse matrix a and the Jacobian J.

Lemma 3.1

Let \(\epsilon \in (0,1/2]\), and assume that

$$\begin{aligned} \begin{aligned}&\Vert v\Vert _{H^{r}}, \Vert w\Vert _{H^{r+0.5}(\Gamma _1)}, \Vert w_t\Vert _{H^{r-0.5}(\Gamma _1)} \le M \mathrm{,\qquad {}} t\in [0,T] , \end{aligned} \end{aligned}$$
(3.1)

where \(M\ge 1\) is as in the statement of Theorem 2.1. Then we have

$$\begin{aligned} \Vert a-I\Vert _{H^{r-1}}, \Vert a-I\Vert _{L^{\infty }}, \Vert J-1\Vert _{H^{r-1}}, \Vert J-1\Vert _{L^{\infty }} \le \epsilon \mathrm{,\qquad {}} t\in [0,T_0] , \end{aligned}$$
(3.2)

where

$$\begin{aligned} T_0= \min \biggl \{T,\frac{\epsilon }{C M^{3}}\biggr \} , \end{aligned}$$
(3.3)

and C is a sufficiently large constant depending on \(C_0\).

First, note that, using the definitions of \(\psi \) and \(\eta \), we have

$$\begin{aligned} \Vert \eta \Vert _{H^{r+1}} \lesssim \Vert \psi \Vert _{H^{r+1}} \lesssim \Vert w\Vert _{H^{r+0.5}(\Gamma _1)} \end{aligned}$$
(3.4)

and

$$\begin{aligned} \Vert \eta _t\Vert _{H^{r}} \lesssim \Vert \psi _t\Vert _{H^{r}} \lesssim \Vert w_t\Vert _{H^{r-0.5}(\Gamma _1)} . \end{aligned}$$
(3.5)

Also, we have

$$\begin{aligned} \Vert J\Vert _{H^{r}} = \Vert \partial _{3}\psi \Vert _{H^{r}} \lesssim \Vert \psi \Vert _{H^{r+1}} \lesssim \Vert w\Vert _{H^{r+0.5}(\Gamma _1)} \lesssim M \end{aligned}$$
(3.6)

and

$$\begin{aligned} \Vert J_t\Vert _{H^{r-1}} \lesssim \Vert \psi _t\Vert _{H^{r}} \lesssim \Vert \eta _t\Vert _{H^{r}} \lesssim \Vert w_t\Vert _{H^{r-0.5}(\Gamma _1)} \lesssim M . \end{aligned}$$
(3.7)

For the matrix a, one may easily check that

$$\begin{aligned} \Vert a\Vert _{H^{r}} \lesssim \Vert \psi \Vert _{H^{r+1}} ( \Vert \psi \Vert _{H^{r}} + 1) \end{aligned}$$
(3.8)

and

$$\begin{aligned} \Vert a_t\Vert _{H^{r-1}} \le \Vert \psi _t\Vert _{H^{r}} ( \Vert \psi \Vert _{H^{r}} + 1) , \end{aligned}$$
(3.9)

provided \(J\ge 1/2\). As usual, \(A\lesssim B\) stands for \(A\le C B\) for a sufficiently large constant \(C\ge 1\).

Proof of Lemma 3.1

Employing \(J(0)-1=\int _{0}^{t}J_t\,ds \) and (3.7), we obtain bounds for the third and the fourth expressions in (3.2), provided \(T_0\) is as in (3.3) and C is sufficiently large. In particular, if \(\epsilon >0\) is sufficiently small, we have \(J\ge 1/2\) for \(t\in [0,T_0]\) with \(T_0\) as in (3.3). Differentiating \(a \nabla \eta =I\) in time, we get \( a_t = - a\nabla \eta _t a \), and then, using also \(a(0)=I\), it follows that

$$\begin{aligned} \begin{aligned} \Vert a-I\Vert _{L^{\infty }} \lesssim \Vert a-I\Vert _{H^{r-1}} \lesssim \biggl \Vert \int _{0}^{t} a \nabla \eta _t a \,ds \biggr \Vert _{H^{r-1}} \lesssim T_0 M^{3} . \end{aligned} \end{aligned}$$

With \(T_0\) as in (3.3), we obtain the bound for the first two norms in (3.2). \(\square \)

In the sequel, we do not distinguish between \(T_0\) and T; thus we assume that (3.1) is satisfied and that

$$\begin{aligned} T\le \frac{\epsilon }{C M^{3}} , \end{aligned}$$
(3.10)

where \(\epsilon =1/C\) and \(C\ge 2\) is sufficiently large. Note that, in particular, \(T\le 1\).

We are now ready to prove the main result.

Proof of Theorem 2.1

Assume that [0, T], where \(T>0\) is as in (3.10), is an interval such that (v, q, w) is a \(C^{\infty }\) solution with the initial data \(v_0\) satisfying (2.17)–(2.18). We intend to prove then that T can be chosen so it depends only on \(M_0\).

We start with the pressure estimates. The interior elliptic equation for the pressure is obtained by applying \(J a_{ji}\partial _{j}\) to the first equation in (2.9). After some rewriting, as in [21, Remark 3.5], we obtain

$$\begin{aligned} \Delta q&= \partial _{j}( ( \delta _{ji}\delta _{ki}- J a_{ji} a_{ki})\partial _{k}q) + \partial _{j}(\partial _{t}(J a_{ji}) v_i) \nonumber \\ {}&\quad {}- \sum _{m=1}^{2} J a_{ji} \partial _{j}(v_m a_{km}) \partial _{k} v_i - J a_{ji} \partial _{j}(J^{-1}(v_3-\psi _t))\partial _{3}v_i \nonumber \\ {}&\quad {}+ \sum _{m=1}^{2} v_m a_{km} \partial _{k} (J a_{ji}) \partial _{j}v_i + J^{-1}(v_3-\psi _t)\partial _{3}( J a_{ji})\partial _{j}v_i \text { in }\Omega . \end{aligned}$$
(3.11)

On the other hand, to obtain the boundary conditions on \(\Gamma _0\) and \(\Gamma _1\), we test the Euler equations with \(a_{3i}\) and evaluate. On the bottom boundary, we have

$$\begin{aligned} \begin{aligned} \partial _{3}q = (J a_{3i}a_{ki} - \delta _{3i}\delta _{ki})\partial _{k}q \text { on }\Gamma _0 , \end{aligned} \end{aligned}$$
(3.12)

while for the top boundary, after some work, as in [21, Remark 3.5], we obtain a Robin-type boundary condition

$$\begin{aligned}&\partial _{3}q + q \quad {}= (\delta _{3i}\delta _{ki}-J a_{3i}a_{ki})\partial _{k}q -\Delta _2 w + \partial _{t}(J a_{3i}) v_i \nonumber \\ {}&\quad {}\quad {}- \frac{1}{J} \biggl ( \sum _{j=1}^{2} J v_k a_{jk} \partial _{j}w_t + w_t \partial _{3} (J a_{3i}) v_i - \partial _{j} (J a_{3i}) v_k J a_{jk} v_i \biggr ) \text { on }\Gamma _1 . \end{aligned}$$
(3.13)

Using (3.8), and the algebra property of \(H^{r-1}\), and elliptic regularity, we get

$$\begin{aligned} \begin{aligned} \Vert q\Vert _{H^{r}} \le P( \Vert v\Vert _{H^{r}}, \Vert w\Vert _{H^{r+0.5}(\Gamma _1)}, \Vert w_{t} \Vert _{H^{r-0.5}(\Gamma _1)} ) \end{aligned} \end{aligned}$$
(3.14)

on the interval [0, T]; here and in the sequel, the symbol P denotes a generic nonnegative polynomial of its arguments.

The control of the displacement is obtained by using a tangential estimate. With \(\Delta _2=\partial _1^2+\partial _2^2\), denote

$$\begin{aligned} \Lambda =(I-\Delta _2)^{1/2} . \end{aligned}$$

Using \(w(0)=0\), we obtain from the wave equation (2.14)

$$\begin{aligned} \frac{1}{2} \Vert \nabla \Lambda ^{r-0.5} w\Vert _{L^2(\Gamma _1)}^2 + \frac{1}{2} \Vert \Lambda ^{r-0.5} w_{t}\Vert _{L^2(\Gamma _1)}^2&= \frac{1}{2} \Vert \Lambda ^{r-0.5} w_1\Vert _{L^2(\Gamma _1)}^2 \nonumber \\&\quad + \int _{0}^{t} \int _{\Gamma _1} q \Lambda ^{2(r-0.5)} w_{t} . \end{aligned}$$
(3.15)

Estimating the last term on the right-hand side as

$$\begin{aligned} \int _{0}^{t} \int _{\Gamma _1} q \Lambda ^{2(r-0.5)} w_{t}&= \int _{0}^{t} \int _{\Gamma _1} \Lambda ^{r-0.5}q \Lambda ^{r-0.5} w_{t} \lesssim \int _{0}^{t} \Vert q \Vert _{H^{r-0.5}(\Gamma _{1})} \Vert w_{t} \Vert _{H^{r-0.5}(\Gamma _{1})} \nonumber \\&\lesssim \int _{0}^{t} \Vert q \Vert _{H^{r}} \Vert w_{t} \Vert _{H^{r-0.5}(\Gamma _{1})} \nonumber \\&\le \int _{0}^{t} P( \Vert v\Vert _{H^{r}}, \Vert w\Vert _{H^{r+0.5}(\Gamma _1)}, \Vert w_{t} \Vert _{H^{r-0.5}(\Gamma _1)} ) , \end{aligned}$$
(3.16)

with a help of (3.14) in the last step. We obtain

$$\begin{aligned} \begin{aligned}&\Vert \nabla \Lambda ^{r-0.5} w\Vert _{L^2(\Gamma _1)}^2 + \Vert \Lambda ^{r-0.5} w_{t}\Vert _{L^2(\Gamma _1)}^2 \lesssim \Vert w_1\Vert _{H^{r-0.5}(\Gamma _1)}^2 \\&\quad +\int _{0}^{t} P( \Vert v\Vert _{H^{r}}, \Vert w\Vert _{H^{r+0.5}(\Gamma _1)}, \Vert w_{t}\Vert _{H^{r-0.5}(\Gamma _1)} )\,ds , \end{aligned} \end{aligned}$$

and since

$$\begin{aligned} \Vert \Lambda ^{r-0.5}w\Vert _{L^2(\Gamma _1)}^2= & {} \left\| \int _{0}^{t} \Lambda ^{r-0.5}w_t\right\| _{L^2(\Gamma _1)}^2\\\le & {} t\int _{0}^{t}\Vert \Lambda ^{r-0.5}w_t\Vert _{L^2(\Gamma _1)}^2 \lesssim \int _{0}^{t}\Vert \Lambda ^{r-0.5} w_t\Vert _{L^2(\Gamma _1)}^2 , \end{aligned}$$

by \(t\le T\le 1\), we get

$$\begin{aligned}&\Vert \Lambda ^{r+0.5} w\Vert _{L^2(\Gamma _1)}^2 + \Vert \Lambda ^{r-0.5} w_{t}\Vert _{L^2(\Gamma _1)}^2 \nonumber \\ {}&\quad {}\lesssim \Vert w_1\Vert _{H^{r-0.5}(\Gamma _1)}^2 +\int _{0}^{t} P( \Vert v\Vert _{H^{r}}, \Vert w\Vert _{H^{r+0.5}(\Gamma _1)}, \Vert w_{t}\Vert _{H^{r-0.5}(\Gamma _1)} )\,ds , \end{aligned}$$
(3.17)

for \(t\in [0,T]\); when not specified, the time argument is understood to be t.

With the pressure and tangential estimates in (3.14) and (3.17) established, we use the ALE vorticity

$$\begin{aligned} \zeta (x,t)={\textrm{curl}}u(\eta (x,t),t) , \end{aligned}$$

where \(\omega ={\textrm{curl}}u\), to get control of the velocity v. In the ALE variables, the vorticity reads

$$\begin{aligned} \zeta _i = \epsilon _{ijk} a_{mj} \partial _{m} v_k \end{aligned}$$

and thus satisfies

$$\begin{aligned} \begin{aligned} \partial _{t}\zeta _i + v_1 a_{j1}\partial _{j} \zeta _i + v_2 a_{j2}\partial _{j} \zeta _i + (v_3-\psi _t) a_{j3} \partial _{j} \zeta _i = \zeta _k a_{mk}\partial _{m}v_i \mathrm{,\qquad {}} i=1,2,3 . \end{aligned} \end{aligned}$$
(3.18)

At this point, we use the Sobolev extension operator \(f\mapsto \tilde{f}\), which is a continuous operator \(H^{k}(\Omega )\rightarrow H^{k}({\mathbb {T}}^2\times {\mathbb {R}})\) for all \(k\in [0,r+5]\), where k is not necessarily an integer. We require the extension to be such that \(\textrm{supp}\tilde{f}\) vanishes in a neighborhood of \({\mathbb {T}}^2\times (-1/2,3/2)^{\text{ c }}\). Then consider the solution \(\theta =(\theta _1,\theta _2,\theta _3)\) of

$$\begin{aligned} \begin{aligned} \partial _{t}\theta _i + \tilde{v}_1 \tilde{a}_{j1}\partial _{j} \theta _i + \tilde{v}_2 \tilde{a}_{j2}\partial _{j} \theta _i + (\tilde{v}_3-\tilde{\psi }_t) \tilde{a}_{j3} \partial _{j} \theta _i = \theta _k \tilde{a}_{mk}\partial _{m}\tilde{v}_i \mathrm{,\qquad {}} i=1,2,3 \end{aligned} \end{aligned}$$
(3.19)

in \(\Omega _0={\mathbb {T}}^2\times {\mathbb {R}}\), with the initial condition \( \theta (0)=\tilde{\zeta }(0) \). (Note that (3.19) is a simpler equation than the one considered in [21].) By the properties of the extension operator and since the equation for \(\theta \) is of transport type, we have

$$\begin{aligned} \theta (x,t) = 0 \mathrm{,\qquad {}} (x,t)\in ({\mathbb {T}}^2\times (-1/2,3/2)^{\text{ c }} )\times [0,T] . \end{aligned}$$

We now introduce the quantity

$$\begin{aligned} X = \int _{\Omega _0} |\Lambda _3^{r-1}\theta |^2 , \end{aligned}$$
(3.20)

where

$$\begin{aligned} \Lambda _3 =(I-\Delta )^{1/2} \end{aligned}$$

on \({{\mathbb {T}}}^2\times {{\mathbb {R}}}\). We claim that we have

$$\begin{aligned} \begin{aligned} \frac{d}{dt}X \lesssim P(\Vert v\Vert _{H^{r}}, \Vert w\Vert _{H^{r+0.5}(\Gamma _1)}, \Vert w_{t}\Vert _{H^{r-0.5}(\Gamma _1)} ) X \mathrm{,\qquad {}} t\in [0,T] . \end{aligned} \end{aligned}$$
(3.21)

Note that, by the continuity properties of the Sobolev extensions, we have

$$\begin{aligned} \Vert \zeta (0)\Vert _{H^{r-1}}^2 \lesssim X(0) \lesssim \Vert \zeta (0)\Vert _{H^{r-1}}^2 . \end{aligned}$$

To prove (3.21), we differentiate (3.20) and use (3.19) to obtain

$$\begin{aligned} \begin{aligned} \frac{1}{2} \frac{d X}{dt}&= - \sum _{m=1}^{2} \int _{\Omega _0} \tilde{v}_m \tilde{a}_{jm}\partial _{j} \Lambda _3^{r-1}\theta _i \Lambda _3^{r-1}\theta _i - \int _{\Omega _0} (\tilde{v}_3-\tilde{\psi }_t) \tilde{a}_{j3} \partial _{j} \Lambda _3^{r-1}\theta _i \Lambda _3^{r-1}\theta _i \\ {}&\quad {}+ \int _{\Omega _0} \Lambda _3^{r-1} (\theta _k \tilde{a}_{mk}\partial _{m}\tilde{v}_i )\Lambda _3^{r-1}\theta _i \\ {}&\quad {}- \sum _{m=1}^2 \int _{\Omega _0} \Bigl (\Lambda _3^{r-1}(\tilde{v}_m \tilde{a}_{jm} \partial _{j} \theta _i ) - \tilde{v}_m \tilde{a}_{jm} \partial _{j} \Lambda _3^{r-1}\theta _i \Bigr )\Lambda _3^{r-1}\theta _i \\ {}&\quad {}- \int _{\Omega _0} \Bigl ( \Lambda _3^{r-1}( (\tilde{v}_3-\tilde{\psi }_t) \tilde{a}_{j3} \partial _{j}\theta _i ) - (\tilde{v}_3-\tilde{\psi }_t) \tilde{a}_{j3} \partial _{j} \Lambda _3^{r-1}\theta _i \Bigr ) \Lambda _3^{r-1}\theta _i \\ {}&\quad {}= I + \bar{I} , \end{aligned} \end{aligned}$$

where I represents the first two terms, which are the ones with an additional derivative on \(\theta \), while \(\bar{I}\) stands for the rest. For I, we integrate by parts in \(x_j\), obtaining

$$\begin{aligned} \begin{aligned} I&= \frac{1}{2} \sum _{m=1}^{2} \int _{\Omega _0} \partial _{j}(\tilde{v}_m \tilde{a}_{jm}) \Lambda _3^{r-1}\theta _i \Lambda _3^{r-1}\theta _i + \frac{1}{2} \int _{\Omega _0} \partial _{j}( (\tilde{v}_3-\tilde{\psi }_t) \tilde{a}_{j3}) \Lambda _3^{r-1}\theta _i \Lambda _3^{r-1}\theta _i . \end{aligned} \end{aligned}$$
(3.22)

Estimating the expression for I in (3.22) and bounding all the terms in \(\bar{I}\) directly, we obtain

$$\begin{aligned} \begin{aligned} I+ \bar{I}&\le P(\Vert v\Vert _{H^{r}}, \Vert w\Vert _{H^{r+0.5}(\Gamma _1)}, \Vert w_{t}\Vert _{H^{r-0.5}(\Gamma _1)} ) \Vert \theta \Vert _{H^{r-1}}^2 , \end{aligned} \end{aligned}$$

and thus (3.21) is established.

To relate the quantity X to the actually vorticity, we claim that

$$\begin{aligned} \Vert \zeta \Vert _{H^{r-1}}^2 \lesssim X . \end{aligned}$$
(3.23)

The difference \(\sigma =\zeta -\theta \) satisfies

$$\begin{aligned} \partial _{t}\sigma _i + v_1 a_{j1}\partial _{j} \sigma _i + v_2 a_{j2}\partial _{j} \sigma _i + (v_3-\psi _t) a_{j3} \partial _{j} \sigma _i = \sigma _k a_{mk}\partial _{m} v_i \text { in }\Omega , \end{aligned}$$

for \(i=1,2,3\). This leads to the energy equality

$$\begin{aligned} \begin{aligned} \frac{1}{2} \frac{d}{dt} \int |\sigma |^2&= - \sum _{m=1}^{2} \int v_m a_{jm}\sigma _i \partial _{j} \sigma _i - \int (v_3-\psi _t) a_{j3} \sigma _i \partial _{j} \sigma _i + \int \sigma _k a_{mk}\partial _{m}v_i \sigma _i \\ {}&= \frac{1}{2} \sum _{m=1}^{2} \int \Bigl (\partial _{j}( v_m a_{jm}) + \partial _{j} (v_3-\psi _t)\Bigr )|\sigma |^2 + \int \sigma _k a_{mk}\partial _{m}v_i \sigma _i , \end{aligned} \end{aligned}$$
(3.24)

where the boundary terms vanish since

$$\begin{aligned} \sum _{m=1}^{2} v_m a_{3m} + ( v_3-\psi _t) a_{33} = 0 \text { on }\partial \Omega ; \end{aligned}$$
(3.25)

the equation (3.25) can be readily checked using (2.7), (2.11), and (2.13). From (3.24) and \(\sigma (0)=0\) in \(\Omega \), we obtain \(\zeta =\theta \) in \(\Omega \), and thus (3.23) holds.

Finally, we summarize the obtained estimates. To use the div-curl estimate from [5], we bound \({\textrm{curl}}v\) in \(\textrm{div}v\) and \(v\cdot N\) in appropriate Sobolev spaces. For \({\textrm{curl}}v\), we have

$$\begin{aligned} \begin{aligned} \Vert {\textrm{curl}}v\Vert _{H^{r-1}}&\lesssim \sum _{i} \Vert \epsilon _{ijk} a_{mj} \partial _{m} v_k \Vert _{H^{r-1}} + \sum _{i} \Vert \epsilon _{ijk} \partial _{m} v_k (a_{mj}-\delta _{mj}) \Vert _{H^{r-1}} \\&\quad \lesssim \Vert \zeta \Vert _{H^{r-1}}+ \epsilon \Vert v\Vert _{H^{r}} , \end{aligned} \end{aligned}$$

using also (3.2). Therefore, with the help of (3.21) and (3.23), we have

$$\begin{aligned}&\Vert {\textrm{curl}}v\Vert _{H^{r-1}} \lesssim \Vert \zeta (0)\Vert _{H^{r-1}} + \epsilon \Vert v\Vert _{H^{r}} \nonumber \\&\quad + \int _{0}^{t} P( \Vert v\Vert _{H^{r}}, \Vert w\Vert _{H^{r+0.5}(\Gamma _1)}, \Vert w_{t}\Vert _{H^{r-0.5}(\Gamma _1)} ) X^{1/2} \,ds . \end{aligned}$$
(3.26)

For the divergence, we simply use the divergence-free condition to write

$$\begin{aligned} \begin{aligned} \Vert \textrm{div}v\Vert _{H^{r-1}}&= \Vert (a_{ki}-\delta _{ki})\partial _{k} v_i \Vert _{H^{r-1}} \lesssim \epsilon \Vert v\Vert _{H^{r}} , \end{aligned} \end{aligned}$$
(3.27)

while for the bottom boundary term, we have

$$\begin{aligned} v_3|_{\Gamma _0} =0 . \end{aligned}$$

Also, by (2.13), we get

$$\begin{aligned} \begin{aligned} \Vert v_3\Vert _{H^{r-0.5}(\Gamma _1)}&\lesssim \Vert (J a_{3i} - \delta _{3i})v_i\Vert _{H^{r-0.5}(\Gamma _1)} + \Vert w_{t}\Vert _{H^{r-0.5}(\Gamma _1)} \\ {}&\lesssim \Vert J a - I\Vert _{H^{r}} \Vert v\Vert _{L^{\infty }} + \Vert J a - I\Vert _{L^{\infty }} \Vert v\Vert _{H^{r}} + \Vert w_{t}\Vert _{H^{r-0.5}(\Gamma _1)} \\ {}&\lesssim \Vert v\Vert _{H^{r-1}} ( \Vert w\Vert _{H^{r+0.5}(\Gamma _1)} + 1) + \epsilon \Vert v\Vert _{H^{r}} + \Vert w_{t}\Vert _{H^{r-0.5}(\Gamma _1)} \\ {}&\lesssim \epsilon \Vert v\Vert _{H^{r}} + \frac{1}{\epsilon ^{r-1}} ( \Vert w\Vert _{H^{r+0.5}(\Gamma _1)} + 1)^{r} \Vert v\Vert _{L^2} + \Vert w_{t}\Vert _{H^{r-0.5}(\Gamma _1)} , \end{aligned} \end{aligned}$$
(3.28)

for every \(\epsilon \in (0,1]\), where we used \(\Vert J a\Vert _{H^{r}}\lesssim \Vert \psi \Vert _{H^{r+1}}+1\lesssim \Vert w\Vert _{H^{r+1/2}(\Gamma _1)}+ 1\) in the third and \(\Vert v\Vert _{H^{r-1}}\lesssim \Vert v\Vert _{L^{2}}^{1/r}\Vert v\Vert _{H^{r}}^{(r-1)/r} \) in the fourth inequality. Therefore, we obtain

$$\begin{aligned} \Vert v_3\Vert _{H^{r-0.5}(\Gamma _1)}&\lesssim \epsilon \Vert v\Vert _{H^{r}} + \frac{1}{\epsilon ^{r-1}} ( \Vert w\Vert _{H^{r+0.5}(\Gamma _1)}^{r} + 1) \Vert v_0\Vert _{L^2} \nonumber \\&\quad {}+ \Vert w_{t}\Vert _{H^{r-0.5}(\Gamma _1)} + \frac{1}{\epsilon ^{r-1}} ( \Vert w\Vert _{H^{r+0.5}(\Gamma _1)}^{r} + 1) \int _{0}^{t}\Vert v_t\Vert _{L^2} , \end{aligned}$$
(3.29)

where \(\epsilon \in (0,1]\) is arbitrary. Using (3.26), (3.27), and (3.29) in the div-curl elliptic estimate

$$\begin{aligned} \Vert v\Vert _{H^{r}} \lesssim \Vert {\textrm{curl}}v\Vert _{H^{r-1}} + \Vert \textrm{div}v\Vert _{H^{r-1}} + \Vert v\cdot N\Vert _{H^{r-0.5}(\Gamma _0\cup \Gamma _1)} + \Vert v\Vert _{L^2} \end{aligned}$$

(see [5]), and fixing a sufficiently small \(\epsilon \) so that \(\epsilon \Vert v\Vert _{H^{r}}\), can be absorbed, we conclude

$$\begin{aligned} \Vert v\Vert _{H^{r}}^2&\lesssim \Vert \zeta (0)\Vert _{H^{r-1}}^2 + \Vert w_{t}\Vert _{H^{r-0.5}(\Gamma _1)}^2 + ( \Vert w\Vert _{H^{r+0.5}(\Gamma _1)}^{2r} + 1) \Vert v_0\Vert _{L^2}^2 \nonumber \\&\quad {}+ t ( \Vert w\Vert _{H^{r+0.5}(\Gamma _1)}^{2r} + 1) \int _{0}^{t}\Vert v_t\Vert _{L^2}^2 + t \int _{0}^{t} P(\Vert w\Vert _{H^{r+0.5}(\Gamma _1)},\Vert w_t\Vert _{H^{r+0.5}(\Gamma _1)}) \nonumber \\&\quad {}+ t \int _{0}^{t} P( \Vert v\Vert _{H^{r}}, \Vert w\Vert _{H^{r+0.5}(\Gamma _1)}, \Vert w_{t}\Vert _{H^{r-0.5}(\Gamma _1)} )X \,ds . \end{aligned}$$
(3.30)

In (3.30), we use (3.17) to estimate the terms involving w and \(w_t\) outside the integral and the inequality

$$\begin{aligned} \Vert v_t\Vert _{H^{r-1}} \le P( \Vert v\Vert _{H^{r}}, \Vert w\Vert _{H^{r+0.5}(\Gamma _1)}, \Vert w_{t}\Vert _{H^{r-0.5}(\Gamma _1)} ) , \end{aligned}$$
(3.31)

which is obtained directly from the first equation in (2.9) and employing the bounds for J and a in (3.6) and (3.8). Thus we obtain

$$\begin{aligned} \Vert v\Vert _{H^{r}}^2&\lesssim \Vert v_0\Vert _{H^{r}}^2 + \Vert w_1\Vert _{H^{r-0.5}(\Gamma _1)}^{2} \nonumber \\&\quad {}+ \Vert v_0\Vert _{H^{r}}^2 \left( \Vert w_1\Vert _{H^{r-0.5}(\Gamma _1)}^{2r} +\int _{0}^{t} P( \Vert v\Vert _{H^{r}}, \Vert w\Vert _{H^{r+0.5}(\Gamma _1)}, \Vert w_{t}\Vert _{H^{r-0.5}(\Gamma _1)} )\,ds \right) \nonumber \\&\quad {}+ (\Vert w_1\Vert _{H^{r-0.5}(\Gamma _1)}^{2r}+1) \int _{0}^{t} P(\Vert w\Vert _{H^{r+0.5}(\Gamma _1)},\Vert w_t\Vert _{H^{r+0.5}(\Gamma _1)}) \nonumber \\&\quad {}+ \int _{0}^{t} P( \Vert v\Vert _{H^{r}}, \Vert w\Vert _{H^{r+0.5}(\Gamma _1)}, \Vert w_{t}\Vert _{H^{r-0.5}(\Gamma _1)} ) X\,ds . \end{aligned}$$
(3.32)

Applying a Gronwall argument on (3.17), (3.21), and (3.32), we obtain an estimate

$$\begin{aligned} \Vert v\Vert _{H^{r}} + \Vert w\Vert _{H^{r+0.5}(\Gamma _1)} + \Vert w_{t}\Vert _{H^{r-0.5}(\Gamma _1)} + X^{1/2} \lesssim 1+M^{r+1} \end{aligned}$$

on [0, T], for \(T>0\) depending on M, and the proof of the theorem is concluded. \(\square \)

Finally, we prove the uniqueness statement, Theorem 2.3.

Proof of Theorem 2.3

Let \((v,q,w,a,\eta )\) and \((\tilde{v},\tilde{q},\tilde{w},\tilde{\eta },\tilde{a})\) be two solutions on [0, T] with the same initial data, both satisfying the assertions in Theorem 2.1, and denote by

$$\begin{aligned} (W,V,Q,E,A,\Psi ) = (w,v,q,\eta ,a,\psi ) - (\tilde{w}, \tilde{v},\tilde{q},\tilde{\eta },\tilde{a},\tilde{\psi }) \end{aligned}$$

the difference. In this section, we allow all the implicit constants to depend on the norms of (v, q, w) and \((\tilde{v}, \tilde{q}, \tilde{w})\) in (2.17)–(2.18).

To obtain an estimate on the difference of the pressures, we subtract (3.11)–(3.13) and the analogous equations for \(\tilde{q}\). Applying the elliptic regularity estimate to the difference, we obtain

$$\begin{aligned} \begin{aligned} \Vert Q\Vert _{H^{r-1}} \lesssim \Vert V\Vert _{H^{r-2}} + \Vert W\Vert _{H^{r-0.5}(\Gamma _1)} + \Vert W_{t}\Vert _{H^{r-1.5}(\Gamma _1)} . \end{aligned} \end{aligned}$$
(3.33)

Next, from the equation satisfied by \(W=w-\tilde{w}\), we have the energy equality

$$\begin{aligned} \frac{1}{2} \frac{d}{dt} \Bigl (\Vert \nabla \Lambda ^{r-1.5} W \Vert _{L^2(\Gamma _1)}^2 + \Vert \Lambda ^{r-1.5} W_{t}\Vert _{L^2(\Gamma _1)}^2 \Bigr ) = \int _{\Gamma _1} Q \Lambda ^{2(r-1.5)} W_{t} , \end{aligned}$$
(3.34)

since \(W(0)=W_t(0)=0\). We estimate the last term on the right-hand side as in (3.16) and then bound the norm of the pressure Q in \(H^{r-1}\) using (3.33) obtaining

$$\begin{aligned} \begin{aligned}&\Vert \Lambda ^{r-0.5} W\Vert _{L^2(\Gamma _1)}^2 + \Vert \Lambda ^{r-1.5} W_{t}\Vert _{L^2(\Gamma _1)}^2 \\&\quad \lesssim \int _{0}^{t} (\Vert V\Vert _{H^{r-1}} + \Vert W\Vert _{H^{r-0.5}(\Gamma _1)} + \Vert W_t\Vert _{H^{r-1.5}(\Gamma _1)} )^2\,ds . \end{aligned} \end{aligned}$$

For the vorticity, we need to estimate the difference \(Z=\zeta -\tilde{\zeta }\). For this purpose, we extend \(\zeta \) and \(\tilde{\zeta }\) to \(\theta \) and \(\tilde{\theta }\) so they are defined on \(\Omega _0={{\mathbb {T}}}^2\times {{\mathbb {R}}}\). For simplicity, we do not distinguish in notation between the functions defined on \(\Omega \) and their extensions defined on \(\Omega _0\). With this agreement, we get from (3.18) that the equation for \(\Theta =\theta -\tilde{\theta }\) reads

$$\begin{aligned} \begin{aligned} \partial _{t}\Theta _i + v_1 a_{j1}\partial _{j} \Theta _i + v_2 a_{j2}\partial _{j} \Theta _i + (v_3-\psi _t) a_{j3} \partial _{j} \Theta _i = F_i \mathrm{,\qquad {}} i=1,2,3 , \end{aligned} \end{aligned}$$
(3.35)

where

$$\begin{aligned} \begin{aligned} F_i&= - V_1 a_{j1}\partial _{j} \tilde{\theta }_i - \tilde{v}_1 A_{j1}\partial _{j}\tilde{\theta }_i - V_2 a_{j2}\partial _{j} \tilde{\theta }_i - \tilde{v}_2 A_{j2}\partial _{j}\tilde{\theta }_i \\ {}&\quad - ( V_3- \Psi _t) a_{j3} \partial _{j} \tilde{\theta }_i - ( \tilde{v}_3- \tilde{\psi }_t) A_{j3} \partial _{j} \tilde{\theta }_i + \theta _k a_{mk}\partial _{m} V_i\\&\quad + \Theta _k a_{mk}\partial _{m} \tilde{v}_i + \tilde{\theta }_k A_{mk}\partial _{m} \tilde{v}_i \mathrm{,\qquad {}} i=1,2,3 . \end{aligned} \end{aligned}$$

The energy equality for \(\Theta \) then reads

$$\begin{aligned} \begin{aligned}&\frac{1}{2} \frac{d}{dt} \int _{\Omega _0} |\Lambda _3^{r-2}\Theta |^2 = - \sum _{m=1}^{2} \int _{\Omega _0} v_m a_{jm}\partial _{j} \Lambda _3^{r-2}\Theta _i \Lambda _3^{r-2}\Theta _i \\&\quad - \int _{\Omega _0} (v_3-\psi _t) a_{j3} \partial _{j} \Lambda _3^{r-2}\Theta _i \Lambda _3^{r-1}\Theta _i \\ {}&\quad - \sum _{m=1}^{2} \int _{\Omega _0} \Bigl (\Lambda _3^{r-2}(v_m a_{jm}\partial _{j} \Theta _i ) - v_m a_{jm}\partial _{j} \Lambda _3^{r-2}\Theta _i \Bigr ) \Lambda _3^{r-2}\Theta _i \\ {}&\quad - \int _{\Omega _0} \Bigl ( \Lambda _3^{r-2}( (v_3-\psi _t) a_{j3} \partial _{j}\Theta _i ) - ( v_3-\psi _t) a_{j3} \partial _{j} \Lambda _3^{r-2}\Theta _i \Bigr ) \Lambda _3^{r-2}\Theta _i \\ {}&\quad + \int _{\Omega _0} \Lambda _3^{r-2} F_i \Lambda _3^{r-2}\Theta _i \\ {}&\quad = I + I_F , \end{aligned} \end{aligned}$$

where \(I_F\) denotes the last term and I is the sum of the first four. Using

$$\begin{aligned} I \lesssim \Vert \Theta \Vert _{H^{r-2}}^2 \end{aligned}$$

and

$$\begin{aligned} I_F \lesssim ( \Vert V\Vert _{H^{r-1}} + \Vert \Theta \Vert _{H^{r-2}} + \Vert W\Vert _{H^{r-0.5}(\Gamma _1)} + \Vert W_{t}\Vert _{H^{r-1.5}(\Gamma _1)} ) \Vert \Theta \Vert _{H^{r-2}} , \end{aligned}$$

we obtain

$$\begin{aligned} \begin{aligned} \frac{d}{dt} \int _{\Omega _0} |\Lambda _3^{r-2}\Theta |^2 \lesssim ( \Vert V\Vert _{H^{r-1}} + \Vert \Theta \Vert _{H^{r-2}}&+ \Vert W\Vert _{H^{r-0.5}(\Gamma _1)}\\&\quad + \Vert W_{t}\Vert _{H^{r-1.5}(\Gamma _1)} ) \Vert \Theta \Vert _{H^{r-2}} , \end{aligned} \end{aligned}$$

which concludes the vorticity estimates. Applying the barrier argument and the div-curl estimates as in the proof of Theorem 2.1, we then conclude the proof of uniqueness.

\(\square \)