Abstract
This paper investigates the question of whether Krivine’s classical realizability can provide a verificationist interpretation of classical logic. We argue that this kind of realizability can be considered an adequate candidate for this semantic role, provided that the notion of verification involved is no longer based on proofs, but on programs. On this basis, we show that a special reading of classical realizability is compatible with a verificationist theory of meaning, insofar as pure logic is concerned. Crucially, in order to remain faithful to a fundamental verificationist tenet, we show that classical realizability can be understood from a single-agent perspective, thus avoiding the usual game-theoretic interpretation involving at least two players.
Access this chapter
Tax calculation will be finalised at checkout
Purchases are for personal use only
Similar content being viewed by others
Notes
- 1.
A numeral \(\overline{n}\) stands for a term of the language of arithmetic of the form
$$\displaystyle{\mathop{\underbrace{s(\ldots s}}\limits _{n\text{ times}}(0)\ldots ).}$$In other words, numerals are terms denoting natural numbers, written in a canonical form.
- 2.
This means that the numbers realizing universal formulas correspond to total recursive functions.
- 3.
The semantic value of a formula A is that feature allowing one to determine the semantic notions associated to A, like meaning and truth (cf. Dummett 1991, pp. 24, 30–31).
- 4.
It is worth noting that the difference we sketched between Kleene’s realizability semantics and the algebraic or relational semantics can be taken as a particular instance of the difference between construction-oriented semantics and conditional-oriented semantics studied by Fine (2014, § 1). According to Fine, the first has to be considered as an exact semantics, while the latter an inexact one. The reason is that, in the first case, the semantical entities are wholly, or exactly, relevant for establishing the truth of a given statement. On the contrary, in the second case, the semantical entities are relevant for establishing the truth of a statement only in a loose and inexact way, which is made particularly evident by the fact that in this kind of semantics the monotonicity of the forcing relation holds (see Fine 2014, p. 551).
- 5.
We due this observation to Göran Sundholm.
- 6.
The idea is that, according to the standard practice in intuitionistic logic, we consider \(\neg A\) as defined by A → ⊥ .
- 7.
Notice that the strategies adopted by the Falsifier correspond, instead, to Kreisel’s counterexamples (Boyer and Sandu 2012, p. 823). In this sense, if we focus on the winning strategies for the Falsifier – instead of the winning strategies for the Verifier – Hintikka’s framework can be adapted for justifying a form of falsificationism.
- 8.
It is worth noting that an idealized human agent is not an agent totally freed from any kind of contingent constraints: on the contrary, she possess the very same epistemic capacities that any other concrete human beings possess, the only difference being that her capacities are perfect. More precisely, like every concrete human being, she can deal with only a finite amount of resources and information, and her actions can be performed only in a finite amount of time and space; however, unlike concrete human beings, her finite capacities are not subject to any fixed bound.
- 9.
A non-canonical proof is called by Dummett a demonstration; its relation with a canonical proof is explained in the following manner:
We […] require a distinction between a proof proper – a canonical proof – and the sort of argument which will normally appear in a mathematical article or textbook, an argument which we may call a ‘demonstration’. A demonstration is just as cogent a ground for the assertion of its conclusion as is a canonical proof, and is related to it in this way: a demonstration of a proposition provides an effective means for finding a canonical proof. (Dummett 1973, p. 240)
According to Dummett, the notion of canonical proof is the semantic key concept of the notion of meaning. More precisely, to know the meaning of a sentence A corresponds to know the conditions for its (direct) assertion, which corresponds, in turn, to know what counts as a canonical proof of A. Thus, grounding the notion of truth on that of canonical proof is a way to assigning priority to the notion of meaning with respect to the notion of truth. Furthermore, as Dummett remarks, the conditions for the truth of a sentence and those for its correct assertion do not, in principle, collapse: possessing an effective method for obtaining a canonical proof does not necessarily mean to be able to concretely execute this method and, eventually, get access to this proof (cf. Dummett 1998, p. 122–123). The reason is that human agents could be subject to contingent limitations – e.g. space or time limitations – which do not allow them to terminate the execution of the procedure (e.g. in the case of the normalization, this procedure corresponds to an algorithm of exponential size complexity, which is unfeasible for concrete human agents with limitation of space). Therefore, it is only when idealized human agents are considered that the collapse between the two notions could obtain.
- 10.
It has been argued that the decidability of the notion of proof is in fact an excessively strong assumption. For example, Sundholm (1986, p. 493) argues that the proof relation is only a semi-decidable notion, since ‘we recognize a proof when we see one, but when we don’t see one that does not necessarily mean that there is no proof there.’ However, prominent representatives of this form of verificationism, especially Dummett himself, have firmly advocated the decidability of the notion of proof. A quite exhaustive list of places in which Dummett supports this idea can be found in Sundholm (1983, p. 155).
- 11.
It is worth noting that some authors, like van Atten (2014, § 4.5.2), considers that an essential aspect of the BHK interpretation is that the concepts ‘that figure in meaning explanations […] have to do with our cognitive capacities’. In particular, the idea is that the concept of construction which figures in the BHK interpretation should be conceived such that we recognize a construction when we see one. Accepting this reading of the BHK interpretation – which means indeed to assume that Dummett’s verification is a declination of it – would then mean to accept that Kleene’s realizability is not a formal version of the BHK interpretation, as claimed before.
- 12.
Think of the fact that it is possible to define a recursive function by making appeal to the principle of the excluded middle, as for example
$$\displaystyle{f(x) = _{\mathit{df }}\left \{\begin{array}{ll} 1 & \text{ if the Goldbach conjecture is true} \\ 0 & \text{ if the Goldbach conjecture is false} \end{array} \right.}$$However, there are also other, and more critical, aspects of the notion of recursive function which are inherently classical. For example, the regularity condition, which is used in order to define a function f from a relation R by minimization, states that \(\forall \vec{x}\exists yR(\vec{x},y)\). Here, the existential quantifier is understood classically, in the sense that there is no algorithmic procedure for extracting the witness, otherwise the definition of algorithmic procedures via the notion of recursive functions would be circular (cf. Heyting 1962, p. 195). For further details about the non-constructive aspects of the definition of recursive functions see Coquand (2014) and Sundholm (2014).
- 13.
Note that Kreisel (1973, p. 268) seems to have in mind a very similar situation when he asks if the ‘(logical) language of the current intuitionistic systems [have been] obtained by uncritical transfer from languages which were, tacitly, understood classically’.
- 14.
This means, in particular, that Kleene’s realizability does not allow one to realize the principle of excluded middle for open formulas.
- 15.
This does not mean that Krivine’s realizability always guarantees the theory to be complete with respect to the notion of (classical) proof. This depends indeed from the language in which the (classical) theory is presented. If it is a first-order theory, then completeness holds, but if it is a second-order theory, this could no more be the case (as it depends from the way in which the predicate variables are interpreted in the model). Our presentation of Krivine’s realizability rests on a second-order theory (see Sect. 3). The possible lack of completeness is then due to the fact that a second-order language is adopted, and not to the way in which the notion of realizability is conceived.
- 16.
Indeed, as Prawitz (2006, p. 511) remarks, ‘an invalid proof is not really a proof’.
- 17.
Notice that under the Curry-Howard correspondence for intuitionistic logic a term representing a program corresponds to a proof written in intuitionistic natural deduction. This means that the form of the term reflects the form of the proof, namely the order of application of the inference rules.
- 18.
We will try to clarify later what do we mean here for ‘presuppositions’ (see Sect. 4.4). For the time being, it is sufficient to remark that since a context is a list of closed terms, it cannot be a set of hypothesis, as hypotheses correspond to free variables. Moreover, while hypotheses do not presuppose any epistemic attitude towards their truth or falseness, presuppositions are believed to be true.
- 19.
Notice that the condition that x does not appear in u is not necessary for t not to use its argument. In other words, t could not use the argument in the computation, even if x appears in u. For instance, consider the term \(t \equiv \lambda x.(\lambda y.a)x\), where x and y do not appear in a. Then t is of the form \(\lambda x.u\), where x appears in u, but we have the following reduction sequence: \(\lambda x.(\lambda y.a)x \star k_{a'\cdot \diamond}\cdot a' \cdot \diamond\rightsquigarrow (\lambda y.a)k_{a'\cdot \diamond} \star a' \cdot \diamond\rightsquigarrow \lambda x.a \star k_{a'\cdot \diamond}\cdot a' \cdot \diamond\rightsquigarrow a \star a' \cdot \diamond\).
- 20.
Strictly speaking, these antinomic situations do not imply the incoherence of the system itself. The reason is that, as we already mentioned, verifiers, as well as falsifiers, are only posits. In this sense, it is not astonishing to conceive two logically incompatible situations together: the resulting conflict between these two situations would be only a conflict in principle, not an actual one. On the contrary, a genuine incoherence is obtained when two contrary evidences are present, namely when it is possible to exhibit two proofs of two opposite propositions, respectively (see Miquel 2009a, p. 81). This way of understanding incoherence is the same professed by Hilbert: incoherence is definable only at the level of ‘concrete objects’ (Hilbert 1926, p. 376), i.e. at the level of finitary arithmetic, and not at the level of logic.
- 21.
Notice that while the notion of execution seems to be (in a form or another) universally accepted as a fundamental ingredient of the notion of computation, the notion of termination needs some explications. In some more specific and “concrete” models of computation, like the one represented by partial recursive functions, termination is not a necessary notion (think precisely of the partiality condition). Following Kreisel (1972), this represents an analysis of mechanical effective computability, in the sense that the execution has to be performed by mechanical following a finite list of instruction. However, nothing is said about who has to follow this list of instruction. If it is a human-agent that has to follow it, then the number of steps that she can perform must be finite, since finiteness is a property defining human-agent (see note 8). This means that each execution has to terminate. The notion of termination seems then to be linked to the analysis of what Kreisel calls human effective computability. Beside this computational aspect, termination plays a second key role in the present context. From a meaning theoretic point of view, termination ensures us that we are not transcending the capacities of the human agent, thus allowing us to respect the pivotal desideratum of an anti-realist theory of meaning.
- 22.
Notice, however, that one defines the interpretation of linear logic formulas, and not classical logic formulas, as it will be clarified below.
- 23.
Although the pole is sometimes defined from a set of processes which could be understood as a notion of termination (see Guillermo and Miquel 2014). For instance, one can take an arbitrary set of processes \(\Omega \) and then define a pole \(\perp \!\!\!\perp _{\Omega }\) by simply considering the closure of \(\Omega \) with respect to anti-reduction.
- 24.
We use the terminology of “wrongful programs” as opposed to “proved programs”. Indeed, programs corresponding to proofs in a formal system are proved in the sense that they do exactly what they are expected to. More precisely, the corresponding proof can be understood as a certificate that ensures the program is well-behaved (i.e. produces the right type of output when given the right type of input), and terminates. With this idea in mind, a wrongful program is a program which is proved using a incorrect arguments: it is therefore provided with an unreliable certificate of well-behaviour and termination.
- 25.
Notice that in this sense a proof is considered as a closed (logically) valid derivation (or argument), respecting the definition given in Prawitz (2006, p. 511).
- 26.
For instance, the term \((\lambda x\lambda y.((y)(x)\lambda z.z)(x)\lambda z.\lambda w.z)\lambda z.(z)z\) is not typable in System F even though it is strongly normalizable (Giannini and Ronchi Della Rocca 1988).
- 27.
Actually, as C. Parsons remarked, Hilbert’s strokes, as well as syntactical objects in general, are quasi-concrete objects: they are not simple tokens, but a particular kind of types, the ‘intrinsic [property of which is] to have instantiations in the concrete’ (Parsons 2008, p. 242).
- 28.
The deduction system adopted here is described in details in Miquel (2009a, p. 85). The idea is that by working in second-order logic we obtain a polymorphic type system, that is a system where terms could be associated to more than one type. Since in this paper we adopted the convention to present terms in Curry style, this means that the information concerning types is not present in the terms, and thus polymorphism is not explicitly manifested inside terms – by means of some abstraction operator –, but remains implicit (see Hindley and Seldin 2008, p. 119–120). It is for this reason that the rule \(\forall ^{2}\) elim is not associated to any new operation on terms.
- 29.
As we mentioned at p. 174, processes usually operate on closed terms.
- 30.
Making appeal to projections is for simplicity and shortness of notation. In fact, as we said at p. 174, these operators can by defined in the second-order setting in which Krivine’s realizability is conceived.
- 31.
Notice that we consider to work here with a natural deduction presented in a sequent calculus style. The proof of the proposition can be found in Negri and von Plato (2001, pp. 134–135). In general, in order to prove the ‘only if’ direction, the idea is to replace every
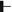 A
i sequent used in the proof of \(\Gamma \)
A
i sequent used in the proof of \(\Gamma \) 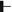 C with an identity sequent A
i
C with an identity sequent A
i 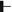 A
i
. While in order to prove the ‘if’ direction, the idea is to apply a cut rule on the A
i
, having
A
i
. While in order to prove the ‘if’ direction, the idea is to apply a cut rule on the A
i
, having 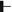 A
i and \(\Gamma,A_{1},\ldots,A_{n}\)
A
i and \(\Gamma,A_{1},\ldots,A_{n}\) 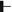 C as premisses.
C as premisses.
- 32.
A Church numeral is a representation in pure \(\lambda\)-calculus of natural numbers, such that a given natural number n corresponds to the \(\lambda\)-term
$$\displaystyle{\lambda f.\lambda x.\mathop{\underbrace{ (f)\ldots (f)}}\limits _{n\text{ times}}x}$$For more details see Sørensen and Urzyczyn (2006, p. 20).
- 33.
Notice that χ does not directly realize ACC. What can be proved instead is that there exists a function \(F: \mathbb{N}^{k+2} \rightarrow \wp (\Sigma )\), with \(\wp (\Sigma )\) the power set of the set of stacks \(\Sigma \), such that:
$$\displaystyle{\chi \Vdash \forall x(\forall y(Nat(y) \rightarrow A(x,F(x,y))) \rightarrow \forall Y (A(x,Y )))}$$where \(Nat(y) \equiv \forall X(X(0) \wedge \forall x(X(x) \rightarrow X(s(x))) \rightarrow X(y))\). It is then easy to show that the term \(\lambda z(z)\chi\) realizes what can be called the intuitionistic countable choice axiom:
$$\displaystyle{(\mathbf{IACC})\quad \exists U\forall x(\forall y(Nat(y) \rightarrow A(x,U(x,y))) \rightarrow \forall Y A(x,Y ))}$$where Y is a k-ary second-order variable, U a k + 2-ary second-order variable, and A(x, Y ) is any arbitrary formula not containing U free. In order to realize ACC it is sufficient to show that ACC can be obtained from IACC by means of (i) logical equivalences, (ii) the least number principle, and (iii) the principle of extensionality for functions (see Miquel 2009b, §§ 8.1, 8.2.). It is by performing these deductive steps that an essential appeal to classical logic is made.
- 34.
A similar kind of blindness with respect to proof-structure is advocated by Kreisel (1951, pp. 155–156, note 1) when he compares his unwinding program with Brouwer’s constructivism.
- 35.
References
Ariola, Z., Herbelin, H., & Sabry, A. (2007). A proof-theoretic foundation of abortive continuations. Higher-Order and Symbolic Computation, 20, 403–429.
van Atten, M. (2014). The development of intuitionistic logic. In E. N. Zalta (Ed.), The Stanford encyclopedia of philosophy. http://plato.stanford.edu/archives/spr2014/entries/intuitionistic-logic-development/.
Barnes, J. (1993). Commentary to Aristotle. In Posterior analytics (pp. 81–271). Oxford: Clarendon.
Bonnay, D. (2002). Le contenu computationnel des preuves: No-counterexemple interpretation et spécification des théorèmes de l’arithmétique. Master thesis, Université Paris 7 Paris Diderot.
Bonnay, D. (2004). Preuves et jeux sémantiques. Philosophia Scientiæ, 8, 105–123.
Bonnay, D. (2007). Règles et signification: le point de vue de la logique classique. In J. B. Joinet (Ed.), Logique, dynamique et cognition (pp. 213–231). Paris: Publications de la Sorbonne.
Boyer, J., & Sandu, G. (2012). Between proof and truth. Synthese, 187, 821–832.
Coquand, T. (2014). Recursive functions and constructive mathematics. In M. Bourdeau & J. Dubucs (Eds.), Constructivity and computability in historical and philosophical perspective (pp. 159–167). Berlin: Springer.
Dowek, G., & Miquel, A. (2007). Cut elimination for Zermelo set theory (manuscript).
Dowek, G., & Werner, B. (2005). Arithmetic as a theory modulo. In J. Giesel (Ed.), Term rewriting and applications (Lecture notes in computer science, Vol. 3467, pp. 423–437). Berlin: Springer.
Dowek, G., Hardin, T., & Kirchner, C. (2003). Theorem proving modulo. Journal of Automated Reasoning, 31, 33–72.
Dummett, M. (1963/1978). Realism. In M. Dummett (Ed.), Truth and other enigmas (pp. 145–165). London: Duckworth.
Dummett, M. (1973/1978). The philosophical basis of intuitionistic logic. In M. Dummett (Ed.), Truth and other enigmas (pp. 215–247). London: Duckworth.
Dummett, M. (1977). Elements of intuitionism. Oxford: Clarendon.
Dummett, M. (1991). The logical basis of metaphysics. London: Duckworth.
Dummett, M. (1998). Truth from the constructive standpoint. Theoria, 64, 122–138.
Fine, K. (2014). Truth-maker semantics for intuitionistic logic. Journal of Philosophical Logic, 43, 549–577.
Friedman, H. (1978). Classically and intuitionistically provably recursive functions. In G. H. Müller & D. Scott (Eds.), Higher set theory (pp. 21–27). Berlin: Springer.
Giannini, P., & Ronchi Della Rocca, S. (1988). Characterization of typings in polymorphic type discipline. In Logic in computer science (pp. 61–70). Los Alamitos: IEEE Computer Society Press.
Girard, J.-Y. (1989). Geometry of interaction I: Interpretation of system F. In R. Ferro, C. Bonotto, S. Valentini, & A. Zanardo (Eds.), Logic colloquium ’88 (pp. 221–260). Amsterdam: North-Holland.
Girard, J.-Y. (2001). Locus solum: From the rules of logic to the logic of rules. Mathematical Structures in Computer Science, 11, 301–506.
Goodman, N. (1970). A theory of constructions equivalent to arithmetic. In A. Kino, J. Myhill, & R. E. Vesley (Eds.), Intuitionism and proof theory (pp. 101–120). Amsterdam: North-Holland.
Goodman, N. (1973a). The faithfulness of the interpretation of arithmetic in the theory of constructions. The Journal of Symbolic Logic, 38, 453–459.
Goodman, N. (1973b). The arithmetic theory of constructions. In A. R. D. Mahtias, & H. Rogers (Eds.), Cambridge summer school in mathematical logic (pp. 274–298). Berlin: Springer.
Guillermo, M., & Miquel, A. (2014). Specifying Peirce’s law in classical realizability. Mathematical Structures in Computer Science. Online first. doi:http://dx.doi.org/10.1017/S0960129514000450.
Griffin, T. (1990). A formulae-as-types notion of control. In Proceedings of the 17th ACM symposium on principles of programming languages, San Francisco (pp. 47–58). ACM.
Heyting, A. (1962). After thirty years. In E. Nagel, P. Suppes, & A. Tarski (Eds.), Logic, methodology and philosophy of science. Proceedings of the 1960 international congress (pp. 194–197). Stanford: Stanford University Press.
Hilbert, D. (1926). Über das Unendliche. [On the infinite] English trans. E. Putnam & G. J. Massey. In P. Benacerraf & H. Putnam (Eds.), Philosophy of mathematics: Selected writings (2nd ed., pp. 183–201). Cambridge: Cambridge University Press, 1983.
Hilbert, D., & Bernays, P. (1934). Grundlagen der Mathematik I. Berlin: Springer.
Hindley, J. R., & Seldin, J. P. (2008). Lambda-calculus and combinators: An introduction. Cambridge: Cambridge University Press.
Hintikka, J. (1996). The principles of mathematics revisited. Cambridge: Cambridge University Press.
Kleene, S. (1945). On the interpretation of intuitionistic number theory. Journal of Symbolic Logic, 10, 109–124.
Kleene, S. (1973). Realizability: A retrospective survey. In A. R. D. Mathias & H. Rogers (Eds.), Cambridge summer school in mathematical logic (pp. 95–112). Berlin: Springer.
Kreisel, G. (1951). On the interpretation of non-finitist proofs. Part I. The Journal of Symbolic Logic, 16(4), 241–267.
Kreisel, G. (1952). On the interpretation of non-finitist proofs: Part II. Interpretation of number theory. The Journal of Symbolic Logic, 17(1), 43–58.
Kreisel, G. (1960). Ordinal logics and the characterization of informal notions of proof. In J. A. Todd (Ed.), Proceedings of the international congress of mathematicians, Edinburgh, 14–21 Aug 1958 (pp. 289–299). Cambridge: Cambridge University Press.
Kreisel, G. (1962). Foundations of intuitionistic logic. In T. Nagel, P. Suppes, & A. Tarski (Eds.), Logic, methodology and philosophy of science (pp. 98–210). Stanford: Stanford University Press.
Kreisel, G. (1965). Mathematical logic. In T. Saaty (Ed.), Lectures on modern mathematics (Vol. 3, pp. 95–195). New York: Wiley.
Kreisel, G. (1972). Which number theoretic problems can be solved in recursive progressions on \(\Pi _{1}^{1}\)-paths through O? The Journal of Symbolic Logic, 37, 311–334.
Kreisel, G. (1973). Perspectives in the philosophy of pure mathematics. In P. Suppes, L. Henkin, A. Joja, & G. C. Moisil (Eds.), Logic, methodology and philosophy of science IV: Proceedings of the fourth international congress for logic, methodology and philosophy of science, Bucharest, 1971 (pp. 255–277). Amsterdam: North-Holland.
Krivine, J.-L. (1994). Classical logic, storage operators and second-order lambda calculus. Annals of Pure and Applied Logic, 68, 53–78.
Krivine, J.-L. (2003). Dependent choice, ‘quote’ and the clock. Theoretical Computer Science, 308, 259–276.
Krivine, J.-L. (2009). Realizability in classical logic. Panoramas et Synthèses, 27, 197–229.
Krivine, J.-L. (2012). Realizability algebras II: New models of ZF + DC. Logical Methods in Computer Science, 8, 1–28.
Martin-Löf, P. (1970). Notes on constructive mathematics. Stockholm: Almqvist & Wiksell.
Martin-Löf, P. (1991). A path from logic to metaphysics. In G. Corsi & G. Sambin (Eds.), Atti del Congresso “Nuovi problemi della logica e della filosofia della scienza” (Vol. 2, pp. 141–149). Bologna: CLUEB.
Miquel, A. (2009a). De la formalisation des preuves à l’extraction de programmes. Habilitation thesis, Université Paris 7 Paris Diderot.
Miquel, A. (2009b). Classical realizability with forcing and the axiom of countable choice (manuscript).
Naibo, A. (2013). Le statut dynamique des axiomes. Des preuves aux modèles. PhD thesis, Université Paris 1 Panthéon-Sorbonne.
Naibo, A., Petrolo, M., & Seiller, T. (2015, in press). On the computational meaning of axioms. In A. Napomuceno, O. Pombo, & J. Redmond (Eds.), Epistemology, knowledge and the impact of interaction. Berlin: Springer.
Negri, S., & von Plato, J. (2001). Structural proof theory. Cambridge: Cambridge University Press.
Negri, S., & von Plato, J. (2011). Proof analysis: A contribution to Hilbert’s last problem. Cambridge: Cambridge University Press.
Oliva, P., & Streicher, T. (2008). On Krivine’s realizability interpretation of classical second-order arithmetic. Fundamenta Informaticæ, 84, 207–220.
Parigot, M. (1992). λ μ-calculus: An algorithmic interpretation of classical natural deduction. Logic Programming and Automated Deduction, 624, 190–201.
Parsons, C. (1972). On n-quantifier induction. The Journal of Symbolic Logic, 37, 466–482.
Parsons, C. (2008). Mathematical thought and its objects. Cambridge: Cambridge University Press.
von Plato, J. (2013). Elements of logical reasoning. Cambridge: Cambridge University Press.
Prawitz, D. (1977). Meaning and proofs: On the conflict between classical and intuitionistic logic. Theoria 43, 2–40.
Prawitz, D. (2006). Meaning approached via proofs. Synthese, 148, 507–524.
Rieg, L. (2014). On forcing and classical realizability. PhD thesis, École Normale Supérieure de Lyon.
Schroeder-Heister, P. (1984). A natural extension of natural deduction. Journal of Symbolic Logic, 49, 1284–1300.
Seiller, T. (2014). Interaction graphs: Graphings. arXiv:1405.6331.
Seldin, J. (1989). Normalization and excluded middle I. Studia Logica, 48, 193–217.
Sørensen, M. H., & Urzyczyn, P. (2006). Lectures on the Curry-Howard isomorphism. Amsterdam: Elsevier.
Sundholm, G. (1983). Constructions, proofs and the meaning of logical constants. Journal of Philosophical Logic, 12, 151–172.
Sundholm, G. (1986). Proof theory and meaning. In D. Gabbay & F. Guenthner (Eds.), Handbook of philosophical logic (Vol. 3, pp. 471–506). Dordrecht: Reidel.
Sundholm, G. (1994). Vestiges of realism. In B. McGuinness & G. Oliveri (Eds.), The philosophy of Michael Dummett (pp. 137–165). Dordrecht: Kluwer.
Sundholm, G. (2014). Constructive recursive functions, Church’s thesis, and Brouwer’s theory of the creating subject: Afterthoughts on a Parisian joint session. In M. Bourdeau & J. Dubucs (Eds.), Constructivity and computability in historical and philosophical perspective (pp. 1–35). Berlin: Springer.
Tait, W. W. (1981). Finitism. The Journal of Philosophy, 78, 524–546.
Tieszen, R. (1992). What is a proof? In M. Detlefsen (Ed.), Proof, logic and formalization (pp. 57–76). London: Routledge.
Troelstra, A. S. (1998). Realizability. In S. R. Buss (Ed.), Handbook of proof theory (pp. 407–473). Amsterdam: Elsevier.
Troelstra, A. S., & van Dalen, D. (1988). Constructivism in mathematics (Vol. 1). Amsterdam: North-Holland.
Zach, R. (2015). Hilbert’s program. In E. N. Zalta (Ed.), The Stanford encyclopedia of philosophy. http://plato.stanford.edu/archives/spr2015/entries/hilbert-program/.
Acknowledgements
We would like to thank Marco Panza for the interest he manifested in our work and Luiz Carlos Pereira for the valuable discussions about the verificationist aspects of classical logic. This work has been partially funded by the French-German ANR-DFG project BeyondLogic (ANR-14-FRAL-0002) and by the CAPES-COFECUB project Preuve, démonstrations et représentation (Sh813–14).
Author information
Authors and Affiliations
Corresponding author
Editor information
Editors and Affiliations
Rights and permissions
Copyright information
© 2016 Springer International Publishing Switzerland
About this chapter
Cite this chapter
Naibo, A., Petrolo, M., Seiller, T. (2016). Verificationism and Classical Realizability. In: Başkent, C. (eds) Perspectives on Interrogative Models of Inquiry. Logic, Argumentation & Reasoning, vol 8. Springer, Cham. https://doi.org/10.1007/978-3-319-20762-9_9
Download citation
DOI: https://doi.org/10.1007/978-3-319-20762-9_9
Publisher Name: Springer, Cham
Print ISBN: 978-3-319-20761-2
Online ISBN: 978-3-319-20762-9
eBook Packages: Religion and PhilosophyPhilosophy and Religion (R0)





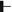 A
i sequent used in the proof of
A
i sequent used in the proof of