Lepton anomaly from QED diagrams with vacuum polarization insertions within the Mellin–Barnes representation

Abstract

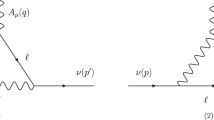
The contributions to the anomalous magnetic moment of the lepton L ($L=e\, \mu$ or $\tau$) generated by a specific class of QED diagrams are evaluated analytically up to the eighth order of the electromagnetic coupling constant. The considered class of the Feynman diagrams involves the vacuum polarization insertions into the electromagnetic vertex of the lepton L up to three closed lepton loops. The corresponding analytical expressions are obtained as functions of the mass ratios $r=m_l/m_L$ in the whole region $0< r < \infty$. Our consideration is based on a combined use of the dispersion relations for the polarization operators and the Mellin–Barnes integral transform for the Feynman parametric integrals. This method is widely used in the literature in multi-loop calculations in relativistic quantum field theories. For each order of the radiative correction, we derive analytical expressions as functions of r, separately at $r<1$ and $r>1$. We argue that in spite of the obtained explicit expressions in these intervals which are quite different, at first glance, they represent two branches of the same analytical function. Consequently, for each order of corrections there is a unique analytical function defined in the whole range of $r\in (0,\infty )$. The results of numerical calculations of the fourth-, sixth- and eighth-order corrections to the anomalous magnetic moments of leptons ($L=e,\mu , \tau$) with all possible vacuum polarization insertions are represented as functions of the ratio $r=m_l/m_L$. Whenever pertinent, we compare our analytical expressions and the corresponding asymptotical expansions with the known results available in the literature.

Appendix A: Some useful relations

Direct employment of the Cauchy residue theorem to the integrals, Eqs. (40), (50), (53), (57), (59) and (68) results in expressions containing a variety of special functions, including polygammas $\psi ^{(m)}(n)$, polylogarithms $\textrm{Li}_n(r)$, generalized harmonic functions $H_n^{(m)}$, Hurwits–Lerch transcendent $\Phi (r,n,a)$, etc. Using the below relations, the number of necessary special functions can be essentially reduced. This allows one to reconcile explicitly our results to the ones known in the literature and reported in different forms with different special functions, cf. Refs. [23, 32, 56]. Also, by using the appropriate properties of the remaining functions for $r<1$ and $r>1$, one can express the corresponding coefficients $A_2(r<1)$ through the coefficients $A_2(r>1)$, which allows one to assert that there exist, for each Feynman diagram, a common analytical function valid in the whole interval $(0\,<\,r\,\infty )$.

$$\begin{aligned}{} {} \Phi (r,2,1/2) &= \frac{2}{\sqrt{r}} \left[ { \textrm{Li}_2 } (\sqrt{r}) - { \textrm{Li}_2 } (-\sqrt{r}) \right]; \nonumber \\{} {} \Phi (r,2,3/2) &=-\frac{4}{r} +\frac{2}{r\sqrt{r}} \left[ { \textrm{Li}_2 } (\sqrt{r}) - { \textrm{Li}_2 } (-\sqrt{r}) \right] ; \nonumber \\{} {} \Phi (r,2,5/2) &=-\frac{4}{9r} - \frac{4}{r^2} +\frac{2}{r^2\sqrt{r}} \left[ { \textrm{Li}_2 } (\sqrt{r}) - { \textrm{Li}_2 } (-\sqrt{r}) \right] ; \nonumber \\{} {} { \textrm{Li}_2 } (1-r)+ { \textrm{Li}_2 } \left( 1-\frac{1}{r}\right) &=-\frac{1}{2} \ln ^2(r); \quad \mathrm{(r>0)}; \nonumber \\{} {} { \textrm{Li}_2 } (r) + { \textrm{Li}_2 } (1/r) &= -\frac{\pi ^2}{6} - \frac{1}{2} \ln ^2(-r); \quad \mathrm{(r>1)}; \nonumber \\{} {} \mathrm{{Li}_3} (r) - \mathrm{{Li}_3} (1/r) &= -\frac{\pi ^2}{6}\ln (-r) - \frac{1}{6} \ln ^3(-r); \quad \mathrm{(r>1)} \nonumber \\{} {} \mathrm{{Li}_3} (r) - \mathrm{{Li}_3} (1/r) &= \frac{\pi ^2}{6}\ln (-1/r) + \frac{1}{6} \ln ^3(-1/r); \quad \mathrm{(r<1)} \nonumber \\{} {} { \textrm{Li}_4 } (r) + { \textrm{Li}_4 } (1/r) &=-\frac{7\pi ^4}{360} -\frac{\pi ^2}{12}\ln^2 (-r) - \frac{1}{24} \ln ^4(-r); \quad \mathrm{(r>1)} \nonumber \\{} {} \mathrm{Li_2} \left( \frac{1-r}{1+r} \right) - \mathrm{Li_2}\left( - \frac{1-r}{1+r} \right) &= \mathrm{Li_2}(-r) - \mathrm{Li_2}(r) +\bigg (\ln (1+r)\ln (r) - \ln (1-r)\bigg )\ln (r) + \frac{\pi ^2}{4}; \nonumber \\{} {} \mathrm{Li_n}(r) + \mathrm{Li_n}(-r) &= \frac{1}{2^{n-1}} \mathrm{Li_n}(r^2). \end{aligned}$$

(A1)

$$\begin{aligned}{} & {} \quad { \mathrm{arctanh}(r)}=\frac{1}{2}\bigg [ \ln (1+r)-\ln (1-r)\bigg ]; \end{aligned}$$

(A2)

$$\begin{aligned}{} & {} \quad \mathrm{H_n^{(1)}} = \psi ^{(1)}(n+1)+\gamma ; \quad \textrm{H}_n^{(2)}=\frac{\pi ^2}{6}-\psi ^{(1)}(n+1), \end{aligned}$$

(A3)

where $\mathrm{Li_n}(r)$, $\Phi (r,s,a)$, $\mathrm{H_n^{(m)}}$ and $\psi ^{(m)}(n)$ are the polylogarithm, Lerch transcendent, generalized harmonic number and Euler polygamma functions, respectively.