Scaling properties of exclusive vector meson production cross section from gluon saturation

Abstract

It is already known from phenomenological studies that in exclusive deep-inelastic scattering off nuclei there appears to be a scaling behavior of vector meson production cross section in both nuclear mass number, A, and photon virtuality, $Q^{2}$, which is strongly modified due to gluon saturation effects. In this work we continue those studies in a realistic setup based upon using the Monte Carlo event generator Sartre. We make quantitative predictions for the kinematics of the Electron-Ion Collider, focusing on this A and $Q^{2}$ scaling picture, along with establishing a small region of squared momentum transfer, t, where there are signs of this scaling that may potentially be observed at the EIC. Our results are represented as pseudo-data of vector meson production diffractive cross section and/or their ratios, which are obtained by parsing data collected by the event generator through smearing functions, emulating the proposed detector resolutions for the future EIC.

Appendices

Appendix A: Calculating diffractive differential cross sections

An extremely complicated task is to calculate and generate total cross sections, for which one has to evaluate complex four-dimensional integrals at each phase-space point. But Sartre uses an approach based on computing the first and second moments of the scattering amplitudes separately, and then stores the results in three-dimensional look-up tables, in terms of $Q^{2}$, $W^{2}$ and t independent variables. The ultimate outcome is a set of four look-up tables for each nuclear species, each final-state vector meson (and DVCS photon), each polarization, and each dipole model (either IPSat or IPNonSat).

$$\begin{aligned}&\bigg<\mathcal {A}_{T}(Q^2, W^2, t)\bigg>_{\Omega } ,\quad \bigg<\mathcal {A}_{L}(Q^2, W^2, t)\bigg>_{\Omega } , \nonumber \\&\bigg<|\mathcal {A}_{T}(Q^2, W^2, t)|^2\bigg>_{\Omega } ,\quad \bigg <|\mathcal {A}_{L}(Q^2, W^2, t)|^2\bigg >_{\Omega } , \end{aligned}$$

(A.1)

These look-up tables contain all the physics information from both dipole models. The program also provides tables for calculating the phenomenological corrections described in Sect. 2.2.2.

The master equation of Sartre is the total diffractive differential cross section, which for electron-nucleus scattering has the following form:

$$\begin{aligned} \left( \frac{\mathrm{d}^{3}\sigma ^{\gamma ^{*}A\,\rightarrow \,V A}}{\mathrm{d}Q^{2}\,\mathrm{d}W^{2}\,\mathrm{d}t} \right)_{\mathrm{tot. diff.}}= & \sum _{T, L}\frac{R_{g}^{2}(1 + B^{2})}{16\pi }\frac{\mathrm{d}\Gamma _{T, L}}{\mathrm{d}Q^{2}\,\mathrm{d}W^{2}}\nonumber \\&\times \,\bigg < \left| \mathcal {A}_{T,L}^{\gamma ^{*}A\,\rightarrow \,V A}(x_{I\!\!P},Q^{2},t,\Omega )\right| ^{2} \bigg >_{\Omega } , \end{aligned}$$

(A.2)

where B is given in Eq. (18), and $R_{g}$ in Eq. (19). The quantity $\Gamma _{T, L}$ is the flux of transversely T and longitudinally L polarized virtual photons [82,83,84,85,86], given by

$$\begin{aligned}&\Gamma _{T} = \frac{\alpha }{2\pi } \left( \frac{1 + (1 - y)^{2}}{y\,Q^{2}} \right) ,\nonumber \\&\Gamma _{L} = \frac{\alpha }{2\pi } \left( \frac{2(1 - y)}{y\,Q^{2}} \right) , \end{aligned}$$

(A.3)

where y is the inelasticity defined as the fraction of the electron’s energy lost in the nucleon rest frame. The averaging over configurations $\Omega$ is defined as

$$\begin{aligned}&\bigg < \left| \mathcal {A}_{T,L}^{\gamma ^{*}A\,\rightarrow \,V A}(x_{I\!\!P},Q^{2},t,\Omega )\right| ^{2} \bigg >_\Omega \nonumber \\&\quad = \frac{1}{N_{\mathrm{max}}}\sum _{j=1}^{N_{\mathrm{max}}} \left| \mathcal {A}_{T,L}^{\gamma ^{*}A\,\rightarrow \,V A} (x_{I\!\!P},Q^{2},t,\Omega _{j})\right| ^{2} , \end{aligned}$$

(A.4)

where $N_{\mathrm{max}}$ is the number of configurations. If it is large enough, then the sum in Eq. (A.4) converges to a true average. It is already shown in [50] that $2 \times 500$ configurations, 500 for T polarized $\gamma ^{*}$ and 500 for L polarized $\gamma ^{*}$, give a good convergence. Such that there are 1000 such integrals for each $(Q^{2}, W^{2}, t)$ phase-space point.

The photon flux may emanate from electrons, as in the case of $e+p$ and $e+A$ scatterings, however, it may be also radiated from protons or nuclei [87], as in the case of $A+A$ or $p+A$ UPC. A phase-space point together with a given beam energy fully determines the final state of a produced vector meson, except for its azimuthal angle, which is distributed uniformly.

The second moment of the amplitude in Eq. (A.4) for the nucleon configurations $\Omega _{j}$ can be calculated based upon [49, 50]:

$$\begin{aligned} \bigg < \left| \mathcal {A}_{T,L}^{\gamma ^{*}A\,\rightarrow \,V A}(x_{I\!\!P},Q^{2},t,\Omega ) \right| ^{2}\bigg >_{\Omega }= & \sum _{j=1}^{N_{\mathrm{max}}}\,\left| \int \mathrm{d}^{2}{} \mathbf{r_{T}} \int \mathrm{d}^{2}{\mathbf {b_{T}}}\int \frac{\mathrm{d} z}{4\pi } \,\times \right. \nonumber \\&\times \,\left. \left[ \left( \Psi ^{*}\Psi _{V} \right) _{T,L}\!(Q^{2},{\mathbf {r_{T}}}, z)\right] J_{0}([1 - z]r_{T}\Delta ) \right. \nonumber \\&\quad \times \,\left. e^{-i{\mathbf {b_{T}}}\cdot {\varvec{\Delta }}}\, \frac{\mathrm{d}\sigma _{q\bar{q}}^{A}}{\mathrm{d}^{2}{\mathbf {b_{T}}}}(x_{I\!\!P}, {\mathbf {r_{T}}}, {\mathbf {b_{T}}}, \Omega _{j})\right| ^{2} , \end{aligned}$$

(A.5)

where the last term $\mathrm{d}\sigma _{q\bar{q}}^{A}/\mathrm{d}^{2}{\mathbf {b_{T}}}$ is defined in Eq. (16).

Thus, Eqs. (A.2) and (A.5) determine the total diffractive differential cross section. Its coherent part is given by

$$\begin{aligned} \left( \frac{\mathrm{d}^{3}\sigma ^{\gamma ^{*}A\,\rightarrow \,V A}}{\mathrm{d}Q^{2}\,\mathrm{d}W^{2}\,\mathrm{d}t} \right) _{\mathrm{coh. diff.}}= & \sum _{T, L} \frac{R_{g}^{2}(1 + B^{2})}{16\pi }\frac{\mathrm{d}\Gamma _{T, L}}{\mathrm{d}Q^{2}\,\mathrm{d}W^{2}}\nonumber \\&\times \,\left| \bigg <\mathcal {A}_{T,L}^{\gamma ^{*}A\,\rightarrow \,V A}(x_{I\!\!P},Q^{2},t,\Omega )\bigg >_{\Omega } \right| ^{2} , \end{aligned}$$

(A.6)

For the first moment of the amplitude, the integral to calculate will be

$$\begin{aligned} \,\left| \bigg<\mathcal {A}_{T,L}^{\gamma ^{*}A\,\rightarrow \,V A}(x_{I\!\!P},Q^{2},t,\Omega )\bigg>_{\Omega } \right| ^{2}= & \left| \int \mathrm{d}^{2}{} \mathbf{r_{T}} \int \mathrm{d}^{2}{\mathbf {b_{T}}}\right. \nonumber \\&\left. \times \,\int \frac{\mathrm{d} z}{4\pi } \left[ \left( \Psi ^{*}\Psi _{V} \right) _{T,L}\!(Q^{2},{\mathbf {r_{T}}}, z)\right] J_{0}([1 - z]r_{T}\Delta ) \right. \nonumber \\&\left. \times \,J_{0}(b_{T}\Delta ) \bigg <\frac{\mathrm{d}\sigma _{q\bar{q}}^{A}}{\mathrm{d}^{2}{\mathbf {b_{T}}}}(x_{I\!\!P}, {\mathbf {r_{T}}}, {\mathbf {b_{T}}}, \Omega )\bigg >_{\Omega } \right| ^{2} , \end{aligned}$$

(A.7)

where the average $< \mathrm{d}\sigma _{q\bar{q}}^{A}/\mathrm{d}^{2}{\mathbf {b_{T}}}>_{\Omega }$ in the last term is defined in Eq. (17).

Thereby, as in Eq. (14), the incoherent part of the total diffractive differential cross section is taken to be the difference between the total and coherent cross sections:

$$\begin{aligned} \left( \frac{\mathrm{d}^{3}\sigma ^{\gamma ^{*}A\,\rightarrow \,V A}}{\mathrm{d}Q^{2}\,\mathrm{d}W^{2}\,\mathrm{d}t} \right) _{\mathrm{incoh. diff.}}&= \left( \frac{\mathrm{d}^{3}\sigma ^{\gamma ^{*}A\,\rightarrow \,V A}}{\mathrm{d}Q^{2}\,\mathrm{d}W^{2}\,\mathrm{d}t} \right)_{\mathrm{tot. diff.}} \nonumber \\ &\quad - \,\left( \frac{\mathrm{d}^{3}\sigma ^{\gamma ^{*}A\,\rightarrow \,V A}}{\mathrm{d}Q^{2}\,\mathrm{d}W^{2}\,\mathrm{d}t} \right) _{\mathrm{coh. diff.}} . \end{aligned}$$

(A.8)

The incoherent part directly gives the probability for the nuclear breakup.

Appendix B: Error analysis of the pseudo-data using the EIC Detector Handbook

We use Sartre 1.33, an exclusive event generator, to produce vector meson production data. A user-edited runcard is called at the initialization of a simulation to set beam energies, decay modes, and ranges on event kinematics such as $Q^{2}$ and |t|. The generator outputs the kinematics of final-state particles, such as their momentum and pseudorapidity. Additionally, important event information such as $Q^{2}$, ${x_{\mathbb {P}}}$, and y are recorded. We refer to the event generator output as truth data. This data, while unobtainable in a physical experiment, allows us to perform perfect event identification and to create pseudo-data. Using detector resolutions outlined in the EIC Detector Handbook [59], true particle kinematics are smeared to create pseudo-data. For instance, according to the handbook, the barrel ($|\eta |<1$) tracking resolution for electrons is $\sigma _p/p = 0.05\%p + 0.5\%$. By having such resolutions written out explicitly for the relevant final-state particles from vector meson production simulations allows us to calculate pseudo-data based on the handbook’s projections. The smearing functions immediately produce smeared kinematics, such as momentum and energy. Event-by-event, we manually smear the final-state kinematics according to these functions. Subsequently, the pseudo-data reconstruction of the scattered electron is used to determine event kinematics such as $Q^{2}$. The event |t| is the only quantity which we smear independently. One of the immediate effects of generating pseudo-data is the reduction of statistics. Final-state particles, which exit at pseudorapidities beyond the coverage of the detector system, render the exclusive event unrecoverable. Systematic errors introduced through particle/event mis-identification are not accounted for when analyzing the pseudo-data. Instead, we opt to use true, event generator information to identify the scattered electron and decay particles. Lastly, we use truth information to separate coherent vector meson production from the incoherent one, as well as events with transversely polarized virtual photons from longitudinally polarized virtual photons.

After the pseudo-data are generated, we are left with the reconstructed particle and event kinematics for a pile of production events. Then, the data are stored event-by-event in a ROOT TTree, which is read and analyzed to produce plots. From the TTree, the analyzed data are used to fill ROOT histogram objects. These histogram objects, initially storing the number of events per bin, are scaled in a variety of ways. This includes scaling to the correct decay mode branching ratios, scaling to the desired luminosity, and scaling by bin size (to create differential cross sections). What follows is a detailed look at how the pseudo-data are analyzed, how this analysis is piped into histograms, how they are scaled, and how the error is propagated.

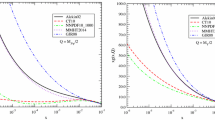
Pseudo-data and error propagation for Figs. 3 and 4. After the pseudo-data are generated, the following cuts are placed event-by-event:

• Reconstructed ${x_{\mathbb {P}}}< 0.01$.

• Reconstructed $1.0< Q^{2} < 10.0\,\mathrm{GeV}^{2}$.

• True event coherent vector meson production.

• Reconstructed final-state particle pseudorapidity between $-3.5< \eta < 3.5$¹⁷.

• Reconstructed final-state particle momentum greater than 1 GeV.

Then, using true event |t| information outputted by Sartre, we generate the reconstructed |t| using the Gaus() function from the ROOT TRandom class. For each event, we select a random variable from a Gaussian centered at true |t| with spread equal to true $|t|\times tsmear$. This random variable represents the reconstructed |t| of the event. The quantity $tsmear$ is changed depending on our desired resolution in |t|. It ranges from 0 all the way to $0.3\,\mathrm{GeV}^{2}$, or is parameterized by the “Method L” in [30]. Once all of the events have been parsed through and either added or discarded, a TGraphErrors object is created. This object will pick up information from the filled histogram and produce a plot.

Below are the individual modifications we make to the entries of the filled histogram, given in order. Beforehand, we define $\mathrm {N}_{\mathrm {entries}}$ to be the number of total entries in the histogram, and w to be the amount of entries in an arbitrary bin.

• 1. First, we multiply each bin of the histogram by the quantity $\sigma \cdot L/A$, where $\sigma$ is the total event cross section (in nanobarns) outputted by Sartre, and L is the integrated luminosity. We also divide by the atomic number A of a nucleus to isolate the scattering cross section of a single nucleon for a given nucleus beam.

• 2. Then, we multiply each bin by the branching ratio (BR) of the truth decay mode.

• 3. Next, we divide each bin by $\mathrm {N}_{\mathrm {entries}}$. And now for each bin, we are left with the number of events expected in an experiment with integrated luminosity L, within the reconstructed |t| bin range:

  $$\begin{aligned} \mathrm {N}_{\mathrm {expected}} \equiv w' = \frac{\mathrm {BR} \times L\times \sigma \times w}{\mathrm {N}_{\mathrm {entries}} \times A}. \end{aligned}$$

  (B.9)

• 4. We further calculate the statistical uncertainty for each bin as the square root of its entries, $\sigma _{w'} = \sqrt{w'}$.

• 5. Afterward, we divide each bin quantity w by the branching ratio, the bin width $\Delta t$, and integrated luminosity. We repeat this for $\sigma _{w}$ as well.

  $$\begin{aligned}&\frac{d\sigma }{dt}{\text{[pseudo-data] }} = \frac{A}{\mathrm {BR} \times \Delta t \times L} \mathrm {N}_{\mathrm {expected}} ,\nonumber \\&\frac{d\sigma }{dt}{\text{[pseudo-data } \text{ statistical } \text{ uncertainty] }}= \frac{A}{\mathrm {BR}\times \Delta t \times L} \sqrt{\mathrm {N}_{\mathrm {expected}}} . \end{aligned}$$

  (B.10)

This step completes the pseudo-data and error analysis for Figs. 3 and 4.

Fig. 16

Cross-sectional conversion scheme for obtaining the scaling plots in Figs. 5, 6, 7, 8, 9, 10, 11, 12, 13, 14 and 15 based upon Sartre 1.33 simulations.

Pseudo-data and error propagation for Figs. 5, 6, 7, 8, 9, 10, 11, 12, 13, 14 and 15. The analysis of these figures differ from the above method in subtle ways.¹⁸ For each collision type ($e+p$ and/or $e+A$), vector meson produced ($J/\Psi$ or $\phi$), and dipole model used (IPSat or IPNonSat), we generate $N = 10^{8}$ exclusive events using Sartre. At the beginning of the simulation, we save the total cross section of the simulation’s phase space. Using this cross section, the projected EIC luminosities ($100\,\mathrm{fb}^{-1}/A$ for $e+p$ at $10 \times 100\,\mathrm{GeV}$, $10\,\mathrm{fb}^{-1}/A$ for $e+A$ at $10 \times 110\,\mathrm{GeV}$), and the branching ratios of the studied vector mesons’ decay modes, we calculate the number of expected events produced at the EIC. This number, we call n, can be divided by N to tell us how much we should scale our simulation size to reflect the specific luminosity. We call this parameter $\text{ Scale } = n/N$.

Event by event, we use the true event generator output to determine if the event was coherent or incoherent. Skipping incoherent events, we then store the virtual photon polarization for an event, also given by Sartre. We place a hard cut on the true event y to minimize the impact of poor smearing in that kinematic regime. By using detector uncertainties projected in [59], we smear the energy, momentum, and polar angle of the final-state electron and decay products. We skip events in which any one of these three-final state particles true pseudorapidity falls outside the reach of the detector system’s coverage. After the smearing is completed, we use the true beam energy of the incoming electron and nuclear/proton target to calculate event kinematics such as $Q^{2}$, y, and ${x_{\mathbb {P}}}$. We smear the event’s t in a way described in Sect. 3, skipping events with $y < 0.05$.

When filling our histograms, we weigh the fill by the factor

$$\begin{aligned} \text{ Scale } \times \frac{d{x_{\mathbb {P}}}}{dy} \frac{Q^{m}}{\Gamma _{T, L}} , \end{aligned}$$

(B.11)

where m is determined by the specific $Q^{2}$ scaling being analyzed, the photon flux factor $\Gamma _{T, L}$ depends on the polarization of the event, and the derivative is given by

$$\begin{aligned} \frac{d{x_{\mathbb {P}}}}{dy} = \frac{s \times {x_{\mathbb {P}}}}{M_{V}^{2} + Q^{2} - t} . \end{aligned}$$

(B.12)

where s is the center-of-mass energy squared. The derivative $d{x_{\mathbb {P}}}/dy$ and $\Gamma _{T, L}$ factor effectively divide out the virtual photon flux element of the event cross section, leaving us with (see Fig. 16) a beam-independent cross section $\sigma \left( \gamma ^{*} + p/A\,\rightarrow \,V + p^{\prime }/A^{\prime } \right)$. The scale factor is included to reflect the number of events expected, given the experimental luminosity. If we had run Sartre and generated exactly the number of events expected in a given luminosity, then the Scale would equal 1. Each time a histogram’s bin is filled, ROOT updates the statistical error of the bin to be the summation in quadrature of all the bin’s individual weights.

After all events have been simulated, we divide each bin of each histogram by the factor $\mathrm {BR} \times L/A$. We then divide out by the $Q^{2}$ bin width, ${x_{\mathbb {P}}}$ range, and proper nuclear size $A^{k}$ scaling, which leaves the bin content being equivalent to $A^{-k}\,Q^{m}\,\sigma ^{T, L}$. To obtain the differential cross section, we finally divide by the t binning, which is equal 0.001. The proper nuclear size A scaling and is applied to each histogram depending on x. For the nonzero |t| scaling plots in Figs. 10, 11, 12 and 13, we additionally divide the vertical axis by G(A, t) (see Eq. (33)).

Appendix C. Addendum to Sect. 3.2.1

In this appendix, we show qualitative comparisons between model calculations and simulated pseudo-data on the $A^{2}$ normalized cross-sectional ratio and normalized $A^{2}/Q^{6}$ cross section shown in Sect. 3.2.1, in Figs. 6 and 8, respectively. The pseudo-data are obtained by passing events generated by Sartre through particle kinematics smearing functions with detector acceptance. In these plots, the high ${x_{\mathbb {P}}}$ denotes the range of pseudo-data made in ${x_{\mathbb {P}}}= [0.005 - 0.009]$, and the low ${x_{\mathbb {P}}}$ denotes the range of ${x_{\mathbb {P}}}= [0.001 - 0.005$]. So, Figs. 17 and 18 have their top and bottom panels being the same as in Figs. 6 and 8, respectively. We also display truth curves corresponding to all sets of the shown pseudo-data that are calculated using the IPSat model in Sartre. The “kink” structures seen in the curves around $\sim 3~\mathrm{GeV^{2}}$ in the top high-${x_{\mathbb {P}}}$ plots, which describe the $\phi$ normalized cross-sectional ratio and cross section, partially come from the fact that these curves are actually calculated at fixed value of ${x_{\mathbb {P}}}= 0.008$. This region of ${x_{\mathbb {P}}}$ is close to 0.01, the point at which Sartre’s reliability starts to fail, especially for light vector meson production. Lastly, the low-${x_{\mathbb {P}}}$ curves are calculated at 0.0012.

Fig. 17

In the top and bottom panels, the pseudo-data and labeling are exactly the same as in Fig. 6. In addition, there are also overlaid curves obtained directly from Sartre’s IPSat model, where any detector smearing/resolution and experimental acceptance considerations are excluded in the normalized cross-sectional ratio computations

Fig. 18

In the top and bottom panels, the pseudo-data and labeling are exactly the same as in Fig. 8. The explanation for the overlaid curves is similar as in Fig. 17

Overall, the need for additional reconstruction algorithms is evidenced by these figures. The comparison between the truth curves and pseudo-data should thus at this point stay qualitative. A future analysis will consider bin-by-bin corrections, such as detection efficiency and bin migration, to alleviate the discrepancies between the Monte Carlo and pseudo-data cross-sectional reconstructions. Although one may expect these systematic effects to “divide themselves out” in the ratio plots, the differing center-of-mass energies planned for the $e+p$ and $e+A$ runs at the future EIC makes this assumption false. During this paper’s composition, the EIC collaboration has begun narrowing down a single detector design for the first interaction region. Coined EPIC, the detector system and relevant software is currently in development, making our future analysis of detector effects for the continuation of the study in this paper extremely timely.