High-precision measurement of tiny Doppler frequency shifts based on quantum weak measurement with energy recycling

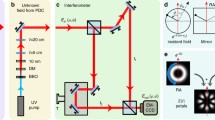
Abstract

It is of great significance to measure tiny Doppler frequency shifts precisely, for its importance in velocity measurement. However, the precision of classical measurement schemes for tiny Doppler frequency shifts is restricted by the shot noise limit. The emergence of quantum weak measurement can significantly reduce technical noise compared with classical measurements. Here, a scheme to realize tiny Doppler measurement by using quantum weak measurement technology is proposed. This scheme realizes the coupling of the polarization state and the transversal position state of a beam and then post-selects the polarization state. Finally, the tiny Doppler frequency shifts are amplified. At the same time, the energy recycling technology is incorporated to increase the best measurement precision by 1000 times compared with the classical measurements. Considering the loss in the energy recycling cavity, we draw the following conclusion: When the loss rate in the cavity does not exceed 20%, the precision of our scheme can be twice of the shot noise limit of the classical measurement.

Appendix

In this part, we give the formula derivation process of the measurement precision and signal-to-noise ratio of the split detector.

According to the quantization theory of electromagnetic field and the related theory of split detection, the electromagnetic field can be expanded under a set of orthogonal basis vectors. The expression of the positive frequency part of the electromagnetic field is

$$ \hat{\varepsilon }^{\left( + \right)} \left( x \right) = i\left( {\frac{\hbar \omega }{{2\varepsilon_{0} cT}}} \right)^{{{1 \mathord{\left/ {\vphantom {1 2}} \right. \kern-\nulldelimiterspace} 2}}} \sum\limits_{n} {\hat{a}_{n} u_{n} } \left( x \right) $$

(7)

In the above formula, $\omega$ is the frequency of the electromagnetic field, c is the speed of light, T is the detection integration time, $\varepsilon_{0}$ is the permittivity of free space, $\hat{a}_{n}$ is the annihilation operator of the nth-order mode, and $u_{n} \left( x \right)$ is the n-order Hermite Gaussian mode. After linearizing the annihilation operator, we can get that only the average value of the n-order Hermite Gaussian mode is not 0, and then, when there is a transversal displacement $d$, the positive frequency part of the signal light can be written as

$$ \hat{\varepsilon }^{ + } \left( x \right) = i\sqrt {\frac{\hbar \omega }{{2\varepsilon_{0} cT}}} \left[ {\left( {\sqrt N + \delta \hat{a}_{0} } \right)u_{0} \left( {x - d} \right) + \sum\limits_{n \ge 1}^{\infty } {\delta \hat{a}_{n} u_{n} \left( {x - d} \right)} } \right] $$

(8)

The laser beam is incident on the split detector, and the split detector needs to subtract the photocurrent measured by the two parts to obtain the difference in the photon number as

$$ \hat{n}_{ - } = \frac{{2\varepsilon_{0} cT}}{\hbar \omega }\int_{ - \infty }^{0} {\left( {\hat{\varepsilon }^{ + } \left( x \right)} \right)}^{ + } \left( {\hat{\varepsilon }^{ + } \left( x \right)} \right)dx - \int_{0}^{\infty } {\left( {\hat{\varepsilon }^{ + } \left( x \right)} \right)}^{ + } \left( {\hat{\varepsilon }^{ + } \left( x \right)} \right)dx $$

(9)

Taking Eq. (8) into Eq. (9) and omitting higher-order terms, we get

$$ \hat{n}_{ - } = \sqrt N \left( {d \times 2\sqrt N {{\sqrt {{2 \mathord{\left/ {\vphantom {2 \pi }} \right. \kern-\nulldelimiterspace} \pi }} } \mathord{\left/ {\vphantom {{\sqrt {{2 \mathord{\left/ {\vphantom {2 \pi }} \right. \kern-\nulldelimiterspace} \pi }} } {w_{0} }}} \right. \kern-\nulldelimiterspace} {w_{0} }} + \delta \hat{X}} \right) $$

(10)

Then, the signal-to-noise ratio satisfies ${\text{SNR}} = \left( {{{d\sqrt {{2 \mathord{\left/ {\vphantom {2 \pi }} \right. \kern-\nulldelimiterspace} \pi }} 2\sqrt N } \mathord{\left/ {\vphantom {{d\sqrt {{2 \mathord{\left/ {\vphantom {2 \pi }} \right. \kern-\nulldelimiterspace} \pi }} 2\sqrt N } {\left( {w_{0} \delta \hat{X}} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {w_{0} \delta \hat{X}} \right)}}} \right)^{2}$.

For coherent light, $\langle {\delta^{2} \hat{X}} \rangle = 1$,

$$ SNR = \frac{2}{\pi }\left( {\frac{2\sqrt N }{{w_{0} }}\Delta d} \right)^{2} $$

(11)

When SNR = 1, we can get the error formula of the displacement measurement

$$ \Delta d = \sqrt {\frac{\pi }{2}} \frac{{w_{0} }}{2\sqrt N } $$

(12)