Modeling Sexual Selection in Túngara Frog and Rationality of Mate Choice

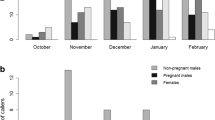
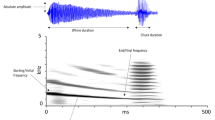
Abstract

The males of the species of frogs Engystomops pustulosus produce simple and complex calls to lure females, as a way of intersexual selection. Complex calls lead males to a greater reproductive success than what simple calls do. However, the complex calls are also more attractive to their main predator, the bat Trachops cirrhosus. Therefore, as M. Ryan suggests in (The túngara frog: a study in sexual selection and communication. University of Chicago Press, Chicago, 1985), the complexity of the calls lets the frogs keep a trade-off between reproductive success and predation. In this paper, we verify this trade-off from the perspective of game theory. We first model the proportion of simple calls as a symmetric game of two strategies. We also model the effect of adding a third strategy, males that keep quiet and intercept females, which would play a role of intrasexual selection. Under the assumption that the decision of the males takes into account this trade-off between reproductive success and predation, our model reproduces the observed behavior reported in the literature with minimal assumption on the parameters. From the model with three strategies, we verify that the quiet strategy could only coexists with the simple and complex strategies if the rate at which quiet males intercept females is high, which explains the rarity of the quiet strategy. We conclude that the reproductive strategy of the male frog E. pustulosus is rational.

Appendix

1.1 Parameters and References

Table 4 shows the values used for the models of two and three strategies with their references.

Table 4 Parameters of the models with two and three strategies

1.2 Model with Two Strategies: Equilibria of the Model

In Sect. 2.1 we defined the following payoff matrix for the game with two strategies:

$$\begin{aligned} A_2 = \frac{r_{{ CS}}}{\rho _{{ CS}}} \begin{pmatrix} \frac{d_S}{\delta _S} &{} \frac{(1-p)}{(1-\pi )} \\ \frac{p}{\pi } &{}\frac{d_C}{\delta _C}\\ \end{pmatrix} =\frac{r_{{ CS}}}{\rho _{{ CS}}} \begin{pmatrix} e_{{ SS}} &{} e_{{ SC}} \\ e_{{ CS}} &{} e_{{ CC}} \\ \end{pmatrix}. \end{aligned}$$

From this matrix, we obtain the replicator equation:

$$\begin{aligned} \dot{s} = s\left( (A_2 \bar{x})_1 - \bar{x}^t A_2 \bar{x}\right) . \end{aligned}$$

(5)

Biologically, each male is competing with many males simultaneously in a chorus of calls to lure mates. Now, when the population is large we may assume that the encounters are homogeneous, where by homogeneity we mean that in a lifetime every male meets in a competition for any female each of the other males with equal chances. In this way we can obtain an expected lifetime payoff value from a weighted average of the payoff values of pairwise interactions using the probabilities that a given male uses each specific strategy. Specifically, let us assume that $N_s$ and $N_c$ are the number of simple and complex callers, respectively, and $N_t = N_s + N_c$ is the total number of males. The total number of encounters is $N_t^2$, where $N_s^2$ are of the type SS, $N_sN_c$ and $N_cN_s$ are of the type SC and ${ CS}$, respectively, and $N_c^2$ are of the type CC. Indeed, the number of encounters SS and SC of one simple caller is approximately $N_s$ and $N_c$, respectively. In consequence, a simple caller has probabilities $s = N_s/N_t$ and $1-s=N_c/N_t$ of having encounters SS and SC, respectively, and the average payoff of one simple caller obtained in one encounter is $(A_2 (s, 1-s)^t)_1= sP(S,S) + (1-s)P(S,C)$. Similarly, the average payoff of one complex caller in one encounter is $(A_2 (s, 1-s)^t )_2= sP(C,S) + (1-s)P(C,C)$. In addition, the probability that one male is a simple caller or a complex caller is s and $1-s$, respectively. In consequence, the average payoff of one male in one encounter is $(s,1-s)A_2(s,1-s)^t=s (A_2 (s, 1-s)^t)_1+(1-s)(A_2 (s, 1-s)^t)_2$. If we define $\bar{x}:= (s,1-s)^t$, we obtain that Eq. (5) states that the success of the simple strategy $\dot{s}/s$ is the difference between the average payoff of one simple caller in one encounter (fitness of a single simple caller $(A_2 \bar{x})_1$) and the average payoff of a male (fitness of a generic male $\bar{x}^tA_2\bar{x}$).

Equation (5) is equivalent to the equation:

$$\begin{aligned} \dot{s} = s(1-s)(a-(a+b)s). \end{aligned}$$

where

$$\begin{aligned} a := \left( \frac{1-p}{1- \pi }-\frac{d_C}{ \delta _C}\right) \frac{r_{{ CS}}}{\rho _{{ CS}}}\quad \text {and}\quad b := \left( \frac{p}{ \pi } -\frac{d_S}{ \delta _S}\right) \frac{r_{{ CS}}}{\rho _{{ CS}}}. \end{aligned}$$

We obtain three possible equilibria of this equation: the pure strategies $s_0=0$, $s_1=1$ and the coexistence equilibrium

$$\begin{aligned} \hat{s} = \frac{a}{a+b}=\frac{e_{{ SC}}-e_{{ CC}}}{e_{{ SC}}-e_{{ CC}}+e_{{ CS}}-e_{{ SS}}} \end{aligned}$$

(6)

if $0<\frac{a}{a+b}<1$.

1.3 Model with Two Strategies: Stability of the Equilibria

Table 5 shows the criteria for the stability of the equilibria presented in the previous subsection.

Table 5 Criteria for the stability of Eq. (3)

We have that $\frac{a}{a+b} = 0$ if $0= \frac{1-p}{1- \pi }-\frac{d_C}{ \delta _C}$ or equivalently

$$\begin{aligned} \pi = \frac{\delta _C}{d_C}p + \left( 1-\frac{\delta _C}{d_C}\right) . \end{aligned}$$

(7)

Equation (7) determines a straight line in the plane $p \pi$. Just below this line, we have that the simple strategy dominates. On the other hand, $\frac{a}{a+b} = 1$ if $0=\frac{p}{ \pi } -\frac{d_S}{ \delta _S}$ or equivalently

$$\begin{aligned} \pi = \frac{\delta _S}{d_S}p. \end{aligned}$$

(8)

Just above the line defined by Eq. (8), the complex strategy dominates. Furthermore, both strategies coexist between the lines defined by Eqs. (7) and (8), as Fig. 1 shows.

We have that the line (7) goes through (1, 1) and the line (8) goes through (0, 0). Moreover, both lines have positive slope and in Fig. 5 we discuss that the two lines must not intersect. Therefore, the slope of the line $a=0$ cannot be smaller than 1, which means that we must have $\frac{\delta _C}{d_C}\ge 1$ (see Eq. (7)). Likewise, the slope of the line $b=0$ cannot be below 1, which is equivalent to $\frac{\delta _S}{d_S}\ge 1$ (see Eq. (8)).

Fig. 5

Grayscale bar shows the value of the stable equilibrium $\hat{s}$ in the two strategies game for $d_c=1, \delta _c = 5, d_s = 0.5, \delta _s = 0.2$. In this case, the two lines $a=0$ and $b=0$, as the slope of $b=0$ is less than 1, intersect in a point with first coordinate $p^*$. We thus obtain a region bounded by these two lines with $p>p^*$, where, once we fix the preference value p, we see that an increase in the predation rate $\pi$ implies an increase in complex calls. We contend that this does not make biological sense, because it would mean that frogs increase their risk with no gain in their reward. Something analogous happens when the slope of $a=0$ is less than one

From Bernal et al. (2007) we have $\hat{s} \ge 0.5$. Therefore, using Eq. (6) we have that $\hat{s} \ge 0.5$ if

$$\begin{aligned} e_{{ CC}} \le e_{{ SS}} - (e_{{ CS}}-e_{{ SC}})=e_{{ SS}} -3/5. \end{aligned}$$

(9)

This is the equation of the line in Fig. 2.

1.4 Model with Three Strategies: Equilibria of the Model

In Sect. 2.2 we defined the following payoff matrix for the game with three strategies:

$$\begin{aligned} A_3= \frac{r_{{ CS}}}{\rho _{{ CS}}} \begin{pmatrix} 0 &{} \theta R \frac{d_S}{\delta _S} &{} \theta R \\ (1-\theta ) R \frac{d_S}{\delta _S} &{} \frac{d_S}{\delta _S} &{} \frac{(1-p)}{(1-\pi )} \\ (1-\theta ) R &{} \frac{p}{\pi } &{}\frac{d_C}{\delta _C}\\ \end{pmatrix}= \frac{r_{{ CS}}}{\rho _{{ CS}}} \begin{pmatrix} 0&{} e_{{ QS}} &{} e_{{ QC}} \\ e_{{ SQ}} &{}e_{{ SS}} &{} e_{{ SC}} \\ e_{{ CQ}} &{} e_{{ CS}} &{} e_{{ CC}} \\ \end{pmatrix}. \end{aligned}$$

We also defined the replicator equation given by:

$$\begin{aligned} {\left\{ \begin{array}{ll} \dot{q} =q\left( (A_3 \bar{x})_1 - \bar{x}^t A_3 \bar{x}\right) \\ \dot{s} =s\left( (A_3 \bar{x})_2 - \bar{x}^t A_3 \bar{x}\right) \\ \end{array}\right. } \end{aligned}$$

We have that there are three equilibria in the corners of the triangle $\{(q,s): q+s\le 1, q\ge 0, s\ge 0\}$, which are (1, 0), (0, 1) and (0, 0). The replicator equation has also the following equilibria:

$$\begin{aligned} w_{{ QC}}:= & {} \left( \frac{e_{{ QC}}-e_{{ CC}}}{e_{{ CQ}}+e_{{ QC}} - e_{{ CC}} },0\right) \end{aligned}$$

(10)

$$\begin{aligned} w_{{ SC}}:= & {} \left( 0, \frac{e_{{ SC}}-e_{{ CC}}}{e_{{ SC}}-e_{{ CC}} + e_{{ CS}} - e_{{ SS}}}\right) \end{aligned}$$

(11)

$$\begin{aligned} w_{{ QS}}= & {} \left( \frac{e_{{ QS}}-e_{{ SS}}}{e_{{ QS}}-e_{{ SS}} + e_{{ SQ}}}, \frac{e_{{ SQ}}}{e_{{ QS}}-e_{{ SS}} + e_{{ SQ}}}\right) \end{aligned}$$

(12)

We have that the equilibrium $w_{{ QC}}$ is within the side of the triangle if $e_{{ QC}} =R \theta > e_{{ CC}}$. The equilibrium $w_{{ SC}}$ is within the side of the triangle if $e_{{ SC}} > e_{{ CC}}$. However, the equilibrium $w_{{ QS}}$ never exists within the side of the triangle because $e_{{ QS}}-e_{{ SS}}<0$ and $e_{{ SQ}}>0$.

In addition, there could be a coexistence equilibrium of the three strategies $w^*$, which is defined as follows:

$$\begin{aligned} w^*=\left( \frac{\nu }{\nu + \xi + \epsilon },\frac{\xi }{\nu + \xi + \epsilon }\right) \end{aligned}$$

(13)

where

$$\begin{aligned} \nu= & {} e_{{ CC}}e_{{ SS}} + e_{{ QS}}e_{{ SC}} + e_{{ QC}}e_{{ CS}} - e_{{ QS}}e_{{ CC}}- e_{{ QC}}e_{{ SS}} - e_{{ SC}}e_{{ CS}} \end{aligned}$$

(14)

$$\begin{aligned} \xi= & {} e_{{ SQ}}e_{{ QC}}+e_{{ CQ}}e_{{ SC}}-e_{{ CQ}}e_{{ QC}}-e_{{ SQ}}e_{{ CC}} \end{aligned}$$

(15)

$$\begin{aligned} \epsilon= & {} e_{{ CQ}}e_{{ QS}} + e_{{ SQ}}e_{{ CS}} -e_{{ SQ}}e_{{ QS}}-e_{{ CQ}}e_{{ SS}} \end{aligned}$$

(16)

1.5 Model with Three Strategies: Stability of the Equilibria

Figure 6 represents the eigenvectors for each equilibrium point, and Table 6 shows their eigenvalues (except for $w^*$).

Fig. 6

Eigenvectors of equilibria of the model with three strategies

Table 6 Eigenvectors and eigenvalues of the replicator equation for the model with three strategies computed in each equilibrium (except the coexistence equilibrium)

We have that $\epsilon$ is always positive. Using that $e_{{ SQ}} = (1-\theta )R e_{{ SS}}$ and $e_{{ QS}} = \theta R e_{{ SS}}$, we have that $\nu >0$ is equivalent to:

$$\begin{aligned} e_{{ CC}} > f_1(\theta ,e_{{ SS}}):= & {} \frac{e_{{ QS}}e_{{ SC}} + e_{{ QC}}e_{{ CS}} - e_{{ QC}}e_{{ SS}}-e_{{ SC}}e_{{ CS}}}{e_{{ QS}} - e_{{ SS}}} \nonumber \\= & {} \frac{\theta R e_{{ SC}}-e_{{ QC}}}{\theta R_1} + \frac{1}{e_{{ SS}}}\frac{e_{{ CS}}(e_{{ QC}}-e_{{ SC}})}{\theta R -1} \end{aligned}$$

(17)

Similarly, $\xi >0$ is equivalent to:

$$\begin{aligned} e_{{ CC}} < f_2(\theta , e_{{ SS}}):= & {} \frac{e_{{ SQ}}e_{{ QC}}+e_{{ CQ}}e_{{ SC}}-e_{{ CQ}}e_{{ QC}}}{e_{{ SQ}}}\nonumber \\= & {} e_{{ QC}} + \frac{1}{e_{{ SS}}}\frac{e_{{ CQ}}(e_{{ SC}}-e_{{ QC}})}{(1-\theta )R} \end{aligned}$$

(18)

In general, if $e_{{ QC}} < e_{{ SC}}$ (i.e., $\theta < e_{{ SC}}/R$), the signs of the first and second derivatives of Eqs. (17) and (18) imply that $f_1$ and $f_2$ are decreasing and convex. Taking the parameters of Table 4, we have that $f_1 > f_2$ and $f_1, f_2$ are above $f_1(\theta ,1)=e_{{ SC}}$ (left side in Fig. 7). In consequence, if $e_{{ CC}}<e_{{ SC}}$ (which is equivalent to the existence of $w_{{ SC}}$), we have that $\nu <0$ and $\xi >0$. This implies that if $\theta < e_{{ SC}}/R$, then $w_{{ SC}}$ is stable, $w_{{ QS}}$ is unstable (if exists) and $w^*$ does not exist.

On the other hand, in general, if $e_{{ QC}} > e_{{ SC}}$ (i.e., $\theta > e_{{ SC}}/R$) we have that $f_1$ and $f_2$ are increasing and concave functions. If we also consider the parameter in Table 4, we get that $f_1<f_2$ and $f_1,f_2$ are below $f(\theta ,1)=e_{{ SC}}$ (right side in Fig. 7). If we assume that $w_{{ SC}}$ always exists (which is equivalent to $e_{{ CC}}<e_{{ SC}}$), then we have $e_{{ QC}} = R \theta > e_{{ CC}}$. This last inequality is equivalent to the existence of $w_{{ QC}}$. Furthermore, if $e_{{ CC}}<f_1$ ($\nu <0$ and $\xi >0$), the equilibrium $w_{{ SC}}$ is stable and if $e_{{ CC}}>f_2$ ($\nu >0$ and $\xi <0$), the equilibrium $w_{{ QC}}$ is stable. We also have that $w^*$ exists if $f_1<e_{{ CC}}<f_2$ (which is equivalent to $\nu >0$ and $\xi >0$).

Fig. 7

Possible scenarios for $f_1$ and $f_2$ depending on the value of $\theta$ when we consider the parameters in Table 4. a $\theta = 0.65 < e_{{ SC}}/R = 3/4$, b $\theta = 0.85 > e_{{ SC}}/R=3/4$

In the case when $w^*$ exists, using the parameters of Table 4, we obtain that the eigenvalues of the model with three strategies depend only on $e_{{ SS}}$ and $e_{{ CC}}$. We get numerically that these eigenvalues are negative for $0\le e_{{ SS}} \le 1$ and $0 \le e_{{ CC}} \le 1$, and in that way $w^*$ is stable.

In the model with two strategies, we have that $\hat{s}>1/2$ if $e_{{ CC}} \le e_{{ SS}} - (e_{{ CS}}-e_{{ SC}})$. We also have that the segment $l(e_{{ SS}}) := e_{{ SS}} - (e_{{ CS}}-e_{{ SC}})$, $e_{{ CC}} \ge 0, e_{{ SS}} \le 1$ is below the concave function $f_2$ for $\theta > e_{{ SC}}/R$. Indeed, $l(1)= 1-(e_{{ CS}}-e_{{ SC}}) \le e_{{ SC}}=f_2(\theta ,1)$ and $f_2(e_{{ CS}}-e_{{ SC}})\ge 0$. In consequence, if $\hat{s}>1/2$ for the model with two strategies, we have $e_{{ CC}}< f_2$ and $w_{{ QC}}$ is unstable.