Temperature-dependent remote control of polarization and coherence intensity with sender’s pure initial state

Abstract

We study the remote creation of the polarization and intensity of the first-order coherence (or coherence intensity) in long spin-1/2 chains with one-qubit sender and receiver. Therewith we use a physically motivated initial condition with the pure state of the sender and the thermodynamical equilibrium state of the other nodes. The main part of the creatable region is a one-to-one map of the initial state (control) parameters, except the small subregion twice covered by the control parameters, which appears owing to the chosen initial state. The polarization and coherence intensity behave differently in the state creation process. In particular, the coherence intensity cannot reach any significant value unless the polarization is large in long chains (unlike the short ones), but the opposite is not true. The coherence intensity vanishes with an increase in the chain length, while the polarization (by absolute value) is not sensitive to this parameter. We represent several characteristics of the creatable polarization and coherence intensity and describe their relation to the parameters of the initial state. The link to the eigenvalue–eigenvector parametrization of the receiver’s state space is given.

Appendices

Appendix 1: amplitude $R_N(\tau )$ as a characteristics of transmission line

The nearest-neighbor Hamiltonian (4) can be diagonalized using the Jordan–Wigner transformation method [45, 46]:

$$\begin{aligned} H=\sum _{k} \varepsilon _k \beta _k^+\beta _k ,\;\; \varepsilon _k = \cos (k) ,\;\;\displaystyle k=\frac{\pi n}{N+1}, \;\;\;n=1,2,\dots ,N, \end{aligned}$$

(84)

where $\beta _j$ are the fermion operators, introduced in terms of the other fermion operators $c_j$ using the Fourier transformation

$$\begin{aligned} \beta _k = \sum _{j=1}^N g_k(j) c_j, \end{aligned}$$

(85)

where the fermion operators $c_j$ read

$$\begin{aligned} c_j=(-2)^{j-1} I_{1z}I_{2z}\dots I_{(j-1)z} I^-_j. \end{aligned}$$

(86)

Here

$$\begin{aligned} g_k(j)=\left( \frac{2}{N+1} \right) ^{1/2} \sin (k j). \end{aligned}$$

(87)

The projection operators $I_{jz}$ can be represented as

$$\begin{aligned} I_{jz} = c^+_j c_j -\frac{1}{2},\;\;\forall \; j. \end{aligned}$$

(88)

Before proceeding to the derivation of the density matrix evolution, we rewrite initial density matrix (2) in the following operator form

$$\begin{aligned} \rho _0= & {} \frac{1}{Z}\left( \frac{1}{2} E + \left( |a_0|^2-|a_1|^2\right) I_{z1} + a_0 a_1^* I^+_1 + a_1 a_0^* I^-_1\right) e^{-{b} I_{z1}} e^{{b} I_z}\nonumber \\= & {} \frac{1}{Z}\left( A_1 E + A_2I_{z1} + A_3 I^+_1 + A_4 I^-_1\right) e^{{b} I_z} , \end{aligned}$$

(89)

where E is the $2\times 2$ unit operator,

$$\begin{aligned} Z= & {} \left( 2 \cosh \frac{{b}}{2}\right) ^{N-1},\;\;I_z =\sum _{i=1}^N I_{zi},\nonumber \\ A_1= & {} \frac{1}{2}e^{-\frac{{b}}{2}} +|a_1|^2 \sinh \frac{{b}}{2},\;\;\; A_2=e^{-\frac{{b}}{2}} -2 |a_1|^2 \cosh \frac{{b}}{2},\nonumber \\ A_3= & {} a_0 a_1^* e^{\frac{{b}}{2}},\;\;\;A_4=a_0^* a_1 e^{-\frac{{b}}{2}} . \end{aligned}$$

(90)

Since $[H,I_z]=0$, the evolution of the density matrix can be written as

$$\begin{aligned} \rho (\tau )=\frac{1}{Z}\sum _{i=1}^4 {r_i(\tau )} e^{{b} I_z}, \end{aligned}$$

(91)

with

$$\begin{aligned} r_1(\tau )= & {} A_1 ,\;\; \nonumber \\ r_2(\tau )= & {} A_2\left( -\frac{1}{2} + \sum _{k,k'=1}^N e^{-i \tau (\varepsilon _k-\varepsilon _{k'})} g_{1k}g_{1k'}\beta ^+_k\beta _{k'} \right) ,\nonumber \\ r_3(\tau )= & {} A_3\sum _{k=1}^N e^{-i \tau \varepsilon _k} g_{1k} \beta ^+_k,\;\;\; r_4(\tau )=A_4\sum _{k=1}^N e^{i \tau \varepsilon _k} g_{1k} \beta _k. \end{aligned}$$

(92)

Reducing this matrix with respect to all the nodes except for the Nth one and writing it in the basis $|0\rangle , |N\rangle$, we obtain the state of the last node:

$$\begin{aligned} \rho _N(\tau )= \left( \begin{array}{cc}\displaystyle \frac{e^{\frac{{b}}{2}}}{2 \cosh \frac{{b}}{2}} + \frac{1}{2}\left( \frac{e^{-\frac{{b}}{2}}}{\cosh \frac{{b}}{2}}- 2|a_1|^2\right) |f_N(\tau )|^2 &{} \displaystyle \left( -\tanh \frac{{b}}{2}\right) ^{N-1} a_0 a_1^* f^*_N(\tau )\\ \displaystyle \left( -\tanh \frac{{b}}{2}\right) ^{N-1} a_0^* a_1 f_N(\tau ) &{}\displaystyle \frac{e^{-\frac{{b}}{2}}}{2 \cosh \frac{{b}}{2}} - \frac{1}{2}\left( \frac{e^{-\frac{{b}}{2}}}{\cosh \frac{{b}}{2}}- 2|a_1|^2\right) |f_N(\tau )|^2 \end{array} \right) ,\nonumber \\ \end{aligned}$$

(93)

where

$$\begin{aligned} f_N(\tau )= \sum _{k=1}^N e^{ i \varepsilon _k \tau } g_{1k} g_{Nk}. \end{aligned}$$

(94)

In our calculations, we use $f_N$ as a global characteristic of the transmission line and represent it in the form

$$\begin{aligned} f_N(\tau )= R_N(\tau ) e^{2 i \pi \Phi _N(\tau )}, \end{aligned}$$

(95)

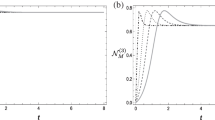
where $R_N$ and $\Phi _N$ are the amplitude and the phase of $f_N$, respectively. Then, Eq. (93) reduces into Eq. (5). The maximal creatable region corresponds to the maximum of $R_N(\tau )$. This maximum R(N) and the appropriate time instant $\tau _{max}(N)$ are found as functions of the chain length N in Sect. 3.1, see Fig. 1.

Appendix 2: the asymptotic behavior of function $R_N(\tau )$ as $N\rightarrow \infty$

Let us rewrite the function $f_N(\tau )$ for odd N as (the case of even N can be treated similarly)

$$\begin{aligned} f_N(\tau )= & {} \frac{2}{N+1}\sum _k e^{i\epsilon _k \tau }\sin (kN) \sin (k)\nonumber \\= & {} \frac{2}{N+1}\sum _{n=1}^N \sin ^2 \left( \frac{\pi n}{N+1} \right) \cos \left[ \tau \cos \left( \frac{\pi n}{N+1}\right) \right] \end{aligned}$$

(96)

We can introduce the Bessel functions into Eq. (96) using the following well-known relation [47]:

$$\begin{aligned} \cos \left[ \tau \cos \left( \frac{\pi n}{N+1}\right) \right] =J_0(\tau )+2\sum _{m=1}^\infty (-1)^m J_{2m}(\tau ) \cos \left( \frac{2\pi mn}{N+1}\right) . \end{aligned}$$

(97)

Substituting Eq. (97) into (96), we obtain:

$$\begin{aligned} f_N(\tau )= & {} -\sum _{p=0}^\infty (-1)^{(2p+1)\frac{N+1}{2}} \left( 2 J_{(2p+1)(N+1)}(\tau ) \right. \nonumber \\&\left. +\; J_{(2p+1)(N+1)-2}(\tau ) + J_{(2p+1)(N+1)+2}(\tau )\right) . \end{aligned}$$

(98)

It can be simply verified using the numerical simulation that the behavior of $f_N(\tau )$ over the time interval $0<\tau \lesssim 2 N$ with $N>2$ is governed by the first term in the above sum over p. In other words, we have for the amplitude of $f_N$:

$$\begin{aligned} R^\mathrm{appr}_N(\tau )= & {} \Big | J_{N+3}(\tau ) +J_{N-1}(\tau ) +2 J_{N+1}(\tau )\Big |. \end{aligned}$$

(99)

Being derived for odd N, this formula holds for even N as well, giving the maximum R in formulas (14) and (15) with the accuracy increasing with N. Thus, $|R(3)-R^\mathrm{appr}(3)| \sim 0.001$ and $|R(5)-R^\mathrm{appr}(5)| \sim 10^{-5}$. Consequently, although formula (99), generally speaking, assumes large N, it is applicable to the short chains as well.