Skip to main content
SpringerLink
Log in

A Linear Implicit Finite Difference Discretization of the Schrödinger–Hirota Equation

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

Abstract

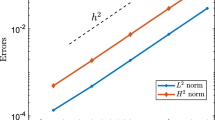
A new linear implicit finite difference method is proposed for the approximation of the solution to a periodic, initial value problem for a Schrödinger–Hirota equation. Optimal, second order convergence in the discrete \(H^1\)-norm is proved, assuming that \(\tau \), h and \(\tfrac{\tau ^4}{h}\) are sufficiently small, where \(\tau \) is the time-step and h is the space mesh-size. The convergence analysis is based on the investigation of a modified version of the proposed finite difference method, which is innovative and handles the stability difficulties due to the presence of a nonlinear derivative term in the equation. The efficiency of the proposed finite difference method is verified by results from numerical experiments.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1

Similar content being viewed by others

References

  1. Achilleos, V., Diamantidis, S., Frantzeskakis, D.J., Karachalios, N.I., Kevrekidis, P.G.: Conservation laws, exact traveling waves and modulation instability for an extended nonlinear Schrödinger equation. J. Phys. A Math. Theor. 48, 355205–355237 (2015)

    Article  MATH  Google Scholar 

  2. Akrivis, G.D.: Finite difference discretization of the cubic Schrödinger equation. IMA J. Numer. Anal. 13, 115–124 (1993)

    Article  MathSciNet  MATH  Google Scholar 

  3. Akrivis, G.D., Dougalis, V.A., Karakashian, O.A.: On fully discrete Galerkin methods of second-order temporal accuracy for the nonlinear Schrödinger equation. Numer. Math. 59, 31–53 (1991)

    Article  MathSciNet  MATH  Google Scholar 

  4. Al-Harbi, W.: Numerical solution of Hirota Equation. Master Thesis, Department of Mathematics, Umm Al-Qura University, Mecca, Kingdom of Saudi Arabia (2009)

  5. Ankiewicz, A., Soto-Crespo, J.M., Akhmediev, N.: Rogue waves and rational solutions of the Hirota equation. Phys. Rev. E 81, 046602 (2010)

    Article  MathSciNet  Google Scholar 

  6. Besse, C.: Schéma de relaxation pour l’ équation de Schrödinger non linéaire et les systèmes de Davey et Stewartson. C. R. Acad. Sci. Paris Sér. I 326, 1427–1432 (1998)

  7. Biswas, A., Jawad, A.J.M., Manrakhan, W.N., Sarma, A.K., Jhan, K.R.: Optical solitons and complexitons of the Schrödinger–Hirota equation. Opt. Laser Technol. 44, 2265–2269 (2012)

    Article  Google Scholar 

  8. Chiao, R.Y., Garmire, E., Townes, C.H.: Self-Trapping of Optical Beams. Phys. Rev. Lett. 13, 479–482 (1964); Erratum, 14, 1056 (1965)

  9. Colliander, J., Keel, M., Staffilani, G., Takaoka, H., Tao, T.: Global well-posedness for Schrodinger equations with derivative. SIAM J. Math. Anal. 33, 649–669 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  10. Delfour, M., Fortin, M., Payre, G.: Finite-difference solutions of a non-linear Schrödinger equation. J. Comput. Phys. 44, 277–288 (1981)

    Article  MathSciNet  MATH  Google Scholar 

  11. Demontis, F., Ortenzi, G., van der Mee, C.: Exact solutions of the Hirota equation and vortex filaments motion. Physica D 313, 61–80 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  12. Fei, Z., Pérez-García, V.M., Váquez, L.: Numerical simulation of nonlinear Schrödinger systems: a new conservative scheme. Appl. Math. Comput. 71, 165–177 (1995)

    MathSciNet  MATH  Google Scholar 

  13. Fukumoto, Y., Miyazaki, T.: N-solitons on a curved vortex filament, with axial flow. J. Phys. Soc. Jpn. 55, 3365–3370 (1988)

    MathSciNet  Google Scholar 

  14. Fukumoto, Y., Miyazaki, T.: Three-dimensional distortions of a vortex filament with axial velocity. J. Fluid Mech. 222, 369–416 (1991)

    Article  MathSciNet  MATH  Google Scholar 

  15. Hasewaga, A., Kodama, Y.: Solitons in Optical Communications, Oxford Series in Optical and Imaging Sciences, vol. 7. Claredon Press, Wotton-under-Edge (1995)

    Google Scholar 

  16. Hasewaga, A., Matsumoto, A.: Optical Solitons in Fibers. Springer Series in Photonics. Springer, Berlin (2003)

    Book  Google Scholar 

  17. Hirota, R.: Exact envelope-soliton solutions of a nonlinear wave equation. J. Math. Phys. 14, 805–809 (1973)

    Article  MathSciNet  MATH  Google Scholar 

  18. Karakashian, O., Akrivis, G.D., Dougalis, V.A.: On optimal order error estimates for the nonlinear Schrödinger equation. SIAM J. Numer. Anal. 30, 377–400 (1993)

    Article  MathSciNet  MATH  Google Scholar 

  19. Karakashian, O., Makridakis, Ch.: A space-time finite element method for the nonlinear Schrödinger equation: the discontinuous Galerkin method. Math. Comput. 67, 479–499 (1998)

    Article  MATH  Google Scholar 

  20. Lamb, G.L.: Elements of Soliton Theory. Wiley, Claredon Press, Wotton-under-Edge (1980)

    MATH  Google Scholar 

  21. Raslan, K.R., El-Danaf, T.S., Ali, K.: Collocation method with quintic \(B-\)spline method for solving the Hirota equation. J. Abstr. Comput. Math. 1, 1–12 (2016)

    Google Scholar 

  22. Robinson, M.P., Fairweather, G.: Orthogonal spline collocation methods for Schrödinger-type equations in one space variable. Numer. Math. 68, 355–376 (1994)

    Article  MathSciNet  MATH  Google Scholar 

  23. Sanz-Serna, J.M.: Methods for the numerical solution of the nonlinear Schroedinger equation. Math. Comput. 43, 21–27 (1984)

    Article  MathSciNet  MATH  Google Scholar 

  24. Sulem, P.L., Sulem, C., Patera, A.: Numerical simulation of singular solutions to the two-dimensional cubic Schrödinger equation. Commun. Pure Appl. Math. 37, 755–778 (1984)

    Article  MATH  Google Scholar 

  25. Taha, T.R.: Numerical simulations of the complex modified Korteweg-de Vries equation. Math. Comput. Simul. 37, 461–467 (1994)

    Article  MathSciNet  MATH  Google Scholar 

  26. Tourigny, Y.: Optimal \(H^1\) estimates for two time-discrete Galerkin approximations of a nonlinear Schrödinger equation. IMA J. Numer. Anal. 11, 509–523 (1991)

    Article  MathSciNet  MATH  Google Scholar 

  27. Uddin, M., Haq, S., Siraj-ul-Islam.: Numerical solution of complex modified Korteweg-de Vries equation by mesh-free collocation method. Comput. Math. Appl. 58, 566–578 (2009)

  28. Wang, J.: A new error analysis of Crank–Nicolson Galerkin FEMs for a generalized nonlinear Schrödinger equation. J. Sci. Comput. 60, 390–407 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  29. Williams, T., Kelley, C.: Gnuplot 4.0: an interactive plotting program, April (2004). http://gnuplot.sourceforge.net/

  30. Zouraris, G.E.: On the convergence of a linear two-step finite element method for the nonlinear Schrödinger equation. Math. Model. Numer. Anal. 35, 389–405 (2001)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics and Applied Mathematics, University of Crete, GR-700 13, Panepistimioupolis, Heraklion, Crete, Greece

    Georgios E. Zouraris

Authors
  1. Georgios E. Zouraris
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Georgios E. Zouraris.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Zouraris, G.E. A Linear Implicit Finite Difference Discretization of the Schrödinger–Hirota Equation. J Sci Comput 77, 634–656 (2018). https://doi.org/10.1007/s10915-018-0718-6

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10915-018-0718-6

Keywords

Mathematics Subject Classification

Search

Navigation