Skip to main content
SpringerLink
Log in

Effect of Rotation on Thermal Convection in an Anisotropic Porous Medium with Temperature-dependent Viscosity

  • Published:
Transport in Porous Media Aims and scope Submit manuscript

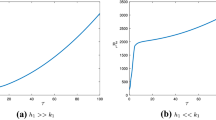
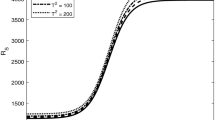
Abstract

A linear stability analysis is performed for mono-diffusive convection in an anisotropic rotating porous medium with temperature-dependent viscosity. The Galerkin variant of the weighted residual technique is used to obtain the eigen value of the problem. The effect of Taylor–Vadasz number and the other parameters of the problem are considered for stationary convection in the absence or presence of rotation. Oscillatory convection seems highly improbable. Some new results on the parameters’ influence on convection in the presence of rotation, for both high and low rotation rates, are presented.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

Abbreviations

a :

Horizontal wave number

a c :

Critical wave number

Br D :

Brinkman–Darcy number, ΛDa

c :

Specific heat

c p :

Specific heat at a constant pressure

Da −1 :

Inverse Darcy number (porous parameter), \({\frac{d^{2}}{k_v }}\)

d :

Height of the porous layer

\({\vec {g}}\) :

Gravitational acceleration (0, 0, −g)

k :

Permeability

k :

Permeability tensor, \({\frac{1}{k_h }\hat{{i}}\hat{{i}}+\frac{1}{k_h }\hat{{j}}\hat{{j}}+\frac{1}{k_v }\hat{{k}}\hat{{k}}}\)

\({\hat{{k}}}\) :

Unit vector in the vertical direction

(k h ,k h ,k v ):

Permeability along x, y and z-direction

l, m :

Wave numbers

p :

Pressure

p * :

Hydrostatic pressure

p H :

Basic state pressure

Pr:

Prandtl number, \({\frac{\nu \Phi }{\chi_{Tv}}}\)

\({\vec {q}}\) :

(u, v, w), velocity vector

\({{\vec {q}}'}\) :

Velocity of the perturbed state

R :

Rayleigh number, \({\frac{\alpha g\Delta Td^{3}}{\nu \chi_{Tv}}}\)

R D :

Darcy–Rayleigh number,RDa

t :

Time

T :

Temperature field

Ta :

Taylor number, \({\frac{4\Omega^{2}d^{4}}{\Phi^{2}\nu^{2}}}\)

T b :

Basic state temperature

T R :

Reference temperature

u, v, w :

Dimensional horizontal and vertical velocity components

u *,v *,w * :

Dimensionless velocity components

V :

Linear variable viscosity parameter, Γ ΔT

Va :

Vadasz or Prandtl–Darcy number, PrDa

Va D :

(Taylor–Vadasz number), Ta Da 2

x :

Horizontal coordinate

x * :

Dimensionless horizontal coordinate

z :

Vertical coordinate

z * :

Dimensionless vertical coordinate

(x, y, z):

Cartesian coordinates with z-axis vertically upward

α :

Coefficient of thermal expansion

(χ Th , χ Tv ):

Thermal conductivities in x- and z-directions

ε :

Mechanical anisotropy parameter, \({\frac{k_h }{k_v }}\)

Φ:

Porosity of the media

η :

Thermal anisotropy parameter, χ Th /χ Tv

γ :

Heat capacity ratio, \({{(\rho_R c_p )_m}/{(\rho_R c_p )_f }}\)

τ :

Scaled dimensionless time, t Da −1

ΔT :

Temperature gradient

▽:

\({\hat{i}\frac{\partial}{\partial x}+\hat{j}\frac{\partial }{\partial y}+\hat{k}\frac{\partial }{\partial z}}\) (vector differential operator)

▽2 :

\({\frac{\partial^{2}}{\partial x^{2}} +\frac{\partial^{2}}{\partial y^{2}}+\frac{\partial^{2}}{\partial z^{2}}}\) (three-dimensional Laplacian operator)

\({\nabla_1^2}\) :

\({\frac{\partial^{2}}{\partial x^{2}} +\frac{\partial^{2}}{\partial y^{2}}}\) (two-dimensional Laplacian operator)

Λ:

Brinkman number, \({\frac{\mu_p }{\mu_f }}\)

μ :

Dynamic viscosity

μ p :

Effective viscosity

μ f :

Viscosity of the fluid

ν :

Kinematic viscosity, \({\frac{\mu_f }{\rho_R }}\)

ρ :

Density

ρ b :

Basic state density

ρ R :

Density of the liquid at reference temperature T = T m

ψ :

Stream function

σ :

Growth rate of perturbation

\({\vec {\Omega }}\) :

Angular velocity of rotation

ω :

Scaled frequency of oscillation

ζ:

z- component of vorticity, \({\left( {\frac{\partial v}{\partial x}-\frac{\partial u}{\partial y}}\right)}\)

b :

Basic state

c :

Critical quantity

f :

Fluid

′:

Dimensional quantities

*:

Dimensionless quantities

o :

Oscillatory

s :

Stationary

References

  • Bhadauria B.S.: Effect of temperature modulation on the onset of Darcy convection in a rotating porous medium. J. Porous Media 11, 361–375 (2008). doi:10.1615/JPorMedia.v11.i4.30

    Article  Google Scholar 

  • Chandrasekhar S.: Hydrodynamic and Hydromagnetic stability. Oxford University Press, London (1961)

    Google Scholar 

  • Ecke R.E., Zhong F., Knobloch E.S.: Hopf bifurcation with broken reflection symmetry in rotating Rayleigh–Bénard convection. Europhys. Lett. 19, 177–182 (1992). doi:10.1209/0295-5075/19/3/005

    Article  Google Scholar 

  • El-Hakiem M.A., Rashad A.M.: Effect of radiation on non-Darcy free convection from a vertical cylinder embedded in a fluid-saturated porous medium with a temperature-dependent viscosity. J. Porous Media 10, 209–218 (2007). doi:10.1615/JPorMedia.v10.i2.80

    Article  Google Scholar 

  • Epherre J.F.: Critere d’apparition de la convection naturelle dans une couche poreuse anisotrope. Rev. Gen. Thermique. 168, 949 (1975)

    Google Scholar 

  • Finlayson B.A.: The Method of Weighted Residuals and Variational Principles. Academic Press, NewYork (1972)

    Google Scholar 

  • Givler R.C., Altobelli S.A.: Determination of the effective viscosity for the Brinkman–Forchheimer flow model. J. Fluid Mech. 258, 355–370 (1994). doi:10.1017/S0022112094003368

    Article  Google Scholar 

  • Govender S.: The effect of anisotropy on stability of convection in a rotating porous layer distant from the center of rotation. J. Porous Media 9, 651–662 (2006). doi:10.1615/JPorMedia.v9.i7.40

    Article  Google Scholar 

  • Govender S.: Linear stability of solutal convection in rotating solidifying mushy layers: permeable mush-melt interface. J. Porous Media 11, 683–690 (2008). doi:10.1615/JPorMedia.v11.i7.60

    Article  Google Scholar 

  • Govender S., Vadasz P.: Moderate time linear study of moderate Stephan number convection in rotating mushy layers. J. Porous Media 5, 113–121 (2002)

    Google Scholar 

  • Govender S., Vadasz P.: The effect of mechanical and thermal anisotropy on the stability of gravity driven convection in rotating porous media in the presence of thermal non-equilibrium. Transp. Porous Media 69, 55–56 (2007). doi:10.1007/s11242-006-9063-6

    Article  Google Scholar 

  • Ingham D.B., Pop I.: Transport Phenomena in Porous Media. Elsevier, New York (2002)

    Google Scholar 

  • Jou J.J., Liaw J.S.: Thermal convection in a porous medium subject to transient heating and rotation. Int. J. Heat Mass Transf. 30, 208–211 (1987). doi:10.1016/0017-9310(87)90076-7

    Article  Google Scholar 

  • Knobloch E.: Rotating convection: recent developments. Int. J. Eng. Sci. 36, 1421–1450 (1998). doi:10.1016/S0020-7225(98)00041-X

    Article  Google Scholar 

  • Malashetty M.S.: Mahantesh Swamy: Combined effect of thermal modulation and rotation on the onset of stationary convection in a porous layer. Transp. Porous Media 69, 313–330 (2007). doi:10.1007/s11242-006-9087-y

    Article  Google Scholar 

  • Nield D.A.: The effect of temperature-dependent viscosity on the onset of convection in a saturated porous medium. ASME J. Heat Transf. 118, 803–805 (1996). doi:10.1115/1.2822705

    Article  Google Scholar 

  • Nield D.A., Bejan A.: Convection in Porous Media. Springer, Berlin (2006)

    Google Scholar 

  • Palm E., Tyvand P.A.: Thermal convection in a rotating porous layer. Z. Angew. Math. Phys. 35, 122–123 (1984). doi:10.1007/BF00945182

    Article  Google Scholar 

  • Patil P.R., Vaidyanathan G.: On setting up of convection currents in a rotating porous medium under the influence of variable viscosity. Int. J. Eng. Sci. 21, 123–130 (1983). doi:10.1016/0020-7225(83)90004-6

    Article  Google Scholar 

  • Riahi D.N.: On stationary and oscillatory modes of flow instability in a rotating porous layer during alloy solidification. J. Porous Media 6, 30 (2003). doi:10.1615/JPorMedia.v6.i3.30

    Article  Google Scholar 

  • Riahi D.N.: Non linear convection in a rotating mushy layer. J. Fluid Mech. 553, 389–400 (2006). doi:10.1017/S0022112006009037

    Article  Google Scholar 

  • Riahi D.N.: The inertial effects on rotating flow in a porous layer. J. Porous Media 10, 343–356 (2007a). doi:10.1615/JPorMedia.v10.i4.20

    Article  Google Scholar 

  • Riahi D.N.: Inertial and Coriolis effects on oscillatory flow in a horizontal dendrite layer. Transp. Porous Media 69, 301–312 (2007b). doi:10.1007/s11242-006-9091-2

    Article  Google Scholar 

  • Richardson L., Straughan B.: Convection with temperature dependent viscosity in a porous medium: non-linear stability and the Brinkman effect. Atti. Accad. Naz.Lincei-Ci-Sci-Fis. Mat. 4, 223–232 (1993)

    Google Scholar 

  • Rudraiah N., Chandna O.P.: Effects of Coriolis force and non-uniform temperature gradient on the Rayleigh–Benard convection. Can. J. Phys. 64, 90–98 (1985)

    Google Scholar 

  • Rudraiah N., Rohini G.: Cellular convection in a rotating fluid through porous medium. Vignana Bharathi India 4, 23–29 (1975)

    Google Scholar 

  • Rudraiah N., Srimani P.K.: Thermal convection in a rotating fluid through a porous medium. Vignana Bharati India 2, 11–17 (1976)

    Google Scholar 

  • Rudraiah N., Vortmeyer D.: Stability of finite amplitude and overstable convection of conducting fluid through fixed porous bed. Warme- und Stoffibertragung 11, 241–254 (1978)

    Article  Google Scholar 

  • Straughan B.: A sharp nonlinear stability threshold in rotating porous convection. Proc. R. Soc. Lond. 457, 87–93 (2000)

    Google Scholar 

  • Vadasz P.: Three dimensional free convection in a long rotating porous box. J. Heat Transf. 115, 639–644 (1993). doi:10.1115/1.2910734

    Article  Google Scholar 

  • Vadasz P.: Stability of free convection in a narrow porous layer subject to rotation. Int. Comm. Heat Mass Transf. 21, 881–890 (1994). doi:10.1016/0735-1933(94)90041-8

    Article  Google Scholar 

  • Vadasz, P.: Flow in rotating porous media. In: Du Plessis, P. (ed.) Fluid Transport in Porous Media, Chap. 4, pp. 161–214. Computational Mechanics Publications, Southampton (1997)

  • Vadasz P.: Coriolis effect on gravity-driven convection in a rotating porous layer heated from below. J. Fluid Mech. 376, 351–375 (1998a). doi:10.1017/S0022112098002961

    Article  Google Scholar 

  • Vadasz P.: Free convection in rotating porous media. In: Ingham, D.B., Pop, I. (eds) Transport Phenomena in Porous Media, Elsevier, New York (1998b)

    Google Scholar 

  • Vadasz P., Olek S.: Transition and chaos for free convection in a rotating porous layer. Int. J. Heat Mass Transf. 41, 1417–1435 (1998). doi:10.1016/S0017-9310(97)00265-2

    Article  Google Scholar 

  • Vafai K., Hadim H.A.: Hand Book of Porous Media. Marcel Decker, New York (2000)

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Maharani’s Science college for Women, Bangalore, 560 001, India

    R. K. Vanishree

  2. Department of Mathematics, Central College Campus, Bangalore University, Bangalore, 560 001, India

    P. G. Siddheshwar

Authors
  1. R. K. Vanishree
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. P. G. Siddheshwar
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to R. K. Vanishree.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Vanishree, R.K., Siddheshwar, P.G. Effect of Rotation on Thermal Convection in an Anisotropic Porous Medium with Temperature-dependent Viscosity. Transp Porous Med 81, 73–87 (2010). https://doi.org/10.1007/s11242-009-9385-2

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11242-009-9385-2

Keywords

Search

Navigation