Skip to main content
SpringerLink
Log in

Pore-to-core simulations of flow with large velocities using continuum models and imaging data

  • Original Paper
  • Published:
Computational Geosciences Aims and scope Submit manuscript

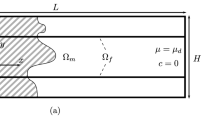
Abstract

We consider computational modeling of flow with small and large velocities at porescale and at corescale, and we address various challenges in simulation, upscaling, and modeling. While our focus is on voxel-based data sets from real porous media imaging, our methodology is verified first on synthetic geometries, and we analyze various scaling and convergence properties. We show that the choice of a voxel-based grid and representative elementary volume size can lead up to 10–20 % difference in calculated conductivities. On the other hand, the conductivities decrease significantly with flow rates, starting in a regime usually associated with the onset of inertia effects. This is accompanied by deteriorating porescale solver performance, and we continue our experiments up until about 50 % reduction in conductivities, i.e., to Reynolds number just under 1. To account for this decrease, we propose a practical power-based fully anisotropic non-Darcy model at corescale for which we calculate the parameters by upscaling.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Porescale Benchmark (2012). In: www.porescalebenchmark.pbworks.com

  2. Akjani, L., Matthai, S.: Finite element-based characterization of pore-scale geometry and its impact on fluid flow. Transp. Porous Media 81, 241–259 (2010)

    Article  Google Scholar 

  3. Andrade, J.S., Costa, U.M.S., Almeida, M.P., Makse, H.A., Stanley, H.E.: Inertial effects on fluid flow through disordered porous media. Phys. Rev. Lett. 82(26), 5249–5252 (1999). doi:10.1103/PhysRevLett.82.5249

    Article  Google Scholar 

  4. ANSYS, Inc.: ANSYS FLUENT User’s Guide, Rel. 14.0 (2011)

  5. Balhoff, M., Wheeler, M.F.: A predictive pore-scale model for non-Darcy flow in porous media. SPE J. 14, 579–587 (2009)

    Google Scholar 

  6. Balhoff, M., Mikelic, A., Wheeler, M.: Polynomial filtration laws for low Reynolds number flows through porous media. Transp. Porous Media 81, 35–60 (2010)

    Article  Google Scholar 

  7. Bear, J.: Dynamics of Fluids in Porous Media. Dover, New York (1972)

    Google Scholar 

  8. Bear, J., Bachmat, Y.: Introduction to Modeling of Transport Phenomena in Porous Media. Kluwer, Dordrecht (1990)

    Book  Google Scholar 

  9. Bear, J., Cheng, A.: Modeling Groundwater Flow and Contaminant Transport. Springer, New York (2010)

    Book  Google Scholar 

  10. Bourgeat, A., Marusić-Paloka, E., Mikelić, A.: Weak nonlinear corrections for Darcy’s law. Math. Models Methods Appl. Sci. 6(8), 1143–1155 (1996)

    Article  Google Scholar 

  11. Cai, R., Lindquist, W., Um, W., Jones, K.: Tomographic analysis of reactive flow induced pore structure changes in column experiments. Adv. Water Resour. 32, 1396–1403 (2009)

    Article  Google Scholar 

  12. Chaudhary, K., Cardenas, M., Deng, W., Bennet, P.: The role of eddies inside pores in the transition from Darcy to Forchheimer flows. Geophys. Res. Lett. 38, L24, 405 (2011). doi:10.1029/2011GL050214

    Article  Google Scholar 

  13. Chen, Z., Lyons, S.L., Qin, G.: Derivation of the Forchheimer law via homogenization. Transp. Porous Media 44(2), 325–335 (2001)

    Article  Google Scholar 

  14. Chu, J., Engquist, B., Prodanovic, M., Tsai, R.: A multiscale method coupling network and continuum models in porous media i: steady-state single phase flow. Multiscale Model. Simul. 10(2), 515–549 (2012). doi:10.1137/110836201, http://epubs.siam.org/doi/abs/10.1137/110836201

    Article  Google Scholar 

  15. Dullien, F.: Porous Media. Academic, San Diego (1979)

    Google Scholar 

  16. Ergun, S.: Fluid flow through packed columns. Chem. Eng. Prog. 48, 89–94 (1952)

    Google Scholar 

  17. Ewing, R.E., Lazarov, R.D., Lyons, S.L., Papavassiliou, D.V., Pasciak, J., Qin, G.: Numerical well model for non-Darcy flow through isotropic porous media. Comput. Geosci. 3(3–4), 185–204 (1999)

    Article  Google Scholar 

  18. Finn, J., Apte, S.: Relative performance of body-fitted and fictitious-domain simulations of flow through porous media. In: Procededings of the ASME Fluids Engineering Summer Meeting (FEDSM2012-72355) (2012)

  19. Forchheimer, P.: Wasserbewegung durch Boden. Zeit. Ver. Deut. Ing. 45, 1781–1788 (1901)

    Google Scholar 

  20. Fourar, M., Lenormand, R., Karimi-Fard, M., Horne, R.: Inertia effects in high-rate flow through heterogeneous porous media. Transp. Porous Media 60, 353–370(18) (2005). doi:10.1007/s11242-004-6800-6

    Article  Google Scholar 

  21. Fourar, M., Radilla, G., Lenormand, R., Moyne, C.: On the non-linear behaviour of a laminar single-phase flow through two and three-dimensional porous media. Adv. Water Resour. 27, 669–677 (2004)

    Article  Google Scholar 

  22. Garibotti, C., Peszyńska, M.: Upscaling non-Darcy flow. Transp. Porous Media 80, 401–430 (2009). doi:10.1007/s11242-009-9369-2

    Article  Google Scholar 

  23. Giorgi, T.: Derivation of Forchheimer law via matched asymptotic expansions. Transp. Porous Media 29, 191–206 (1997)

    Article  Google Scholar 

  24. Hassanizadeh, S.M., Gray, W.: High velocity flow in porous media. Transp. Porous Media 2, 521–531 (1987)

    Article  Google Scholar 

  25. Huang, H., Ayoub, J.: Applicability of the Forchheimer equation for non-Darcy flow in porous media. SPE J. (SPE 102715), 112–122 (2008)

    Google Scholar 

  26. Koivu, V., Decain, M., Geindreau, C., Mattila, K., Bloch, J.F., Kataju, M.: Transport properties of heterogeneous materials. combining computerised x-ray micro-tomography and direct numerical simulations. Int. J. Comput. Fluid Dyn. 23(10), 713–721 (2009)

    Article  Google Scholar 

  27. Marusić-Paloka, E., Mikelić, A.: The derivation of a nonlinear filtration law including the inertia effects via homogenization. Nonlinear Anal. Ser. A: Theory Methods 42(1), 97–137 (2000)

    Article  Google Scholar 

  28. Masad, E., Muhunthan, B., Martys, N.: Simulation of fluid flow and permeability in cohesionless soils. Water Resour. Res. 36(4), 851–864 (2000)

    Article  Google Scholar 

  29. Mattis, S., Dawson, C., Kees, C., Farthing, M.W.: Numerical modeling of drag for flow through vegetated domains and porous structures. Adv. Water Resour. 39, 44–59 (2012)

    Article  Google Scholar 

  30. Mei, C.C., Auriault, J.L.: The effect of weak inertia on flow through a porous medium. J. Fluid Mech. 222, 647–663 (1991)

    Article  Google Scholar 

  31. Narsilio, G., Buzzi, O., Fityus, S., Yun, T., Smith, D.: Upscaling of Navier-Stokes equations in porous media: Theoretical, numerical and experimental approach. Comput. Geotech. 36, 1200–1206 (2009)

    Article  Google Scholar 

  32. Patankar, S.V.: Numerical Heat Transfer and Fluid Flow Fluent software. Hemisphere, Washington DC (1980)

    Google Scholar 

  33. Peszyńska, M., Trykozko, A.: Convergence and stability in upscaling of flow with inertia from porescale to mesoscale. Int. J. Multiscale Comput. Eng. 9(2), 215–229 (2011). doi:10.1615/IntJMultCompEng.v9.i2.60

    Article  Google Scholar 

  34. Peszynska, M., Trykozko, A., Augustson, K.: Computational upscaling of inertia effects from porescale to mesoscale. In: Allen, G., Nabrzyski, J., Seidel, E., van Albada, D., Dongarra, J., Sloot, P. (eds.) ICCS 2009 Proceedings, LNCS 5544, Part I, pp. 695–704. Springer, Berlin (2009)

    Google Scholar 

  35. Peszyńska, M., Trykozko, A., Kennedy, K.: Sensitivity to anisotropy in non-Darcy flow model from porescale through mesoscale. In: Proceedings of CMWR XVIII in Barcelona, June 21–24, 2010. Available online at http://congress.cimne.com/CMWR2010/Proceedings (2010). http://congress.cimne.com/CMWR2010/Proceedings/docs/p46.pdf. Paper 46

  36. Peszyńska, M., Trykozko, A., Sobieski, W.: Forchheimer law in computational and experimental studies of flow through porous media at porescale and mesoscale. In: Mathematical Sciences and Applications, Current Advances in Nonlinear Analysis and Related Topics, vol. 32, pp. 463–482. GAKUTO Internat. Ser. Math. Sci. Appl. (2010)

  37. Porter, M., Schaap, M., Wildenschild, D.: Lattice-Boltzmann simulations of the capillary pressure- saturation-interfacial area relationship for porous media. Adv. Water Resour. 32, 1632–1640 (2009)

    Article  Google Scholar 

  38. Renard, P., Genty, A., Stauffer, F.: Laboratory determination of the full permeability tensor. J. Geophys. Res. 106(Bll), 26,443–26,452 (2001)

    Google Scholar 

  39. Ruth, D., Ma, H.: On the derivation of the Forchheimer equation by means of the averaging theorem. Transp. Porous Media 7(3), 255–264 (1992)

    Article  Google Scholar 

  40. Sánchez-Palencia, E.: Nonhomogeneous Media and Vibration Theory. In: Lecture Notes in Physics, vol. 127. Springer, Berlin (1980)

    Google Scholar 

  41. Schaap, M., Porter, M., Christensen, B., Wildenschild, D.: Comparison of pressure-saturation characteristics derived from computed tomography and lattice Boltzmann simulations. Water Resour. Res. 43(W12S06) (2007)

  42. Scheidegger, A.E.: The Physics of Flow Through Porous Media. Revised edition. The Macmillan Co., New York (1960)

  43. Smolarkiewicz, P., Winter, C.L.: Pores resolving simulation of Darcy flows. J. Comput. Phys. 229, 3121–3133 (2010)

    Article  Google Scholar 

  44. Sobieski, W., Trykozko, A.: Sensitivity aspects of Forchheimer’s approximation. Transp. Porous Media 89, 155–164 (2011)

    Article  Google Scholar 

  45. Succi, S.: The lattice Boltzmann equation for fluid dynamics and beyond. Numerical Mathematics and Scientific Computation. Oxford University Press. Oxford Science Publications (2001)

  46. Tartakovsky, A., Meakin, P.: Pore scale modeling of immiscible and miscible fluid flows using smoothed particle hydrodynamics. Adv. Water Resour. 29, 1464–1478 (2006)

    Article  Google Scholar 

  47. Tartakovsky, A., Scheibe, T., Meakin, P.: Pore-scale model for reactive transport and biomass growth. J. Porous Media 12, 417–434 (2009)

    Article  Google Scholar 

  48. Tartar, L.: Incompressible Fluid Flow in a Porous Medium–Convergence of the Homogenization Process. In: Nonhomogeneous Media and Vibration Theory, vol. 127, pp. 368–377. Lecture Notes in Physics. Springer, Berlin (1980)

    Google Scholar 

  49. Wesseling, P.: Principles of Computational Fluid Dynamics. In: Springer Series in Computational Mathematics, vol. 29. Springer, Berlin (2001)

    Google Scholar 

  50. Wodié, J.C., Lévy, T.: Correction nonlinéaire de la loi de Darcy. C. R. Acad. Sci. Paris Sér. II Méc. Phys. Chim. Sci. Univers Sci. Terre 312(3), 157–161 (1991)

    Google Scholar 

  51. Zaman, E., Jalali, P.: On hydraulic permeability of random packs of monodisperse spheres: direct flow simulations versus correlations. Phys. A 389, 205–214 (2010)

    Article  Google Scholar 

  52. Zaretskiy, Y., Geiger, S., Sorbie, K., Foerster, M.: Efficient flow and transport simulations in reconstructed 3d pore geometries. Adv. Water Resour. 33, 1508–1516 (2010)

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, Oregon State University, Corvallis, OR, 97330, USA

    Malgorzata Peszynska

  2. Interdisciplinary Centre for Mathematical and Computational Modeling, University of Warsaw, Pawinskiego 5a, blok D, 02-106, Warsaw, Poland

    Anna Trykozko

Authors
  1. Malgorzata Peszynska
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Anna Trykozko
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Malgorzata Peszynska.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Peszynska, M., Trykozko, A. Pore-to-core simulations of flow with large velocities using continuum models and imaging data. Comput Geosci 17, 623–645 (2013). https://doi.org/10.1007/s10596-013-9344-4

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10596-013-9344-4

Keywords

Mathematics Subject Classifications (2010)

Search

Navigation