Skip to main content
SpringerLink
Log in

Inductive Reasoning with Equality Predicates, Contextual Rewriting and Variant-Based Simplification

  • Conference paper
  • First Online:
Rewriting Logic and Its Applications (WRLA 2020)

Part of the book series: Lecture Notes in Computer Science ((LNTCS,volume 12328))

Included in the following conference series:

Abstract

We present an inductive inference system for proving validity of formulas in the initial algebra \(T_{\mathcal {E}}\) of an order-sorted equational theory \(\mathcal {E}\) with 17 inference rules, where only 6 of them require user interaction, while the remaining 11 can be automated as simplification rules and can be combined together as a limited, yet practical, automated inductive theorem prover. The 11 simplification rules are based on powerful equational reasoning techniques, including: equationally defined equality predicates, constructor variant unification, variant satisfiability, order-sorted congruence closure, contextual rewriting and recursive path orderings. For \(\mathcal {E} = (\varSigma , E \uplus B)\), these techniques work modulo B, with B a combination of associativity and/or commutativity and/or identity axioms.

This is a preview of subscription content, log in via an institution to check access.

Access this chapter

Log in via an institution
Chapter
USD 29.95
Price excludes VAT (USA)
  • Available as PDF
  • Read on any device
  • Instant download
  • Own it forever
eBook
USD 39.99
Price excludes VAT (USA)
  • Available as EPUB and PDF
  • Read on any device
  • Instant download
  • Own it forever
Softcover Book
USD 54.99
Price excludes VAT (USA)
  • Compact, lightweight edition
  • Dispatched in 3 to 5 business days
  • Free shipping worldwide - see info

Tax calculation will be finalised at checkout

Purchases are for personal use only

Institutional subscriptions

Similar content being viewed by others

Notes

  1. 1.

    As explained in [22], there is no real loss of generality in assuming that all atomic formulas are equations: predicates can be specified by equational formulas using additional function symbols of a fresh new sort Pred with a constant tt, so that a predicate \(p(t_{1},\ldots , t_{n})\) becomes \(p(t_{1},\ldots , t_{n})={tt}\).

  2. 2.

    If \(B = B_{0} \uplus U\), with \(B_{0}\) associativity and/or commutativity axioms, and U identity axioms, the B-preregularity notion can be broadened by requiring only that: (i) \(\varSigma \) is \(B_{0}\)-preregular in the standard sense that \({ls}(u\rho )={ls}(v\rho )\) for all \(u=v \in B_{0}\) and substitutions \(\rho \); and (ii) the axioms U oriented as rules \(\vec {U}\) are sort-decreasing in the sense explained in Sect. 2.2.

  3. 3.

    In [13] the equality predicate is denoted \(\_\sim \_\), instead of the standard notation \(\_=\_\). Here we use \(\_=\_\) throughout. This has the pleasant effect that a QF formula \(\varphi \) is both a formula and a Boolean expression, which of course amounts to mechanizing by equational rewriting the Tarskian semantics of QF formulas in first-order-logic for initial algebras.

  4. 4.

    That is, there is a subtheory inclusion \(\mathcal {B} \subseteq \mathcal {E}\), with \(\mathcal {B}\) having signature \(\varSigma _{\mathcal {B}}\) and only sort NewBool such that: (i) \(T_{\mathcal {B}}\) the initial algebra of the Booleans, and (ii) \(T_{\mathcal {E}^{=}}|_{\varSigma _{\mathcal {B}}} \cong T_{\mathcal {B}}\).

  5. 5.

    An \(\vec {\mathcal {E}}_{1}\)-variant (or \(\vec {E}_{1},B_{1}\)-variant) of a \(\varSigma _{1}\)-term t is a pair \((v,\theta )\), where \(\theta \) is a substitution in canonical form, i.e., \(\theta = \theta !_{\vec {\mathcal {E}}_{1}}\), and \(v =_{B_{1}} (t\theta )!_{\vec {\mathcal {E}}_{1}}\). \(\vec {\mathcal {E}_{1}}\) is FVP iff any such t has a finite set of variants \(\{(u_{1},\alpha _{1}),\ldots , (u_{n},\alpha _{n})\}\) which are “most general possible” in the precise sense that for any variant \((v,\theta )\) of t there exist i, \({1 \leqslant i \leqslant n}\), and substitution \(\gamma \) such that: (i) \(v =_{B_{1}} u_{i}\gamma \), and (ii) \(\theta =_{B_{1}} \alpha _{i}\gamma \).

  6. 6.

    Even when, say, an induction hypothesis in H might originally be a superclause \(\varGamma \rightarrow \bigwedge _{l\in L}\varDelta _l\), for executability reasons we will always decompose it into its corresponding set of clauses \(\{\varGamma \rightarrow \varDelta _l\}_{l\in L}\).

  7. 7.

    Recall that \(\varGamma \) is a conjunction and \(\varLambda \) a conjunction of disjunctions. Therefore, the equality predicate rewrite rules together with \(\vec {H}_{e_U}\) may have powerful “cascade effects.” For example, if either \(\varGamma !\,_{\vec {\mathcal {E}}_{\overline{X}_U}^=\cup \,\vec {H}_{e_U}} = \bot \) or \(\varLambda !\,_{\vec {\mathcal {E}}_{\overline{X}_U}^=\cup \,\vec {H}_{e_U}} = \top \), then \((\varGamma \rightarrow \varLambda ) !\,_{\vec {\mathcal {E}}_{\overline{X}_U}^=\cup \,\vec {H}_{e_U}}\) is a tautology and the goal is proved.

  8. 8.

    The net effect is not only that (EPS) both subsumes (ERL) and (ERR) and becomes more powerful: by adding such extra rules to \(\vec {\mathcal {E}}_{U}^=\), the ICC simplification rule discussed next, which also performs simplification with equality predicates, also becomes more powerful.

  9. 9.

    More generally, the equality predicate theory \(\vec {\mathcal {E}}_{U}^=\) can be extended by adding to it conditional rewrite rules that orient inductive theorems of \(\mathcal {E}\) or \(\mathcal {E}_{U}^=\), are executable, and keep \(\vec {\mathcal {E}}_{U}^=\) operationally terminating. For example, if c and \(c'\) are different constructors whose sorts belong to the same connected component having a top sort, say, s, then the conditional rewrite rule \(x=c(x_{1},\ldots , x_{n})\wedge x=c'(y_{1},\ldots , y_{m})\rightarrow \bot \), where x has sort s orients an inductively valid lemma, clearly terminates, and can thus be added to \(\vec {\mathcal {E}}_{U}^=\). In particular, if p is a Boolean-valued predicate and \(u_{i}=_{B_{0}}v_{i}\), \(p(u_{1},\ldots , u_{n})= {true}\wedge p(v_{1},\ldots , v_{n})= {false}\) rewrites to \(\bot \).

  10. 10.

    A cover set for s is a finte set of \(\varOmega \)-terms such that \({{ls}(u_i) \leqslant s}\), \({1\leqslant i \leqslant n}\), and generating all constructor ground terms of sort s modulo \(B_{\varOmega }\), i.e., .

References

  1. Bouhoula, A., Rusinowitch, M.: SPIKE: a system for automatic inductive proofs. In: Alagar, V.S., Nivat, M. (eds.) AMAST 1995. LNCS, vol. 936, pp. 576–577. Springer, Heidelberg (1995). https://doi.org/10.1007/3-540-60043-4_79

    Chapter  Google Scholar 

  2. Boyer, R., Moore, J.: A Computational Logic. Academic Press, San Diego (1980)

    MATH  Google Scholar 

  3. Clavel, M., et al.: All About Maude - A High-Performance Logical Framework. LNCS, vol. 4350. Springer, Heidelberg (2007). https://doi.org/10.1007/978-3-540-71999-1

    Book  MATH  Google Scholar 

  4. Clavel, M., Palomino, M.: The ITP tool’s manual, universidad Complutense, Madrid, April 2005. http://maude.sip.ucm.es/itp/

  5. Comon, H., Nieuwenhuis, R.: Induction = i - axiomatization + first-order consistency. Inf. Comput. 159(1–2), 151–186 (2000)

    Article  MathSciNet  Google Scholar 

  6. Dershowitz, N., Jouannaud, J.P.: Rewrite systems. In: van Leeuwen, J. (ed.) Handbook of Theoretical Computer Science, vol. B, pp. 243–320. North-Holland (1990)

    Google Scholar 

  7. Durán, F., Lucas, S., Meseguer, J.: Termination modulo combinations of equational theories. In: Ghilardi, S., Sebastiani, R. (eds.) FroCoS 2009. LNCS (LNAI), vol. 5749, pp. 246–262. Springer, Heidelberg (2009). https://doi.org/10.1007/978-3-642-04222-5_15

    Chapter  MATH  Google Scholar 

  8. Escobar, S., Meadows, C., Meseguer, J.: Maude-NPA: cryptographic protocol analysis modulo equational properties. In: Aldini, A., Barthe, G., Gorrieri, R. (eds.) FOSAD 2007-2009. LNCS, vol. 5705, pp. 1–50. Springer, Heidelberg (2009). https://doi.org/10.1007/978-3-642-03829-7_1

    Chapter  MATH  Google Scholar 

  9. Escobar, S., Sasse, R., Meseguer, J.: Folding variant narrowing and optimal variant termination. J. Algebraic Logic Programm. 81, 898–928 (2012)

    Article  MathSciNet  Google Scholar 

  10. Găină, D., Lucanu, D., Ogata, K., Futatsugi, K.: On automation of OTS/CafeOBJ method. In: Iida, S., Meseguer, J., Ogata, K. (eds.) Specification, Algebra, and Software. LNCS, vol. 8373, pp. 578–602. Springer, Heidelberg (2014). https://doi.org/10.1007/978-3-642-54624-2_29

    Chapter  Google Scholar 

  11. Goguen, J., Meseguer, J.: Order-sorted algebra I: equational deduction for multiple inheritance, overloading, exceptions and partial operations. Theoret. Comput. Sci. 105, 217–273 (1992)

    Article  MathSciNet  Google Scholar 

  12. Gutiérrez, R., Meseguer, J., Skeirik, S.: The Maude termination assistant. In: Pre-Proceedings of WRLA 2018

    Google Scholar 

  13. Gutiérrez, R., Meseguer, J., Rocha, C.: Order-sorted equality enrichments modulo axioms. Sci. Comput. Program. 99, 235–261 (2015)

    Article  Google Scholar 

  14. Hendrix, J.D.: Decision procedures for equationally based reasoning. Ph.D. thesis, University of Illinois at Urbana-Champaign (2008). http://hdl.handle.net/2142/10967

    Google Scholar 

  15. Kapur, D., Zhang, H.: An overview of rewrite rule laboratory (RRL). In: Dershowitz, N. (ed.) RTA 1989. LNCS, vol. 355, pp. 559–563. Springer, Heidelberg (1989). https://doi.org/10.1007/3-540-51081-8_138

    Chapter  Google Scholar 

  16. Kaufmann, M., Manolios, P., Moore, J.: Computer-Aided Reasoning: An Approach. Kluwer, Dordrecht (2000)

    Book  Google Scholar 

  17. Lucas, S., Meseguer, J.: Normal forms and normal theories in conditional rewriting. J. Log. Algebr. Meth. Program. 85(1), 67–97 (2016)

    Article  MathSciNet  Google Scholar 

  18. Meier, S., Schmidt, B., Cremers, C., Basin, D.: The TAMARIN prover for the symbolic analysis of security protocols. In: Sharygina, N., Veith, H. (eds.) CAV 2013. LNCS, vol. 8044, pp. 696–701. Springer, Heidelberg (2013). https://doi.org/10.1007/978-3-642-39799-8_48

    Chapter  Google Scholar 

  19. Meseguer, J.: Conditional rewriting logic as a unified model of concurrency. Theoret. Comput. Sci. 96(1), 73–155 (1992)

    Article  MathSciNet  Google Scholar 

  20. Meseguer, J.: Membership algebra as a logical framework for equational specification. In: Presicce, F.P. (ed.) WADT 1997. LNCS, vol. 1376, pp. 18–61. Springer, Heidelberg (1998). https://doi.org/10.1007/3-540-64299-4_26

    Chapter  Google Scholar 

  21. Meseguer, J.: Order-sorted rewriting and congruence closure. In: Jacobs, B., Löding, C. (eds.) FoSSaCS 2016. LNCS, vol. 9634, pp. 493–509. Springer, Heidelberg (2016). https://doi.org/10.1007/978-3-662-49630-5_29

    Chapter  MATH  Google Scholar 

  22. Meseguer, J.: Variant-based satisfiability in initial algebras. Sci. Comput. Program. 154, 3–41 (2018)

    Article  Google Scholar 

  23. Meseguer, J., Skeirik, S.: Inductive reasoning with equality predicates, contextual rewriting and variant-based simplification. Technical report, University of Illinois at Urbana-Champaign, Computer Science Department, July 2020. http://hdl.handle.net/2142/107774

  24. Rocha, C., Meseguer, J.: Proving safety properties of rewrite theories. In: Corradini, A., Klin, B., Cîrstea, C. (eds.) CALCO 2011. LNCS, vol. 6859, pp. 314–328. Springer, Heidelberg (2011). https://doi.org/10.1007/978-3-642-22944-2_22

    Chapter  Google Scholar 

  25. Rocha, C.: Symbolic reachability analysis for rewrite theories. Ph.D. thesis, University of Illinois at Urbana-Champaign (2012)

    Google Scholar 

  26. Rubio, A.: Automated deduction with constrained clauses. Ph.D. thesis, Universitat Politècnica de Catalunya (1994)

    Google Scholar 

  27. Rubio, A.: A fully syntactic AC-RPO. Inf. Comput. 178(2), 515–533 (2002)

    Article  MathSciNet  Google Scholar 

  28. Sasse, R.: Security models in rewriting logic for cryptographic protocols and browsers. Ph.D. thesis, University of Illinois at Urbana-Champaign (2012). http://hdl.handle.net/2142/34373

  29. Sasse, R., King, S.T., Meseguer, J., Tang, S.: IBOS: a correct-by-construction modular browser. In: Păsăreanu, C.S., Salaün, G. (eds.) FACS 2012. LNCS, vol. 7684, pp. 224–241. Springer, Heidelberg (2013). https://doi.org/10.1007/978-3-642-35861-6_14

    Chapter  Google Scholar 

  30. Skeirik, S., Meseguer, J., Rocha, C.: Verification of the IBOS browser security properties in reachability logic. In: Escobar, S., Martí-Oliet, N. (eds.) WRLA 2020, LNCS 12328, pp. 176–196 (2020)

    Google Scholar 

  31. Skeirik, S.: Rewriting-based symbolic methods for distributed system verification. Ph.D. thesis, University of Illinois at Urbana-Champaign (2019)

    Google Scholar 

  32. Skeirik, S., Meseguer, J.: Metalevel algorithms for variant satisfiability. J. Log. Algebr. Meth. Program. 96, 81–110 (2018)

    Article  MathSciNet  Google Scholar 

  33. Skeirik, S., Stefanescu, A., Meseguer, J.: A constructor-based reachability logic for rewrite theories. Fundam. Inform. 173(4), 315–382 (2020)

    Article  MathSciNet  Google Scholar 

  34. Tang, S.: Towards secure web browsing. Ph.D. thesis, University of Illinois at Urbana-Champaign (2011), 25 May 2011. http://hdl.handle.net/2142/24307

    Google Scholar 

  35. Tang, S., Mai, H., King, S.T.: Trust and protection in the Illinois browser operating system. In: Proceedings of the 9th USENIX Symposium on Operating Systems Design and Implementation, OSDI 2010, Vancouver, BC, Canada, pp. 17–32, 4–6 October 2010. USENIX Association (2010)

    Google Scholar 

  36. Zhang, H.: Contextual rewriting in automated reasoning. Fundam. Inform. 24(1/2), 107–123 (1995)

    Article  MathSciNet  Google Scholar 

Download references

Acknowledgements

We cordially thank the referees for their very helpful suggestions to improve the paper. Work partially supported by NRL under contract N00173-17-1-G002.

Author information

Authors and Affiliations

  1. Department of Computer Science, University of Illinois at Urbana-Champaign, Urbana, USA

    José Meseguer & Stephen Skeirik

Authors
  1. José Meseguer
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Stephen Skeirik
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to José Meseguer .

Editor information

Editors and Affiliations

  1. Valencian Research Institute for Artificial Intelligence, Universitat Politècnica de València, Valencia, Spain

    Santiago Escobar

  2. Facultad de Informática, Universidad Complutense de Madrid, Madrid, Spain

    Narciso Martí-Oliet

A The Natural Numbers Theory \(\mathcal {N}\)

A The Natural Numbers Theory \(\mathcal {N}\)

Fig. 1.
figure 1

Natural number theory specification.

Full size image

Note that we have a “sandwich” of theories \(\mathcal {N}_{\varOmega } \subseteq \mathcal {N}_{1} \subseteq \mathcal {N}\), where \(\mathcal {N}_{\varOmega }\) is given by the operators marked as ctor and the associativity-commutativity of \(+\), and \(\mathcal {N}_{1}\) is the FVP theory extending \(\mathcal {N}_{\varOmega }\) with the other symbols for \(+\) and the equation for as identity element for \(+\) (Fig. 1).

Rights and permissions

Reprints and permissions

Copyright information

© 2020 Springer Nature Switzerland AG

About this paper

Check for updates. Verify currency and authenticity via CrossMark

Cite this paper

Meseguer, J., Skeirik, S. (2020). Inductive Reasoning with Equality Predicates, Contextual Rewriting and Variant-Based Simplification. In: Escobar, S., Martí-Oliet, N. (eds) Rewriting Logic and Its Applications. WRLA 2020. Lecture Notes in Computer Science(), vol 12328. Springer, Cham. https://doi.org/10.1007/978-3-030-63595-4_7

Download citation

  • DOI: https://doi.org/10.1007/978-3-030-63595-4_7

  • Published:

  • Publisher Name: Springer, Cham

  • Print ISBN: 978-3-030-63594-7

  • Online ISBN: 978-3-030-63595-4

  • eBook Packages: Computer ScienceComputer Science (R0)

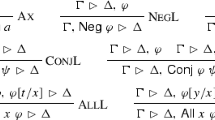
Publish with us

Policies and ethics

Search

Navigation