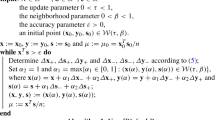Abstract
Recent works have shown that a wide class of Interior Point methods employing linesearch along the Newton step may manifest a weakness of convergence. In order to alleviate such drawback, in this paper we introduce a new globalization strategy. It performs backtracking along a piecewise linear path which can be easily constructed. The proposed strategy is embedded into an Interior Point method. Computational results show that the resulting procedure is remarkably successful, shows fast local rate of convergence and low computational cost.
This research was supported by MURST, Rome,Italy, through “Cofinanziamenti Programmi di Ricerca Scientifica di Interesse Nazionale” and by GNCS, Italy.
Access this chapter
Tax calculation will be finalised at checkout
Purchases are for personal use only
Preview
Unable to display preview. Download preview PDF.
Similar content being viewed by others
References
S. Bellavia, (1998) “An Inexact Interior Point method”, J. Optimization Theory Appl., Vol. 96, pp. 109–121.
H.Y. Benson, D. F. Shanno, R. J. Vanderbei, (2000) “Interior Point Methods for Nonconvex nonlinear programming: Jamming and comparative numerical testing”, Technical report ORFE-00–02, Operation Research and Financial Engineering, Princeton University.
R.H. Byrd, M.E. Hribar, J. Nocedal, (1999) “An interior point algorithm for large-scale nonlinear programming”, SIAM J. Optim., Vol. 9, pp. 877–900.
R. H. Byrd, M. Marazzi, J. Nocedal, (2001), “ On the convergence of Newton Iterations to Non-Stationary Points”, Report OTC 2001/01, Optimization Technology Center.
M. D. Canon, C. D. Cullum, Jr., E. Polak, (1970) Theory of optimal control and mathematical programming, McGraw-Hill, New York, NY.
J.E. Dennis, R.B. Schnabel, (1983), Numerical methods for unconstrained optimization and nonlinear equations, Prentice Hall, Englewood Cliffs, NJ.
C. Durazzi, (2000) “On the Newton Interior-Point method for nonlinear programming problems”, J. Optimization Theory Appl., Vol. 104, pp. 73–90.
A. S. El-Bakry, R. A. Tapia, T. Tsuchiya, Y. Zhang, (1996), “On the Formulation and Theory of the Newton Interior-Point Method for Nonlinear Programming”, J. Optimization Theory Appl., Vol. 89, pp. 507–541.
M.C. Ferris, C. Kanzow, (2002), “Complementarity and related problems: a survey”, in P.M. Pardalos and M.G.C. Resende (eds.) Handbook of Applied Optimization, pp. 514–530, Oxford University Press, New York, NY.
M.C. Ferris, J.S. Pang, (1997) “Engineering and economic applications of cornplementarity problems” SIAM Review,Vol. 39, pp. 669–713.
C.A. Floudas et al., (1999) Handbook of test problems in local and global optimization, Nonconvex Optimization and its Applications, 33, Kluwer Academic Publishers, Dordrecht, The Netherlands.
D. M. Gay, M. L. Overton, M. H. Wright, (1998) “A primal-dual interior method for nonconvex nonlinear programming”, in Y. Yuan Ed., Advances in Nonlinear Programming, pp. 31–56, Kluwer Academic Publishers, Dordrecht, The Netherlands.
M. Kojima, T. Noma, A. Yoshise, (1994), “Global convergence in InfeasibleInterior-Point algorithms”, Math. Progr., Vol. 65, pp. 43–72.
M. Marazzi, J. Nocedal, (2001), “Feasibility Control in nonlinear optimization”, in Devore, A. Iserles, and E. Suli Eds., Foundations of Computational Mathematics, London Mathematical Society Lecture Note Series 284, Cambridge University Press.
J. Nocedal, S.J. Wright,(1999) Numerical Optimization,Springer Series in Operations Research, Springer-Verlag, New York, NY.
E.M. Simantiraky, D.F. Shanno, (1997), “An infeasible-interior-point method for linear complementarity problems”, Siam J. Optim., Vol.7, pp.620–640.
E.M. Simantiraky, D.F. Shanno, (1995) “Computing Equilibria of Oligopolistic Pricing Models”, Rutcor Research Report RRR.41–95.
L. T. Watson, (1979), “Solving the nonlinear complementarity problem by a homotopy method”, Siam J. Control and Optim., Vol. 17, pp.36–46.
H. Yamashita, H. Yabe, T. Tanabe, (1997) “A globally and superlinearly convergent primal-dual interior point trust region method for large scale constrained optimization”, Technical report, Mathematical System Ic.
R. J. Vanderbei, D. F. Shanno, (1999) “An Interior Point algorithm for Non-convex nonlinear programming”, Comput. Optim. Appl.,Vol. 13, pp. 231–252, 1999.
A. Wachter, L.T. Biegler, (2000) “Failure of global convergence for a class of Interior Point methods for nonlinear programming”, Math. Program., Series A, Vol. 88, pp. 565–574.
S. J. Wright, D. Ralph, (1996), “A Superlinear Infeasible-Interior Point Algorithm for Monotone Complementarity Problems”, Math. Operation Res.,Vol. 21, pp. 815–838.
S. J. Wright, (1997), Primal-Dual Interior-Point Methods, Siam Publications, Philadelphia, PA.
Author information
Authors and Affiliations
Editor information
Editors and Affiliations
Rights and permissions
Copyright information
© 2003 Kluwer Academic Publishers B.V.
About this chapter
Cite this chapter
Bellavia, S., Morini, B. (2003). A globalization strategy for Interior Point Methods for Mixed Complementarity Problems. In: Di Pillo, G., Murli, A. (eds) High Performance Algorithms and Software for Nonlinear Optimization. Applied Optimization, vol 82. Springer, Boston, MA. https://doi.org/10.1007/978-1-4613-0241-4_4
Download citation
DOI: https://doi.org/10.1007/978-1-4613-0241-4_4
Publisher Name: Springer, Boston, MA
Print ISBN: 978-1-4613-7956-0
Online ISBN: 978-1-4613-0241-4
eBook Packages: Springer Book Archive