The light-by-light contribution to the muon (g-2) from lightest pseudoscalar and scalar mesons within nonlocal chiral quark model

Abstract

The light-by-light contribution from the lightest neutral pseudoscalar and scalar mesons to the anomalous magnetic moment of muon is calculated in the framework of the nonlocal SU(3)×SU(3) quark model. The model is based on chirally symmetric four-quark interaction of the Nambu–Jona-Lasinio type and Kobayashi–Maskawa–’t Hooft U _A (1) breaking six-quark interaction. Full kinematic dependence of vertices with off-shell mesons and photons in intermediate states in the light-by-light scattering amplitude is taken into account. The small positive contributions from the scalar mesons stabilize the total result with respect to change of model parameters and reduces to $a_{\mu}^{\mathrm{LbL},\mathrm{PS}+\mathrm{S}}=(6.25\pm0.83)\cdot10^{-10}$.

Appendices

Appendix A: Four-quark coupling constants, polarization operators and mixing angles

The elements of G _ch -matrices take the form

$$ \begin{aligned} &G_{00} = G \pm\frac{H}{3} (2S_u + S_s ), \\ &G_{88} = G \mp \frac{H}{6} (4S_u - S_s ), \\ &G_{08} = G _{80}= \mp\frac{\sqrt{2}}{6}H(S_u -S_s ) , \qquad G_{33}=G \mp\frac{H}{2} S_s,\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\! \end{aligned} $$

(A.1)

where the upper sign corresponds to the scalar channel, while the lower sign corresponds to the pseudoscalar channel. For the pion, G _π equals G ₃₃ of the pseudoscalar interaction, and, for the a ₀-meson, $G_{a_{0}}$ equals G ₃₃ of the scalar interaction.

The elements of $\mathrm{\varPi}_{\mathit{ch}}(P^{2})$-matrix for the scalar and pseudoscalar mesons are diagonal in the quark-flavor basis, and in the singlet-triplet-octet basis they are given by

$$ \begin{aligned} &\varPi_{00}\bigl(P^{2}\bigr) = \frac{1}{3}\bigl(2 \varPi_{uu}\bigl(P^{2}\bigr) +\varPi_{ss} \bigl(P^{2}\bigr) \bigr), \\ &\varPi_{88}\bigl(P^{2}\bigr) = \frac{1}{3}\bigl( \varPi_{uu}\bigl(P^{2}\bigr) +2\varPi_{ss} \bigl(P^{2}\bigr) \bigr), \\ &\varPi_{08}\bigl(P^{2}\bigr) = \varPi_{80} \bigl(P^{2}\bigr) =\frac{\sqrt{2}}{3}\bigl(\varPi_{uu} \bigl(P^{2}\bigr) -\varPi_{ss}\bigl(P^{2}\bigr) \bigr), \\ &\varPi_{33}\bigl(P^{2}\bigr)=\varPi_{uu} \bigl(P^{2}\bigr), \end{aligned} $$

(A.2)

where the difference between the scalar and pseudoscalar channels is in the polarization operators

(A.3)

where K _±=K±P/2. Similarly to Eq. (A.1), the upper sign corresponds to the scalar channel and the lower sign corresponds to the pseudoscalar channel, $\varPi_{a_{0}}$ equals Π ₃₃ for the scalar channel and Π _π equals Π ₃₃ for the pseudoscalar channel. The unrenormalized mesonic propagators for the scalar mesons are

$$ \begin{aligned} &D_{a_0}^{-1}\bigl(P^{2}\bigr)=-G_{a_0}^{-1}+ \varPi_{a_0}\bigl(P^{2}\bigr), \\ &D_{\sigma,f_0}^{-1}\bigl(P^{2}\bigr)=\frac{1}{2} \bigl[ (A+C) \pm\sqrt{(A-C)^{2}+4B^{2}} \bigr] , \\ &A=-G_{88}/\det(\mathbf{G}_{\mathit{ch}})+ \varPi_{00}\bigl(P^{2}\bigr), \\ &B=+G_{08} /\det(\mathbf{G}_{\mathit{ch}})+ \varPi_{08}\bigl(P^{2}\bigr), \\ &C=-G_{00}/\det(\mathbf{G}_{\mathit{ch}})+ \varPi_{88}\bigl(P^{2}\bigr), \\ &\det(\mathbf{G}_{\mathit{ch}})=G_{00}G_{88}-G_{08}^{2}. \end{aligned} $$

(A.4)

The mixing angle depends on the meson virtuality

(A.5)

Expressions for the unrenormalized propagators for the pseudoscalar mesons are similar to the scalar meson propagators, Eqs. (A.4), (A.5), with replacements a ₀→π, σ→η, f ₀→η′ and θ _S →θ _P .

Appendix B: Feynman rules for nonlocal vertices

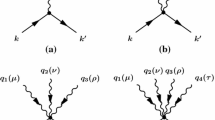
The total vertex of photon interaction with quark–antiquark pair (Fig. 4a) contains local and nonlocal parts

(B.1)

where T ^a≡Q and m⁽¹⁾(p ₁,p ₂) is the first order finite-difference of the dynamical quark mass

(B.2)

Fig. 4

Vertices $\mathrm{\varGamma}^{\mu}_{p_{2},p_{1}}$, Eq. (B.1), $\mathrm{\varGamma}^{M;\mu}_{p_{2},p_{1},q}$, Eq. (B.3), with one photon

The contact interaction vertex of meson, photon and quark–antiquark pair (Fig. 4b) is purely nonlocal and takes the form

(B.3)

In order to express the vertices with two external photons we introduce the following functions:

$$ \begin{aligned} &G_{\mu}^{a} (k,q ) =iT^{a} ( 2k+q )_{\mu}\mathrm{f}^{(1)}(k,k+q), \\ &G_{\mu\nu}^{ab} \bigl( k,q,q^{\prime},k^{\prime} \bigr) = -f \bigl( k^{\prime} \bigr) \bigl\{ T^{a}T^{b} \bigl[ g_{\mu\nu}\mathrm{f}^{(1)}\bigl(k,k+q+q^{\prime} \bigr)\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\! \\ &\hphantom{G_{\mu\nu}^{ab} \bigl( k,q,q^{\prime},k^{\prime} \bigr) =} {}+ \bigl[ 2 \bigl( k+q^{\prime} \bigr) +q \bigr]_{\mu} \bigl( 2k+q^{\prime} \bigr)_{\nu} \\ &\hphantom{G_{\mu\nu}^{ab} \bigl( k,q,q^{\prime},k^{\prime} \bigr) =} {}\times \mathrm{f}^{(2)} \bigl( k,k+q^{\prime},k+q+q^{\prime} \bigr) \bigr] \\ &\hphantom{G_{\mu\nu}^{ab} \bigl( k,q,q^{\prime},k^{\prime} \bigr) =}{}+ \bigl[ ( q,a,\mu) \longleftrightarrow\bigl( q^{\prime },b,\nu \bigr) \bigr] \bigr\} , \end{aligned} $$

(B.4)

where f⁽²⁾(k ₁,k ₂,k ₃) is the second order finite-difference

(B.5)

With this notation, the vertex of two-photon interaction with quark–antiquark pair (Fig. 5a) is

(B.6)

and the interaction vertex for two photons, meson and quark–antiquark pair (Fig. 5b) becomes

(B.7)

Fig. 5

Vertices $\mathrm{\varGamma}^{\mu,\nu}_{p_{2},p_{1},q_{1},q_{2}}$, Eq. (B.6), and $\mathrm{\varGamma}^{M;\mu,\nu }_{p_{2},p_{1},q_{1},q_{2}}$, Eq. (B.7), with two photons

Appendix C: Amplitude with meson and two photons

The photon–meson transition amplitude is a sum of diagrams shown in Fig. 6, where all particles are virtual. For the scalar meson it takes the form

$$ \begin{aligned} &A \bigl(\gamma_{1}^{\ast}\gamma_{2}^{\ast} \rightarrow M^{\ast} \bigr) =e^{2}\epsilon_{1}^{\mu} \epsilon_{2}^{\nu}\varDelta^{\mu\nu} ( p, q_{1}, q_{2} ), \\ &\varDelta^{\mu\nu} ( p, q_{1}, q_{2} ) \\ &\quad = -i N_{c} \int\frac{d^{4}k}{(2\pi)^{4}} \\ &\qquad{}\times\mathrm{Tr} \bigl(2 \mathrm{\varGamma}^{M}_{k_2,k_1}S(k_1) \mathrm{\varGamma}^\mu_{k_1,k_3}S(k_3)\mathrm{ \varGamma}^\nu_{k_3,k_2}S(k_2) \\ &\qquad{}+ \mathrm{\varGamma}^{M;\mu}_{k_2,k_3,q_1}S(k_3) \mathrm{\varGamma}^\nu_{k_3,k_2}S(k_2) \\ &\qquad {}+ \mathrm{ \varGamma}^{M;\nu}_{k_3,k_1,q_2}S(k_1)\mathrm{ \varGamma}^\mu_{k_1,k_3}S(k_3) \\ &\qquad{}+ \mathrm{\varGamma}^{M}_{k_2,k_1}S(k_1) \mathrm{\varGamma}^{\mu,\nu}_{k_1,k_2,q_1,q_2} + \mathrm{\varGamma }^{M;\mu,\nu}_{k_3,k_3,q_1,q_2}S(k_3)\bigr), \end{aligned} $$

(C.1)

where the symbols are the photon momenta q _1,2, the photon polarization vectors ϵ _1,2, the meson momentum p=q ₁+q ₂, and the quark momenta k _1,2,3 (k ₁=k+q ₁, k ₂=k−q ₂, k ₃=k). The first term in parentheses corresponds to the quark triangle diagrams¹⁰ (Fig. 6b and crossed term Fig. 6c) and next terms corresponds to the diagrams in Figs. 6d–g with effective nonlocal vertices defined in (B.3), (B.6), (B.7).

Fig. 6

The diagrams for the photon–meson transition

For different scalar meson states one has the following combinations of nonstrange and strange components:

(C.2)

One can easily see from Eqs. (17), (18) that the mixing for the form factors A _S , B _S , $\mathrm{B}^{\prime}_{S}$ from the components A _u , B _u , $B^{\prime}_{u}$ and A _s , B _s , $B^{\prime}_{s}$ is similar. One should project A _i and B _i (i=u,s) from loops of nonstrange and strange quarks

$$ \begin{aligned} &A_i \bigl( p^{2};q_{1}^{2} ,q_{2}^{2} \bigr) \\ &\quad =\frac{\delta_i^{\mu\nu} ( p^{2}, q_{1}^{2}, q_{2}^{2} ) }{2(q_1\cdot q_2)} \biggl[ g^{\mu\nu}-\frac{(q_1\cdot q_2)\;q_1^\nu q_2^\mu}{(q_1\cdot q_2)^2-q_1^2 q_2^2} \biggr], \\ &B_i \bigl( p^{2};q_{1}^{2} ,q_{2}^{2} \bigr)=-\frac{\delta_i^{\mu\nu} ( p^{2}, q_{1}^{2}, q_{2}^{2} ) }{2(q_1\cdot q_2) ((q_1\cdot q_2)^2-q_1^2 q_2^2 )} \\ &\hphantom{B_i \bigl( p^{2};q_{1}^{2} ,q_{2}^{2} \bigr)=} {}\times\biggl[ g^{\mu\nu}-3\frac{(q_1\cdot q_2)\;q_1^\nu q_2^\mu }{(q_1\cdot q_2)^2-q_1^2 q_2^2} \biggr], \end{aligned} $$

(C.3)

where

$$ \delta_i^{\mu\nu} ( p^{2}, q_{1}^{2}, q_{2}^{2} ) =- 2i \int\frac{d^{4}k}{(2\pi)^{4}} \bigl[J^{\mu\nu}_{bc}+J^{\mu \nu}_{de}+J^{\mu\nu}_f+J^{\mu\nu}_g \bigr], $$

and different terms in brackets, J ^μν, correspond to the diagrams shown in Figs. 6, with lower indices being the symbol of the figure.

Below for simplicity, a momentum is denoted as a lower index and a quark-flavor index i is omitted:

Then, one has

(C.4)

where

and

$$ \begin{aligned} J^{\mu\nu}_\mathrm{de}&=\frac{f_2}{D_2D_3}\mathrm {f}^{(1)}_{13}(k_1+k_3)^{\mu} \\[2pt] &\quad{}\times\bigl(m_2k_3^{\nu} + m_3k_2^{\nu} -\mathrm{m}^{(1)}_{32}(k_2+k_3)^{\nu} \\[2pt] &\quad {}\times \bigl((k_3k_2)+m_3m_2\bigr) \bigr) +\frac{f_1}{D_1D_3}\mathrm{f}^{(1)}_{23}(k_2+k_3)^{\nu} \\[2pt] &\quad{}\times\bigl(m_3k_1^{\mu} +m_1k_3^{\mu}-\mathrm{m}^{(1)}_{13}(k_1+k_3)^{\mu} \\[2pt] &\quad {}\times \bigl((k_3k_1)+m_3m_1 \bigr) \bigr), \\[2pt] J^{\mu\nu}_\mathrm{f}&=\frac{f_1f_2}{D_1D_2}\bigl((k_1k_2)+m_1m_2 \bigr)m_d \bigl[(f_1+f_2) g^{\mu\nu} \mathrm{f}^{(1)}_{12} \\[2pt] &\quad {}+(k_1+k_3)^{\mu}(k_2+k_3)^{\nu} \bigl((f_1+f_2) \\[2pt] &\quad {}\times\bigl(\mathrm{f}^{(2)}_{231}+ \mathrm{f}^{(2)}_{132}\bigr) -\mathrm{f}^{(1)}_{13} \mathrm{f}^{(1)}_{23} \bigr) \bigr], \\[2pt] J^{\mu\nu}_\mathrm{g}&= -\frac{f_2m_2}{D_2} \bigl[g^{\mu\nu} \mathrm{f}^{(1)}_{12}+(k_1+k_3)^{\mu}(k_2+k_3)^{\nu} \mathrm{f}^{(2)}_{231}\bigr] \\[2pt] &\quad{}-\frac{f_1m_1}{D_1} \bigl[g^{\mu\nu}\mathrm {f}^{(1)}_{12}+(k_2+k_3)^{\nu}(k_1+k_3)^{\mu} \mathrm{f}^{(2)}_{132}\bigr] \\[2pt] &\quad{}+\frac{f_3m_3}{D_3} \mathrm{f}^{(1)}_{13} \mathrm{f}^{(1)}_{23}(2k_1+k_3)^{\mu}(2k_2+k_3)^{\nu}. \end{aligned} $$

(C.5)

Analytical expressions for the form factors $A_{i} ( p^{2};q_{1}^{2} ,q_{2}^{2} )$ and $B_{i}^{\prime}( p^{2};q_{1}^{2} ,q_{2}^{2} )$ in the case of special kinematics,¹¹ when one photon is real, $q_{1}^{2}=0$, and the virtuality of second photon is equal to the virtuality of meson $p_{2}^{2}=q_{2}^{2}$, can be obtained by expanding the quark-loop expressions, Eqs. (C.3), (C.4), (C.5), in $q_{1}^{2}$. The resulting expressions contain derivatives of the nonlocal function f(k ²) up to third order. These expressions are rather cumbersome and not presented here. Alternatively, one can calculate the form factors for small but nonzero $q_{1}^{2}$ and then take the limit numerically.

Appendix D: Local limit of γ ^∗ γ ^∗→S ^∗ amplitude

In the local model with constituent quark masses m _i , the triangle quark-loop diagrams, depicted in Figs. 6b–c, reduce to the following expression:

(D.1)

where $I_{\mathrm{g}}(m_{i}^{2})$ is a gauge non-invariant term (constant)

$$ \begin{aligned} &I_{\mathrm{g}}\bigl(m_i^2\bigr) = \frac{1}{2 \pi^2 } \int^{1}_{0}dx_1 \int^{1-x_1}_{0} dx_2 \frac{m_i^2-X}{m_i^2-X}=\frac{1}{4 \pi^2 } , \\[2pt] & X = x_1 (1-x_1-x_2) q_2^2+x_2 (1-x_1-x_2) q_1^2+x_1 x_2 p^2,\!\!\!\!\!\!\!\!\!\!\!\! \end{aligned} $$

(D.2)

which should be eliminated by suitable regularization, e.g., the Pauli–Villars regularization $I_{\mathrm{g}}(m_{i}^{2})-I_{\mathrm {g}}(\varLambda_{\mathrm{PV}}^{2})=0$, and the form factors read

$$ \begin{aligned} &A_{\mathrm{loc};i} \bigl( p^{2};q_{1}^{2} ,q_{2}^{2} \bigr) \\[2pt] &\quad =\frac{m_i}{4 (q_1 q_2)\pi^2 } \int^{1}_{0}dx_1\int ^{1-x_1}_{0} dx_2 \\[2pt] &\qquad{}\times\frac{4X-p^2 +q_2^2 (1 -2x_1)+q_1^2 (1 -2x_2)}{m_i^2-X}, \\[2pt] &B_{\mathrm{loc};i} \bigl( p^{2};q_{1}^{2} ,q_{2}^{2} \bigr) \\[2pt] &\quad =\frac{m_i}{(q_1 q_2)\pi^2 } \int^{1}_{0}dx_1\int^{1-x_1}_{0} dx_2 \frac{ x_2 (1 -2 x_2)}{q_2^2(m_i^2-X)}. \end{aligned} $$

(D.3)

Note that, if one takes the local limit of the nonlocal expression Eq. (C.3) by setting Λ→∞, the contribution of nonlocal diagrams completely cancel a gauge non-invariant term.

For special kinematics considered above, the form factors become $(\bar {x} = 1-x)$

$$ \begin{aligned} &A_{\mathrm{loc};i} \bigl( p^{2}; p^{2} ,0 \bigr) \\[2pt] &\quad =- \frac{m_i}{12 \pi^2 } \int^{1}_{0}dx \frac{2 m_i^2 - p^2 \bar{x} x (1+4x\bar{x} )}{(m_i^2-x\bar {x}p^2)^2} , \\[2pt] &B^\prime_{\mathrm{loc};i} \bigl( p^{2}; p^{2} ,0 \bigr)= -\frac{m_i}{6 \pi^2 }\int^{1}_{0}dx \frac{1-6x \bar{x}}{m_i^2-p^2x\bar{x}} . \end{aligned} $$

(D.4)

Appendix E: Tensor structures for LbL amplitude

Functions averaged over muon momenta can be represented as

(E.1)

where 〈A〉 _j are the averages of scalar products with muon momentum in the numerator and muon propagators in the denominator ($\mathrm{D}_{1}= (P+Q_{1} )^{2}+m_{\mu}^{2}$, $\mathrm{D}_{2}= (P-Q_{2} )^{2}+\nobreak m_{\mu}^{2}$)

$$ \begin{aligned} & \langle A \rangle_1= \biggl\langle\frac{1}{\mathrm{D}_{1}} \biggr \rangle=\frac{R_{1}-1}{2m_{\mu}^{2}},\qquad\langle A \rangle_2= \biggl\langle \frac{1}{\mathrm{D}_{2}} \biggr\rangle =\frac{R_{2}-1}{2m_{\mu}^{2}},\!\!\!\!\!\! \\ & \langle A \rangle_3 = \biggl\langle\frac{PQ_{2}}{\mathrm {D}_{1}} \biggr \rangle=(Q_{1}Q_{2})\frac{ ( 1-R_{1} )^{2}}{8m_{\mu}^{2}}, \\ & \langle A \rangle_4= \biggl\langle\frac{PQ_{1}}{\mathrm{D}_{2}} \biggr \rangle=-(Q_{1}Q_{2})\frac{ ( 1-R_{2} )^{2}}{8m_{\mu}^{2}}, \\ & \langle A \rangle_5= \biggl\langle\frac{1}{\mathrm{D}_{1} \mathrm{D}_{2}} \biggr \rangle=\frac{1}{M_{\mu}^{2}\vert Q_{1}\vert \vert Q_{2}\vert x}\arctan\biggl[ \frac{zx}{1-zt} \biggr] , \\ & \langle A \rangle_6= \langle1 \rangle=1, \end{aligned} $$

(E.2)

m _μ is the muon mass $( P^{2}=-m_{\mu}^{2} )$ and

$$ \begin{aligned} & t=\frac{(Q_{1}Q_{2})}{\vert Q_{1}\vert \vert Q_{2}\vert }, \qquad x=\sqrt{1-t^{2}}, \\ & R_{i}=\sqrt{1+\frac{4m_{\mu}^{2}}{Q_{i}^{2}}}, \qquad z=\frac{Q_{1}Q_{2}}{4m_{\mu}^{2} } ( 1-R_{1} ) ( 1-R_{2} ). \end{aligned} $$

(E.3)

$Z^{\mathrm{XY}}_{\mathbf{i},j}$ are polynomials in the photon momenta:

$$ \begin{aligned} &Z^{\mathrm{AA}}_{\mathbf{1},1}=(Q_1 Q_2) \bigl(Q_1^2+(Q_1 Q_2)\bigr) , \qquad \\ &Z^{\mathrm{AA}}_{\mathbf{1},2}=Q_2^2 \frac{3Q_1^2+Q_2^2+Q_3^2}{4} ,\qquad Z^{\mathrm{AA}}_{\mathbf{1},3}=-Q_1^2 ,\qquad \\ &Z^{\mathrm{AA}}_{\mathbf{1},4}=Q_2^2 ,\qquad Z^{\mathrm{AA}}_{\mathbf{1},6}=\frac{(Q_1 Q_2)-Q_2^2}{2} ,\qquad \\ &Z^{\mathrm{AA}}_{\mathbf{1},5}=Q_2^2 \bigl(2 m_{\mu}^{2}-Q_1^2-(Q_1 Q_2)\bigr) \bigl(Q_1^2+(Q_1 Q_2)\bigr), \end{aligned} $$

(E.4)

$$ \begin{aligned} &Z^{\mathrm{AA}}_{\mathbf{2},1}=\frac{Q_1^2(Q_1^2-2Q_3^2)}{2} ,\qquad Z^{\mathrm{AA}}_{\mathbf{2},2}= \frac{Q_2^2(Q_2^2-2Q_3^2)}{2} ,\!\!\!\!\!\!\!\!\!\!\!\! \\ &Z^{\mathrm{AA}}_{\mathbf{2},3}=-Q_1^2 ,\qquad Z^{\mathrm{AA}}_{\mathbf{2},4}= Q_2^2 , \\ &Z^{\mathrm{AA}}_{\mathbf{2},6}= Q_3^2- \frac{Q_1^2+Q_2^2}{2} , \\ &Z^{\mathrm{AA}}_{\mathbf{2},5}= Q_3^2 \bigl(Q_1^2 Q_2^2-2 m_{\mu}^{2} (Q_1 Q_2)\bigr) , \end{aligned} $$

(E.5)

$$ \begin{aligned} &Z^{\mathrm{AB}}_{\mathbf{1},1}=-Q_1^2 Q_2^2\bigl((Q_1 Q_2)+Q_1^2 \bigr) \frac{Q_3^2-Q_1^2}{2} , \\ &Z^{\mathrm{AB}}_{\mathbf{1},2}=-Q_2^2 \frac{(Q_1 Q_2)^2 (Q_2^2+(Q_1 Q_2))+Q_1^2 Q_2^2 Q_3^2}{2} , \\ &Z^{\mathrm{AB}}_{\mathbf{1},3}=-Q_1^2 (Q_1 Q_2) \bigl((Q_1 Q_2)+Q_2^2 \bigr) , \\ &Z^{\mathrm{AB}}_{\mathbf{1},4}= Q_2^2 (Q_1 Q_2) \bigl((Q_1 Q_2)+Q_2^2 \bigr) , \\ & Z^{\mathrm{AB}}_{\mathbf{1},5}= Q_1^2 Q_2^4 Q_3^2\frac{(Q_1 Q_2)+Q_1^2}{2} , \\ &Z^{\mathrm{AB}}_{\mathbf{1},6}= \bigl((Q_1 Q_2)^2+Q_1^2 Q_2^2\bigr)\frac{(Q_1 Q_2)+Q_2^2}{2} , \end{aligned} $$

(E.6)

$$ \begin{aligned} Z^{\mathrm{AB}}_{\mathbf{2},1}&=\frac{Q_1^2}{2} \bigl(Q_2^2 \bigl(Q_1^4 +(Q_1 Q_2) \bigl(Q_3^2-Q_1^2\bigr)\bigr) \\ &\quad{}+Q_1^2 \bigl((Q_1 Q_2) \bigl((Q_1 Q_2)+5 Q_2^2\bigr)+2 Q_2^4\bigr) \bigr) ,\quad \\ Z^{\mathrm{AB}}_{\mathbf{2},2}&=\frac{Q_2^2}{2} \bigl(Q_1^2 \bigl(Q_2^4 +(Q_1 Q_2) \bigl(Q_3^2-Q_2^2\bigr)\bigr) \\ &\quad{}+Q_2^2 \bigl((Q_1 Q_2) \bigl((Q_1 Q_2)+5 Q_1^2 \bigr)+2 Q_1^4\bigr) \bigr) , \\ Z^{\mathrm{AB}}_{\mathbf{2},3}&= Q_1^2 \bigl((Q_1 Q_2)+Q_1^2\bigr) \bigl((Q_1 Q_2)+Q_2^2\bigr) , \\ Z^{\mathrm{AB}}_{\mathbf{2},4}&=-Q_2^2 \bigl((Q_1 Q_2)+Q_1^2\bigr) \bigl((Q_1 Q_2)+Q_2^2\bigr) , \\ Z^{\mathrm{AB}}_{\mathbf{2},5}&=-Q_1^2 Q_2^2 Q_3^2 \frac{(Q_1 Q_2) (Q_2^2+Q_1^2)+2Q_1^2 Q_2^2}{2} , \\ Z^{\mathrm{AB}}_{\mathbf{2},6}&=\frac{(Q_1^2 Q_2^2-(Q_1 Q_2)^2) (Q_2^2+Q_1^2)}{2}-Q_1^2 Q_2^2 Q_3^2 . \end{aligned} $$

(E.7)