Skip to main content
SpringerLink
Log in

Symbolic computation of conservation laws and exact solutions of a coupled variable-coefficient modified Korteweg–de Vries system

  • Published:
Computational Mathematics and Mathematical Physics Aims and scope Submit manuscript

Abstract

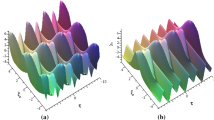
In this paper we study a generalized coupled variable-coefficient modified Korteweg–de Vries (CVCmKdV) system that models a two-layer fluid, which is applied to investigate the atmospheric and oceanic phenomena such as the atmospheric blockings, interactions between the atmosphere and ocean, oceanic circulations and hurricanes. The conservation laws of the CVCmKdV system are derived using the multiplier approach and a new conservation theorem. In addition to this, a similarity reduction and exact solutions with the aid of symbolic computation are computed.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. M. J. Ablowitz and P. A. Clarkson, Solitons, Nonlinear Evolution Equations, and Inverse Scattering (Cambridge Univ. Press, New York, 1991).

    Book  MATH  Google Scholar 

  2. R. Hirota, “Exact solution of the Korteweg–de Vries equation for multiple collisions of solitons,” Phys, Rev. Lett. 27, 1192–1194 1971.

    Article  MATH  Google Scholar 

  3. A. M. Wazwaz, “Exact solutions for the ZK-MEW equation by using the tanh and sine-cosine methods,” Int. J. Comput. Math. 82, 699–708 2005.

    Article  MathSciNet  MATH  Google Scholar 

  4. A. M. Wazwaz, “A study on KdV and Gardner equations with time-dependent coefficients and forcing terms,” Appl. Math. Comput. 217, 2277–2281 2010.

    MathSciNet  MATH  Google Scholar 

  5. J. Zhang, X. Wei, and J. Hou, “Symbolic computation of exact solutions for the compound KdV–Sawada–Kotera equation,” Int. J. Comput. Math. 87, 94–102 2010.

    Article  MathSciNet  MATH  Google Scholar 

  6. M. V. Demina, N. A. Kudryashov, and D. I. Sinel’shchikov, “The polygonal method for constructing exact solutions to certain nonlinear differential equations describing water waves,” Comput. Math. Math. Phys. 48, 2182–2193 2008.

    Article  MathSciNet  Google Scholar 

  7. L. Wei, “Exact soliton solutions for the general fifth Korteweg–de Vries equation,” Comput. Math. Math. Phys. 49 1429–1434 (2009).

    Article  MathSciNet  Google Scholar 

  8. S.-H. Zhu, Y.-T. Gao, X. Yu, Z.-Y. Sun, X.-L. Gai, and D.-X. Meng, “Painlevé property, soliton-like solutions and complexifications for a coupled variable-coefficient modified Korteweg–de Vries system in a two-layer fluid model,” Appl. Math. Comput. 217, 295–307 2010.

    MathSciNet  MATH  Google Scholar 

  9. S. Y. Lou and H. Y. Ruan, “Infinite conservation laws for the variable-coefficient KdV and MKdV equations,” Acta Phys. Sinica 41, 182 1992.

    MathSciNet  Google Scholar 

  10. X. L. Gai, Y. T. Gao, D. X. Meng, L. Wang, Z. Y. Sun, X. Lu, Q. Feng, M. Z. Wang, X. Yu, and S. H. Zhu, “Darboux transformation and soliton solutions for a variable coefficient modified Korteweg–de Vries model from ocean dynamics, fluid mechanics, and plasma physics,” Commun. Theor. Phys. 53, 673 2010.

    Article  MATH  Google Scholar 

  11. Z. Yan, “Symmetry reductions and soliton-like solutions for the variable coefficient MKdV equations,” Commun. Nonlinear Sci. Numer. Simul. 4, 284–288 1999.

    Article  MathSciNet  MATH  Google Scholar 

  12. R. J. Leveque, Numerical Methods for Conservation Laws (Birkhäuser, Basel, 1992).

    Book  MATH  Google Scholar 

  13. A. C. Newell, “The history of the soliton,” J. Appl. Mech. 50, 1127–1137 1983.

    Article  MathSciNet  MATH  Google Scholar 

  14. A. V. Mikhailov, A. B. Shabat, and R. I. Yamilov, “On an extension of the module of invertible transformations,” Sov. Math. Dokl. 36, 60–63 1988.

    MathSciNet  Google Scholar 

  15. A. V. Mikhailov, A. B. Shabat, and R. I. Yamilov, “Extension of the module of invertible transformations and classification of integrable systems,” Commun. Math. Phys. 115, 1–19 1988.

    Article  MathSciNet  MATH  Google Scholar 

  16. Y. Kodama and A. V. Mikhailov, “Obstacles to asymptotic integrability,” in Algebraic Aspects of Integrability, Ed. by I. M. Gelfand and A. Fokas (Birkhäuser, Basel, 1996), pp. 173–204.

    Google Scholar 

  17. S. C. Anco and G. W. Bluman, “Direct construction method for conservation laws of partial differential equations. Part I: Examples of conservation law classifications,” Eur. J. Appl. Math. 13, 545–566 2002.

    MathSciNet  MATH  Google Scholar 

  18. N. H. Ibragimov, “A new conservation theorem,” J. Math. Anal. Appl. 333, 311–328 2007.

    Article  MathSciNet  MATH  Google Scholar 

  19. N. H. Ibragimov, CRC Handbook of Lie Group Analysis of Differential Equations (CRC, Boca Raton, FL), Vols. 1–3.

  20. N. A. Kudryashov, “Exact solitary waves of the Fisher equation,” Phys. Lett. A 342, 99–106 2005.

    Article  MathSciNet  MATH  Google Scholar 

  21. N. A. Kudryashov, “Simplest equation method to look for exact solutions of nonlinear differential equations,” Chaos, Solitons Fractals 24, 1217–1231 2005.

    Article  MathSciNet  MATH  Google Scholar 

  22. N. A. Kudryashov, “One method for finding exact solutions of nonlinear differential equations,” Commun. Nonlinear Sci. Numer. Simul. 17, 2248–2253 2012.

    Article  MathSciNet  MATH  Google Scholar 

  23. N. K. Vitanov, “Application of simplest equations of Bernoulli and Riccati kind for obtaining exact travelingwave solutions for a class of PDEs with polynomial nonlinearity,” Commun. Nonlinear Sci. Numer. Simul. 15, 2050–2060 2010.

    Article  MathSciNet  MATH  Google Scholar 

  24. I. S. Gradshteyn and I. M. Ryzhik, Table of Integrals, Series, and Products, 7th ed. (Academic, New York, 2007).

    MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. International Institute for Symmetry Analysis and Mathematical Modelling, Department of Mathematical Sciences, North-West University, Mafikeng Campus, Private Bag X 2046, Mmabatho, 2735, Republic of South Africa

    Abdullahi Rashid Adem & Chaudry Masood Khalique

Authors
  1. Abdullahi Rashid Adem
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Chaudry Masood Khalique
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Abdullahi Rashid Adem.

Additional information

The article is published in the original.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Adem, A.R., Khalique, C.M. Symbolic computation of conservation laws and exact solutions of a coupled variable-coefficient modified Korteweg–de Vries system. Comput. Math. and Math. Phys. 56, 650–660 (2016). https://doi.org/10.1134/S0965542516040023

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1134/S0965542516040023

Keywords

Search

Navigation