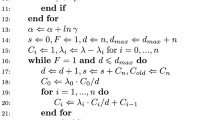Abstract
Reference analysis is an objective Bayesian approach to finding non-informative prior distributions. For various models involving nuisance parameters the reference prior has been shown to be superior to the multiparameter Jeffreys prior. In this article, the performance of the reference prior is evaluated for models that are defined at the extremes of parameter ranges, in which case reference and Jeffreys priors are shown to be potentially informative. Two quantities of interest are analyzed: the ratio of two multinomial parameters and the ratio of two independent binomial parameters, where the latter is just one example of inference based on the common 2 × 2 contingency table. For these two specific examples, we show that reference and/or Jeffreys priors lead to overinformative inference in extreme data situations that would seem common in, for example, many medical contexts when interest is in rare events. It is recommended that in the context of noninformative priors and binary data with extreme observed data, practitioners adopt priors for which prior predictive distributions are uniform, as per Bayes’s original argument.
Similar content being viewed by others
References
Abramowitz, M., and I. A. Stegun. 1964. Handbook of mathematical functions. New York, NY: Dover.
Agresti, A., and Y. Min. 2005. Frequentist performance of Bayesian confidence intervals for comparing proportions in 2 × 2 contingency tables. Biometrics 61:515–23.
Aitchison, J., and J. Bacon-Shone. 1981. Bayesian risk ratio analysis. American Statistician 35 (4):254–57.
Aitkin, M., R. J. Boys, and T. Chadwick. 2005. Bayesian point null hypothesis testing via the posterior likelihood ratio. Statistics and Computing 15:217–30.
Altham, P. M. E. 1969. Exact Bayesian analysis of a 2 × 2 contingency table, and Fisher’s “exact” significance test. Journal of the Royal Statistical Society, Series B 31 (2):261–69.
Angus, J. E., and R. E. Schafer. 1984. Improved onfidence statements for the binomial parameter. American Statistician 38 (3):189–91.
Bayes, T. R. 1763. An essay towards solving a problem in the doctrine of chances. Philosophical Transactions of the Royal Society, London 53:370–418.
Berger, J. O., B. Liseo, and R. L. Wolpert. 1999. Integrated likelihood methods for eliminating nuisance parameters. Statistical Science 14 (1):1–22.
Bernardo, J. M. 1979. Reference posterior distributions for Bayesian inference. Journal of the Royal Statistical Society, Series B 41:113–47 (with discussion).
Bernardo, J. M. 2005. Reference analysis. In Bayesian thinking: Modeling and computation, ed. D. K. Dey and C. R. Rao, 17–90. Amsterdam, The Netherlands: Elsevier.
Bernardo, J. M., and J. M. Ramon. 1998. An introduction to Bayesian reference analysis: Inference on the ratio of multinomial parameters. Statistician 47:101–35.
Bernardo, J. M., and A. F. M. Smith. 1994. Bayesian theory. New York, NY: Wiley.
Berry, G., and P. Armitage. 1995. Mid-P confidence intervals: A brief review. Statistician 44 (4):417–23.
Box, G. E. P., and G. C. Tiao. 1973. Bayesian inference in statistical analysis. New York, NY: Wiley Classics.
Brown, L. D., T. Cai, and A. DasGupta. 2001. Interval estimation for a binomial proportion. Statistical Science 16 (2):101–33 (with discussion).
Clopper, C. J., and E. S. Pearson. 1934. The use of confidence or fiducial limits illustrated in the case of the binomial. Biometrika 26:404–16.
Davison, A. C. 2003. Statistical models. Cambridge, UK: Cambridge University Press.
Dawid, A. P., N. Stone, and J. V. Zidek. 1973. Marginalization paradoxes in Bayesian and structural inference. Journal of the Royal Statistical Society, Series B 35:189–233 (with discussion).
Draper, N. R., W. G. Hunter, and D. E. Tierney. 1969. Which product is better? Technometrics 11 (2):309–20.
Edwards, A. W. F. 1978. Commentary on the arguments of Thomas Bayes. Scandinavian Journal of Statistics 5:116–18.
Geisser, S. 1984. On prior distributions for binary trials. American Statistician 38 (4):244–51.
Geweke, J. 2005. Contemporary Bayesian econometrics and statistics. Hoboken, NJ: Wiley.
Gottvall, K., C. Grunewald, and U. Waldenström. 2004. Safety of birth centre care: Perinatal mortality over a 10-year period. BJOG: An International Journal of Obstetrics and Gynaecology 111:71–78.
Gupta, R. C., R. A. Albanes, J. W. Penn, and T. J. White. 1997. Bayesian estimation of relative risk in biomedical research. Environmetrics 8:133–43.
Hartigan, J. A. 1983. Bayes theory. New York, NY: Springer-Verlag.
Hashemi, L., B. Nandram, and R. Goldberg. 1997. Bayesian analysis for a single 2 × 2 table. Statistics in Medicine 16:1311–28.
Huzurbazar, V. S. 1976. Sufficient statistics. New York, NY: Marcel Dekker.
Jeffreys, H. 1946. An invariant form for the prior probability in estimation problems. Proceedings of the Royal Society of London—Series A 186 (1007):453–61.
Jeffreys, H. 1961. Theory of probability, 3rd ed., Oxford, UK: Oxford University Press.
Jeffreys, H. 1980. Some general points in probability theory, In Bayesian analysis in econometrics and statistics, ed. A. Zellner, 451–54. Amsterdam, The Netherlands: North-Holland.
Jovanovic, B. D., and P. S. Levy. 1997. A look at the rule of three. American Statistician 51 (2):137–39.
Lindley, D. V. 1965. Introduction to probability and statistics from a bayesian viewpoint. New York, NY: Cambridge University Press.
Louis, T. A. 1981. Confidence intervals for a binomial parameter after observing no successes. American Statistician 35 (3):154.
Tebbs, J. M., R. B. Bilder, and B. K. Moser. 2001. An empirical Bayes group-testing approach to estimating small proportions. Communications in Statistics—Theory and Methods 32 (5):983–95.
Thomas, D. G., and J. J. Gart. 1977. A table of exact confidence limits for differences and ratios of two proportions and their odds ratios. Journal of the American Statistical Association 72 (357):73–76.
Tuyl, F., R. Gerlach, and K. Mengersen. 2008a. A comparison of Bayes–Laplace, Jeffreys, and other priors: the case of zero events. American Statistician 62 (1):40–44.
Tuyl, F., R. Gerlach, and K. Mengersen. 2008b. Inference for proportions in a 2 × 2 contingency table: HPD or not HPD? Biometrics 64 (4):1293–96.
Tuyl, F., R. Gerlach, and K. Mengersen. 2009a. Posterior predictive arguments in favor of the Bayes–Laplace prior as the consensus prior for binomial and multinomial parameters. Bayesian analysis 4 (1):151–58.
Tuyl, F., R. Gerlach, and K. Mengersen. 2009b. The rule of three, its variants and extensions. International Statistical Review 77 (2):266–75.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Tuyl, F., Gerlach, R. & Mengersen, K. Consensus priors for multinomial and binomial ratios. J Stat Theory Pract 10, 736–754 (2016). https://doi.org/10.1080/15598608.2016.1219684
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1080/15598608.2016.1219684