Skip to main content
SpringerLink
Log in

Stochastic response of a parametrically excited vibro-impact system with a nonzero offset constraint

  • Published:
International Journal of Dynamics and Control Aims and scope Submit manuscript

Abstract

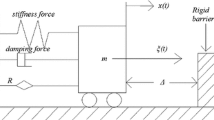
In this paper, a recently proposed solution procedure is extended in a straightforward manner to obtain the probability density function (PDF) of the stochastic response of a vibro-impact Duffing system with a nonzero offset constraint. The Duffing system is simultaneously excited by external and parametric Gaussian white noises and it undergoes repetitive instantaneous impacts against the constraint. First, in terms of the Zhuravlev non-smooth coordinate transformation, the original equation of motion and the impact condition are combined into a new equation without any constraints by adding an additional impulsive damping term. Second, the PDF of the new system is governed by the associated Fokker–Planck equation which is solved by the exponential-polynomial closure method. Last, the PDF of the original vibro-impact Duffing system is formulated in terms of the methodology on seeking the PDF distribution of a function of random variables. In order to evaluate the effectiveness of the proposed solution procedure, four illustrative examples are studied considering different values of the parameters, namely nonzero offset, nonlinear stiffness and parametric excitation intensity. A comparison with the direct energy balance method is also presented. Comparison with the simulation result shows that the proposed solution procedure can provide a satisfactory PDF solution, even in the tail region for the examined examples. In addition, the nonzero offset constraint significantly affects the PDF distribution of the response.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6

Similar content being viewed by others

References

  1. Ibrahim RA, Babitsky VI, Okuma M (2009) Vibro-impact dynamics of ocean systems, LNACM 44. Springer, Berlin

    Google Scholar 

  2. Ibrahim RA (2009) Vibro-impact dynamics: modeling, mapping and applications. Springer, Berlin

    Book  Google Scholar 

  3. Dimentberg MF, Iourtchenko DV (2004) Random vibrations with impacts: a review. Nonlinear Dyn 36:229–254

    Article  MathSciNet  MATH  Google Scholar 

  4. Langley RS (2012) The analysis of impact forces in randomly vibrating elastic systems. J Sound Vib 331:3738–3750

    Article  Google Scholar 

  5. McMillan AJ, Monroy Aceves C, Sutcliffe MPF (2012) Moderate energy impact analysis combining phenomenological contact law with localised damage and integral equation method. Int J Impact Eng 43:29–39

    Article  Google Scholar 

  6. Davies HG (1980) Random vibration of a beam impacting stops. J Sound Vib 68:479–487

    Article  MATH  Google Scholar 

  7. Baratta A (1990) Dynamics of a single-degree-of-freedom system with a unilateral obstacle. Struct Saf 8:181–194

    Article  Google Scholar 

  8. Jing HS, Sheu KC (1990) Exact stationary solutions of the random response of a single-degree-of-freedom vibro-impact system. J Sound Vib 141:363–373

    Article  MathSciNet  Google Scholar 

  9. Jing HS, Young M (1990) Random response of a single-degree-of-freedom vibro-impact system with clearance. Earthq Eng Struct Dyn 19:789–798

    Article  Google Scholar 

  10. Jing HS, Young M (1991) Impact interactions between two vibration systems under random excitation. Earthq Eng Struct Dyn 20:667–681

    Article  Google Scholar 

  11. Huang ZL, Liu ZH, Zhu WQ (2004) Stationary response of multi-degree-of-freedom vibro-impact systems under white noise excitations. J Sound Vib 275:223–240

    Article  Google Scholar 

  12. Sri Namachchivaya N, Park JH (2005) Stochastic dynamics of impact oscillators. J Appl Mech 72:862–870

    Article  MathSciNet  MATH  Google Scholar 

  13. Feng JQ, Xu W, Wang R (2008) Stochastic responses of vibro-impact duffing oscillator excited by additive Gaussian noise. J Sound Vib 309:730–738

    Article  Google Scholar 

  14. Feng JQ, Xu W, Rong HW, Wang R (2009) Stochastic responses of Duffing-Van der Pol vibro-impact system under additive and multiplicative random excitations. Int J Non-Linear Mech 44: 51–57

    Article  MATH  Google Scholar 

  15. Zhuravlev VF (1976) A method for analyzing vibration-impact systems by means of special functions. Mech Solids 11:23–27

    MathSciNet  Google Scholar 

  16. Li C, Xu W, Feng JQ, Wang L (2013) Response probability density functions of Duffing-Van der Pol vibro-impact system under correlated Gaussian white noise excitations. Phys A 392: 1269–1279

    Article  MathSciNet  Google Scholar 

  17. Xu W, Li C, Yue XL, Rong HW (2015) Stochastic responses of a vibro-impact system with additive and multiplicative colored noise excitations. Int J Dyn Control. doi:10.1007/s40435-014-0143-0

  18. Dimentberg MF, Iourtchenko DV (1999) Towards incorporating impact losses into random vibration analyses: a model problem. Probab Eng Mech 14:323–328

    Article  Google Scholar 

  19. Iourtchenko DV, Dimentberg MF (2001) Energy balance for random vibrations of piecewise-conservative systems. J Sound Vib 248:913–923

    Article  Google Scholar 

  20. Dimentberg M, Gaidai O, Naess A (2009) Random vibrations with inelastic impacts. In: Ibrahim RA, Babitsky VI, Okuma M (eds) Vibro-impact dynamics of ocean systems and related problems, LNACM 44. Springer, Berlin, pp 67–78

    Chapter  Google Scholar 

  21. Iourtchenko DV, Song LL (2006) Numerical investigation of a response probability density function of stochastic vibroimpact systems with inelastic impacts. Int J Non-Linear Mech 41:447–455

    Article  MATH  Google Scholar 

  22. Song LL (2006) Nonlinear random vibration of vibroimpact systems. Dissertation, University of Miami

  23. Thampi SK, Niedzwecki JM (1992) Parametric and external excitation of marine risers. J Eng Mech 118:942–960

    Article  Google Scholar 

  24. Cottone G, Di Paola M, Ihrahim R, Pirrotta A, Santoro R (2009) Ship roll motion under stochastic agencies using path integral method. In: Ibrahim RA, Babitsky VI, Okuma M (eds) Vibro-impact dynamics of ocean systems and related problems, LNACM 44. Springer, Berlin, pp 29–40

    Chapter  Google Scholar 

  25. Vasta M, Luongo A (2004) Dynamic analysis of linear and nonlinear oscillations of a beam under axial and transversal random Poisson pulses. Nonlinear Dyn 36:421–435

    Article  MATH  Google Scholar 

  26. Kovacic I, Brennan MJ (2011) The Duffing equation: nonlinear oscillators and their behaviour. John Wiley & Sons Ltd., West Sussex

    Book  MATH  Google Scholar 

  27. Zhu HT (2014) Stochastic response of vibro-impact Duffing oscillators under external and parametric Gaussian white noises. J Sound Vib 333:954–961

    Article  Google Scholar 

  28. Zhu HT (2014) Probabilistic solution of vibro-impact stochastic Duffing systems with a unilateral non-zero offset barrier. Phys A 410:335–344

    Article  MathSciNet  Google Scholar 

  29. Zhu HT (2014) Response of a vibro-impact Duffing system with a randomly varying damping term. Int J Non-Linear Mech 65:53–62

    Article  Google Scholar 

  30. Er GK (1998) An improved closure method for analysis of nonlinear stochastic systems. Nonlinear Dyn 17:285–297

    Article  MathSciNet  MATH  Google Scholar 

  31. Er GK (1999) A consistent method for the solution to reduced FPK equation in statistical mechanics. Phys A 262:118–128

    Article  Google Scholar 

  32. Er GK, Iu VP (1999) Probabilistic solutions to nonlinear random ship roll motion. J Eng Mech 125:570–574

    Article  Google Scholar 

  33. Zhu HT, Er GK, Iu VP, Kou KP (2010) Probability density function solution of nonlinear oscillators subjected to multiplicative Poisson pulse excitation on velocity. J Appl Mech 77:031001

    Article  Google Scholar 

  34. Lutes LD, Sarkani S (2004) Random vibrations: analysis of structural and mechanical systems. Elsevier, New York

    Google Scholar 

  35. Caughey TK (1963) Equivalent linearization techniques. J Acoust Soc Am 35:1706–1711

    Article  MathSciNet  Google Scholar 

  36. Spanos PD (1981) Stochastic linearization in structural dynamics. ASME Appl Mech Rev 34:1–8

    MathSciNet  Google Scholar 

  37. Roberts JB, Spanos PD (2003) Random vibration and statistical linearization. Dover Publications Inc., Mineola

    MATH  Google Scholar 

  38. Sun JQ (2006) Stochastic dynamics and control. Elsevier Science Ltd, Oxford

  39. Dimentberg MF, Menyailov AI (1979) Response of a single-mass vibroimpact system to white-noise random excitation. ZAMM-Z Angew Math Mech 59:709–716

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgments

The author would like to thank the financial supports from the National Basic Research Program of China (973 Program) under Grant No. 2013CB035904, the Programme of Introducing Talents of Discipline to Universities under Grant No. B14012, the National Natural Science Foundation of China under Grant No. 51478311, the Natural Science Foundation of Tianjin, China under Grant No. 14JCQNJC07400 and the Innovation Foundation of Tianjin University under Grant No. 60301014. The valuable and helpful suggestions from the anonymous reviewers are greatly acknowledged.

Author information

Authors and Affiliations

  1. State Key Laboratory of Hydraulic Engineering Simulation and Safety, Tianjin University, Tianjin, 300072, People’s Republic of China

    H. T. Zhu

Authors
  1. H. T. Zhu
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to H. T. Zhu.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Zhu, H.T. Stochastic response of a parametrically excited vibro-impact system with a nonzero offset constraint. Int. J. Dynam. Control 4, 180–194 (2016). https://doi.org/10.1007/s40435-015-0165-2

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s40435-015-0165-2

Keywords

Search

Navigation