Invading and Receding Sharp-Fronted Travelling Waves

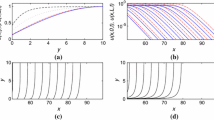
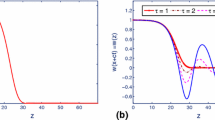
Abstract

Biological invasion, whereby populations of motile and proliferative individuals lead to moving fronts that invade vacant regions, is routinely studied using partial differential equation models based upon the classical Fisher–KPP equation. While the Fisher–KPP model and extensions have been successfully used to model a range of invasive phenomena, including ecological and cellular invasion, an often-overlooked limitation of the Fisher–KPP model is that it cannot be used to model biological recession where the spatial extent of the population decreases with time. In this work, we study the Fisher–Stefan model, which is a generalisation of the Fisher–KPP model obtained by reformulating the Fisher–KPP model as a moving boundary problem. The nondimensional Fisher–Stefan model involves just one parameter, $\kappa$, which relates the shape of the density front at the moving boundary to the speed of the associated travelling wave, c. Using numerical simulation, phase plane and perturbation analysis, we construct approximate solutions of the Fisher–Stefan model for both slowly invading and receding travelling waves, as well as for rapidly receding travelling waves. These approximations allow us to determine the relationship between c and $\kappa$ so that commonly reported experimental estimates of c can be used to provide estimates of the unknown parameter $\kappa$. Interestingly, when we reinterpret the Fisher–KPP model as a moving boundary problem, many overlooked features of the classical Fisher–KPP phase plane take on a new interpretation since travelling waves solutions with $c < 2$ are normally disregarded. This means that our analysis of the Fisher–Stefan model has both practical value and an inherent mathematical value.