Distributed space-time coding scheme with differential detection and power allocation for cooperative relay network

Abstract

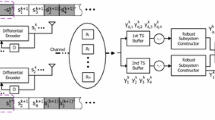
By introducing orthogonal space-time coding (STC) scheme in wireless cooperative relay network, two distributed differential STC (DSTC) schemes based on the amplify-and-forward (AF) and decode-and- forward (DF) methods are, respectively, developed. The scheme performance is investigated in symmetric and asymmetric wireless relay networks. The presented schemes require no channel information at both relay terminals and destination terminal, and have linear decoding complexity when compared with the existing scheme. Moreover, they are suitable for the application of different constellation modulations and DSTC schemes, and thus provide more freedoms of design. Besides, the power allocations between source and relay terminals are jointly optimized to minimize the system pairwise error probability for symmetric and asymmetric networks, and two practical methods are presented to solve the complicated optimized problem from asymmetric network. Simulation results show that the scheme with DF method has better performance than that with AF method due to no amplification of noise power, but the performance superiority will decrease at high SNR due to the error propagation of decoding at the relays. Furthermore, the distributed DSTC schemes with optimal power allocation have better performance than those with conventional fixed power allocation.

Appendices

Appendix 1

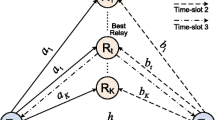
In this appendix, we will give the derivation of the optimized objective function in (19). For analysis simplicity, we consider two-relay cases (the extension to multi-relay cases will be straightforward).

By using the related results from [2] and [21], and averaging the above (18) on $\mathbf{x}_{i-1}$, the following inequality will be obtained.

$$\begin{aligned} \Pr \left( \mathbf{D}\rightarrow \mathbf{E}\right) \le \mathop E\limits _{\left\{ \beta _{ik} \right\} } \left\{ {{\det }^{-1}\left[ {\mathbf{I}_2 +E_s /\left( 4\kappa N_0 \right) \mathbf{BF}} \right] } \right\} \end{aligned}$$

(46)

where $\mathbf{F}=\hbox {diag}\{|\beta _{i1}|^{2},|\beta _{i2}|^{2}\},\,\mathbf{B}=\mathbf{G}^{H}{} \mathbf{PS}_{i-1}^H \mathbf{C}^{H}{} \mathbf{CS}_{i-1} \mathbf{PG}$. Since $\mathbf{B}=\mathbf{B}^{H}$, B is a Hermitian matrix. Let $\lambda _{v} (v=1,2)$ be the two eigenvalues of matrix B, then det $(\mathbf{B})=\lambda _{1}\lambda _{2}$ .Thus according to the analysis of [21], there exists an unitary matrix W such that $\mathbf{WBW}^{H}=\hbox {diag}\{\lambda _{1}, \lambda _{2}\}$. Moreover, $\lambda _{1}$ and $\lambda _{2}$ are nonnegative real values due to the fact that B is also a nonnegative-definite Hermitian matrix. Based on the analysis above, we will have:

$$\begin{aligned}&\det \left[ {\mathbf{I}_2 +E_s /(4\kappa N_0 )\mathbf{BF}} \right] \nonumber \\&\quad =\det \left[ {\mathbf{I}_2 +E_s /(4\kappa N_0 )\mathbf{W}^{H}\hbox {diag}\left\{ \lambda _1 ,\lambda _2 \right\} \mathbf{WF}} \right] \nonumber \\&\quad =\det \left[ {\mathbf{I}_2 +E_s /\left( 4\kappa N_0 \right) \hbox {diag}\left\{ \lambda _1 ,\lambda _2 \right\} \mathbf{Q}} \right] \end{aligned}$$

(47)

where $\mathbf{Q}=\mathbf{WFW}^{H}=[q_{11}\, q_{12};\,q_{21} \, q_{22}]$. Let $\mathbf{w}_{1}=[w_{11},\,w_{21}]^{T}$ and $\mathbf{w}_{2}=[w_{12},\,w_{22}]^{T}$ be two column vectors of W, then $q_{11}=|\beta _{i1}|^{2}|w_{11}|^{2}+|\beta _{i2}|^{2}|w_{12}|^{2}>0$, and $q_{22}=|\beta _{i1}|^{2}|w_{21}|^{2}+|\beta _{i2}|^{2}|w_{22}|^{2}>0$. Considering that $\lambda _{1},\,\lambda _{2}$ and $E_{s}/(4\kappa N_{0})$ are nonnegative, (47) can be changed to

$$\begin{aligned}&\det \left[ {\mathbf{I}_2 +E_s /(4\kappa N_0 )\mathbf{BF}} \right] \nonumber \\&\quad =\left[ 1+q_{11} \lambda _1 E_s /(4\kappa N_0 )\right] \left[ 1+q_{22} \lambda _2 E_s /(4\kappa N_0 )\right] \nonumber \\&\qquad -\,\lambda _1 \lambda _2 \left[ E_s /(4\kappa N_0 )\right] ^{2}q_{12} q_{21} \nonumber \\&\quad \ge q_{11} \lambda _1 E_s /(4\kappa N_0 )q_{22} \lambda _2 E_s /(4\kappa N_0 )\nonumber \\&\quad -\,\lambda _1 \lambda _2 \left[ E_s /(4\kappa N_0 )\right] ^{2}q_{12} q_{21} \nonumber \\&\quad =\lambda _1 \lambda _2 \left[ E_s /(4\kappa N_0 )\right] ^{2}\det (\mathbf{Q}) \end{aligned}$$

(48)

Substituting (48) into (46), the PEP is rewritten as

$$\begin{aligned} \Pr \left( \mathbf{D}\rightarrow \mathbf{E}\right)\le & {} \mathop {E}\limits _{\left\{ \beta _{ik} \right\} } \left\{ {\left[ \left( E_s /\left( 4N_0 \right) \right) ^{2}\lambda _1 \lambda _2 /\kappa ^{2}\right] ^{-1}\det ^{-1}(\mathbf{Q})} \right\} \nonumber \\= & {} \left( E_s /N_0 \right) ^{-2}\mathop {E}\limits _{\left\{ \beta _{ik}\right\} } \left\{ {\left[ 4^{-2}\lambda _1 \lambda _2 /\kappa ^{2}\right] ^{-1}\det ^{-1}(\mathbf{Q})} \right\} \nonumber \\ \end{aligned}$$

(49)

According to the definition of the diversity gain [24], using (49), we can evaluate the diversity gain $G_{d}$ as follows:

$$\begin{aligned} G_d =\mathop {\lim }\limits _{E_s /N_0 \rightarrow \infty } -\frac{\log \left( \Pr \left( \mathbf{D}\rightarrow \mathbf{E}\right) \right) }{\log (E_s /N_0 )}=2+0=2 \end{aligned}$$

(50)

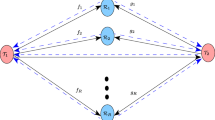
Hence, the system achieves full diversity order of 2 for two-relay case. Similarly, we can use the above analytical method to derive the diversity gain of the system with multiple relays. Namely, full diversity order of K will be obtained.

Besides, from (49), it is found that the PEP will be minimized when $\lambda _{1}\lambda _{2}/\kappa ^{2}$ (i.e. $\hbox {det}(\mathbf{B})/\kappa ^{2})$ is maximized. According to the definition of matrix G and B, we have:

$$\begin{aligned} \det (\mathbf{B})= & {} \det \left( \mathbf{G}^{H}{} \mathbf{PS}_{i-1}^H \mathbf{C}^{H}\mathbf{CS}_{i-1} \mathbf{PG}\right) \nonumber \\= & {} \det \left( \mathbf{G}^{H}{} \mathbf{G}\right) \det \left( \mathbf{P}^{2}\right) \det (\mathbf{C}^{H}\mathbf{C}) \nonumber \\= & {} \rho _{s1}^2 \rho _{s2}^2 \rho _{1d}^2 \rho _{2d}^2 \mu _1 \mu _2 P_0^2 P_1 P_2 \det \left( \mathbf{C}^{H}{} \mathbf{C}\right) \nonumber \\= & {} \zeta \mu _1 \mu _2 P_0^2 P_1 P_2 \det \left( \mathbf{C}^{H}\mathbf{C}\right) \end{aligned}$$

(51)

where $\zeta =\rho _{s1}^2 \rho _{s2}^2 \rho _{1d}^2 \rho _{2d}^2$, and $\mathbf{S}_{i-1}^{H}{} \mathbf{S}_{i-1}=\mathbf{I}_{2}$ is utilized. Since $\zeta$ and $\hbox {det}(\mathbf{C}^{H}{} \mathbf{C})$ are independent of the power optimization, we only need to optimize $\mu _1 \mu _2 P_0^2 P_1 P_2 /\kappa ^{2}$. Based on this, subject to the total power constraint, we may establish the optimized objective function shown as (19).

Appendix 2

In this appendix, we give the derivation of (29). With (28), setting $\partial \mathcal{L}/\partial P_v =0\,(v=0,1,2)$ yields

$$\begin{aligned} \frac{\partial \mathcal{L}}{\partial P_0 }= & {} \frac{2P_0 P_1 P_2 \left[ 2P_0^2 \rho _{s1}^2 \rho _{s2}^2 +3N_0 P_0 \left( \rho _{s1}^2 +\rho _{s2}^2 \right) +4N_0^2 \right] }{\left[ P_1 P_0 \rho _{1d}^2 \rho _{s2}^2 +P_2 P_0 \rho _{2d}^2 \rho _{s1}^2 +P_0^2 \rho _{s1}^2 \rho _{s2}^2 +2N_0 \left( P_1 \rho _{1d}^2 +P_2 \rho _{2d}^2 +P_0 \rho _{s1}^2 +P_0 \rho _{s2}^2 \right) +4N_0^2 \right] ^{2}} \nonumber \\&-\,\frac{2P_0^2 P_1 P_2 \left[ P_0^2 \rho _{s1}^2 \rho _{s2}^2 +2N_0 P_0 \left( \rho _{s1}^2 +\rho _{s2}^2 \right) +4N_0^2 \right] \left[ P_1 \rho _{1d}^2 \rho _{s2}^2 +P_2 \rho _{2d}^2 \rho _{s1}^2 +2P_0 \rho _{s1}^2 \rho _{s2}^2 +2N_0 \left( \rho _{s1}^2 +\rho _{s2}^2 \right) \right] }{\left[ P_1 P_0 \rho _{1d}^2 \rho _{s2}^2 +P_2 P_0 \rho _{2d}^2 \rho _{s1}^2 +P_0^2 \rho _{s1}^2 \rho _{s2}^2 +2N_0 \left( P_1 \rho _{1d}^2 +P_2 \rho _{2d}^2 +P_0 \rho _{s1}^2 +P_0 \rho _{s2}^2 \right) +4N_0^2 \right] ^{3}}-2\eta =0\nonumber \\ \end{aligned}$$

(52a)

$$\begin{aligned} \frac{\partial \mathcal{L}}{\partial P_1 }= & {} \frac{P_0^2 P_2 \left[ P_0^2 \rho _{s1}^2 \rho _{s2}^2 +2N_0 P_0 \left( \rho _{s1}^2 +\rho _{s2}^2 \right) +4N_0^2 \right] }{\left[ P_1 P_0 \rho _{1d}^2 \rho _{s2}^2 +P_2 P_0 \rho _{2d}^2 \rho _{s1}^2 +P_0^2 \rho _{s1}^2 \rho _{s2}^2 +2N_0 \left( P_1 \rho _{1d}^2 +P_2 \rho _{2d}^2 +P_0 \rho _{s1}^2 +P_0 \rho _{s2}^2 \right) +4N_0^2 \right] ^{2}} \nonumber \\&-\,\frac{2P_0^2 P_1 P_2 \left[ P_0^2 \rho _{s1}^2 \rho _{s2}^2 +2N_0 P_0 \left( \rho _{s1}^2 +\rho _{s2}^2 \right) +4N_0^2 \right] \left[ P_0 \rho _{1d}^2 \rho _{s2}^2 +2N_0 \rho _{1d}^2 )\right] }{\left[ P_1 P_0 \rho _{1d}^2 \rho _{s2}^2 +P_2 P_0 \rho _{2d}^2 \rho _{s1}^2 +P_0^2 \rho _{s1}^2 \rho _{s2}^2 +2N_0 \left( P_1 \rho _{1d}^2 +P_2 \rho _{2d}^2 +P_0 \rho _{s1}^2 +P_0 \rho _{s2}^2 \right) +4N_0^2 \right] ^{3}}-\eta =0 \end{aligned}$$

(52b)

$$\begin{aligned} \frac{\partial \mathcal{L}}{\partial P_2 }= & {} \frac{P_0^2 P_1 \left[ P_0^2 \rho _{s1}^2 \rho _{s2}^2 +2N_0 P_0 \left( \rho _{s1}^2 +\rho _{s2}^2 \right) +4N_0^2 \right] }{\left[ P_1 P_0 \rho _{1d}^2 \rho _{s2}^2 +P_2 P_0 \rho _{2d}^2 \rho _{s1}^2 +P_0^2 \rho _{s1}^2 \rho _{s2}^2 +2N_0 \left( P_1 \rho _{1d}^2 +P_2 \rho _{2d}^2 +P_0 \rho _{s1}^2 +P_0 \rho _{s2}^2 \right) +4N_0^2 \right] ^{2}} \nonumber \\&-\,\frac{2P_0^2 P_1 P_2 \left[ P_0^2 \rho _{s1}^2 \rho _{s2}^2 +2N_0 P_0 \left( \rho _{s1}^2 +\rho _{s2}^2 \right) +4N_0^2 \right] \left[ P_0 \rho _{2d}^2 \rho _{s1}^2 +2N_0 \rho _{2d}^2 )\right] }{\left[ P_1 P_0 \rho _{1d}^2 \rho _{s2}^2 +P_2 P_0 \rho _{2d}^2 \rho _{s1}^2 +P_0^2 \rho _{s1}^2 \rho _{s2}^2 +2N_0 \left( P_1 \rho _{1d}^2 +P_2 \rho _{2d}^2 +P_0 \rho _{s1}^2 +P_0 \rho _{s2}^2 \right) +4N_0^2 \right] ^{3}}-\eta =0 \end{aligned}$$

(52c)

Using Eqs. (52a) and (52b), we have:

$$\begin{aligned}&\left( P_0 \rho _{s1}^2 +2N_0 \right) \rho _{2d}^2 \left[ \left( \rho _{s1}^2 \rho _{s2}^2 P_0^2 +4N_0^2 \right) \left( P_0 -P_1 \right) \right. \nonumber \\&\qquad \left. +\,2P_0^2 N_0 \left( \rho _{s1}^2 +\rho _{s2}^2 \right) -P_0 P_1 N_0 \left( \rho _{s1}^2 +3\rho _{s2}^2 \right) \right] P_2 \nonumber \\&\quad =\left( P_0 \rho _{s2}^2 +2N_0 \right) \left[ \left( P_0 \rho _{s1}^2 +2N_0 \right) \left( \rho _{s2}^2 \rho _{1d}^2 P_0^2 \right. \right. \nonumber \\&\qquad \left. +\,\rho _{s1}^2 P_0 N_0 +\rho _{s2}^2 P_0 N_0 +2\rho _{1d}^2 P_0 N_0 +4N_0^2 \right) P_1 \nonumber \\&\qquad +\,\left( \rho _{s1}^2 \rho _{s2}^2 P_0^2 +\rho _{s2}^2 P_0 N_0 +3\rho _{s1}^2 P_0 N_0 +4N_0^2 \right) \rho _{1d}^2 P_1^2 \nonumber \\&\qquad \left. -\left( P_0 \rho _{s2}^2 +2N_0 \right) \left( P_0 \rho _{s1}^2 +2N_0 \right) ^{2}P_0 \right] \end{aligned}$$

(53)

With equations (52b) and (52c), we can further obtain:

$$\begin{aligned}&\left( P_0 \rho _{s1}^2 +2N_0 \right) \rho _{2d}^2 P_2^2 +\left[ 2N_0 P_0 \left( \rho _{s1}^2 +\rho _{s2}^2 \right) \right. \nonumber \\&\qquad +\,2N_0 P_1 \left( \rho _{2d}^2 -\rho _{1d}^2 \right) +P_0 P_1 \left( \rho _{s1}^2 \rho _{2d}^2 -\rho _{s2}^2 \rho _{1d}^2 \right) \nonumber \\&\qquad \left. +\,P_0^2 \rho _{s1}^2 \rho _{s2}^2 \right] P_2\nonumber \\&\quad =\left( P_0 \rho _{s2}^2 +2N_0 \right) \left( P_0 \rho _{s1}^2 +2N_0 +P_1 \rho _{1d}^2 \right) P_1 \end{aligned}$$

(54)

According to the power constraint condition $2P_{0}+P_{1}+P_{2}=P_{t}$, $P_{2}$ is rewritten as

$$\begin{aligned} P_2 =P_t -2P_0 -P_1 . \end{aligned}$$

(55)

Substituting (55) into (54) gives

$$\begin{aligned}&\left[ 2\left( \rho _{s1}^2 \rho _{2d}^2 +\rho _{s2}^2 \rho _{1d}^2 -\rho _{s1}^2 \rho _{s2}^2 \right) P_0^2 \right. \nonumber \\&\qquad +\,4P_0 N_0 \left( \rho _{1d}^2 +\rho _{2d}^2 -\rho _{s1}^2 -\rho _{s2}^2 \right) -2P_t N_0 \left( \rho _{1d}^2 +\rho _{2d}^2 \right) \nonumber \\&\quad \quad \left. -\,P_0 P_t \left( \rho _{s1}^2 \rho _{2d}^2 +\rho _{s2}^2 \rho _{1d}^2 \right) -8N_0^2 \right] P_1 =\left( P_0 \rho _{s1}^2 +2N_0 \right) \nonumber \\&\quad \left( -P_t +2P_0 \right) \left( P_0 \rho _{s2}^2 +P_t \rho _{2d}^2 -2P_0 \rho _{2d}^2 +2N_0 \right) \end{aligned}$$

(56)

Substituting (55) and (56) into (53) yields:

$$\begin{aligned}&\left( P_0 \rho _{s1}^2 +2N_0 \right) \left( P_0 \rho _{s2}^2 +2N_0 \right) \left( P_0 \rho _{s2}^2 +P_t \rho _{2d}^2 \right. \nonumber \\&\quad \left. -\,2P_0 \rho _{2d}^2 +2N_0 \right) \left( 4\rho _{s1}^2 \rho _{s2}^2 P_0^3 \right. \nonumber \\&\quad -\,8\rho _{s1}^2 \rho _{2d}^2 P_0^3 -8\rho _{s2}^2 \rho _{1d}^2 P_0^3 \nonumber \\&\quad +\,16\rho _{1d}^2 \rho _{2d}^2 P_0^3 +12\rho _{s1}^2 N_0 P_0^2 +12\rho _{s2}^2 N_0 P_0^2\nonumber \\&\quad +\,6\rho _{s1}^2 \rho _{2d}^2 P_t P_0^2 +6\rho _{s2}^2 \rho _{1d}^2 P_t P_0^2\nonumber \\&\quad -\,24\rho _{2d}^2 N_0 P_0^2 -24\rho _{1d}^2 N_0 P_0^2 \nonumber \\&\quad -\,24\rho _{1d}^2 \rho _{2d}^2 P_t P_0^2 -2\rho _{s1}^2 N_0 P_t P_0 -2\rho _{s2}^2 N_0 P_t P_0 \nonumber \\&\quad -\,\rho _{s1}^2 \rho _{2d}^2 P_t^2 P_0 -\rho _{s2}^2 \rho _{1d}^2 P_t^2 P_0 +20\rho _{2d}^2 N_0 P_t P_0 \nonumber \\&\quad +\,20\rho _{1d}^2 N_0 P_t P_0 +32N_0^2 P_0 +12\rho _{1d}^2 \rho _{2d}^2 P_t^2 P_0 \nonumber \\&\quad \left. -\,4\rho _{1d}^2 N_0 P_t^2-4\rho _{2d}^2 N_0 P_t^2 -8N_0^2 P_t -2\rho _{1d}^2 \rho _{2d}^2 P_t^3 \right) =0\nonumber \\ \end{aligned}$$

(57)

Considering that $P_{0},\,\rho _{s1}$, $\rho _{s2}$, and $N_{0}$ are positive, the first factor $(P_0 \rho _{s1}^2 +2N_0)$ and the second factor $(P_0 \rho _{s2}^2 +2N_0)$ as well as the third factor $(P_0 \rho _{s2}^2 +P_t \rho _{2d}^2 -2P_0 \rho _{2d}^2 +2N_0)$ are all not equal to zero, otherwise $P_{1}$ will become zero in terms of Eq. (56). Hence, (57) can be further simplified as (29).

Appendix 3

In this appendix, we will give the derivation of (31) and proof of the Shengjin’s Formula 4.

By means of variable transformation $x=t-b/(3a)$, the general cubic equation ${ ax}^{3}+{ bx}^{2}+{ cx}+d=0$ can be changed as

$$\begin{aligned} t^{3}+pt+q=0 \end{aligned}$$

(58)

where $p=({ 3ac-b}^{2})/(3a^{2}),\,q=(2b^{3}-9{ abc}+27a^{2}d)/(27a^{3})$, and $\Delta$ is given by (30). For $\Delta <0$, there exist three distinct real roots for cubic equation, so Eq. (58) will have three real roots accordingly. Based on this, we may employ the trigonometric method in [23] to obtain the three roots for Eq. (58). i.e.,

$$\begin{aligned} t_k= & {} 2\sqrt{-p/3}\cos \left( \frac{1}{3}\arccos \left( \frac{3q}{2p}\sqrt{\frac{-3}{p}}\right) -\frac{2\pi k}{3}\right) , \quad k=0,1,2\nonumber \\ \end{aligned}$$

(59)

Substituting $p=({ 3ac-b}^{2})/(3a^{2})$ and $q=(2b^{3}-9{ abc}+27a^{2}d)/(27a^{3})$ into (59) gives

$$\begin{aligned} t_k= & {} \frac{2\sqrt{b^{2}-3ac}}{3|a|}\cos \left( \frac{1}{3}\arccos \left( -\frac{2b^{3}-9abc+27a^{2}d}{2(b^{2}-3ac)^{3/2}a}|a|\right) -\frac{2\pi k}{3}\right) \nonumber \\ \end{aligned}$$

(60)

Considering $T=(2{ Ab}-3{ aB})/(2A^{3/2})$, $A=b^{2}-3{ ac}$, and $B={ bc}-9{ ad}$, we have:

$$\begin{aligned} T=\left( 2b^{3}-9abc+27a^{2}d\right) /\left[ 2\left( b^{2}-3ac\right) ^{3/2}\right] \end{aligned}$$

(61)

Substituting (61) and $A=b^{2}-3{ ac}$ as well as $x=t-b/(3a)$ into (60) yields final three roots:

$$\begin{aligned} x_k =\left[ -b-2\sqrt{A}\cos \left( \left( \theta -2\pi k\right) /3\right) \right] /(3a), \quad k=0,1,2\nonumber \\ \end{aligned}$$

(62)

where $\theta =\hbox {arccos}T$. This is the Shengjin’s Formula 4.

With (62), considering the constrain condition of $P_{0}$, $P_{0}$ can be expressed as (31).