Skip to main content
SpringerLink
Log in

Unconditional optimal error estimates of linearized backward Euler Galerkin FEMs for nonlinear Schrödinger-Helmholtz equations

  • Original Paper
  • Published:
Numerical Algorithms Aims and scope Submit manuscript

Abstract

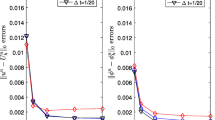
In this paper, we establish unconditionally optimal error estimates for linearized backward Euler Galerkin finite element methods (FEMs) applied to nonlinear Schrödinger-Helmholtz equations. By using the temporal-spatial error splitting techniques, we split the error between the exact solution and the numerical solution into two parts which are called the temporal error and the spatial error. First, by introducing a time-discrete system, we prove the uniform boundedness for the solution of this time-discrete system in some strong norms and derive error estimates in temporal direction. Second, by the above achievements, we obtain the boundedness of the numerical solution in \(L^{\infty }\)-norm. Then, the optimal L2 error estimates for r-order FEMs are derived without any restriction on the time step size. Numerical results in both two- and three-dimensional spaces are provided to illustrate the theoretical predictions and demonstrate the efficiency of the methods.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7

Similar content being viewed by others

References

  1. Adams, R.A., Fournier, J.J.: Sobolev Spaces. Academic press, New York (2003)

    MATH  Google Scholar 

  2. Akrivis, G.D., Dougalis, V.A., Karakashian, O.A.: On fully discrete Galerkin methods of second-order temporal accuracy for the nonlinear Schrödinger equation. Numer. Math. 59, 31–53 (1991)

    MathSciNet  MATH  Google Scholar 

  3. Antoine, X., Besse, C., Klein, P.: Absorbing boundary conditions for general nonlinear Schrödinger equations. SIAM J. Sci. Comput. 33, 1008–1033 (2011)

    MathSciNet  MATH  Google Scholar 

  4. Bao, W., Cai, Y.: Uniform error estimates of finite difference methods for the nonlinear Schrödinger equation with wave operator. SIAM J. Numer. Anal. 50, 492–521 (2012)

    MathSciNet  MATH  Google Scholar 

  5. Bao, W., Mauser, N.J., Stimming, H.P.: Effective one particle quantum dynamics of electrons: a numerical study of the Schrödinger-Poisson-Xα model. Commun. Math. Sci. 1, 809–828 (2003)

    MathSciNet  MATH  Google Scholar 

  6. Berezin, F.A., Shubin, M.A.: The Schrödinger Equation. Kluwer Academic Publishers, Dordrecht (1991)

    MATH  Google Scholar 

  7. Bohun, S., Illner, R., Lange, H., Zweifel, P.F.: Error estimates for Galerkin approximations to the periodic Schrödinger-Poisson system. Z.MM Z. Angew. Math. Mech. 76, 7–13 (1996)

    MATH  Google Scholar 

  8. Borz, A., Decker, E.: Analysis of a leap-frog pseudospectral scheme for the Schrödinger equation. J. Comput. Appl. Math. 193, 65–88 (2006)

    MathSciNet  MATH  Google Scholar 

  9. Bratsos, A.G.: A modified numerical scheme for the cubic Schrödinger equation. Numer. Methods Part Differ. Equ. 27, 608–620 (2011)

    MathSciNet  MATH  Google Scholar 

  10. Brenner, S., Scott, L.R.: The Mathematical Theory of Finite Element Methods. Springer, Berlin (1994)

    MATH  Google Scholar 

  11. Cao, Y., Musslimani, Z.H., Titi, E.S.: Nonlinear Schrödinger-Helmholtz equation as numercal regularization of the nonlinear Schrödinger equation. Nonlinearity 21, 879–898 (2008)

    MathSciNet  MATH  Google Scholar 

  12. Dehghan, M., Taleei, A.: Numerical solution of nonlinear Schrödinger equation by using time-space pseudo-spectral method. Numer. Methods Part DifferNumer. Methods Part Differ. Equ. 26, 979–990 (2010)

    MATH  Google Scholar 

  13. Douglas, J.J., Ewing, R.E., Wheeler, M.F.: A time-discretization procedure for a mixed finite element approximation of miscible displacement in porous media. RAIRO Anal. Numer. 17, 249–265 (1983)

    MathSciNet  MATH  Google Scholar 

  14. Ewing, R.E., Wheeler, M.F.: Galerkin methods for miscible displacement problems in porous media. SIAM J. Numer. Anal. 17, 351–365 (1980)

    MathSciNet  MATH  Google Scholar 

  15. Evans, L.C.: Partial Differential Equations, 2nd edn. AMS, Providence (2010)

    MATH  Google Scholar 

  16. Gao, H.: Optimal error estimates of a linearized backward Euler FEM for the Landau-Lifshitz equation. SIAM J. Numer. Anal. 52, 2574–2593 (2014)

    MathSciNet  MATH  Google Scholar 

  17. Gao, H., Li, B., Sun, W.: Optimal error estimates of linearized Crank-Nicolson Galerkin FEMs for the time-dependent Ginzburg-Landau equations in superconductivity. SIAM J. Numer. Anal. 52, 1183–1202 (2014)

    MathSciNet  MATH  Google Scholar 

  18. Harrison, R., Moroz, I., Tod, K.P.: A numerical study of the Schrödinger-Newton equations. Nonlinearity 16, 101–122 (2003)

    MathSciNet  MATH  Google Scholar 

  19. Hou, Y., Li, B., Sun, W.: Error estimates of splitting Galerkin methods for heat and sweat transport in textile materials. SIAM J. Numer. Anal. 51, 88–111 (2013)

    MathSciNet  MATH  Google Scholar 

  20. Hecht, F.: New development in Freefem++. J. Numer. Math. 20, 251–265 (2012)

    MathSciNet  MATH  Google Scholar 

  21. Heywood, J.G., Rannacher, R.: Finite element approximation of the nonstationary Navier-Stokes problem. Part IV: Error analysis for the second order time discretization. SIAM J. Numer. Anal. 2, 353–384 (1990)

    MATH  Google Scholar 

  22. Leo, M.D., Rial, D.: Well posedness and smoothing effect of Schrödinger-Poisson equation. J. Math. Phys. 48, 093509 (2007)

    MathSciNet  MATH  Google Scholar 

  23. Li, B., Sun, W.: Error analysis of linearized semi-implicit Galerkin finite element methods for nonlinear parabolic equations. Inter. J. Numer. Anal. Model. 10, 622–633 (2013)

    MathSciNet  MATH  Google Scholar 

  24. Li, B.: Mathematical Modeling, Analysis and Computation for Some Complex and Nonlinear Flow Problems. PhD Thesis. City University of Hong Kong, Hong Kong (2012)

    Google Scholar 

  25. Li, B., Sun, W.: Unconditional convergence and optimal error estimates of a Galerkin-mixed FEM for incompressible miscible flow in porous media. SIAM J. Numer. Anal. 51, 1959–1977 (2013)

    MathSciNet  MATH  Google Scholar 

  26. Li, B., Gao, H., Sun, W.W.: Unconditionally optimal error estimates of a Crank-Nicolson Galerkin method for the nonlinear thermistor equations. SIAM J. Numer. Anal. 52, 933–954 (2014)

    MathSciNet  MATH  Google Scholar 

  27. Lu, T., Cai, W.: A Fourier spectral-discontinuous Galerkin method for time-dependent 3-D Schrödinger-Poisson equations with discontinuous potentials. J. Comput. Appl. Math. 220, 588–614 (2008)

    MathSciNet  MATH  Google Scholar 

  28. Lubich, C.: On splitting methods for Schrödinger-Poisson and cubic nonlinear Schrödinger equations. Math. Comput. 77, 2141–2153 (2008)

    MATH  Google Scholar 

  29. Liao, H., Sun, Z., Shi, H.: Error estimate of fourth-order compact scheme for linear Schrödinger equations. SIAM J. Numer Anal. 47, 4381–4401 (2010)

    MathSciNet  MATH  Google Scholar 

  30. Masaki, S.: Energy solution to a Schrödinger-Poisson system in the two-dimensional whole space. SIAM J. Math. Anal. 43, 2719–2731 (2011)

    MathSciNet  MATH  Google Scholar 

  31. Mu, M., Huang, Y.: An alternating Crank-Nicolson method for decoupling the Ginzburg-Landau equations. SIAM J. Numer. Anal. 35, 1740–1761 (1998)

    MathSciNet  MATH  Google Scholar 

  32. Nirenberg, L.: An extended interpolation inequality. Ann. Scuola Norm. Sup. Pisa (3) 20, 733–737 (1966)

    MathSciNet  MATH  Google Scholar 

  33. Pathria, D.: Exact solutions for a generalized nonlinear Schrödinger equation. Phys. Scr. 39, 673–679 (1989)

    Google Scholar 

  34. Pelinovsky, D.E., Afanasjev, V.V., Kivshar, Y.S.: Nonlinear theory of oscillating, decaying, and collapsing solitons in the generalized nonlinear Schrödinger equation. Phys. Rev. E 53, 1940–1953 (1996)

    Google Scholar 

  35. Reichel, B., Leble, S.: On convergence and stability of a numerical scheme of coupled nonlinear Schrödinger equations. Comput. Math. Appl. 55, 745–759 (2008)

    MathSciNet  MATH  Google Scholar 

  36. Sanz-Serna, J.M.: Methods for the numerical solution of nonlinear Schrödinger equation. Math. Comput. 43, 21–27 (1984)

    MATH  Google Scholar 

  37. Stimming, H.P.: The IVP for the Schrödinger-Poisson-Xα equation in one dimension. Math. Models Methods Appl. Sci. 8, 1169–1180 (2005)

    MathSciNet  MATH  Google Scholar 

  38. Sulem, C., Sulem, P.L.: The Nonlinear Schrödinger Equation: Self-Focusing and Wave Collapse. Springer, New York (1999)

    MATH  Google Scholar 

  39. Sun, W., Wang, J.: Optimal error analysis of Crank-Nicolson schemes for a coupled nonlinear Schrödinger system in 3D. J. Comput. Appl. Math. 317, 685–699 (2017)

    MathSciNet  MATH  Google Scholar 

  40. Sun, Z., Zhao, D.: On the \(L^{\infty }\) convergence of a difference scheme for coupled nonlinear Schrödinger. Comput. Math. Appl. 59, 3286–3300 (2010)

    MathSciNet  MATH  Google Scholar 

  41. Thomee, V.: Galerkin Finite Element Methods for Parabolic Problems. Springer, Berlin (2006)

    MATH  Google Scholar 

  42. Tourigny, Y.: Optimal H1 estimates for two time-discrete Galerkin approximations of a nonlinear Schrödinger equation. IMA J. Numer. Anal. 11, 509–523 (1991)

    MathSciNet  MATH  Google Scholar 

  43. Wang, J.: A new error analysis of Crank-Nicolson Galerkin FEMs for a generalized nonlinear Schrödinger equation. J. Sci. Comput. 60, 390–407 (2014)

    MathSciNet  MATH  Google Scholar 

  44. Zhang, Y., Dong, X.: On the computation of ground state and dynamics of Schrödinger-Poisson-Slater system. J. Comput. Phys. 220, 2660–2676 (2011)

    MATH  Google Scholar 

  45. Zouraris, G.E.: On the convergence of a linear two-step finite element method for the nonlinear Schrödinger equation. M2AN Math. Model. Numer. Anal. 35, 389–405 (2001)

    MathSciNet  MATH  Google Scholar 

Download references

Funding

This work was supported by the Natural Science Foundation of China (NSFC) under grants 11871393 and 61663043, the key project of the International Science and Technology Cooperation Program of Shaanxi Research & Development Plan (2019KWZ-08), and the Doctoral Foundation of Yunnan Normal University (No. 00800205020503093) and the Scientific Research Program Funded by Yunnan Provincial Education Department under grant no. 2019J0076.

Author information

Authors and Affiliations

  1. Department of Mathematics, Yunnan Normal University, Kunming, 650500, People’s Republic of China

    Yun-Bo Yang

  2. School of Mathematics and Statistics, Xi’an Jiaotong University, Xi’an, 710049, People’s Republic of China

    Yao-Lin Jiang

Authors
  1. Yun-Bo Yang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Yao-Lin Jiang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Yun-Bo Yang.

Additional information

Publisher’s note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Yang, YB., Jiang, YL. Unconditional optimal error estimates of linearized backward Euler Galerkin FEMs for nonlinear Schrödinger-Helmholtz equations. Numer Algor 86, 1495–1522 (2021). https://doi.org/10.1007/s11075-020-00942-5

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11075-020-00942-5

Keywords

Mathematics Subject Classification (2010)

Search

Navigation