Skip to main content
SpringerLink
Log in

Pulses and snakes in Ginzburg–Landau equation

  • Original Paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

An Erratum to this article was published on 09 October 2014

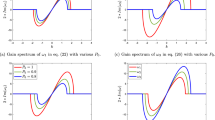
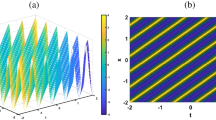
Abstract

Using a variational formulation for partial differential equations combined with numerical simulations on ordinary differential equations (ODEs), we find two categories (pulses and snakes) of dissipative solitons, and analyze the dependence of both their shape and stability on the physical parameters of the cubic-quintic Ginzburg–Landau equation (CGLE). In contrast to the regular solitary waves investigated in numerous integrable and non-integrable systems over the last three decades, these dissipative solitons are not stationary in time. Rather, they are spatially confined pulse-type structures whose envelopes exhibit complicated temporal dynamics. Numerical simulations reveal very interesting bifurcations sequences as the parameters of the CGLE are varied. Our predictions on the variation of the soliton amplitude, width, position, speed and phase of the solutions using the variational formulation agree with simulation results. Firstly, we develop a variational formalism which explores the various classes of dissipative solitons. Given the complex dynamics, the trial functions have been generalized considerably over conventional ones to keep the shape relatively simple, and the trial function integrable while allowing arbitrary temporal variation of the amplitude, width, position, speed and phase of the pulses and snakes. In addition, the resulting Euler–Lagrange (EL) equations from the variational formulation are treated in a completely novel way. Rather than considering the stable fixed points which correspond to the well-known stationary solitons, we use dynamical systems theory to focus on more complex attractors, viz. periodic (pulses) and quasiperiodic (snakes). Periodic evolution of the trial function parameters on stable periodic attractors yields solitons whose amplitudes and widths are non-stationary or time dependent. Secondly, we investigate the dissipative solitons of the CGLE and analyze its qualitative behavior by using numerical methods for ODEs. To solve numerically the nonlinear systems of ODEs that represent EL equations obtained from variational technique, we use an explicit Runge–Kutta fourth-order method. Finally, we elucidate the Hopf bifurcation mechanism responsible for the various pulsating solitary waves, as well as its absence in Hamiltonian and integrable systems where such structures are absent due to the lack of dissipation.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13

Similar content being viewed by others

References

  1. Akhmediev, N., Ankiewicz, A.: Dissipative Solitons in the CGLE and Swift-Hohenberg Equations. Dissipative Solitons. Springer, Berlin (2005)

    Google Scholar 

  2. Akhmediev, N., Soto-Crespo, J., Grelu, P.: Spatiotemporal optical solitons in nonlinear dissipative media: from stationary light bullets to pulsating complexes. Chaos 17, 037112 (2007)

  3. Akhmediev, N., Soto-Crespo, J.M., Town, G.: Pulsating solitons, chaotic solitons, period doubling, and pulse coexistence in mode-locked laser: CGLE approach. Phys. Rev. E 63, 056602 (2001)

  4. Alvarez, R., van Hecke, M., van Saarloos, W.: Sources and sinks separating domains of left-and right-traveling waves: experiment versus amplitude equations. Phys. Rev. E 56, R1306 (1997)

    Article  Google Scholar 

  5. Aranson, I., Kramer, L.: The world of the complex Ginzburg–Landau equation. Rev. Mod. Phys. 74, 99 (2002)

    Article  MATH  MathSciNet  Google Scholar 

  6. Artigas, D., Torner, L., Akhmediev, N.: Robust heteroclinic cycles in the one-dimension CGLE. Opt. Commun. 143, 322 (1997)

    Article  Google Scholar 

  7. Balmforth, N.: Solitary waves and homoclinic orbits. Ann. Rev. Fluid Mech. 27, 335 (1995)

    Article  MathSciNet  Google Scholar 

  8. Bowman, C., Newell, A.: Natural patterns and wavelets. Rev. Mod. Phys. 70, 289 (1998)

    Article  Google Scholar 

  9. Brusch, L., Torcini, A., Bär, M.: Nonlinear analysis of the Eckhaus instability: modulated amplitude waves and phase chaos. Phys. D 160, 127 (2001)

    Article  MATH  MathSciNet  Google Scholar 

  10. Brusch, L., Torcini, A., van Hecke, M., Zimmermann, M., Bär, M.: Modulated amplitude waves and defect formation in the one-dimensional complex Ginzburg–Landau equation. Phys. D 160, 127 (2001)

    Article  MATH  MathSciNet  Google Scholar 

  11. Cao, Y., Chung, K., Xu, J.: A novel construction of homoclinic and heteroclinic orbits in nonlinear oscillators by a perturbation-incremental method. Nonlinear Dyn. 64, 221 (2011)

    Article  MathSciNet  Google Scholar 

  12. Dai, C.Q., Zhang, J.F.: Controllable dynamical behaviors for spatiotemporal bright solitons on continuous wave background. Nonlinear Dyn. 73, 2049 (2013)

    Article  MathSciNet  Google Scholar 

  13. Dodd, R., Eilbeck, J., Gibbon, J., Morris, H.: Solitons and Nonlinear Wave Equations. Academic, London (1982)

    MATH  Google Scholar 

  14. Doelman, A.: Slow time-periodic solutions of the GL equation. Phys. D 40, 156 (1989)

    Article  MATH  MathSciNet  Google Scholar 

  15. Doelman, A.: Periodic and quasiperiodic solutions of degenerate modulation equations. Phys. D 53, 249 (1991)

    Article  MATH  MathSciNet  Google Scholar 

  16. Doelman, A.: Traveling waves in the complex GL equation. J. Nonlinear Sci. 3, 225 (1993)

    Article  MATH  MathSciNet  Google Scholar 

  17. Drazin, P., Johnson, R.S.: Solitons: An Introduction. Cambridge University Press, Cambridge (1989)

    Book  MATH  Google Scholar 

  18. Drazin, P., Reid, W.: Hydrodynamic Stability. Cambridge University Press, Cambridge (1981)

    MATH  Google Scholar 

  19. Duan, J., Holmes, P.: Fronts, domain walls and pulses in a generalized GL equation. Proc. Edinb. Math. Soc. 38, 77 (1995)

    Article  MATH  MathSciNet  Google Scholar 

  20. El-Wakil, S., Abulwafa, E., Zahran, M., Mahmoud, A.: Time-fractional KdV equation: formulation and solution using variational methods. Nonlinear Dyn. 64, 221 (2011)

  21. Fadeev, L., Takhtajan, L.: Hamiltonian Methods in the Theory of Solitons. Springer, Berlin (1986)

    Google Scholar 

  22. Holmes, P.: Spatial structure of time periodic solutions of the GL equation. Phys. D 23, 84 (1986)

    Article  MATH  MathSciNet  Google Scholar 

  23. Holodniok, M., Kubicek, M.: Computation of Period Doubling Points in ODEs. Institut für Mathematik Report TUM-8406, Technic Univ. of München, Germany (1984)

  24. Kaup, D., Malomed, B.: The variational principle for nonlinear waves in dissipative systems. Phys. D 87, 155 (1995)

    Article  MATH  MathSciNet  Google Scholar 

  25. Kaup, D., Malomed, B.: Embedded solitons in Lagrangian and semi-Lagrangian systems. Phys. D 184, 153 (2003)

    Article  MATH  MathSciNet  Google Scholar 

  26. Kaup, D., Vogel, T.K.: Quantitative measurement of variational approximations. Phys. Lett. A 362, 289 (2007)

    Article  MATH  Google Scholar 

  27. Kaup, D., Yang, J.: Stability and evolution of solitary waves in perturbed generalized nonlinear Schrödinger equations. SIAM J. Appl. Math. 60, 967 (2000)

  28. Keefe, L.: Dynamics of perturbed wavetrain solutions to the Ginzburg–Landau equation. Stud. Appl. Math. 73, 91 (1985)

    MATH  MathSciNet  Google Scholar 

  29. Landman, M.: Solutions of the GL equation of interest in shear flow. Stud. Appl. Math. 76, 187 (1987)

    MATH  MathSciNet  Google Scholar 

  30. Mancas, S., Choudhury, S.: Bifurcations and competing coherent structures in the cubic-quintic Ginzburg–Landau equation. I. Plane wave (CW) solutions. Chaos Solitons Fractals 27, 1256 (2006)

    Article  MATH  MathSciNet  Google Scholar 

  31. Mancas, S., Choudhury, S.R.: A novel variational approach to pulsating solitons in the cubic-quintic Ginzburg–Landau equation. Theor. Math. Phys. 152, 1160 (2007)

    Article  MATH  MathSciNet  Google Scholar 

  32. Mancas, S., Choudhury, S.R.: A novel variational approach to pulsating solitons in the cubic-quintic Ginzburg-Landau equation. Teoreticheskaya i Matematicheskaya Fizika 152, 339 (2007)

    Article  MathSciNet  Google Scholar 

  33. Murray, J.: Mathematical Biology. Springer, Berlin (1989)

    Book  MATH  Google Scholar 

  34. Nayfeh, A., Balachandran, B.: Applied Nonlinear Dynamics. Wiley, New York (1995)

    Book  MATH  Google Scholar 

  35. Newton, P., Sirovich, L.: Instabilities of the Ginzburg–Landau equation: periodic solutions. Q. Appl. Math. XLIV, 49 (1984)

  36. Newton, P., Sirovich, L.: Instabilities of the Ginzburg–Landau equation Pt.II: secondary bifurcations. Q. Appl. Math. XLIV, 367 (1986)

  37. Nohara, B.T.: A note on the two-dimensional Ginzburg–Landau equation for directional. Nearly monochromatic waves. Nonlinear Dyn. 33, 431 (2003)

    Article  MATH  MathSciNet  Google Scholar 

  38. Satsuma, J., Yajima, N.: Initial-value problems of one-dimensional self-modulation of nonlinear-waves in dispersive media. Prog. Theor. Phys. Suppl. 55, 284 (1974)

    Article  MathSciNet  Google Scholar 

  39. Soto-Crespo, J., Akhmediev, N., Ankiewicz, A.: Pulsating, creeping, and erupting solitons in dissipative systems. Phys. Rev. Lett. 85, 2937 (2000)

  40. Soto-Crespo, J., Akhmediev, N., Town, G.: Interrelation between various branches of stable solitons in dissipative systems. Opt. Commun. 199, 283 (2001)

    Article  Google Scholar 

  41. Soto-Crespo, J., Akhmediev, N., Mejia-Cortes, C., Devine, N.: Dissipative ring solitons with vorticity. Opt. Express 17, 4236 (2009)

    Article  Google Scholar 

  42. van Hecke, M., Storm, C., van Saarloos, W.: Sources, sinks and wave number selection in coupled CGL equations. Phys. D 134, 1 (1999)

  43. van Saarloos, W., Hohenberg, P.: Fronts, pulses, sources and sinks in generalized complex Ginzburg–Landau equation. Phys. D 56, 303 (1992)

  44. Williamson, J.: Low-storage Runge–Kutta schemes. J. Comput. Phys. 35, 48 (1980)

  45. Yang, J., Camassa, R., Kaup, D.: Private communication. In: 4th IMACS Conference on Nonlinear Waves. Athens, Georgia (2005)

Download references

Acknowledgments

We would like to acknowledge extremely insightful comments by David Kaup on the variational formulation, as well as on soliton perturbation theory. Helpful inputs were also provided by Jianke Yang, Roberto Camassa [45], and Harihar Khanal on the implementation of the RK4 method.

Author information

Authors and Affiliations

  1. Department of Mathematics, Embry-Riddle Aeronautical University, Daytona Beach, FL, 32114, USA

    Stefan C. Mancas

  2. Department of Mathematics, University of Central Florida, Orlando, FL , 32816, USA

    Roy S. Choudhury

Authors
  1. Stefan C. Mancas
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Roy S. Choudhury
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Stefan C. Mancas.

Appendix

Appendix

  1. 1.

    For the pulsating solitons, the nonlinear functions \(f_i,\,i=1,2,3 \) from (3.16) are shown in Fig. 13.

  2. 2.

    For the snaking solitons, the nonlinear functions \(f_i,\,i=4,5,6\) from (3.19) are shown in Fig. 14.

Fig. 14
figure 14

Nonlinear functions \(f_i,\,i=4,5,6\) from (3.19)

Full size image

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Mancas, S.C., Choudhury, R.S. Pulses and snakes in Ginzburg–Landau equation. Nonlinear Dyn 79, 549–571 (2015). https://doi.org/10.1007/s11071-014-1686-5

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-014-1686-5

Keywords

Search

Navigation